RBSE Class 11 Economics Notes Chapter 5 Measures of Central Tendency

These comprehensive RBSE Class 11 Economics Notes Chapter 5 Measures of Central Tendency will give a brief overview of all the concepts.

RBSE Class 11 Economics Chapter 5 Notes Measures of Central Tendency

Central Tendency:
Arithmetic mean is the sum total of all the observations divided by the number of observations. 

Commonly Used Averages 

• Arithmetic Mean
• Median
• Mode

Properties of an Ideal Average

• Easy to understand
• Easy to compute
• Represent the whole series
• Not affected by the extreme values
• Simple, definite and absolute

The relationship between mean, median and mode can be represented with the help of the following i formula:
Mode = 3Median - 2Mean
Mo = 3Me - 2X̄

Relationship between X̄, Me and Mo on the Basis of Distribution

• In symmetric distribution, X̄ = Me = Mo.
• In asymmetric distribution, Mo < Me < X̄ if the distribution is skewed to the right and X̄ < Me < Mo if the distribution is skewed to the left.

Arithmetic Mean:
Arithmetic mean is the sum total of all the observations divided by the number of observations.
Mean is denoted by X.

Arithmetic mean is given by
X̄ = \(\frac{X_1+X_2+X_3+\ldots+X_N}{N}\)
= \(\frac{\sum X}{N}\)

Combined arithmetic mean for two series is given by
X₁₂ = \(\frac{\bar{X}_1 N_1+\bar{X}_2 N_2}{N_1-N_2}\)
The sum of deviations of items about arithmetic mean is always equal to zero.

Types of Arithmetic Mean

• Simple arithmetic mean
• Weighted arithmetic mean

Weighted arithmetic mean is the mean calculated on the basis of weights assigned to the various items, according to their relative importance.

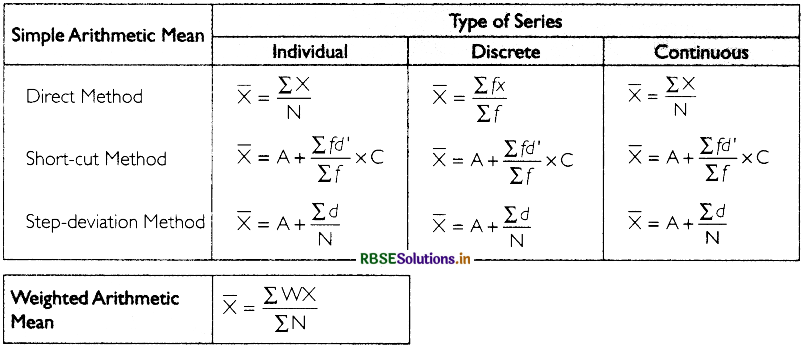
Formulae for Calculating Arithmetic Mean:

Median:

• Median is the measure of central tendency that divides the distribution into two equal parts.
• One part comprises of all the items less than or equal the median; while the second part comprises of all the items greater than or equal the median.
• For calculating median, the data must be arranged in descending or ascending order.
• When the number of values is odd, median is the middle most value.
• When the number of values is even, median is the arithmetic mean of the two middle values. 

The position of the median is to be determined to calculate the median. The position of the median can be calculated as:
Position of Mean = \(\frac{(N+1)}{2}\) th item

In continuous series, the formula for calculating median is:
Me = L₁ + \(\frac{\frac{N}{2}-C . F .}{f} \)× i

Partition Values
(i) Quartiles divide the distribution into four equal parts.

• First Quartile (Q₁) has 25% of the items below it.
  Position of Q₁ = \(\frac{(\mathrm{N}+1)}{4}\)th item
• Second Quartile (Q₂)or median has 50% of items below it.
• Third Quartile (Q₃) has 75% of items below it.
  Position of Q₃ = \(\frac{3(\mathrm{N}+1)}{4}\) th item

(ii) Deciles divide the distribution into 10 equal parts.
(iii) Percentiles divide the distribution into 100 equal parts.

Mode:
Mode is the value with the highest frequency in the distribution.
Types of Modal Data

• Uni-modal Data - data with unique mode
• Bi-modal Data - data with two modes
• Multi-modal Data - data with more than two modes Modal class is the class with largest frequency.

In continuous series, the formula for calculating mode is:
Mo = L₁ + \(\frac{f_1-f_0}{2 f_1-f_0-f_2}\) × i