RBSE Solutions for Class 11 Economics Chapter 6 Measures of Dispersion

Rajasthan Board RBSE Solutions for Class 11 Economics Chapter 6 Measures of Dispersion Textbook Exercise Questions and Answers.

RBSE Class 11 Economics Solutions Chapter 6 Measures of Dispersion

RBSE Class 11 Economics Measures of Dispersion Textbook Questions and Answers

Question 1.
A measure of dispersion is a good supplement to the central value in understanding a frequency distribution. Comment.
Answer:
Dispersion is the extent to which values in a distribution differ from the average of the distribution. Central value only tells the single aspect of a distribution, that is, a representative size of the values. It does not reveal the variability (spread of values) present in the data. The measures of dispersion, on the other hand, seek to quantify variability of the data. Thus, a measure of dispersion is considered as a good supplement to the central value in understanding a frequency distribution.

Question 2. 
Which measure of dispersion is the best and how?
Answer:
Standard deviation is the best and the most widely used measure of dispersion. Standard deviation possesses almost all the qualities of a good measure of dispersion such as:
(i) It is based on all values and can be easily applied in advanced statistical problems.
(ii) The sampling stability standard deviation is the maximum compared to all other measures of dispersion.
(iii) While mean deviation ignores the algebraic signs of deviations, standard deviation does not suffer from this demerit as it takes the positive square root of the squared deviations from mean.
(iv) Standard deviation is not affected by the value of the constant from which deviations are calculated.         
(v) It plays a significant role in sampling theory and correlation analysis.

Question 3. 
Some measures of dispersion depend upon the spread of values whereas some calculate the variation of values from a central value. Do you agree?
Answer:
Yes, measures of dispersion such as range and quartile deviation depend upon the spread of values. They do not attempt to calculate how far the values are from their average. However, they give a fairly good idea about the dispersion by calculating the spread of values. On the other hand, measures such as standard deviation and mean deviation depend upon the variation of values from a central value.

Question 4.
In a town, 25% of the persons earned more than ₹ 45,000 whereas 75% earned more than ₹ 18,000. Calculate the absolute and relative values of dispersion.
Answer:
In this case, the absolute measure of dispersion is quartile deviation and the relative measure is coefficient of quartile deviation. Given: =

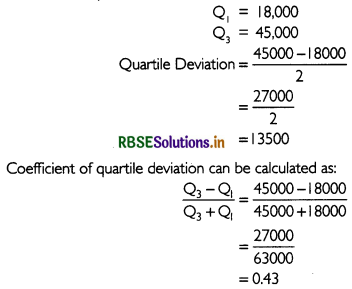
Thus, absolute value of dispersion is ₹ 13,500 and the relative value of dispersion is 0.43. 

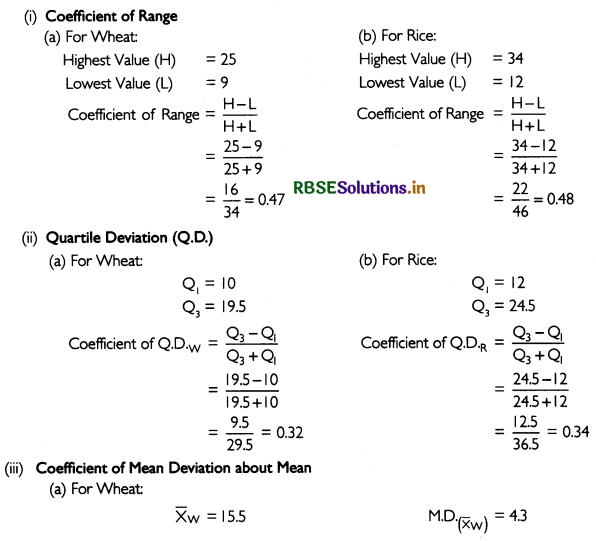
Question 5.
The yield of wheat and rice per acre for 10 districts of a state is as under:

Calculate for each crop:
(i) Range    
(ii) Q.D.
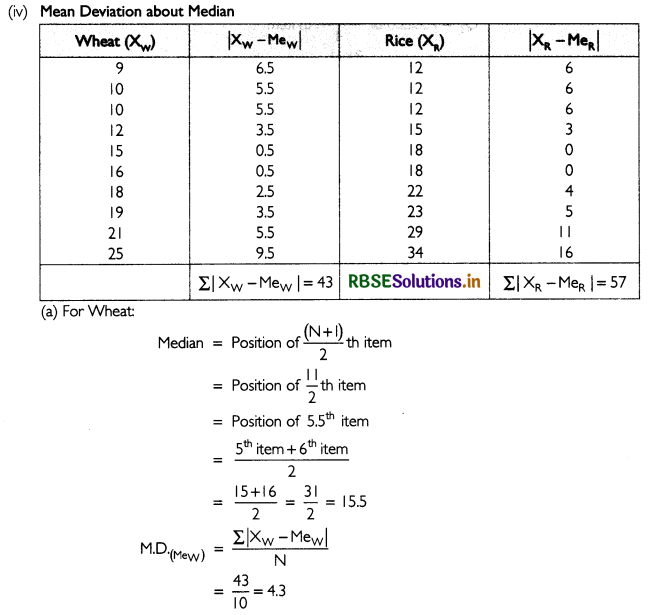
(iii) Mean Deviation about Mean    
(iv) Mean Deviation about Median
(v) Standard Deviation    
(vi) Which crop has greater variation?
(vii) Compare the values of different measures for each crop.
Answer:
(i) Range
(a) For Wheat
Highest Value (H) = 25
Lowest Value (L) = 9
Range of Wheat = H- L = 25 - 9 = 16

(b) For Rice:
Highest Value (H) = 34
Lowest Value (L) = 12
Range of Wheat = H-L = 34 - 12 = 22 

(ii) Quartile Deviation (Q.D.)
Arrange the data in ascending order

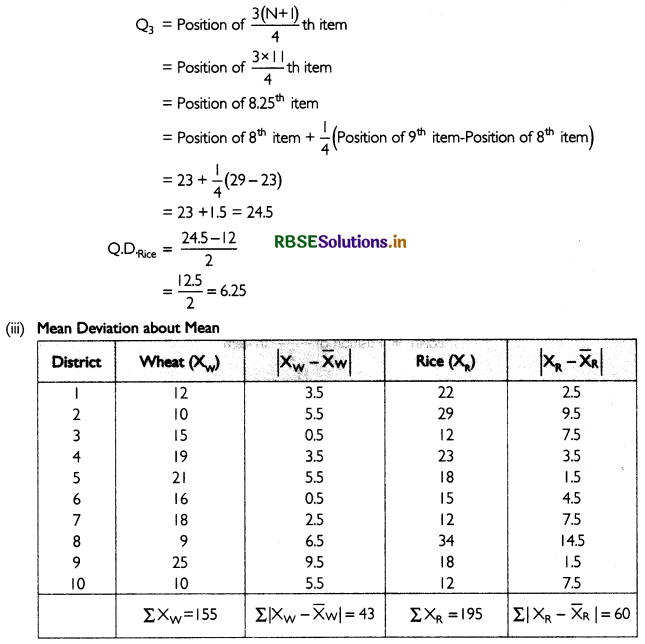
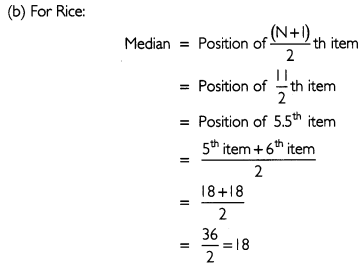
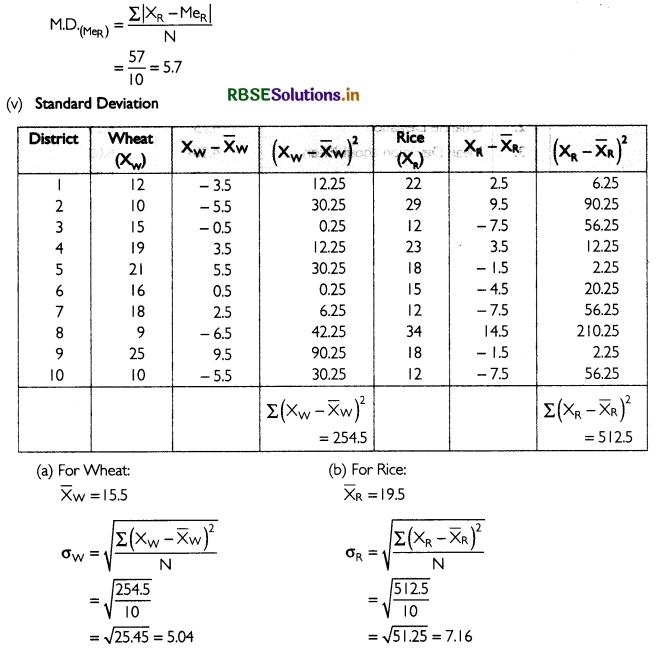
(vi) Crop with Greater Variation
For this we need to calculate the coefficient of variation for wheat and rice:

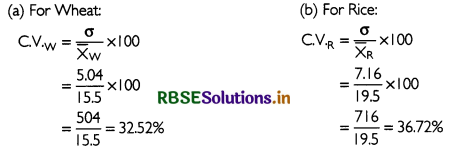
Since the coefficient of variation for rice is greater than that for rice, rice has greater variation.

(vii) Comparison
The table below shows the comparison between the values of different measures for each crop:

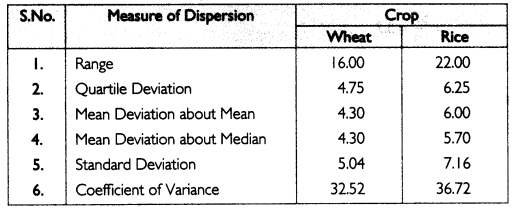
For all the measures of dispersion, the variation is higher in the yield of rice compared to wheat.

Question 6. 
In the previous question, calculate the relative measures of variation and indicate the value which, in your opinion, is more reliable.
Answer:
The relative measures of variation for wheat and rice are calculated below:

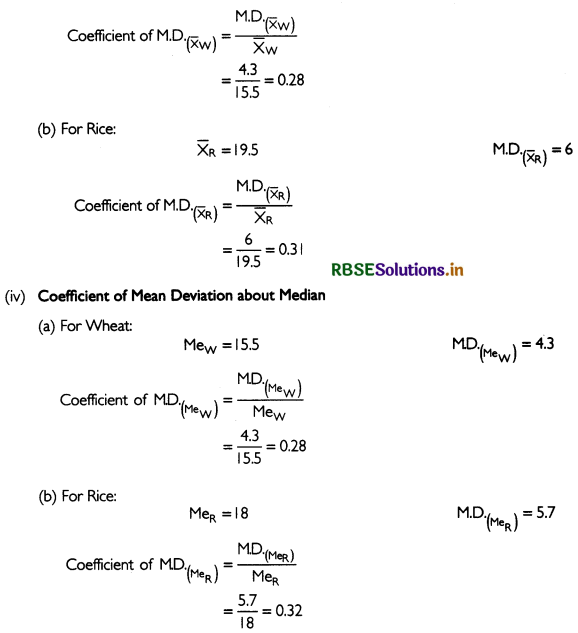
Question 7. 
A batsman is to be selected for a cricket team.The choice is between X and Y on the basis of their five previous scores which are:

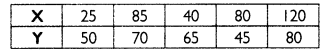
Which batsman should be selected if we want.
(i) a higher run getter, or
(ii) a more reliable batsman in the team?
Answer:
To determine who scores better run, we need to calculate standard deviation. In order to find out a more reliable batsman, coefficient of variation for each batsman should be calculated.
(a) For Batsman X

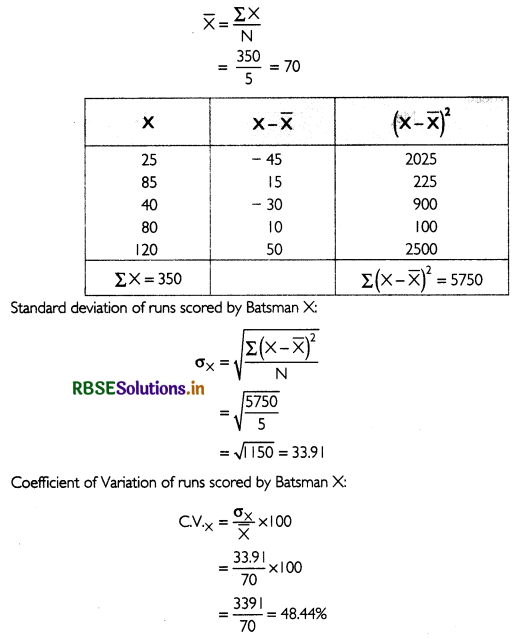
(i) Batsman X should be selected if we want higher runs because σ_x > σ_Y.
(ii) Batsman Y should be selected if we want more reliable batsman in the team because C.V._x > C.V._Y

Question 8. 
To check the quality of two brands of lightbulbs, their life in burning hours was estimated as under for 100 bulbs of each brand.

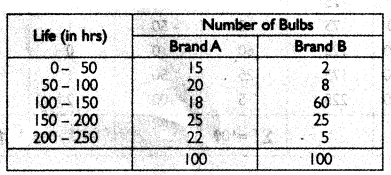
(i) Which brand gives higher life?
(ii) Which brand is more dependable?
Answer:
To determine which brand gives higher life, we need to calculate standard deviation. In order to; find out a more dependable brand, coefficient of variation for each brand should be calculated.
(a) For Brand A
Let assumed mean be A = 125. d is the deviation of each value from the assumed mean.

(b) For Brand B
Let assumed mean be A = 25. d is the deviation of each value from the assumed mean.

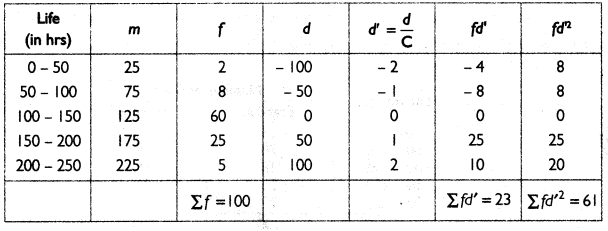
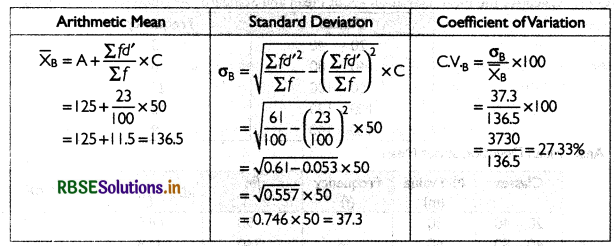
(i) Brand A of Iight bulbs gives higher life because σ_A > σ_B.
(ii) Brand B of light bulbs more dependable because C.V._A > C.V._B

Question 9. 
Average daily wage of 50 workers of a factory was ₹ 200 with a standard deviation of ₹ 40. Each worker is given a raise of ₹ 20. What is the new average daily wage and standard deviation? Have the wages become more or less uniform?
Answer:
If a constant is added to all observations of a series, the arithmetic mean also increases by that constant. Thus, when each worker is given a raise of ₹ 20, the new average daily wage will be:
200 + 20 = ₹ 220
Standard deviation is independent of origin. That is, it is not affected by the constant from which deviations are taken. Thus,
Olds = News = ₹ 40
Since standard deviation has remained unchanged, the uniformity of the wages would be unaffected.

Question 10.
If in the previous question, each worker is given a hike of 10% in wages, how are the Mean and Standard Deviation values affected?
Answer:
Assume the wage of the worker be W_r
Since each worker is given a hike of 10 percent in wages, new wage of the worker will be:

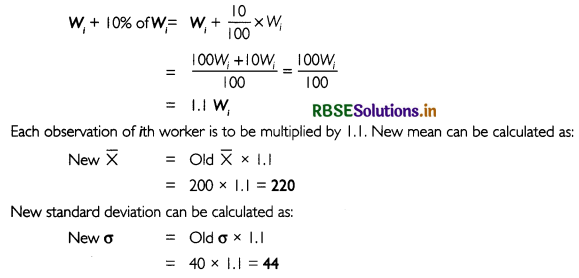
Question 11.
Calculate the Mean Deviation about Mean and Standard Deviation for the following distribution.

Answer:

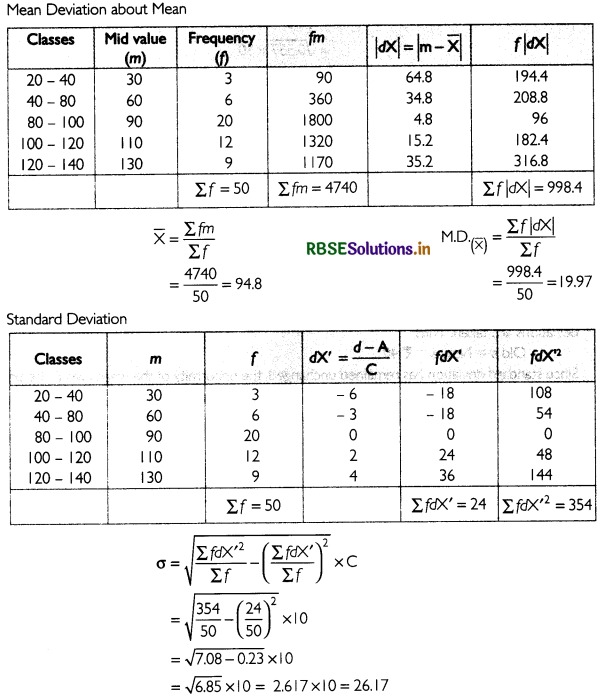
Thus, the mean deviation about mean and standard deviation for the given distribution are 19.97 and 26.17 respectively.

Question 12. 
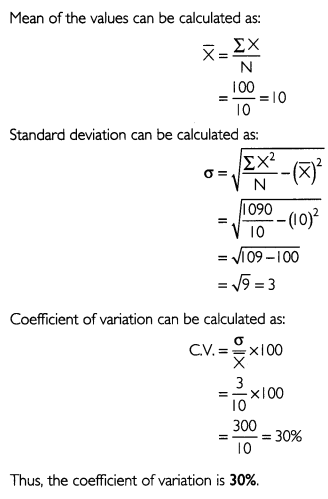
The sum of 10 values is 100 and the sum of their squares is 1090. Find the Coefficient of variation. 
Answer:
Given: N= 10; ∑X = 100; ∑X² =1090