RBSE Solutions for Class 12 Maths Chapter 7 समाकलन Ex 7.2

Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 7 समाकलन Ex 7.2 Textbook Exercise Questions and Answers.

RBSE Class 12 Maths Solutions Chapter 7 समाकलन Ex 7.2

1 से 37 तक के प्रश्नों में प्रत्येक फलन का समाकलन ज्ञात कीजिए

प्रश्न 1.
$\frac{2 x}{1+x^2}$
हल:
∫$\frac{2 x}{1+x^2}$ dx,
यहाँ 1 + x² = 1 रखने पर ∴ 2x dx = at
अतः ∫$\frac{2 x}{1+x^2}$ dx = ∫$\frac{d t}{t}$ = log |t| + C, t का मान रखने पर
= log |(1 + x²)| + C

प्रश्न 2.
$\frac{(\log x)^2}{x}$
हल:
∫$\frac{(\log x)^2}{x}$ dx
यहाँ log x = t रखने पर ∴ $\frac{1}{x}$ dx = dt

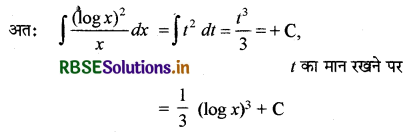
प्रश्न 3.
$\frac{1}{x+x \log x}$
हल:

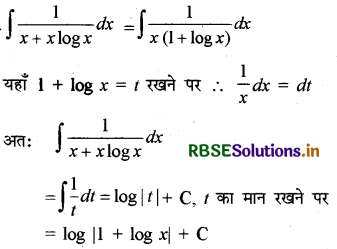
प्रश्न 4.
sin x sin (cos x)
हल:
∫sin x sin(cos x) dx
यहाँ cos x = t रखने पर, ∴ - sin x dx = dt
अतः = - ∫sin t dt = cost + C, t का मान रखने पर
= cos (cos x) + C

प्रश्न 5.
sin (ax + b) cos (ax + b)
हल:
माना I = sin(ax + b) cos(ax + b) dx
यहाँ sin (ax + b) = t रखने पर, ∴ a cos (ax + b) = dt
अत: = ∫sin(ax + b) cos(ax + b) dx

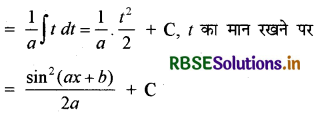
प्रश्न 5 की द्वितीय विधि

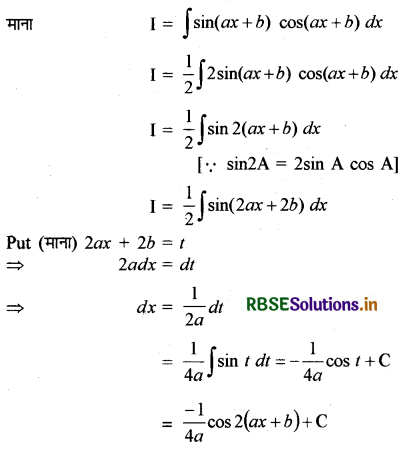
प्रश्न 6.
√ax + b
हल:
∫√ax + b dx
यहाँ ax + b= 1 रखने पर ∴ a dx = dt

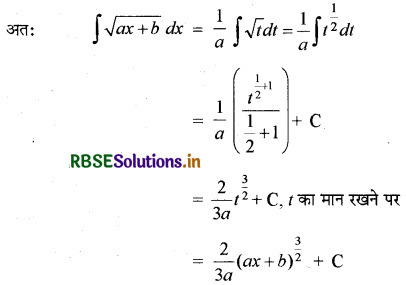
प्रश्न 7.
x√x + 2
हल:
माना

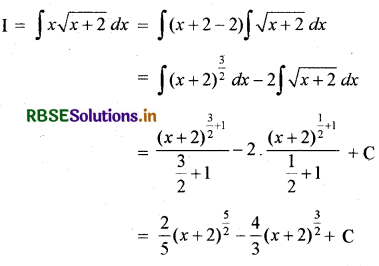
प्रश्न 8.
x√1 + 2x²
हल:
माना I = ∫x√1 + 2x² dx
यहाँ 1 + 2x² = 1 रखने पर 4x dx = dt

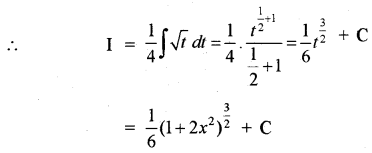
प्रश्न 9.
(4x + 2)$\sqrt{x^2+x+1}$
हल:
माना = ∫(4x + 2)$\sqrt{x^2+x+1}$ dx
= 2∫(2x + 1)$\sqrt{x^2+x+1}$ dx
अब x² + x + 1 = 1 रखने पर ∴ (2x + 1) x = dt

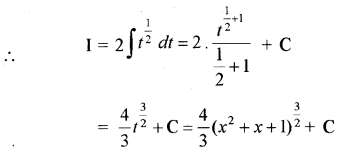
प्रश्न 10.
$\frac{1}{x-\sqrt{x}}$
हल:

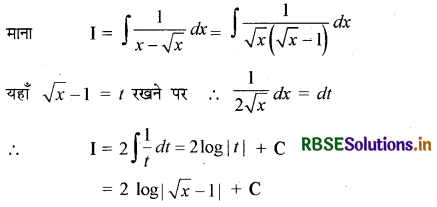
प्रश्न 11.
$\frac{x}{\sqrt{x+4}}$; x > 0
हल:
माना

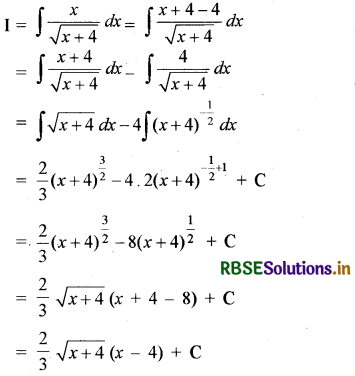
प्रश्न 12.
(x³ - 1)^{$\frac{1}{3}$} x⁵
हल:
माना I = ∫(x³ - 1)^{$\frac{1}{3}$} x⁵ dx = ∫(x³ - 1)^{$\frac{1}{3}$} x³ . x² dx
यहाँ x³ - 1 = 1 या x³ = 1 + t रखने पर, ∴ 3x² dx = dt

प्रश्न 13.
$\frac{x^2}{\left(2+3 x^3 \cdot\right)^3}$
हल:

प्रश्न 14.
$\frac{1}{x(\log x)^m}$, x > 0, m ≠ 1
हल:

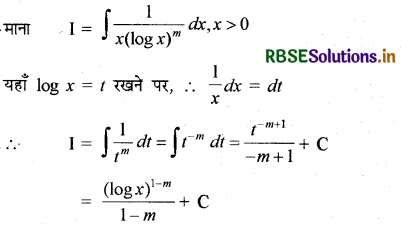
प्रश्न 15.
$\frac{x}{9-4 x^2}$
हल:

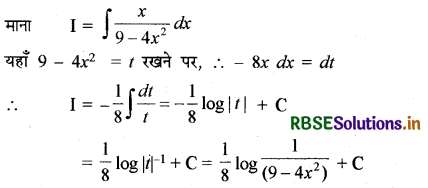
प्रश्न 16.
e^2x+3
हल:
माना I = ∫e^2x+3 dx
यहाँ 2x + 3 = t रखने पर, ∴ 2 dx = dt
∴ I = $\frac{1}{2}$∫e^t dt = $\frac{1}{2}$e^t + C = $\frac{1}{2}$e^2x+3 + C

प्रश्न 17.
$\frac{x}{e^{x^2}}$
हल:

प्रश्न 18.
$\frac{e^{\tan ^{-1}}}{1+x}$
हल:
माना I = $\frac{e^{\tan ^{-1}}}{1+x}$
यहाँ tan^-1 x = 1 रखने पर, ∴ $\frac{1}{1+x^2}$ dx = dt
∴ I = ∫e^t dt = e^t + C = e^tan-1 + C

प्रश्न 19.
$\frac{e^{2 x}-1}{e^{2 x}+1}$
हल:

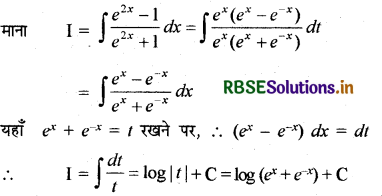
प्रश्न 20.
$\frac{e^{2 x}-e^{-2 x}}{e^{2 x}+e^{-2 x}}$
हल:
माना I = $\frac{e^{2 x}-e^{-2 x}}{e^{2 x}+e^{-2 x}}$
यहाँ e^2x + e^-2x = 1 रखने पर, ∴ (2e^2x - 2e^-2x) dx = dt
या 2(e^2x - e^-2x) dx = dt
∴ I = $\frac{1}{2} \int \frac{d t}{t}$ = $\frac{1}{2}$ log|t| + C
= $\frac{1}{2}$ = log|e^2x + e^-2x| + C

प्रश्न 21.
tan² (2x - 3)
हल:
माना I = ∫tan² (2x + 3) dx = ∫[sec² (2x - 3) - 1] dx
= ∫sec² (2x - 3) dx - ∫dx
= ∫sec² (2x - 3)dx - x
यहाँ 2x - 3 = t रखने पर, ∴ 2 dx = dt
= $\frac{1}{2}$∫sec² t dt - x = $\frac{1}{2}$ tan t - x + C
= $\frac{1}{2}$tan (2x - 3) - x + C

प्रश्न 22.
sec² (7 - 4x)
हल:
माना I = sec² (7 - 4x)
यहाँ 7 - 4x = t रखने पर, ∴ - 4 dx = dt
∴ I = - $\frac{1}{4}$∫sec² t dt = - $\frac{1}{4}$ tan t + C
= - $\frac{1}{4}$ tan(2x - 3) + C

प्रश्न 23.
$\frac{\sin ^{-1} x}{\sqrt{1-x^2}}$
हल:

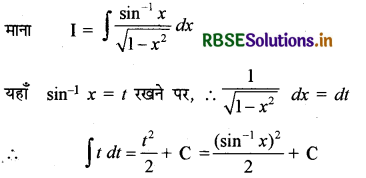
प्रश्न 24.
$\frac{2 \cos x-3 \sin x}{6 \cos x+4 \sin x}$
हल:

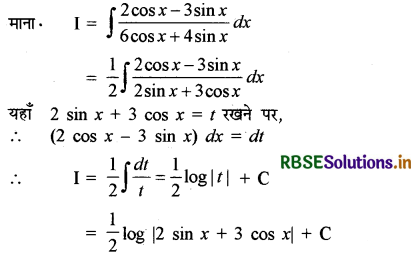
प्रश्न 25.
$\frac{1}{\cos ^2 x(1-\tan x)^2}$
हल:

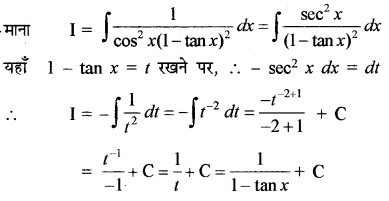
प्रश्न 26.
$\frac{\cos \sqrt{x}}{\sqrt{x}}$
हल:

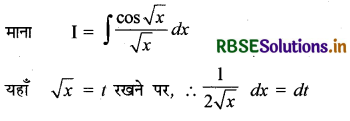
∴ = 2∫cos t dt = 2 sin t + C
= 2 sin√x + C

प्रश्न 27.
$\sqrt{\sin 2 x}$ cos 2x
हल:

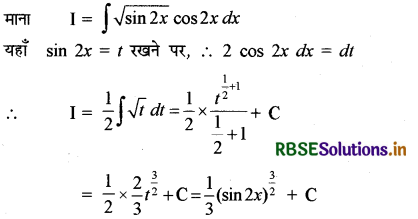
प्रश्न 28.
$\frac{\cos x}{\sqrt{1+\sin x}}$
हल:

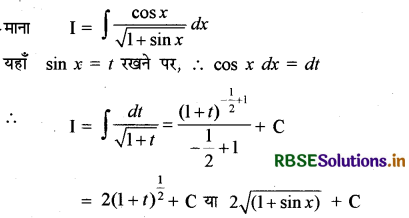
प्रश्न 29.
cot x log sin x
हल:
माना I = ∫cot x log sin x dx
यहाँ log sin x = 1 रखने पर, ∴ cot x dx = dt
∴ I = ∫t dt = $\frac{t^2}{2}$ + C = $\frac{1}{2}$(log sin x)² + C

प्रश्न 30.
$\frac{\sin x}{1+\cos x}$
हल:
माना I = ∫$\frac{\sin x}{1+\cos x}$ dx
यहाँ 1 + cos x = t रखने पर, ∴ - sin x dx = dt
∴ I = - ∫$\frac{1}{t}$ dt = - log |t| + C
= - log |1 + cos x| + C
= log$\left|\frac{1}{1+\cos x}\right|$ + C

प्रश्न 31.
$\frac{\sin x}{(1+\cos x)^2}$
हल:
माना I = ∫$\frac{\sin x}{(1+\cos x)^2}$ dx
यहाँ 1 + cos x = t रखने पर,
∴ - sin x dx = dt

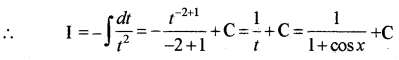
प्रश्न 32.
$\frac{1}{1+\cot x}$
हल:

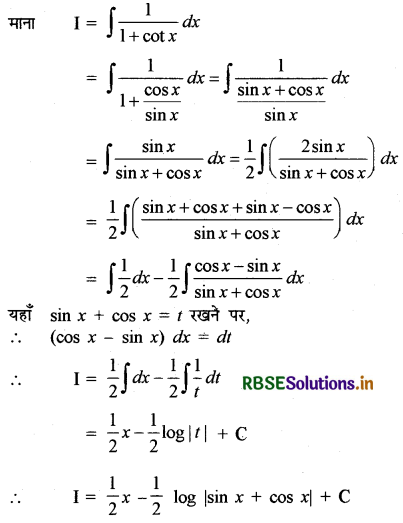
प्रश्न 33.
$\frac{1}{1-\tan x}$
हल:

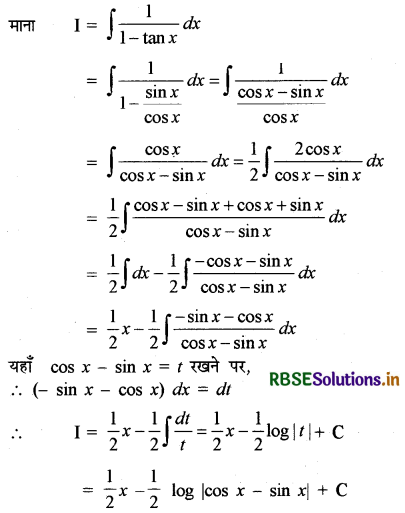
प्रश्न 34.
$\frac{\sqrt{\tan x}}{\sin x \cos x}$
हल:

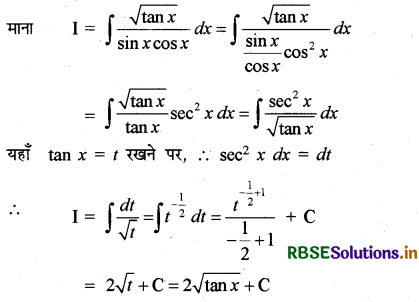
प्रश्न 35.
$\frac{(1+\log x)^2}{x}$
हल:
माना I = ∫$\frac{(1+\log x)^2}{x}$ dx
यहाँ 1 + log x + t रखने पर, ∴ $\frac{1}{x}$ dx = dt
∴ I = ∫t² dt = $\frac{t^3}{3}$ + C = $\frac{1}{3}$(1 + log x)³ + C

प्रश्न 36.
$\frac{(x+1)(x+\log x)^2}{x}$
हल:

प्रश्न 37.
$\frac{x^3 \sin \left(\tan ^{-1} x^4\right)}{1+x^8}$
हल:

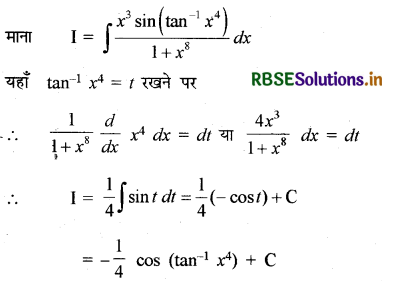
प्रश्न 38 एवं 39 में सही उत्तर का चयन कीजिए-

प्रश्न 38.
∫$\frac{10 x^9+10^x \log _{e^{10}}}{x^{10}+10^x}$ dx बराबर है-
(A) 10^x - x¹⁰ + C
(B) 10^x + x¹⁰ + C
(C) (10^x - x¹⁰)^-1 + C
(D) log (10^x + x¹⁰) + C
उत्तर:
(D) log (10^x + x¹⁰) + C

हल:
∫$\frac{10 x^9+10^x \log _{e^{10}}}{x^{10}+10^x}$ dx
Put (माना) x¹⁰ + 10^x = 1
(10x⁹ + 10^x log_e 10) dx = dt
∫$\frac{d t}{t}$ = log(t) + C = log (x¹⁰ + 10^x) + C
अतः सही विकल्प D है।

प्रश्न 39.
∫$\frac{d x}{\sin ^2 x \cos ^2 x}$ dx बराबर है
(A) tan x + cot x + C
(B) tan x - cot x + C
(C) tan x cot x + C
(D) tan x - cot 2x + C
उत्तर:
(B) tan x - cot x + C

हल:

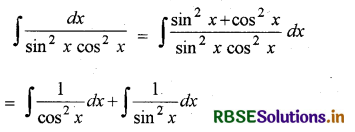
= ∫sec² x dx + ∫cosec²x dx
= tan x - cot x + C
अतः सही विकल्प (B) है।