RBSE Solutions for Class 12 Maths Chapter 7 Integrals Ex 7.11

Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 7 Integrals Ex 7.11 Textbook Exercise Questions and Answers.

RBSE Class 12 Maths Solutions Chapter 7 Integrals Ex 7.11

Question 1.
$\int_{0}^{\pi / 2}$ cos² x dx
Answer:

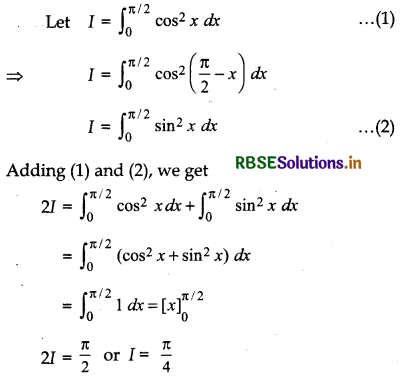
Question 2.
$\int_{0}^{\pi / 2} \frac{\sqrt{\sin x}}{\sqrt{\sin x}+\sqrt{\cos x}}$ dx
Answer:

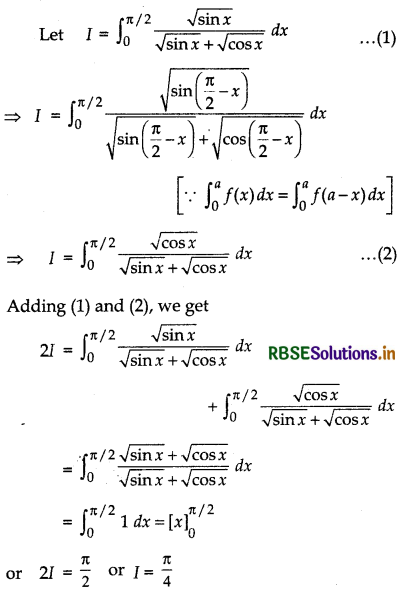
Question 3.
$\int_{0}^{\pi / 2} \frac{\sin ^{3 / 2} x}{\sin ^{3 / 2} x+\cos ^{3 / 2} x}$ dx
Answer:

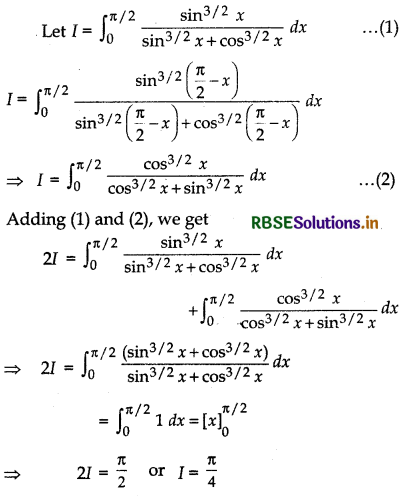
Question 4.
$\int_{0}^{\pi / 2} \frac{\cos ^{5} x}{\sin ^{5} x+\cos ^{5} x}$ dx
Answer:

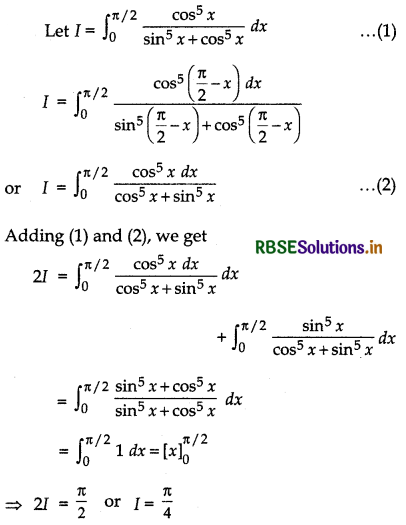
Question 5.
$\int_{-5}^{5}$ |x + 2| dx
Answer:

Question 6.
$\int_{2}^{8}$ |x - 5| dx
Answer:

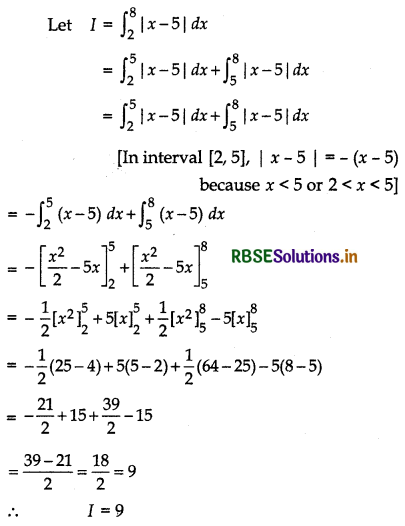
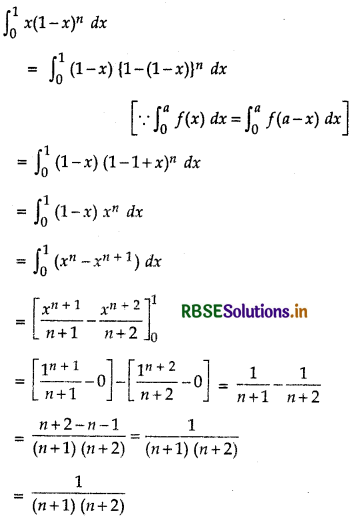
Question 7.
$\int_{0}^{1}$ x(1 - x)ⁿ dx
Answer:

Question 8.
$\int_{0}^{\pi / 4}$ log (1 + tan x) dx
Answer:

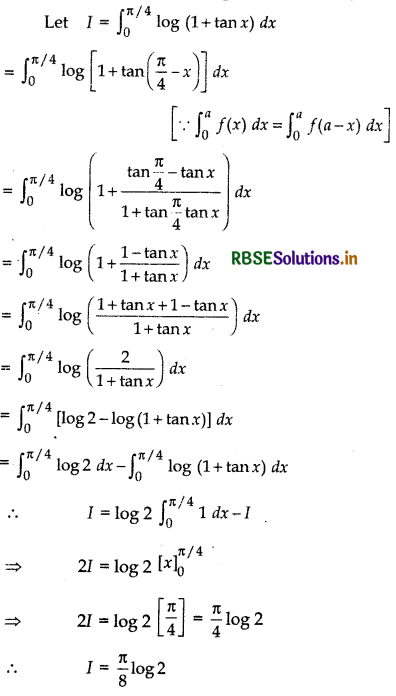
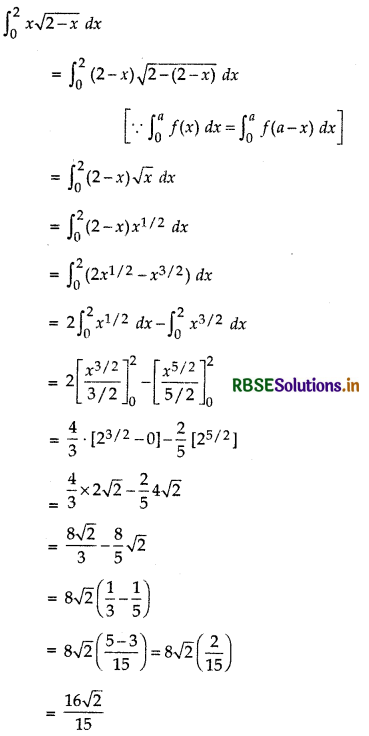
Question 9.
$\int_{0}^{2} x\sqrt{2-x}$ dx
Answer:

Question 10.
$\int_{0}^{\pi / 2}$ (2 log sin x - log sin 2x) dx
Answer:

Question 11.
$\int_{-\pi / 2}^{\pi / 2}$ sin² x dx
Answer:

Question 12.
$\int_{0}^{\pi} \frac{x d x}{1+\sin x}$
Answer:

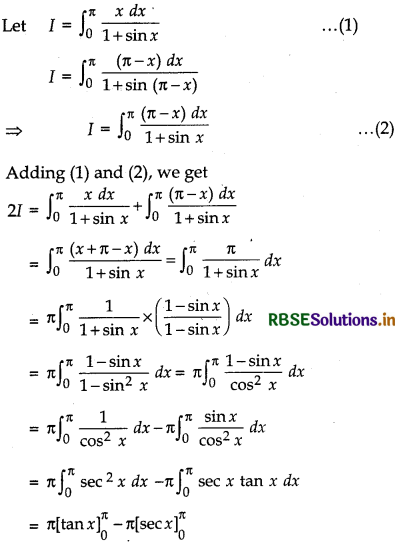
= π[tan π - tan 0] - π[sec π - sec 0]
= π × 0 - π( - 1 - 1) = 2π
2I = 2π
Thus, I = π

Question 13.
$\int_{-\pi / 2}^{\pi / 2}$ sin⁷ x dx
Answer:
Let I = $\int_{-\pi / 2}^{\pi / 2}$ sin⁷ x dx
Then f(- x) = sin⁷ (- x) = [sin (- x)]⁷
= [- sin x]⁷
⇒ f(- x) = - sin⁷ x = - f(x)
i.e., sin⁷ x is an odd function .
$\int_{-a}^{a}$ f(x) dx = 0 If f(- x) = - f(x)
i.e., f is an odd function.
∴ I = $\int_{-\pi / 2}^{\pi / 2}$ sin⁷ x dx = 0

Question 14.
$\int_{0}^{2 \pi}$ cos⁵ x dx
Answer:

Question 15.
$\int_{0}^{\pi / 2} \frac{\sin x-\cos x}{1+\sin x \cos x}$ dx
Answer:

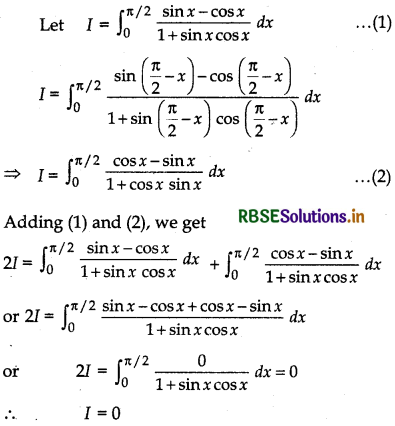
Question 16.
$\int_{0}^{\pi}$ log (1 + cos x) dx
Answer:

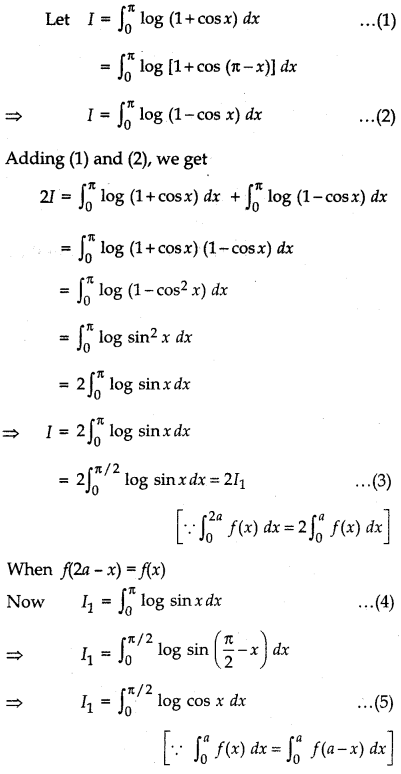
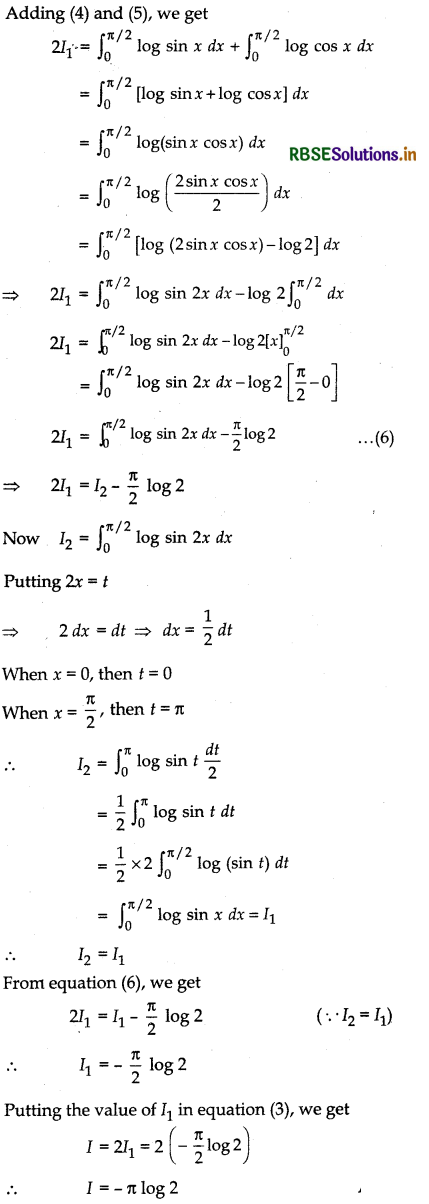
Question 17.
$\int_{0}^{a} \frac{\sqrt{x}}{\sqrt{x}+\sqrt{a-x}}$ dx
Answer:

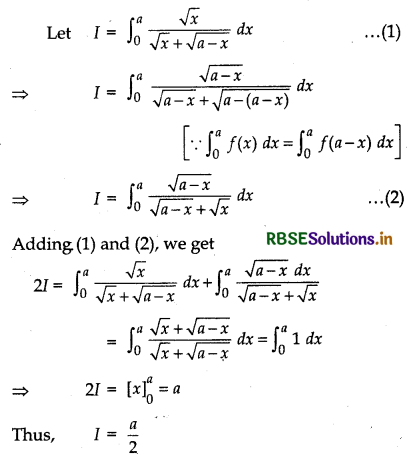
Question 18.
$\int_{0}^{4}$ |x - 1| dx
Answer:

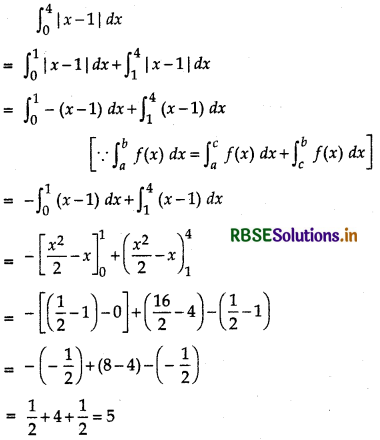
Question 19.
Show that
$\int_{0}^{a}$ f(x) g(x) dx = 2$\int_{0}^{a}$ f(x) dx
if f and g are defined as
f(x) = f(a - x) and g(x) + g(a - x) = 4
Answer:

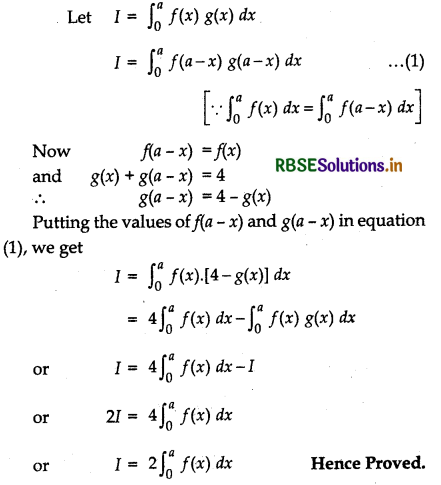
Question 20.
The value of
$\int_{-\pi / 2}^{\pi / 2}$ (x³ + x cos x + tan⁵ x + 1) dx is:
(A) 0
(B) 2
(C) π
(D) 1
Answer:
Let I = $\int_{-\pi / 2}^{\pi / 2}$ (x³ + x cos x + tan⁵ x + 1) dx
= $\int_{-\pi / 2}^{\pi / 2}$ (x³ + x cos x + tan⁵ x + 1) dx + $\int_{-\pi / 2}^{\pi / 2}$ 1 dx
I = I₁ + $\int_{-\pi / 2}^{\pi / 2}$ 1 dx ...... (1)
Again f(x) = x³ + x cos x + tan⁵ x
= - x³ - x cos x - tan⁵ x
= - (x³ + x cos x + tan⁵ x)
= - f(x)
i.e., f is an odd function, because
f(- x) = - f(x)

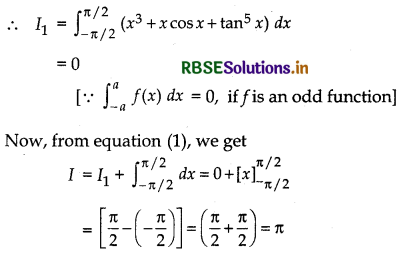
∴ I = π
Hence, (C) is the correct answer.

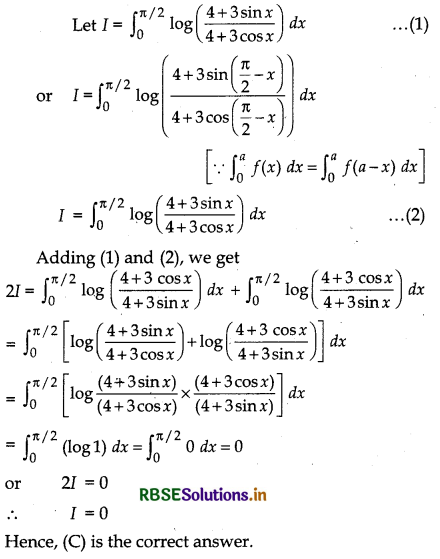
Question 21.
The value of
$\int_{0}^{\pi / 2} \log \left(\frac{4+3 \sin x}{4+3 \cos x}\right)$ dx is:
(A) 2
(B) $\frac{3}{4}$
(C) 0
(D) - 2
Answer: