RBSE Solutions for Class 12 Maths Chapter 5 Continuity and Differentiability Ex 5.1

Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 5 Continuity and Differentiability Ex 5.1 Textbook Exercise Questions and Answers.

RBSE Class 12 Maths Solutions Chapter 5 Continuity and Differentiability Ex 5.1

Question 1.
Prove that the function f(x) = 5x - 3 is continuous at x = 0, at x = - 3 and at x = 5.
Answer:
Given, function, f(x) = 5x - 3

(i) For continuity at x = 0

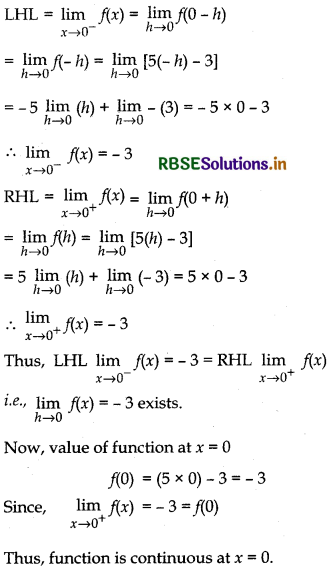
(ii) For continuity at x = 3

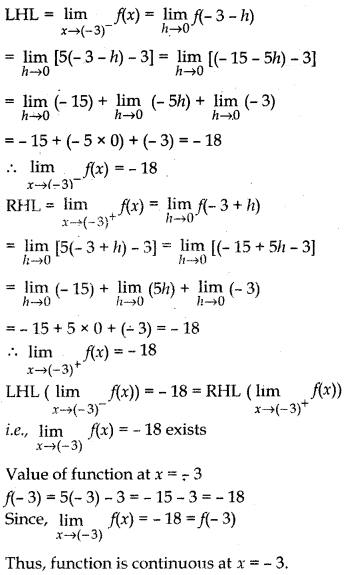
(iii) For continuity at x = 5

Question 2.
Examine the continuity of the function
f(x) = 2x² - 1 at x = 3
Answer:
Given function, f(x) = 2x² - 1

For continuity at x = 3

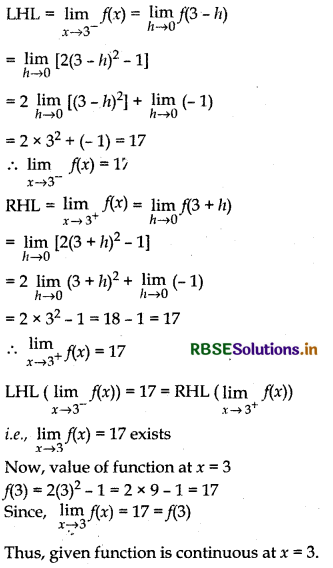
Question 3.
Examine the following functions for continuity.
(a) f(x) = x - 5
Answer:
Given, f(x) = x - 5
Given function is polynomial function.
Polynomial function is continuous everywhere.
Thus, (x - 5) also continuous everywhere.
i.e., function f is continuous everywhere, where x ∈ R.

(b) f(x) = \(\frac{1}{x-5}\), x ≠ 5
Answer:
f(x) = \(\frac{1}{x-5}\), x ≠ 5
Function is not defined at x = 5 so domain of function is R - [5]. Now x - 5 is polynomial function which is continuous everywhere in domain R - [5] and 1 is a constant function which is continuous everywhere.
Let g(x) = 1 and h(x) = (x - 5), x ≠ 5
Then f(x) = \(\frac{g(x)}{h(x)}\)
which is a rational function which is continuous. Thus, function f(x) is continuous every where in domain R - {5}.

Note: Function f(x) is not defined at x = 5. Thus, it is not continuous at x = 5.

(c) f(x) = \(\frac{x^{2}-25}{x+5}\), x ≠ - 5
Answer:
Given, f(x) = \(\frac{x^{2}-25}{x+5}\), x ≠ - 5
Function f(x) is not defined at x = - 5 so it is not continuous at x = - 5.
and domain of f(x) is R - {- 5}.
Let c ∈ R-{- 5} and c ≠ - 5
For continuity at x = c

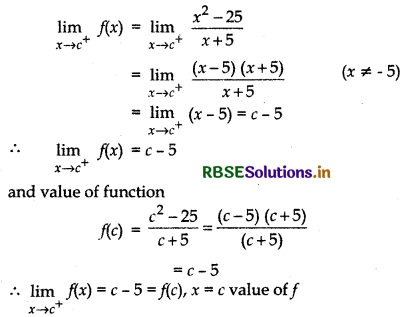
Thus, function is continuous everywhere in domain R - {- 5}.

(d) f(x) = |x - 5|
Answer:

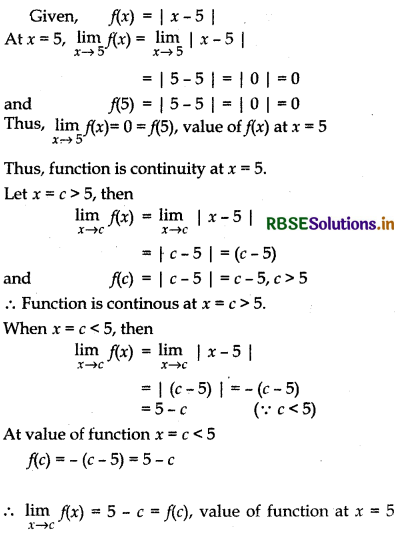
where c < 5
∴ Function is continuous at c < 5.
Thus, function is continuous everywhere in R.

Question 4.
Prove that the function f(x) = xⁿ is continuous at x = n, where n is a positive integer.
Answer:
Given function f(x) = xⁿ is a polynomial function. Polynomial function is continuous everywhere in domain R. Thus function f(x) = xⁿ is continuous where x ∈ R and x ∈ N is positive integer. Hence Proved.

Question 5.
Is the function f defined by

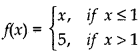
continuous at x = 0, at x = 1 and at x = 2?
Answer:
Given function,

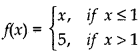
(i) For continuity at x = 0

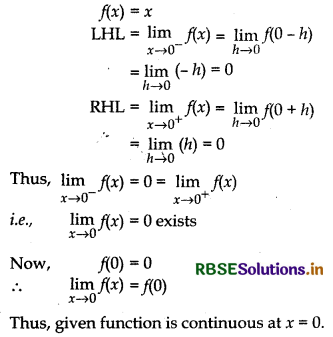
(ii) For continuity at x = 1

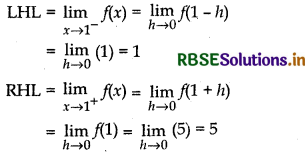
∴ (LHL) 1 ≠ 5 (RHL)
Thus, function is not continuous at x = 1.

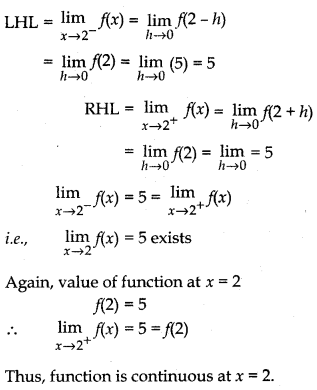
(iii) For continuity at x = 2

Find all points of discontinuity of f, where f is defined by

Question 6.

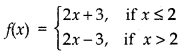
Answer:
Given function

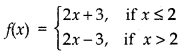
For continuity at x = 2

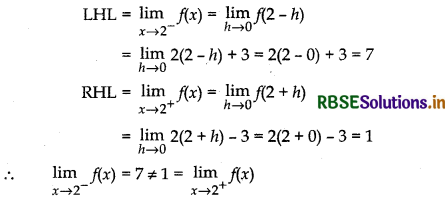
Thus, function is not continuous at x = 2 i.e., x = 2 is the point of discontinuity of f.

Question 7.

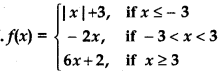
Answer:
(i) continuity of function at x = - 3

(ii) continuity of function at x = 3

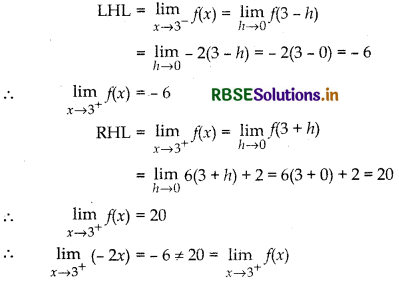
So, function is not continuous at x = 3.
Thus, x = 3 is point of discontinuity of function.

Question 8.

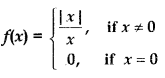
Answer:
For continuity at x = 0

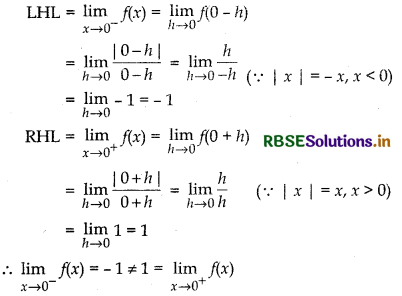
∴ Function is not continuous at x = 0.
Thus, x = 0 is point of discontinuity of function.

Question 9.

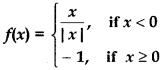
Answer:
For continuity of function at x = 0

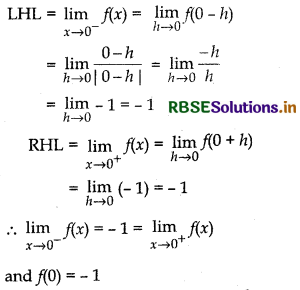
So, function is continuous at x = 0.
Thus, there is no point of discontinuity of function.

Question 10.

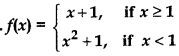
Answer:
For continuity of function at x = 1

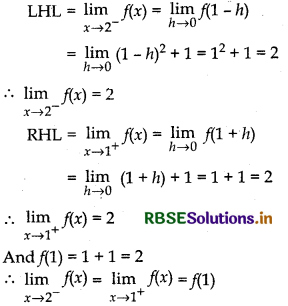
Therefore, f is continuous at all points of x such that x > 1. Thus, the given function f has no point of discontinuity.

Question 11.

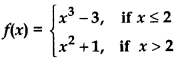
Answer:
For continuity of function at x = 2

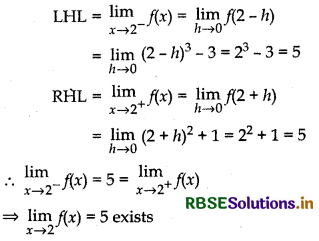
value of function at x = 2
f(2) = 2³ - 3 = 8 - 3 = 5
∴ \(\lim _{x \rightarrow 2}\) f(x) = 5 = f(2), value of function at x = 2
Thus, given function is continuous at x = 2 and there is no point of discontinuity of function.

Question 12.

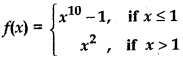
Answer:
Given function

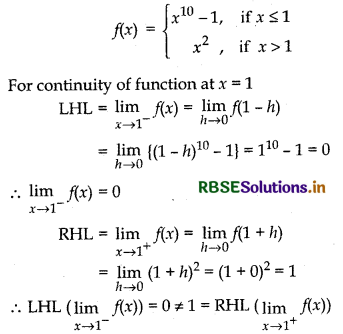
For continuity of function at x = 1

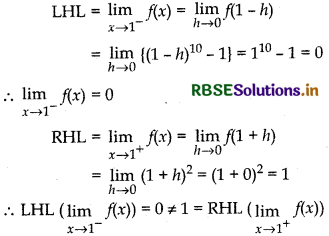
Thus, function is not continuous at x = 1
∴ x = 1 is point of discontinuity of function.

Question 13.
Is the function defined by

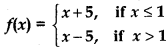
a continuous function?
Answer:
For continuity of function at x = 1

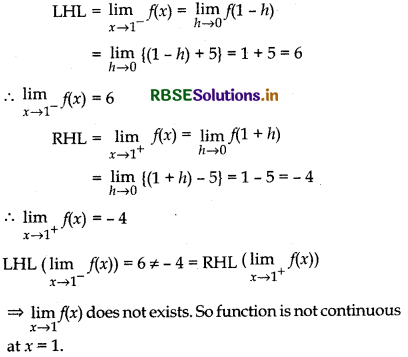
Discuss the continuity of the function f, when f is defined by:

Question 14.

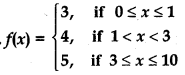
Answer:
(i)

(ii) For continuity at x = 3

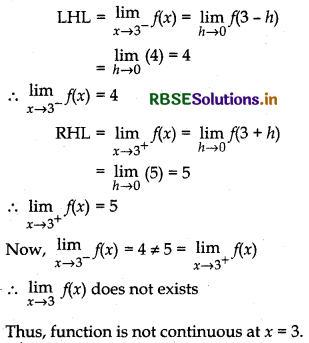
Question 15.

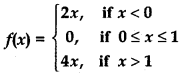
Answer:
(i) For continuity at x = 0

(ii) For continuity at x = 1

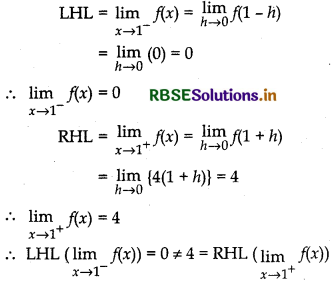
i.e., \(\lim _{x \rightarrow 1}\) f(x) does not exists.
Thus, function is not continuous at x = 1, i.e., x = 1 is point of discontinuity of function.

Question 16.

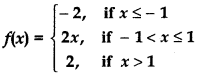
Answer:
(i) For continuity at x = - 1

(ii) For continuity at x = 1

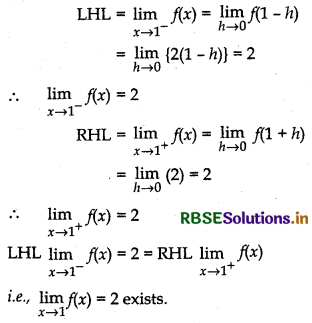
Value of at f(x) x = 1 = f(1) = 2 × 1 = 2
∴ \(\lim _{x \rightarrow 1}\) f(x) = 2 = f(1), value of f at x = 1
Thus, function is continuous at x = 1.

Question 17.
Find the relationship between a and b so that the function f defined by

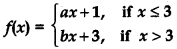
is continuous at x= 3.
Answer:
Function is continuous at x = 3

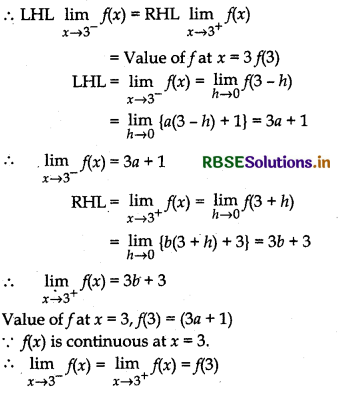
⇒ 3a = 3b + 3 - 1
⇒ 3a = 3b + 2
⇒ a = b + \(\frac{2}{3}\)
If b = k an arbitrary real number,
then a = k + \(\frac{2}{3}\)
Thus, a = k + \(\frac{2}{3}\) and b = k

Question 18.
For what value of λ is the function defined by

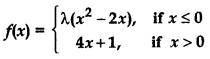
continuous at x = 0? What about continuity at x = 1?
Answer:
(i) For continuity at x = 0

Thus, function is not continuous at x = 0 for any value of λ.

(ii) For continuity at x = 1

Value of f(x) at x = 1
f(1) = 4 × 1 + 1 = 4 + 1 = 5
∴ \(\lim _{x \rightarrow 1}\) f(x) = 5 = f(1), value of f at x = 1
Thus, function is continuous at x = 1.

Question 19.
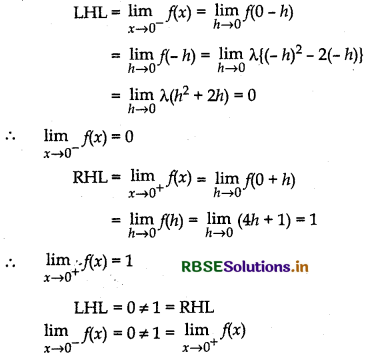
Show that the function defined by g(x) = x - [x] is discontinuous at all integral points. Here [x] denotes the greatest integer less than or equal to x.
Answer:
Given function, g(x) = x - [x]
(i) For continuity or discontinuity at x = 0

Since, -1 is the greatest integer before 0 as shown in real number line.

i.e., Left hand limit = 1 ≠ 0 = right hand limit
∴ \(\lim _{x \rightarrow 0}\) g(x) does not exists.
∴ Function is not continuous at x = 0, i.e., discontinuous.

(ii) Let x = c ≠ 0, is any arbitrary real integer, then for continuity or discontinuity at x = c

Thus, function is not continuous at x = c, i.e., discontinuous.
Since, c is an arbitrary integer. Thus, g(x) is discontinuous at all integer.

Question 20.
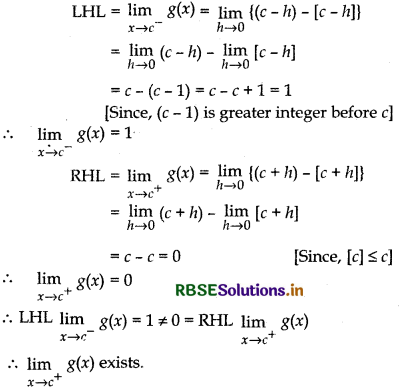
Is the function defined by f(x) = x² - sin x + 5 continuous at x = π ?
Answer:
Given function, f(x) = x² - sin x + 5
For continuity at x = π

Question 21.
Discuss the continuity of the following functions:
(a) f(x) = sin x + cos x
Answer:
Given, f(x) = sin x + cos x
Let x = c be any arbitrary real number.
Then at x = c for continuity of function f(x) = sin x + cos x

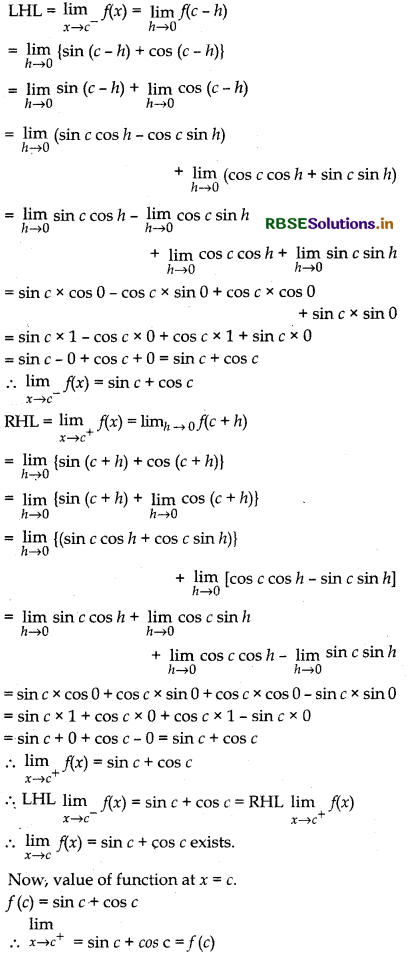
Thus, function is continuous at x = c.
∵ c is an arbitrary real number.
∴ f(x) = sin x + cos x is continuous for all real numbers.

(b) f(x) = sin x - cos x
Answer:
Given, f(x) = sin x - cos x
Let x = c be any arbitrary real number.
Thus, for continuity of function f(x) = sin x - cos x at x = c

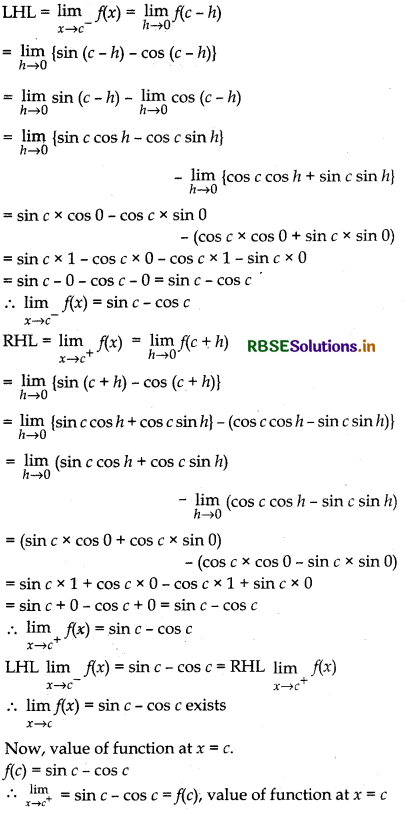
Thus, function is continuous at x = c.
∵ c is an arbitrary real number so function is continuous for all real numbers.

(c) f(x) = sin x.cos x
Answer:
Given, f(x) = sin x.cos x
Let x = c be any arbitrary real number, Now for continuity of function at x = c

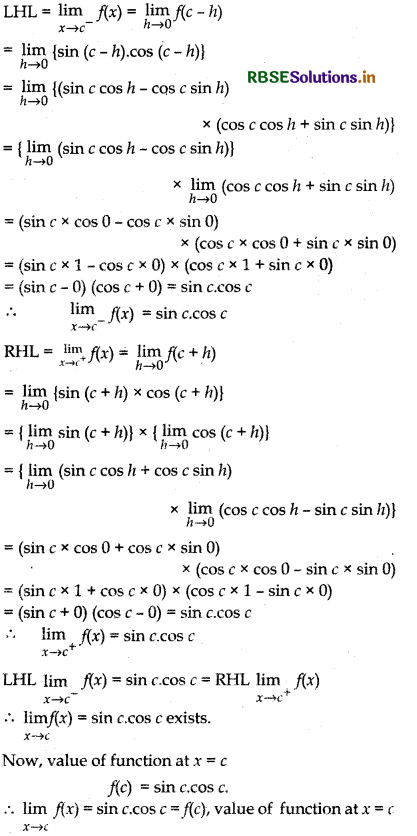
∴ Function is continuous at x = C.
∵ x = c is an arbitrary real number. Thus, function is continuous for all real numbers.
Thus, function f(x) = sin x.cos x is continuous.

Question 22.
Discuss the continuity of the cosine, cosecant, secant and cotangent functions.
Answer:
(a) Continuity of cosine function
Let f(x) = cos x and x = c is an arbitrary real number.
For continuity of function at x = c

∴ Function is continuous at x = c.
∵ c is an arbitrary real number. Thus, function is continuous for all real numbers.
Therefore, cosine function is continuous.

(b) Continuity of cosecant function
Let f(x) = cosec x and x = c, where c ∈ R - {nπ}, n ∈ Z (n is integer). Since, cosec x is not defined for x = nπ.
For continuity of function at x = c [c ∈ R - {nπ}]

∴ Function is continuous at .i c, where c ∈ R - {nπ}.
∵ C ∈ R - {nπ} is an arbitrary real number. Thus, cosec function is continuous in domain R - {nπ}.

(c) Continuity of secant function
Let f(x) = sec’ x
and x = c ∈ R - \({(2n + 1)\frac{\pi}{2}}\)
[since, at \({(2n + 1)\frac{\pi}{2}}\) sec x is not defined and n ∈ Z]
For continuity at x = c

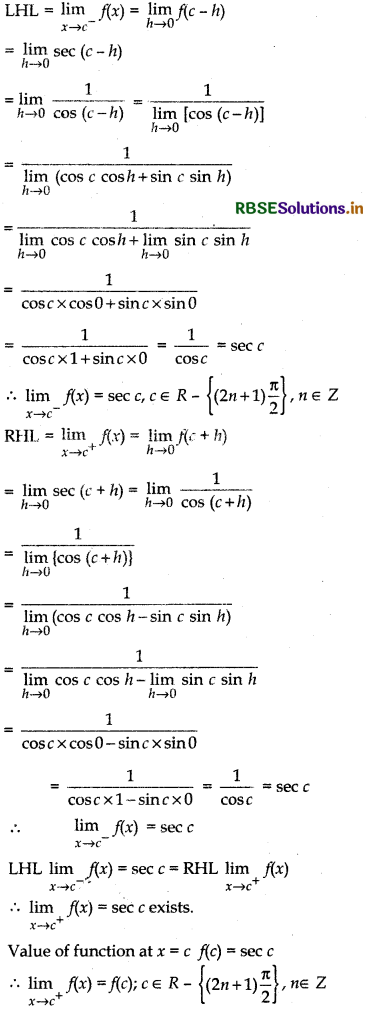
∴ Function is continuous at x c.
∵ c is an arbitrary real number. Thus, secant function is continuous for all real number, where
c ∈ R - {(2n + 1)\(\frac{\pi}{2}\)}, n ∈ Z

(d) Continuity of cotangent function
Let f(x) = cot x and x = c is an arbitrary real number,
where C ∈ R - {nπ}, n ∈ Z since cot x, is not defined at x = nπ.
For continuity at x = c


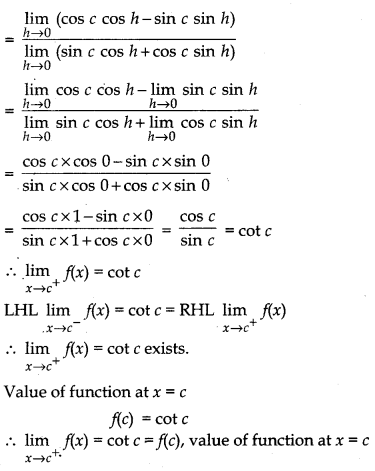
∴ Function f(x) = cot x, c is continuous at C₁ where c ∈ R - {nπ}, n ∈ Z.
∵ c is an arbitrary real number. Thus cotangent function is continuous for all values of R - {nπ}.

Question 23.
Find all points of discontinuity of f, where

Answer:

∴ Function is continuous at x = 0.
Thus f(x) is continuous for all value of real number.
Therefore, there is no point of discontinuity of function.

Question 24.
Determine if f defined by

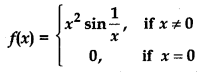
is a continuous function ?
Answer:

∴ Function is continuous at x = 0.
Thus f(x) is true for all real number.

Question 25.
Examine the continuity of f, where f is defined by

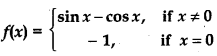
Answer:
Five continuity at x = 0

∴ Function is continuous at x = 0.
Thus, function f(x) is continuous for all real numbers.

Find the values of k so that the function f is continuous at the indicated point in Q. 26 to 29.

Question 26.

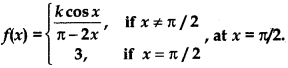
Answer:

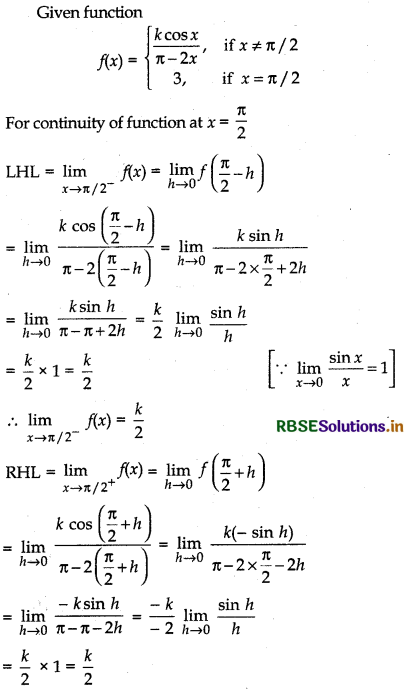
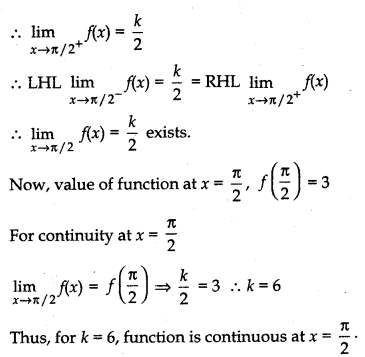
Question 27.

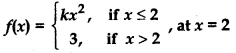
Answer:

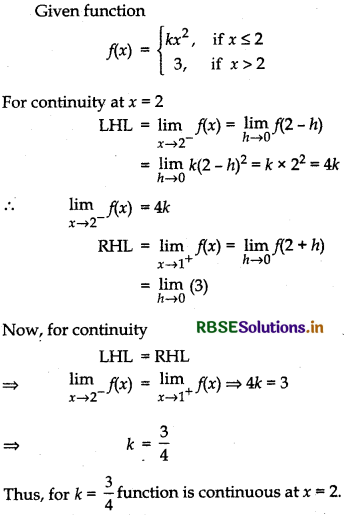
Question 28.

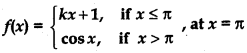
Answer:

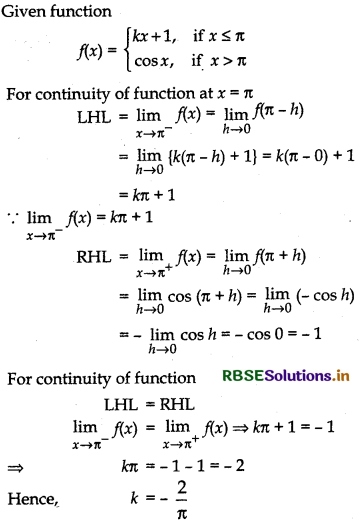
Question 29.

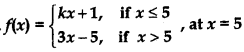
Answer:

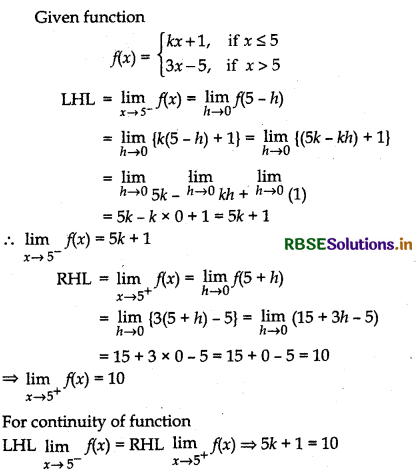
⇒ 5k = 10 - 1 = 9 ⇒ k = \(\frac{9}{5}\)
Thus, at k = \(\frac{9}{5}\) function is continuous.

Question 30.
Find the values of a and b such that the function defined by

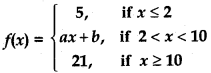
is a continuous function.
Answer:
Given function

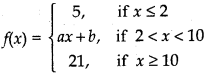
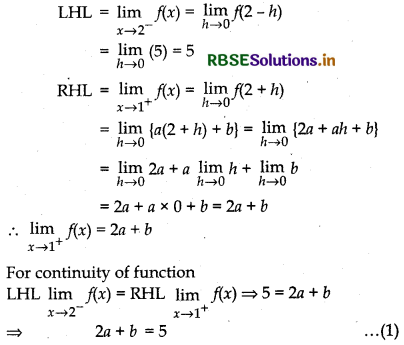
(i) For continuity of function at x = 2

(ii) For continuity of function at x = 10

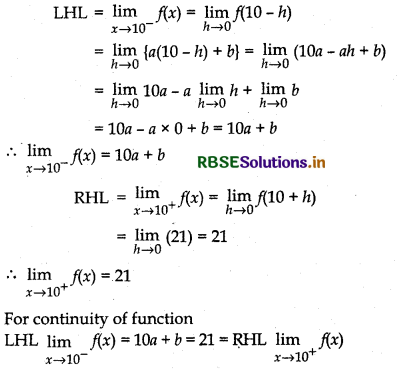
∴ 10a + b = 21
Solving equation 2a + b = 5 and 10a + b = 21
a = 2, b = 1

Question 31.
Show that the function defined by f(x) = cos x² is a continuous function.
Answer:
Given function, f(x) = cos x²
Let x = c ∈ R be any arbitrary real number, then

∴ Function is continuous at x = c.
Since, c is arbitrary real numbers.
∴ f is continuous for all real numbers.
Thus, function cos x² is continuous.
Hence proved.

Question 32.
Show that the function defined by f(x) = |cos x| is a continuous function.
Answer:
Given function, f(x) = |cos x|
Let x = c ∈ R be any arbitrary real number, then

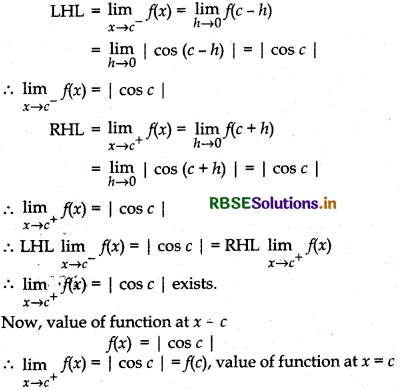
∴ Function is continuous at x = c
Since, c is an arbitrary real number. Therefore function is continuous for all real numbers.
Thus, function f(x) = |cos x| is continuous
Note: f(x) = |cos x|
If x < 0, f(x) = |cos (- x)| = |cos x| [Since, cos (- θ) = cos θ] and x > 0, f(x) = |cos x|
Hence Proved.

Question 33.
Examine that sin |x| is a continuous function.
Answer:
Given function, f(x) = sin |x|
Let x = c ∈ R be any arbitrary real number, then

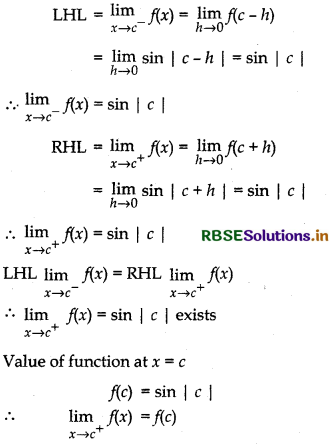
∴ Function is continuous at x = c
Since, c is an arbitrary real number. so function is continuous for all real numbers.
Thus, function sin |x| is continuous.

Question 34.
Find all the points of discontinuity of f defined by f(x) = | x | - | x + 1 |.
Answer:

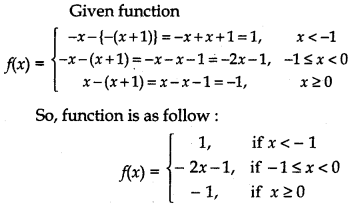
(i) For continuity at x = - 1

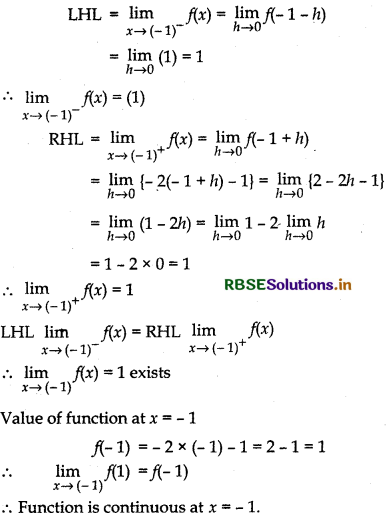
(ii) For or continuity at x = 0

∴ Function is continuous at x = 0.
Thus, there is no point of discontinuity of function.