RBSE Solutions for Class 12 Maths Chapter 4 Determinants Ex 4.5

Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 4 Determinants Ex 4.5 Textbook Exercise Questions and Answers.

RBSE Class 12 Maths Solutions Chapter 4 Determinants Ex 4.5

Question 1.
\(\left[\begin{array}{ll} 1 & 2 \\ 3 & 4 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{ll} 1 & 2 \\ 3 & 4 \end{array}\right]\)
Determinant of matrix A
|A| = \(\left[\begin{array}{ll} 1 & 2 \\ 3 & 4 \end{array}\right]\) = (1 × 4) - (3 × 2) = 4 - 6 = - 2
If A_ij is cofactor of a_ij in A, then
A₁₁ = (- 1)^{1 + 1} |4| = 4
A₁₂ = (- 1)^{1 + 2} |3| = - 3
A₂₁ = (- 1)^{2 + 1} |2| = - 2
A₂₂ = (- 1)^{2 + 2} |1| = 1
Matrix formed by the cofactors of |A| is
B (say) = \(\left[\begin{array}{cc} 4 & -3 \\ -2 & 1 \end{array}\right]\)
Now, adj A = Transpose of B = \(\left[\begin{array}{cc} 4 & -3 \\ -2 & 1 \end{array}\right]\)

Question 2.
\(\left[\begin{array}{rrr} 1 & -1 & 2 \\ 2 & 3 & 5 \\ -2 & 0 & 1 \end{array}\right]\)
Answer:

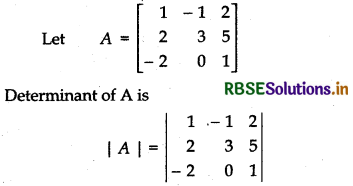
(3 × 1) - (0 × 5) + 1{2 × 1 - (- 2) × 5} + 2{2 × 0 - (- 2) × 3)
= 3 + 0 + 2 + 10 + 2(0 + 6)
= 3 + 2 + 10 + 12 = 27 ≠ 0
If A_ij is cofactor of a_ij in A, then
A₁₁ = (- 1)^{1 + 1} [(3 × 1) - (0 × 5)] = 3
A₁₂ = (- 1)^{1 + 2} [(- 1) (2 + 10)0] = - 12
A₁₃ = (- 1)^{1 + 3}[2 × 0 - (- 2) × 3] = 0 + 6 = 6
A₂₁ = (- 1)^{2 + 1} 1 = [(- 1) (- 1)] = 1
A₂₂ = (- 1)^{2 + 2} = [1 + 4] 5
A₂₃ = (- 1)^{2 + 3} = [(- 1) (- 2)] = 2
A₃₁ = (- 1)^{3 + 1} = [- 5 - 6] = - 11
A₃₂ = (- 1)^{3 + 2} = [(- 1) (5 - 4)] = - 1
A₃₃ = (- 1) ^{3 + 3} = [1 × 3 - 2(- 1)] = 3 + 2 = 5
Matrix formed by the cofactors of |A| is

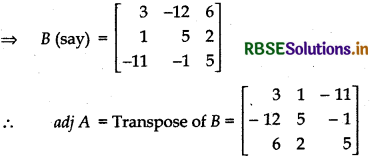
Verify A(adj A) = (adj A) = |A| I in question 3 and 4:

Question 3.
\(\left[\begin{array}{rr} 2 & 3 \\ -4 & -6 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{rr} 2 & 3 \\ -4 & -6 \end{array}\right]\)
Determinant of matrix A is
|A| = \(\left|\begin{array}{rr} 2 & 3 \\ -4 & -6 \end{array}\right|\)
= 2 × (- 6) - (- 4) × 3 = - 12 + 12 = 0
If A_ij is cofactor of a_ij in A then,
A₁₁ = (- 1)^{1 + 1} (- 6) = - 6
A₁₂ = (- 1)^{1 + 2} (- 4) = 4
A₂₁ = (- 1)^{2 + 1} (3) = - 3
A₂₂ = (- 1)^{2 + 2} (2) = 2
Matrix formed by the cofactors of |A| is

Question 4.
\(\left[\begin{array}{rrr} 1 & -1 & 2 \\ 3 & 0 & -2 \\ 1 & 0 & 3 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{rrr} 1 & -1 & 2 \\ 3 & 0 & -2 \\ 1 & 0 & 3 \end{array}\right]\)
Determinant of A is
|A| = \(\left|\begin{array}{rrr} 1 & -1 & 2 \\ 3 & 0 & -2 \\ 1 & 0 & 3 \end{array}\right|\)
Expanding along C₂, we get
|A| = {3 × 3 - 1 × (- 2)} + 0 + 0
∴ |A| = (9 + 2) = 11
A₁₁ = (- 1)^{1 + 1} [(0 × 3 - 0 × (- 2)] = 3
A₁₂ = (- 1)^{1 + 2} [(3 × 3 - 1) × (- 2 )] = (- 1) (9 + 2) = - 11
A₁₃ = (- 1)^{1 + 3}[(3 × 0 - 1 × 0)] = 0
A₂₁ = (- 1)^{2 + 1} 1 = [(- 1 × 3 - 0 × 2)] = (- 1) (- 3) = 3
A₂₂ = (- 1)^{2 + 2} = [1 × 3 - 1 × 2] = 3 - 2 = 1
A₂₃ = (- 1)^{2 + 3} = [1 × 0 - 1 × (- 1)] = - 1(0 + 1) = - 1
A₃₁ = (- 1)^{3 + 1} = {- 1 × (- 2) - 0 × 2} = 2
A₃₂ = (- 1)^{3 + 2} = {1 × (- 2) - 3 × 2} = (- 1) (- 2 - 6) = (- 1) (- 8) = 8
A₃₃ = (- 1) ^{3 + 3} = [1 × 0 - 3 × (- 1)] = 8
Matrix formed by the cofactors of |A| is:

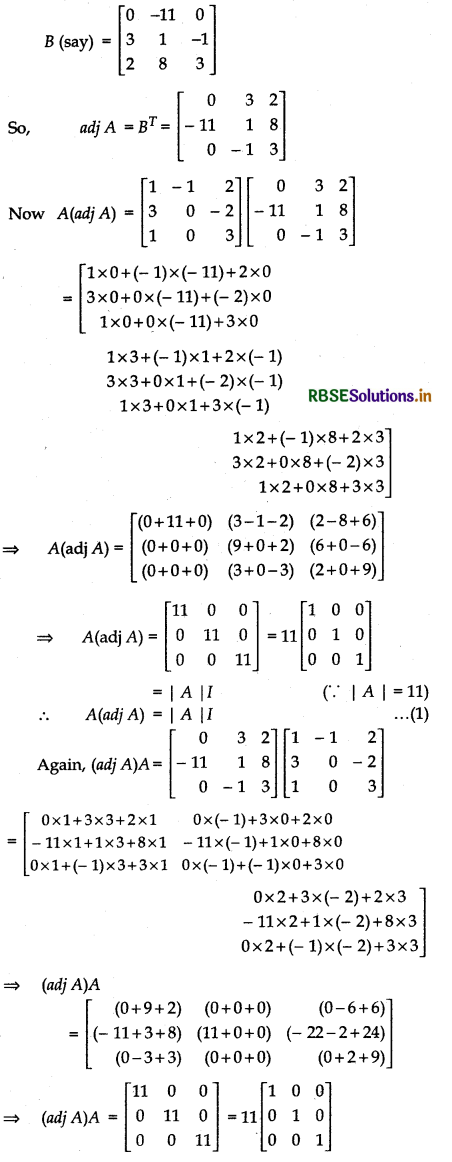
= |A| (∵ |A| = 11)
∴ (adj A)A = |A|I ........ (2)
From (1) and (2), we get
A(adj A) = (adj A)A = |A|I
Hence Proved.

Find the inverse of each of the matrices (if it exists) given in questions 5 to 11:

Question 5.
\(\left[\begin{array}{rr} 2 & -2 \\ 4 & 3 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{rr} 2 & -2 \\ 4 & 3 \end{array}\right]\)
Determinant of A is:
|A| = \(\left|\begin{array}{rr} 2 & -2 \\ 4 & 3 \end{array}\right|\)
= 2 × 3 - 4 × (- 2) = 6 + 8 = 14 ≠ 0
Then, matrix A is non-singular hence A^-1 exists.
If A_ij is cofactor of a_ij in A, then
A₁₁ = (- 1)^{1 + 1} |3| = 3
A₁₂ = (- 1)^{1 + 2} = - 4
A₂₁ = (- 1)^{2 + 1} |- 2| = - (- 2) = 2
A₂₂ = (- 1)^{2 + 2} |2| = 2
Matrix formed by the cofactor of A is

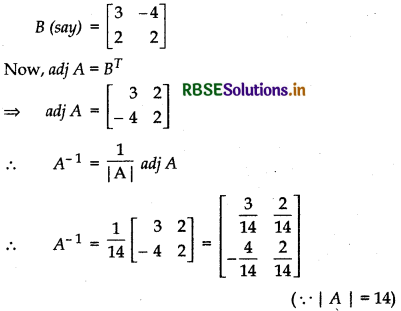
Question 6.
\(\left[\begin{array}{ll} -1 & 5 \\ -3 & 2 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{ll} -1 & 5 \\ -3 & 2 \end{array}\right]\)
Determinant of matrix A is
|A| = \(\left|\begin{array}{ll} -1 & 5 \\ -3 & 2 \end{array}\right|\)
= - 1 × 2 - (- 3) × 5 = - 2 + 15 = 13 ≠ 0
Then matrix A is non-singular hence A^-1 exists.
If A_ij is cofactor of a_ij in A, then
A₁₁ = (- 1)^{1 + 1} (2) = 2
A₁₂ = (- 1)^{1 + 2} (- 3) = - (- 3) = 3
A₂₁ = ( - 1)^{2 + 1} (5) = - 5
A₂₂ = (- 1)^{2 + 2} (- 1) = - 1
Matrix formed by the cofactor of A is

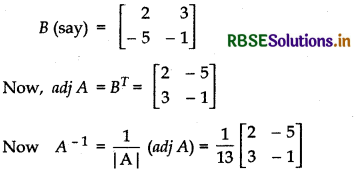
Question 7.
\(\left[\begin{array}{lll} 1 & 2 & 3 \\ 0 & 2 & 4 \\ 0 & 0 & 5 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{lll} 1 & 2 & 3 \\ 0 & 2 & 4 \\ 0 & 0 & 5 \end{array}\right]\)
Determinant of matrix A is
|A| = \(\left[\begin{array}{lll} 1 & 2 & 3 \\ 0 & 2 & 4 \\ 0 & 0 & 5 \end{array}\right]\)
Expanding along C₁
= 1(2 × 5 - 0 × 4) = 10 ≠ 0
Thus, matrix A is non-singuiar, i.e., A ^-1 exists.
If A_ij is cofactor of in a_ij in A, then
A₁₁ = (- 1)^{1 + 1}[2 × 5 - 0 × 4] = 10
A₁₂ = (- 1)^{1 + 2}[(0 × 5 - 0 × 4)] = 0
A₁₃ = (- 1)^{1 + 3}[0 × 0 - 0 × 2)] = 0
A₂₁ = (- 1)^{2 + 1}[(- 1) (2 × 5 - 0 × 3)] = - 10
A₂₂ = (- 1)^{2 + 2}[(1 × 5 - 0 × 3)] = 5
A₂₃ = (- 1)^{2 + 3}[(- 1) (1 × 0 - 0 × 2)] = 0
A₃₁ = (- 1)^{3 + 1}[2 × 4 - 2 × 3 = 8 - 6] = 2
A₃₂ = (- 1)^{3 + 2}[(- 1) (1 × 4 - 0 × 3)] = - 4
A₃₃ = (- 1)^{3 + 3}[(1 × 2 - 0 × 2)] = 2
Matrix formed by the cofactors of |A| is

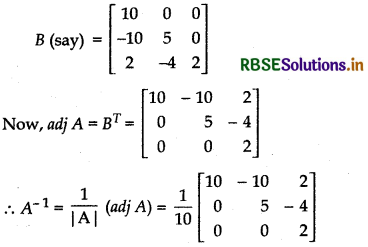
Question 8.
\(\left[\begin{array}{rrr} 1 & 0 & 0 \\ 3 & 3 & 0 \\ 5 & 2 & -1 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{rrr} 1 & 0 & 0 \\ 3 & 3 & 0 \\ 5 & 2 & -1 \end{array}\right]\)
= 1{3 × (- 1) - 2 × 0} - 0 + 0 = - 3 ≠ 0
So, matrix A is non-singular, ie., A^-1 exists.
If A_ij is cofactor of a_ij in A, then
A₁₁ = [3 × (- 1) - 2 × 0] = - 3
A₁₂ = - [3 × (- 1) - 5 × 0] = - 1 (- 3) = 3
A₁₃ = + [3 × 2 - 5 × 3] = 6 - 15 = - 9
A₂₁ = - [0 × (- 1) - 2 × 0] = 0
A₂₂ = +[1 × (- 1) - 5 × 0] = - 1
A₂₃ = - [(1 × 2 - 5 × 0)] = - 2
A₃₁ = +[0 × 0 - 3 × 0] = 0
A₃₂ = - [(1 × 0 - 3 × 0)] = 0
A₃₃ = +[1 × 3 - 3 × 0] = 3
Matrix formed by the cofactors of |A| is

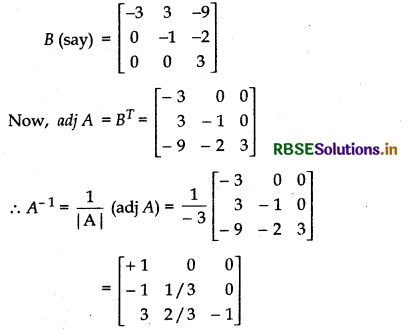
Question 9.
\(\left[\begin{array}{rrr} 2 & 1 & 3 \\ 4 & -1 & 0 \\ -7 & 2 & 1 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{rrr} 2 & 1 & 3 \\ 4 & -1 & 0 \\ -7 & 2 & 1 \end{array}\right]\)
Determinant of matrix A is
|A| = \(\left[\begin{array}{rrr} 2 & 1 & 3 \\ 4 & -1 & 0 \\ -7 & 2 & 1 \end{array}\right]\)
= 2(- 1 × 1 - 2 × 0) - 1(4 × 1 - (- 7) × 0) + 3{4 × 2 - (- 7) × (- 1)}
= - 2 - 4 + 3(8 - 7) = - 2 - 4 + 3 = - 3 ≠ 0
So, matrix A is non-singular, i.e., A^-1 exists.
If A_ij is cofactor of a_ij in A, then
A₁₁ = + [- (1 × 1) - (2 × 0)] = - 1
A₁₂ = - [4 × 1 - (- 7) × 0] = - 4
A₁₃ = +[4 × 2 - (- 7) × (- 1)] = 8 - 7 = 1
A₂₁ = - [(1 × 1 - 2 × 3)] = (- 1) (1 - 6) = 5
A₂₂ = +[2 × 1 - (- 7) × 3] = 2 + 21 = 23
A₂₃ = - [2 × 2 - (- 7) × 1] = (- 1) (4 + 7) = - 11
A₃₁ = + [1 × 0 - (- 1) × 3] = 3
A₃₂ = - [(2 × 0 - 4 × 3)] = (- 1) (- 12) = 12
A₃₃ = + [(2 × (- 1) - 4 × 1] = - 2 - 4 = - 6
Matrix formed by the cofactors of |A| is

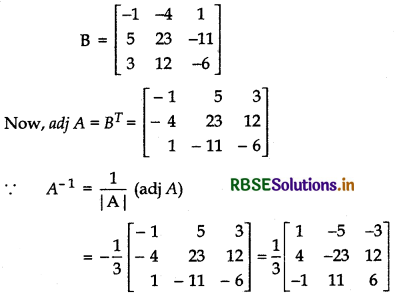
Question 10.
\(\left[\begin{array}{rrr} 1 & -1 & 2 \\ 0 & 2 & -3 \\ 3 & -2 & 4 \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{rrr} 1 & -1 & 2 \\ 0 & 2 & -3 \\ 3 & -2 & 4 \end{array}\right]\)
Determinant of matrix A is
|A| = \(\left[\begin{array}{rrr} 1 & -1 & 2 \\ 0 & 2 & -3 \\ 3 & -2 & 4 \end{array}\right]\)
= 1{2 × 4 - (- 2) × (- 3)} + 1{0 × 4 - 3 × (- 3)} + 2{0 × (- 2) - 3 × 2}
= (8 - 6) + (0 + 9) + 2(- 6)
= 2 + 9 - 12 = - 1 ≠ 0
So, matrix A is non-singular, i.e., A^-1 exists.
If A_ij is cofactor of a_ij in A, then
A₁₁ = (- 1)^{1 + 1}[2 × 4 - (- 2) × (- 3)] = 8 - 6 = 2
A₁₂ = (- 1)^{1 + 2} [0 × 4 - 3 × (- 3)] = - 1(+ 9) = - 9
A₁₃ = (- 1)^{1 + 3} [0 × (- 2) - 3 × 2] = - 6
A₂₁ = (- 1)^{2 + 1} [- 1 × 4 - (- 2) × 2] = (- 1) (- 4 + 4) = 0
A₂₂ = (- 1)^{2 + 2} [1 × 4 - 3 × 2] = 4 - 6 = - 2
A₂₃ = (- 1)^{2 + 3} [1 × (- 2) - 3 × (- 1)] = (- 1) (- 2 + 3) = - 1
A₃₁ = (- 1)^{3 + 1} [(- 1) × (- 3) - 2 × 2] = 3 - 4 = - 1
A₃₂ = (- 1)^{3 + 2} [1 × (- 3) - 0 × 2] = (- 1) (- 3) = 3
A₃₃ = (- 1)^{3 + 3} [1 × 2 - 0 × (- 1)] = 2
Matrix formed by the cofactors of |A| is

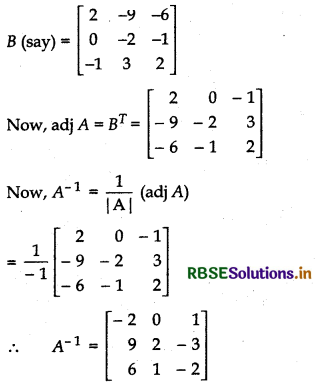
Question 11.
\(\left[\begin{array}{ccc} 1 & 0 & 0 \\ 0 & \cos \alpha & \sin \alpha \\ 0 & \sin \alpha & -\cos \alpha \end{array}\right]\)
Answer:
Let A = \(\left[\begin{array}{ccc} 1 & 0 & 0 \\ 0 & \cos \alpha & \sin \alpha \\ 0 & \sin \alpha & -\cos \alpha \end{array}\right]\)
Determinant of matrix A is
|A| = \(\left|\begin{array}{ccc} 1 & 0 & 0 \\ 0 & \cos \alpha & \sin \alpha \\ 0 & \sin \alpha & -\cos \alpha \end{array}\right|\)
= 1{cos α × (- cos α) - sin α (sin α)} - 0 + 0
= - cos² α - sin² a = - (cos² α + sin² α)
∴ | A | = - 1 ≠ 0
So, matrix A is non-singular, i.e., A^-1 exists.
If A_ij is cofactor of a_ij in A, then
A₁₁ = +[cos α (- cos α - sin α (sin α)] = - cos² α - sin² α = - (cos² α + sin² α) = - 1
A₁₂ = - {0 × (- cos α) - 0 × (sin α)} = 0
A₁₃ = + [0 × sin α - 0 × cos α] = 0
A₂₁ - [0 × (- cos α) - 0 × sin α] = 0
A₂₂ = + [1 × (- cos α) - 0 × 0] = - cos α
A₂₃ = - [(1 × sin α - 0 × 0)] = - sin α
A₃₁ = + [0 × sin α - cos α × 0] = 0
A₃₂ = - [(1 × sin α - 0 × 0)] = - sin α
A₃₃ = + [1 × cos α - 0 × 0] = cos α
Matrix formed by the cofactors of |A| is

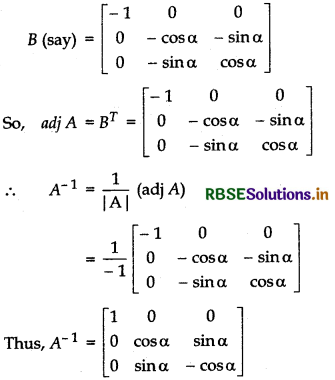
Question 12.
If A = \(\left[\begin{array}{ll} 3 & 7 \\ 2 & 5 \end{array}\right]\) and B = \(\left[\begin{array}{ll} 6 & 8 \\ 7 & 9 \end{array}\right]\), then verify that: (AB)^-1 = B^-1A^-1
Answer:
Let A = \(\left[\begin{array}{ll} 3 & 7 \\ 2 & 5 \end{array}\right]\)
Determinant of matrix A is
|A| = \(\left|\begin{array}{ll} 3 & 7 \\ 2 & 5 \end{array}\right|\)
= 3 × 5 - 2 × 7 = 15 - 14 = 1 ≠ 0
So, matrix A is non-singular, i.e., A^-1 exists.
If A_ij is cofactor of a_ij in A, then
A₁₁ = (- 1)^{1 + 1} (5) = 5
A₁₂ = (- 1)^{1 + 2} (2) = - 2
A₂₁ = (- 1)^{2 + 1} (7) = - 7
A₂₂ = (- 1)^{2 + 2} (3) = 3
Matrix formed by the cofactors of |A| is

Determinant of matrix B is
|B| = \(\left|\begin{array}{ll} 6 & 8 \\ 7 & 9 \end{array}\right|\) = 6 × 9 - 7 × 8 = - 2 ≠ 0
∴ Matrix B is non-singular so B^-1 exists.
If B_ij is cofactor of b_ij in B, then
B₁₁ = (- 1)^{1 + 1} (9) = 9
B₁₂ = (- 1)^{1 + 2} (7) = - 7
B₂₁ = (- 1)^{2 + 1} (8) = - 8
B₂₂ = (- 1)^{2 + 2} (6) = 6
Matrix formed by the cofactor of |B| is

Question 13.
If A = \(\left[\begin{array}{rr} 3 & 1 \\ -1 & 2 \end{array}\right]\), show that A² - 5A + 7I = 0. Hence, find A^-1.
Answer:

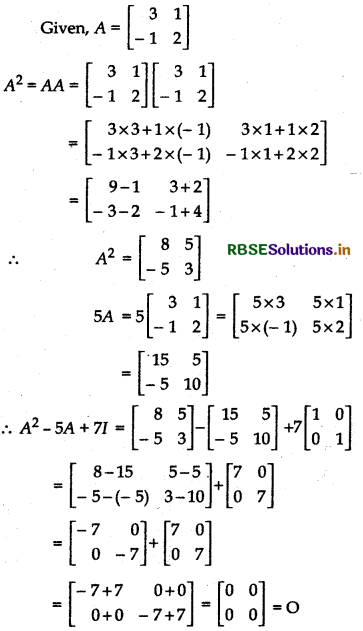
∴ A² - 5A + 7I = 0
⇒ A² - 5A = - 7I
⇒ AA - 5A = - &I
Pre-multiplying both sides by A^-1
(A^-1A)A - 5A^-1A = - 7A^-1I
⇒ IA - 5I = - 7A^-1 (∵ A^-1A = I, A^-1I = A^-1)

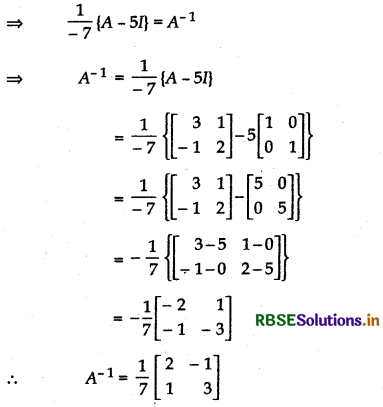
Question 14.
For the matrix A = \(\left[\begin{array}{ll} 3 & 2 \\ 1 & 1 \end{array}\right],\) find the numbers a and b such that A² + aA + bI = 0.
Answer:

⇒ 11 + 3a + b = 0 ........ (1)
8 + 2a = 0 .......... (2)
4 + a = 0 ............ (3)
3 + a + b = 0 .......... (4)
Now, from equation (3)
4 + a = 0
⇒ a = - 4
Putting a = - 4 in 3 + a + b = 0
3 - 4 + b = 0
⇒ - 1 + b = 0 ⇒ b = 1
Thus a = - 4, b = 1
By a = - 4, b = 1 solving 11 + 3a + b = 0 and 8 + 2a = 0.
11 + 3 × (- 4) + 1 = 11 - 12 + 1 = 0
And 8 + 2 × (- 4) = 8 - 8 = 0
Thus, a and b satisfies 11 + 3a + b = 0 and 8 + 2a = 0.

Question 15.
For the matrix A = \(\left[\begin{array}{rrr} 1 & 1 & 1 \\ 1 & 2 & -3 \\ 2 & -1 & 3 \end{array}\right]\), show that A³ - 6A² + 5A + 11I = 0. Hence, find A^-1.
Answer:


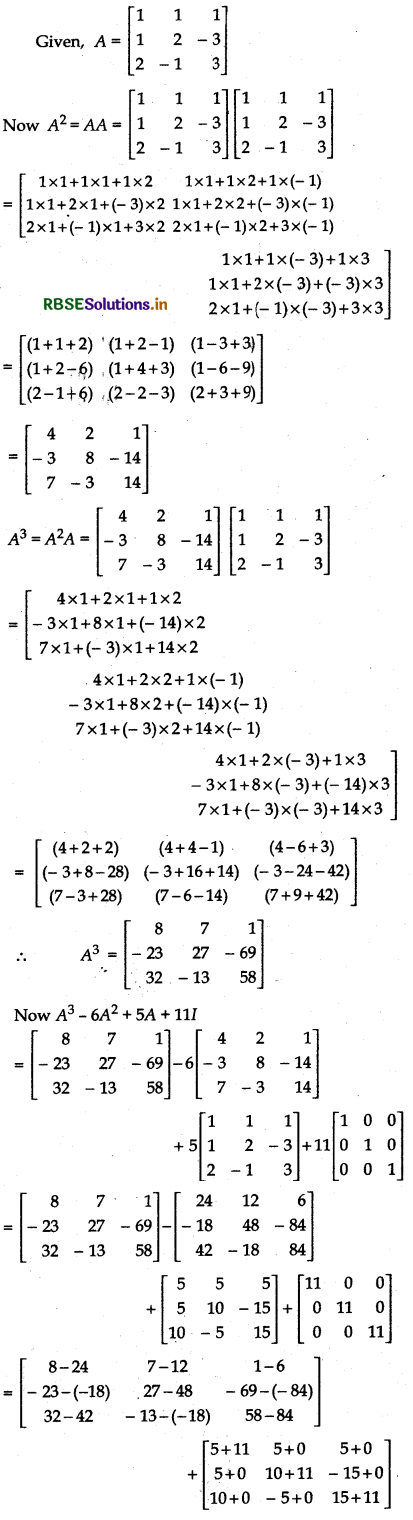
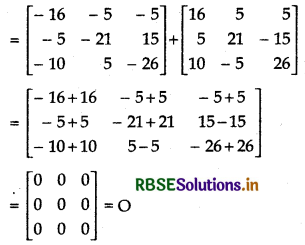
Thus, A³ - 6A² + 5A + 11I = 0
Multiplying equation A³ - 6A² + 5A + 11I = 0 by A^-1
(A^-1A)A² - 6(AA^-1)A + 5(A^-1A) + 11(A^-1I) = A^-1 = 0
⇒ IA² - 6IA + 5I + 11A^-1 = 0
[∵ A^-1 A = I, A^-1I = A^-1, A^-10 = 0]
⇒ A² - 6A + 5I + 11A^-1 = 0, (∵ IA = A)
⇒ 11A^-1 = - A² + 6A - 5I

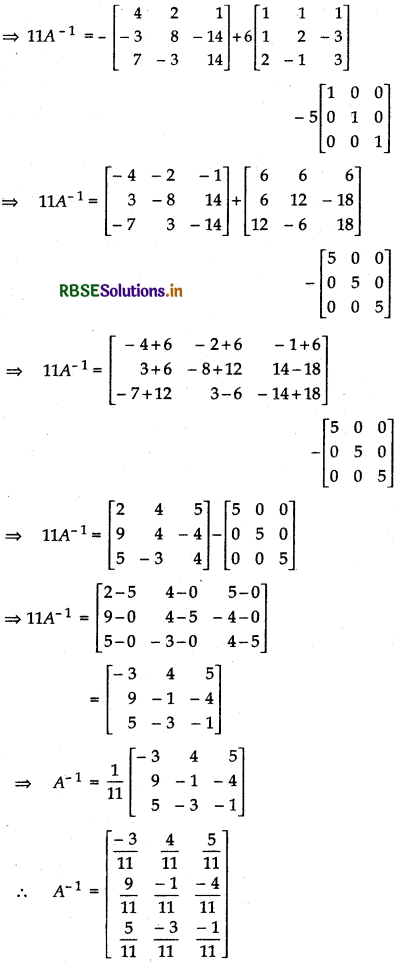
Question 16.
If A = \(\left[\begin{array}{rrr} 2 & -1 & 1 \\ -1 & 2 & -1 \\ 1 & -1 & 2 \end{array}\right]\), verify that A³ - 6A² + 9A - 4I = 0 and hence find A^-1.
Answer:

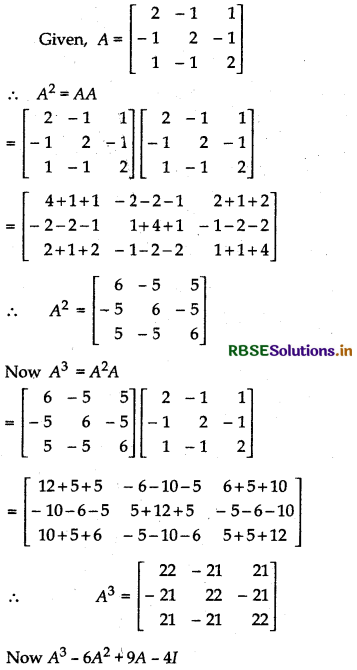
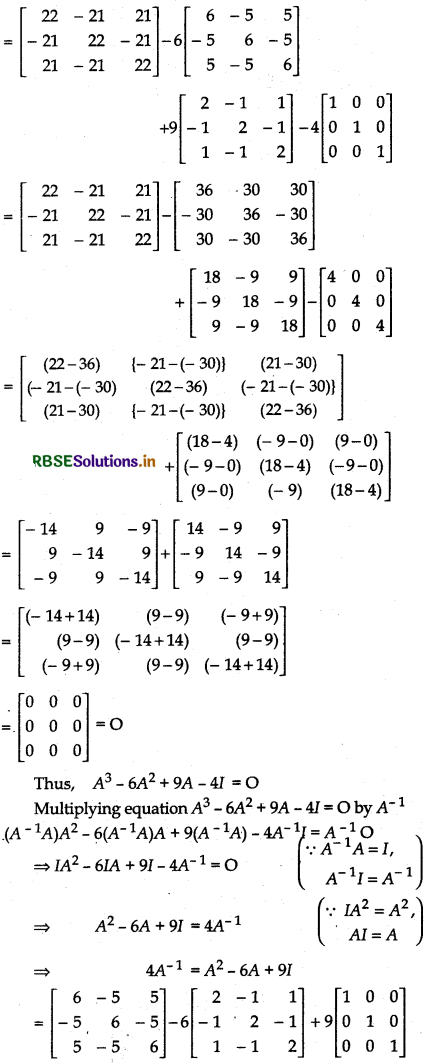
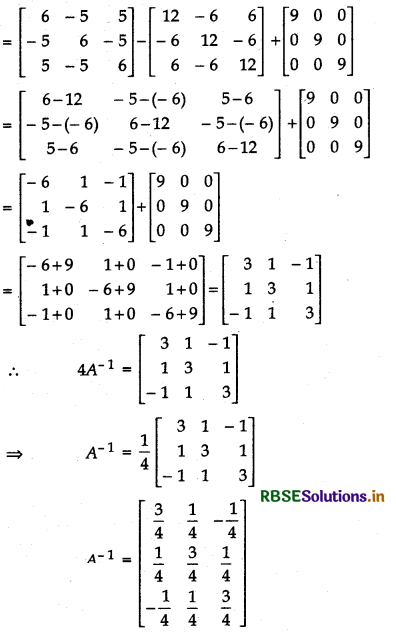
Question 17.
If A be a non-singular square matrix of order 3 × 3, then | adj A | is equal to:
(A) | A |
(B) | A |²
(C) | A |³
(D) 3| A |
Answer:
Given, A be a square matrix of order n × n, then
| adj A |=| A |^{n - 1}
Here n = 3
∴ |adjA | = | A |^{3 - 1} = | A |²
Thus, option (B) is correct.

Question 18.
If A be an invertible matrix of order 2, then det (A^-1) is equal to:
(A) det
(B) \(\frac{1}{{det}(A)}\)
(C) 1
(D) 0
Answer:
Since, A is invertible matrix so its determinant value will not be zero, i.e.,
|A| ≠ 0
∴ AA^-1 = 1
⇒ |AA^-1| = | I | = 1
⇒ |A| |A^-1| = 1 (∵ |AB| = |A| |B|)
⇒ |A^-1| = \(\frac{1}{|A|}\)
⇒ det(A^-1) = \(\frac{1}{{det}(A)}\)
Thus, option (B) is correct.