RBSE Solutions for Class 12 Maths Chapter 3 Matrices Miscellaneous Exercise

Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 3 Matrices Miscellaneous Exercise  Textbook Exercise Questions and Answers.

RBSE Class 12 Maths Solutions Chapter 3 Matrices Miscellaneous Exercise 

Question 1.
Let A = \(\left[\begin{array}{ll} 0 & 1 \\ 0 & 0 \end{array}\right]\), then show that 
(aI + bA)ⁿ = aⁿI + na^{n - 1}BA
Where I is identity matrix of order 2 and n ∈ N.
Answer:

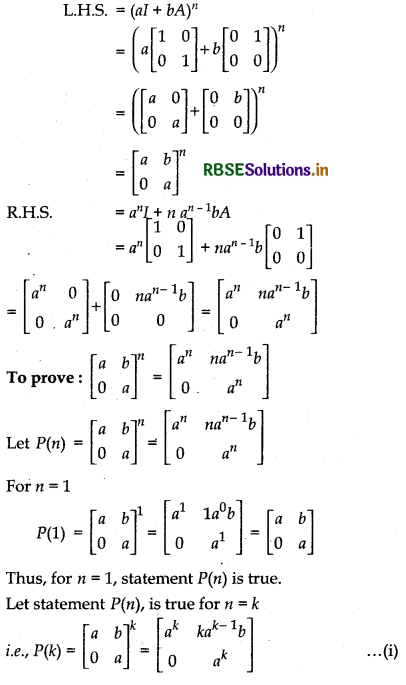
Now we will show that statement is true for n = k + 1 also i.e.,

i.e., statement P(n) is true for n = k + 1.
Thus, according to principle of mathematical induction statement P(n) is true for ∀ n ∈ N.
⇒ \(\left[\begin{array}{ll} a & b \\ 0 & a \end{array}\right]^{n}=\left[\begin{array}{cc} a^{n} & n a^{n-1} b \\ 0 & a^{n} \end{array}\right]\)
∴ (aI + bA)ⁿ = aⁿI + na^{n - 1}bA
Hence Proved.

Question 2.
If A = \(\left[\begin{array}{lll} 1 & 1 & 1 \\ 1 & 1 & 1 \\ 1 & 1 & 1 \end{array}\right]\), then prove that Aⁿ = \(\left[\begin{array}{lll} 3^{n-1} & 3^{n-1} & 3^{n-1} \\ 3^{n-1} & 3^{n-1} & 3^{n-1} \\ 3^{n-1} & 3^{n-1} & 3^{n-1} \end{array}\right]\), n ∈ N
Answer:

Thus, statement P(n), is true for n = 1
Let statement is true for n = k i.e.,
P(k) = A^k = \(\left[\begin{array}{lll} 3^{k-1} & 3^{k-1} & 3^{k-1} \\ 3^{k-1} & 3^{k-1} & 3^{k-1} \\ 3^{k-1} & 3^{k-1} & 3^{k-1} \end{array}\right]\)
Now , we will prove that the statement is true for n = k + 1 also.

∴ Statement P(n), is true for n = k + 1, while it is true for n = k.
Thus, according to the principle of mathematical induction, given statement is true for all positive integer n ∈ N.
Hence Proved.

Question 3.
If A = \(\left[\begin{array}{ll} 3 & -4 \\ 1 & -1 \end{array}\right]\), then prove that Aⁿ = \(\left[\begin{array}{cc} 1+2 n & -4 n \\ n & 1-2 n \end{array}\right]\), when n is any positive integer.
Answer:

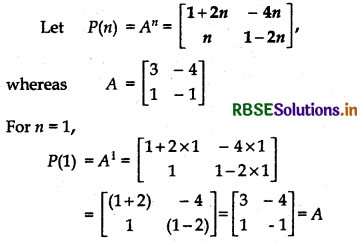
Thus, statement P(n), is true for n = 1.
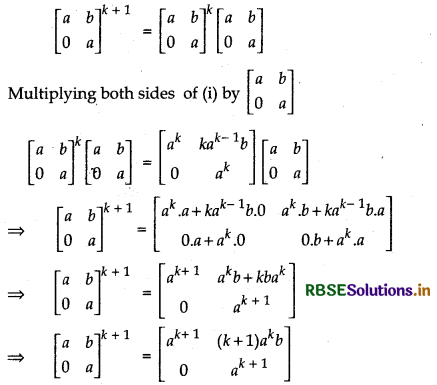
Let statement is true for n = k, i.e.,
p(k) = A^k = \(\left[\begin{array}{cc} 1+2 k & -4 k \\ k & 1-2 k \end{array}\right]\) ......... (i)
Now, we will show that statement is true for n = k + 1, Multiplying both sides of Eq. (i) by A
A^kA = A^{k + 1}

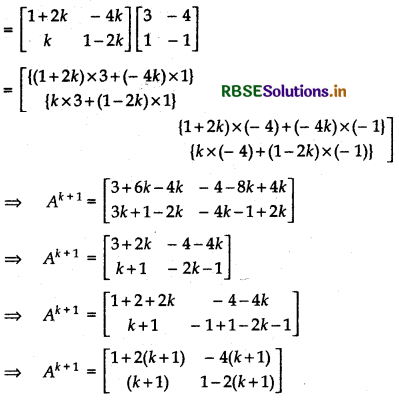
∴ Statement P(n) is true for n = k + 1 also, when it is true for n = k
Thus, according to principle of mathematical induction given statement is true for all positive integers n ∈ N.
Hence Proved.

Question 4.
If A and B are symmetric matrices, prove that AB - BA is a skew-symmetric matrix.
Answer:
We have, A and B are symmetric matrices.
So, A’ = A and B’ = B
(AB - BA)’ = (AB)’ - (BA)’ [∵ (X - Y)’ = X’ - Y’]
= B’A’ - A’B’ [∵ (XY)’ = Y’X']
= BA - AB (∵ A’ = A, B’ = B)
= - (AB - BA)
= skew-symmetric
Thus, (AB - BA) is a skew-symmetric matrix.
Hence Proved.

Question 5.
Show that matrix B’AB is symmetric or skew symmetric according as A is symmetric or skew-symmetric.
Answer:
(i) Let A is symmetric matrix, then
A’ = (-A)
∴ (B’ AB)’ = (B’(AB))’
= (AB)’ (B’)’ [By De Morgon’s law]
⇒ (B’AB)’ = (AB)’B [∵ (B')' = B']
⇒ (B’AB)’ = B’A’B [∵ (AB)' = B'A']
⇒ (B’AB)’ = B’AB [∵ A' = A]
⇒ (B’AB) is a symmetric matrix.
Hence proved

(ii) Let A is a skew-symmetric matrix.
∴ A’ = - A
Now (B’(AB))’ = (AB)’ (B’)’
= (B’A’)B (∵ (B')' = B)
= B’(- A)B (∵ A' = - A)
= - (B’AB)
∴ B’AB is a skew-symmetric matrix.
Hence Proved.

Question 6.
Find the values of x, y and z if matrix A = \(\left[\begin{array}{ccc} 0 & 2 y & z \\ x & y & -z \\ x & -y & z \end{array}\right]\) satisfies the equation A'A = I.
Answer:
We have

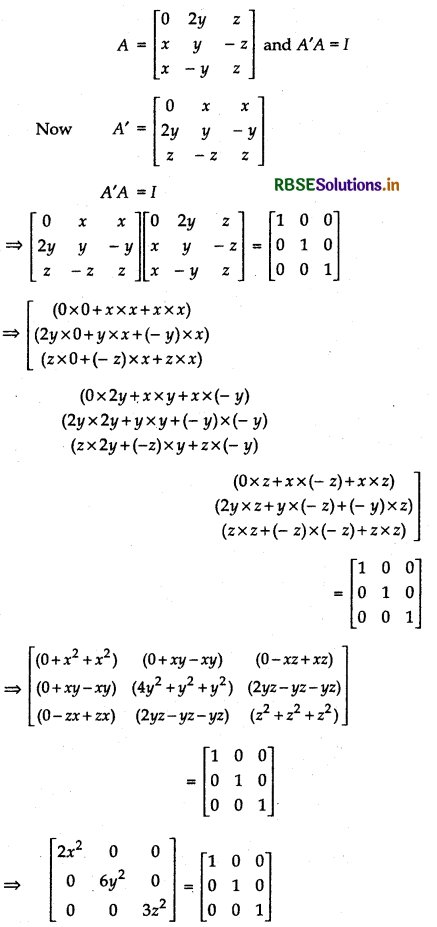
∴ Comparing corresponding elements in both sides.
2x² = 1, 6y² = 1, 3z² = 1
∴ x = ±\(\frac{1}{\sqrt{2}}\), y = ±\(\frac{1}{\sqrt{6}}\), z = ±\(\frac{1}{\sqrt{3}}\)

Question 7.
For what values of x:
[1 2 1] \(\left[\begin{array}{lll} 1 & 2 & 0 \\ 2 & 0 & 1 \\ 1 & 0 & 2 \end{array}\right]\left[\begin{array}{l} 0 \\ 2 \\ x \end{array}\right]\) = 0?
Answer:

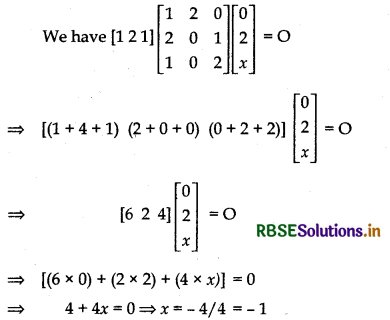
Question 8.
If A = \(\left[\begin{array}{rr} 3 & 1 \\ -1 & 2 \end{array}\right]\), show that A² - 5A + 7I = 0
Answer:

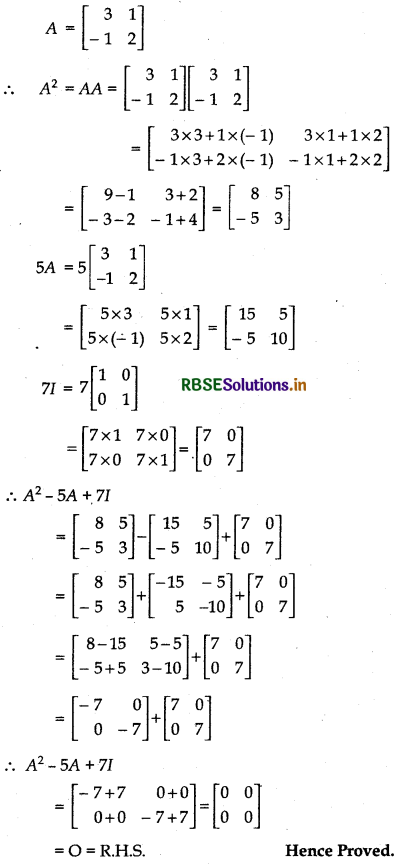
Question 9.
Find x, if
[x - 5 - 1] \(\left[\begin{array}{lll} 1 & 0 & 2 \\ 0 & 2 & 1 \\ 2 & 0 & 3 \end{array}\right]\left[\begin{array}{l} x \\ 4 \\ 1 \end{array}\right]\) = 0
Answer:
we have

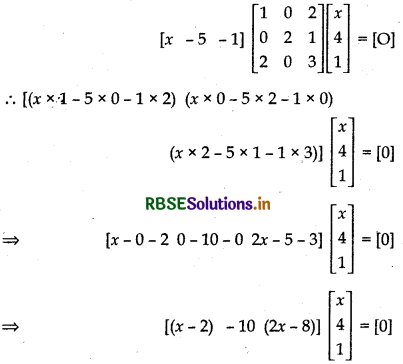
⇒ [(x - 2) × x - 10 × 4 + (2x - 8) × 1] = [0]
⇒ [x² - 2x - 40 + 2x - 8] = [0]
⇒ x² - 48 = 0
⇒ x² = 48 ⇒ x² = 16 × 3 ⇒ x = ± 4√3.

Question 10.
A manufacturer produces three products x, y, z which he sells in two markets. Annual sales are indicated below:

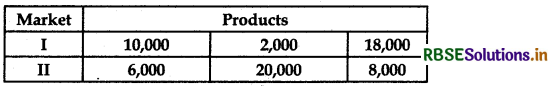
(a) If unit sale prices of x, y and z are ₹ 2.50, ₹ 1.50 and ₹ 1.00 respectively, find the total revenue in each market with the help of matrix algebra.
(b) If the unit costs of the above three commodities are ₹ 2.00, ₹ 1.00 and 50 paise respectively. Find the gross profit.
Answer:
Annual sales of products are as follows:

Sale prices of each unit of production x, y, z are ₹ 2.50, ₹ 1.50 and ₹ 1.00 respectively. Writing this in matrix form
\(\left[\begin{array}{l} 2.50 \\ 1.50 \\ 1.00 \end{array}\right]\)

(a) Income of both markets is obtained by multiplying both matrices. Thus,

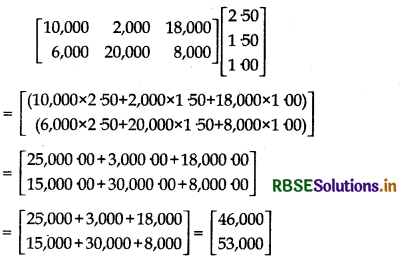
Thus, income from each market is ₹ 46,000 and ₹ 53,000.

(b) When unit costs of each product x, y, z are ₹ 2.00, ₹ 1.00 and ₹ 0.50, then writing them in matrix form.
\(\left[\begin{array}{l} 2.00 \\ 1.00 \\ 0.50 \end{array}\right]\)
Thus, cost price in market will be obtained as:

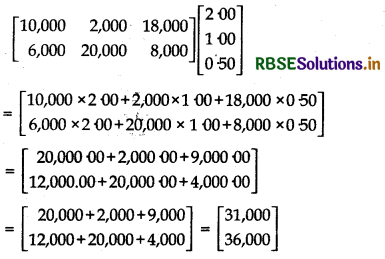
Profit of 1^st market
= selling price - cost price
= 46,000 - 31,000 = ₹ 15,000
Profit of II^nd market
= 53,000 - 36000 = ₹ 17,000

Question 11.
Find the matrix X so that X
X\(\left[\begin{array}{lll} 1 & 2 & 3 \\ 4 & 5 & 6 \end{array}\right]=\left[\begin{array}{rrr} -7 & -8 & -9 \\ 2 & 4 & 6 \end{array}\right]\)
Answer:
We have

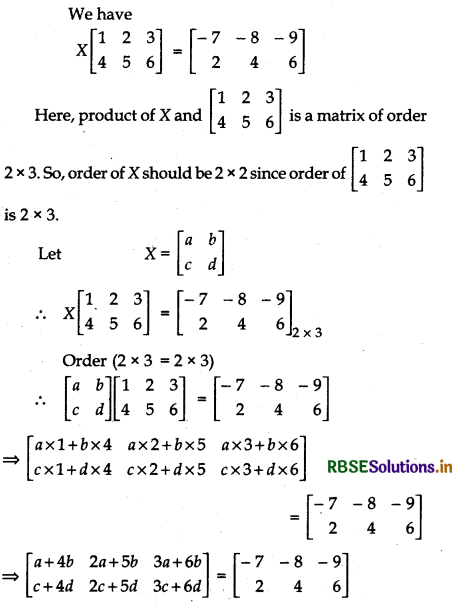
Comparing corresponding elements of both sides.
a + 4b = - 7 ...... (i)
2a + 5b = - 8 ....... (ii)
3a + 9 = - 9 ........... (iii)
c + 4d = 2 .......... (v)
2c + 5d = 4 ....... (v)
3c + 6d = 6 ........ (vi)
Multiply equation (i) by 2 and then subtracting equation

(ii) from it

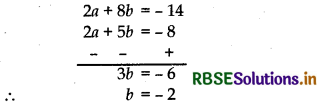
Substituting value of b in euqation (iii), we get
3a + 6 × (- 2) = - 9
⇒ 3a - 12 = - 9
⇒ 3a = 12 - 9
⇒ 3a = 3
∴ a = 1
Multiplying Eq. (iv) by 2 and then substracting Eq. (v) from it

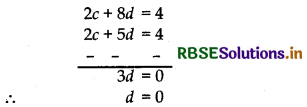
Putting value of d in equation (vi), we get
3c + 6 × 0 = 6
⇒ 3c =6
∴ c = 2
Now, putting values of a, b, c and d in \(\left[\begin{array}{ll} a & b \\ c & d \end{array}\right]\),
Thus, X = \(\left[\begin{array}{rr} 1 & -2 \\ 2 & 0 \end{array}\right]\)

Question 12.
If A and B are square matrices of the same order such that AB = BA, then prove by induction that ABⁿ = BⁿA. Further, prove that (AB)ⁿ = AⁿBⁿ for all n ∈ N.
Answer:
Let P(n) = ABⁿ = BⁿA
where AB = BA
For n = 1
P(n) = AB¹ = AB = BA (Given)
∴ For n = 1, statement P(n) is true
Let for n = k, statement is true, i.e.,
P(k) = AB^k = B^kA ....... (i)
Now, we will show that statement is true for n = k + 1 also.
Multiplying both sides of Eq. (i) by B.
L.H.S. = AB^kB = AB^{k + 1}
and R.H.S. = (B^kA)B = B^k(AB) .
(By associativity of multiplication of matrices)
= B^k(BA) (∵ AB = BA)
= (B^kB)A .
(By associativity of multiplication of matrices)
= B^{k + 1}A
∴ AB^{k + 1} = B^{k + 1}A (AB = BA, given)
⇒ P(n), is true for n = k + 1.
Thus, by the principle of mathematical induction, given statement is true for all positive integers n ∈ N.
Again, let P(n) : (AB)ⁿ = AⁿBⁿ
Puffing n = 1
P(n):(AB)¹ = A¹B¹
⇒ AB = AB
Thus, given statement is true for n = 1.
Let given statem. is true for n = k
i.e., P(k) : (AB)^nk = A^kB^k .......... (ii)
Multiplying both sides of Eq. (ii) by AB.
L.H.S. = (AB)^k (AB) = (AB)^{k + 1}
R.H.S. = (A^kB^k) (AB) = A^kB^k (BA) (∵ AB = BA)
= A^k (B^kB)A
(By associativity of multiplication of matrices)
= A^k (B^{k + 1}A)
= A^k (AB^{k + 1}) [∵ AB^k = B^kA]
= (A^kA) B^{k + 1} = A^{k + 1} B^{k + 1}
(By associativity of multiplication of matrices)
Thus (AB)^{k + 1} = ^{k + 1} B^{k + 1}
∴ Given statement P(n), is true for n = k + 1 also it is true for n = k.
Thus, by the principle of mathematical induction, given statement is true for all positive integers n ∈ N.
Hence Proved.

Choose the correct answer in the following questions:

Question 13.
If A = \(\left[\begin{array}{rr} \alpha & \beta \\ \gamma & -\alpha \end{array}\right]\) is such that A² = I, then:
(A) 1 + α² + βγ = 0
(B) 1 - α² + βγ = 0
(C) 1 - α² - βγ = 0
(D) 1 + α² - βγ = 0
Answer:

Comparing elements of both sides,
α² + βγ = 1 or γβ + α² = 1
∴ 1 - α² - βγ = 0
Thus, option (C) is correct.

Question 14.
If the matrix A is both symmetric and skew-symmetric, then
(A) A is a diagonal matrix
(B) A is a zero matrix
(C) A is a square matrix
(D) None of these
Answer:
Let A = [a_ij]
For symmetric matrix
a_ij = a_ji ......... (i)
For skew-symmetric matrix
a_ij = - a_ji ........... (ii)
Since, given matrix is symmetric and skew-symmetric both. Thus, it will satisfy (i) and (ii) together.
Adding (i) and (ii),
a_ij + a_ij = a_ji - a_ji
⇒ 2_ij = 0
⇒ a_ij = 0
∴ a_ij = a_ij = 0, (∀ i, j)
Thus, given square matrix is null matrix.
∴ Option (B) is correct.

Question 15.
If A is square matrix such that A² = A, then (I + A)³ - 7A is equal to:
(A) A
(B) I - A
(C) I
(D) 3A
Answer:
We have
A² = A
∵ A³ = A²A = AA
= A² = A (∵ A² = A, AA = A²)
∴ A³ = A
∵ (I + A)³ - 7A = I³ + 3I²A + 3IA² + A³ - 7A
= I + 3A + 3A² + A³ - 7A
[∵ (I + A)³ = (I + A) (I + A) (I + A)]
= I + 3A + 3A + A - 7A
= I + 7A - 7A = I
Thus, option (C) is correct.