RBSE Solutions for Class 12 Maths Chapter 12 Linear Programming Ex 12.1

Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 12 Linear Programming Ex 12.1 Textbook Exercise Questions and Answers.

RBSE Class 12 Maths Solutions Chapter 12 Linear Programming Ex 12.1

Question 1.
Solve the following linear programming problems graphically:
Maximize Z = 3x + 4y Subject to the constraints :
x + y ≤ 4, x ≥ 0, y ≥ 0.
Answer:
Given that: Objective function Z = 3x + 4 y
and constraints x + y< 4, x ≥ 0, y ≥ 0.
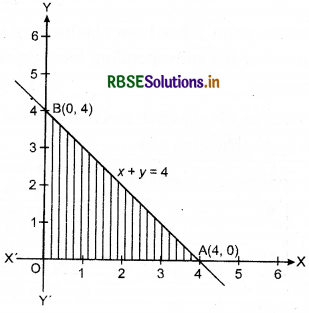
(i) Graph of x + y < 4: Line x + y = 4 passes through point A(4, 0) and B(0, 4).
Putting x = 0 and y = 0in x + y ≤ 4
0 ≤ 4, which is true.
∴ The origin lines in this region.

⇒ Region x + y ≤ 4 lies on line x + y = 4 and the origin below the line.

(ii) x ≥ 0 is the region and to the right of y-axis.
(iii) y ≥ 0 is the region and above x-axis.
The common shade region is ΔOAB.

Maximize : Z = 3x + 4y
At point 0(0, 0), Z = 3 × 0 + 4 × 0 = 0
At point A (4, 0), Z = 3 × 4 + 4 × 0 = 12
At point B( 0, 4), Z = 3 × 0 + 4 × 4 = 16
Hence, maximum value of Z is 16 at the point B(0,4).

 

Question 2.
Minimize, Z = - 3x + 4y subject to : x + 2y ≤ 8, 3x + 2y ≤ 12, x ≥ 0, y ≥ 0.
Answer:
Given that: Objective function :
Z = - 3x + 4y
and constraints %
x + 2y ≤ 8,
3x + 2y ≤ 12
x ≥ 0 and y ≥ 0
(i) Graph of x + 2y ≤ 8 : Line x + 2y = 8, passes through points A (8, 0) and B(0, 4).
Its graph is line AB.
Putting x = 0 and y = 0 in inequality x + 2y ≤ 8, 0 ≤ 8, which is true.
⇒ Region x + 2y < 8 lies on line x + 2y = 8 and the origin below the line.

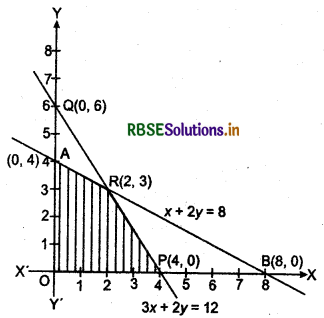
(ii) 3x + 2y ≤ 12 : Line 3x + 2y = 12 passes through point P(4, 0) and Q(0, 6). Its graph is PQ.
Putting x = 0, y = 0 in inequality 3x + 2y ≤ 12,0 ≤ 12, which is true.
Then, this region lies on line 3x + 2y = 12 and the origin below the line.

(iii) x ≥ 0 is the region on and to the right of y-axis.

(iv) y ≥ 0 is the region on and above x-axis.
The feasible region of this problem is OARP.
The line AB, x + 2y = 8 and PQ, 3x + 2y = 12 intersect at point R. Solving these equations
x = 2 and y = 3
∴ Point R is R(2,3).
Maximise, Z = - 3x + 4y
At point A(0,4), Z = -3 × 0 + 4 × 4 = 16
At point R(2,3), Z = -3 × 2 + 4 × 3 = - 6 +12 = 6
At point P(4,0), Z = - 3 × 4 + 4 × 0 = -12
Hence, minimum value of Z is - 12 at the point P(4, 0).

Question 3.
Maximize Z = 5x + 3y subject to
3x + 5y ≤ 15
5x + 2y ≤ 10
x ≥ 0, y ≥ 0
Answer:
Converting the given inequations into equations:
3x + 5y = 10
5x + 2y = 10
Region represented by 3x + 5y ≤ 15 :
The line 3x + 5y = 15 meets the coordinates axis at A(5,0) and B(0,3)
3x + 5y = 15

x	5	0
y	0	3

A(5,0) B(0,3)
Join point A and B to be obtain the line. Clearly, (0,0) satisfies the inequation 3x + 5y < 15. So, the region containing the origin represent the solution set of the inequation 3x + 5y ≤ 15.

Region represented by 5x + 2y < 10 : The line 5x + 2y = 0 meets the coordinates axis at C(2, 0) and D(0,5) respectively. 5x + 2y = 0

x	2	0
y	0	5

C(2,0), D(0,5) Join points C and D to obtain the line.
Clearly, (0,0) satisfies the inequation 5x + 2y ≤ 10. So, the region containing the origin represent the solution set of the inequation 5x + 2y ≤ 10.

Region represented by x ≥ 0, y ≥ 0 :
Since every point in the first quadrant satisfies these inequations. So, the first quadrant is the region represented by the inequation x ≥ 0 and y ≥ 0.
The shaded region OCEB represents the common region of the above inequations. This region is the feasible region of the given LPP.

The coordinates of the comer-points of the shaded feasible region are 0(0, 0), C(2, 0) E\(\left(\frac{20}{19}, \frac{45}{19}\right)\) and B(0, 3), where point E has been obtained by solving the equations of the corresponding intersecting lines simultaneously.

The value of the objective function at these points are given in the following table:


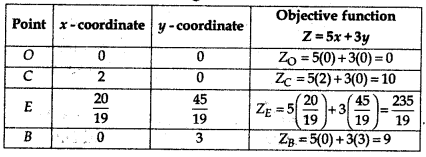
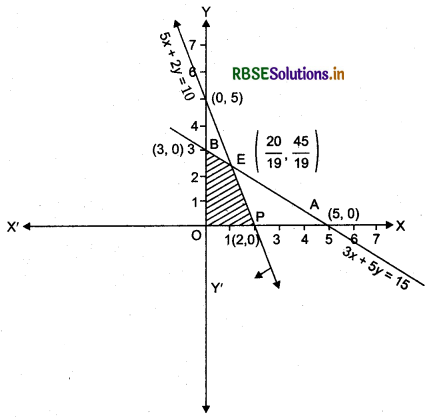
Clearly, Z is maximum at P\(\left(\frac{20}{19}, \frac{45}{19}\right)\). Hence, x = \(\frac{20}{19}\) y = \(\frac{45}{19}\) is the optimal solution of the given LPP and optimal value of Z is \(\frac{235}{19}\)

Question 4.
Minimize Z = 3x + 5 y such that
x + 3y ≥ 3
x + y ≥ 2
x, y ≥ 0
Answer:
Converting the given inequations into equations
x + 3y = 3
x + y = 2

Region represented by x + 3y ≥ 3:
The line x + 3y = 3 meets the coordinate axis at A(3,0) and B(0,1).
x + 3y = 3

x	3	0
y	0	1

A(3, 0); B(0,1)
Join the point A to B to obtain a line. Clearly (0, 0) does not satisfy the inequation x + 3y ≥ 3. So the region opposite to the origin, represents the solution set of the inequation.

Region represented by x + y ≥ 2:
The line x + y = 2 meets the coordinate axis at points C(2, 0) and D(0, 2)

Linear Programming
x + y = 2

x	2	0
y	0	2

C(2,0); D(0, 2)
Join the points C to D to obtain the line. Clearly (0,0) does not safitsy the inequation.: x + y ≥ 2.
So the region opposite to origin, represents the solution set of the inequation.

Region represented by x ≥ 0, y ≥ 0 :
Since every point in the first quadrant satisfies these inequations. So the first quadrant is the region represented by the equation x ≥ 0, y ≥ 0.
The point of intersection of lines x + 3y = 3 and x + y = 2 is E\(\left(\frac{3}{2}, \frac{1}{2}\right)\).

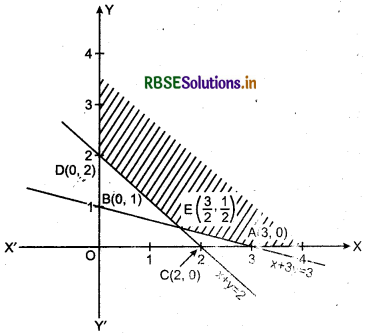
The shaded region AED is an open and common region of given inequations. This is the proper solution of the given linear programming problem.
The coordinates of the shaded region are A (3, 0), E\(\left(\frac{3}{2}, \frac{1}{2}\right)\) and D(0, 2)

The values of the objective function of these points are given in following table :

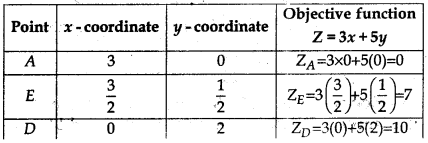
Clearly Z is minimum at x = \(\frac{3}{2}\) and y = \(\frac{1}{2}\)
Therefore x = \(\frac{3}{2}\) and y = \(\frac{1}{2}\) is the required solution of the L.P. problem and the minimum value of Z is 7.

Question 5.
Maximize Z = 3x + 2y subject to x + 2y ≤ 10, 3x + y ≤ 15, x, y ≥ 0.
Answer:
Given that:    objective function: Z = 3x + 2y and constraints: x + 2y ≤ 10, 3x + y ≤ 15, x, y ≥ 0.
(i) Graph of x + 2y < 10 : Line x + 2y = 10 passes through point A(10,0) and B(0,5).
So, graph of line x + 2y = 10 is AB.
Putting x = 0, y = 0 in inequality x + 2y ≤ 10 0 ≤ 10 which is true.
⇒ The points of region x + 2y ≤ 10 lies on the line AB

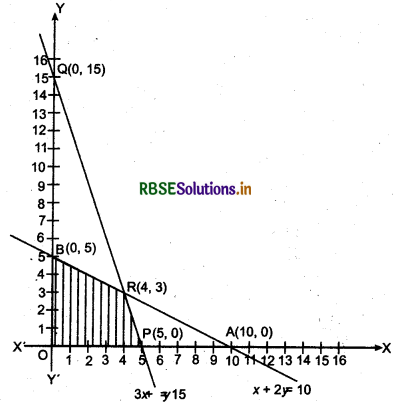
(ii) Graph of 3x + y ≤ 15 : Line 3x + y = 5 passes through points P(5,0) and Q(0,15).
So, graph of line 3x + y = 15 is PQ.
Putting x = 0, y = 0 in inequality 3x + y ≤ 15
0 ≤ 15 which is true.
⇒ The points of region 3x + y ≤ 15 lies on the line PQ and below the line PQ.

(iii) The points of region x ≥ 0 lies on y-axis and right side of y-axis.

(iv) The points of region y ≥ 0 lies on and above x-axes.
∴ The feasible region of .this problem is OBRP when -ever the intersection point of AB and PQ is R.
On solving equations AB : x + 2y = 10 and PQ: 3x + y = 15, we get point R(4, 3).

Now, objective function:
Z = 3x + 2y
at point P(5,0),
Z = 3 × 5 + 2 × 0 = 15 

at point R(4, 3)
Z = 3 × 4 + 2 × 3 = 12 + 6 = 18 

at point B(0, 5),
Z = 3 × 0 + 2 × 5 = 10
Hence, maximum value of Z at point R(4, 3) is 18.

Question 6.
Minimize Z = x + 2y subject to 2x + y ≥ 3, x + 2y ≥ 6, x, y ≥ 0.
Answer:
Given that:
objective function: Z = x + 2y and constraints :2x + y ≥ 3, x + 2y ≥ 6 and x, y ≥ 0.
(i)    Graph of 2x + y ≥ 3  Line 2x + y = 3, passes through point A(3/2, 0) and B(0, 3).
∴ Graph of line 2x + y = 3 is line AB.
⇒ Putting x = 0, y = 0 in inequality 2x + y ≥ 3 0 ≥ 3 which is not true
∴ The points of region 2x + y ≥ 3 lies on the line AB and above the line AB.

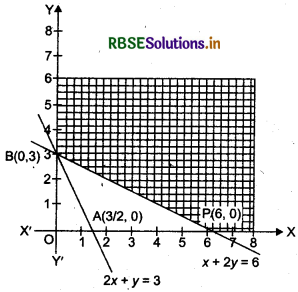
(ii) Graph of x + 2y ≥ 6 : Line x + 2y = 6 passes through points P(6,0) and B(0,3)
∴ Graph of line x + 2y = 6 is line PB.
⇒ Putting x = 0, y = 0 in inequality x + 2y ≥ 6 0 ≥ 6 which is not true
⇒ The points of region x + 2y > 6 lies on the line PB and above the line PB. 

(iii) The points of region x ≥ 0 lies on y-axes and right side of y-axis.

(iv) The points of region y ≥ 0 lies on x-axes and above x-axis.
The feasible region of this problem is YBPX.

Now, objective function :
Z = x + 2y
at point B(0, 3),
Z = 0 + 2 × 3 = 6

at point P(6, 0),
Z = 6 + 2 × 0 = 6
Also, this region is unbounded and any point of x + 2y ≥ 6 does not lie in feasible region.
Hence, Minimum value of Z is 6 which is true for each value of line PB.

Question 7.
Show that the minimum of Z occurs at more than two points.
Minimize and maximize Z = 5x + 10y subject to x + 2y ≤ 120, x + y ≥ 60, x - 2y ≥ 0, x, y ≥ 0.
Answer:
Given that:
Objective function: Z = 5x + 10y and
constraints : x + y ≥ 60, x + 2y ≤ 120, x - 2y ≥ 0 and x, y ≥ 0.
(i) Graph of x + 2y < 120 : Line x + 2y = 120, passes through points A(120, 0) and B(0, 60) Graph of line x + 2y = 120 is line AB. ⇒ Putting x = 0, y = 0 in inequality x + 2y ≤ 120 0 ≤ 120 which is true ∴ The points of region x + 2y ≤ 120 lies on the AB and below the line AB towards the origin.

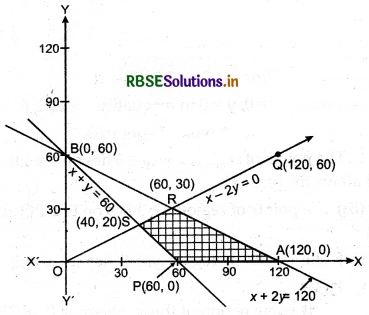
(ii) Graph of x + y > 60 : Line x + y = 60 passes through points P(60, 0) and B(0, 60).
∴ Graph of x + y = 60 is line PB.
⇒ Putting x = 0, y = 0 in inequality x + y ≥ 60 0 ≥ 60 which is not true.
The points of region x + y ≥ 60 lies on the line PB and above the line PB.

(iii) Graph of x - 2y > 0 : Line x - 2y = 0 passes through the origin 0(0, 0) and Q(120, 60)
∴ Graph of line x - 2y > 0 is line OQ.
⇒ Putting x = 1, y = 0 in inequality x - 2y ≥ 0 1 ≥ 0 which is true.
⇒ (1, 0) lies in this region. The points or region x - 2y ≥ 0 lies on the line OQ and below the line OQ towards (1,0)
(iv) The points of region x ≥ 0 lies on y-axis and right side of y-axis.
(v) The points of region y ≥ 0 lies on x-axis and above x-axis.
The feasible region on this problem is PSRA.
Intersection point of PB : x + y = 60 and OQ : x - 2y = 0 is S(40, 20).

Now, objective function Z = 5x + 10y
at point A (120,0),
Z = 5 × 120 + 10 × 0 = 600
at point S(40, 20),
Z = 5 × 40 + 10 × 20 = 200 + 200 = 400
at point P(60,0),
Z = 5 × 60 + 10 × 0 = 300
Hence, minimum value of Z at point P(60, 0) is 300 and maximum value of Z is 600 at all points of RA.

Question 8.
Minimize and maximize Z = x + 2y. subject to x + 2y ≥ 100, 2x - y ≤ 0,2x + y ≤ 200; x, y ≥ 0
(CBSE 2017)
Sol. Given that: objective function Z = x + 2y and
constraints : x + 2y ≥ 100, 2x - y ≤ 0,
2x + y ≤ 200 and x, y ≥ 0.
(i) Graph of x + 2y ≥ 100 : Line x + 2y = 100 passes through points A (100,0) and B(0,50).
∴ Graph of x + 2y = 100 is line AB.
⇒ Putting x = 0, y - 0 in x + 2y ≥ 100
C ≥ 100 which is not true.

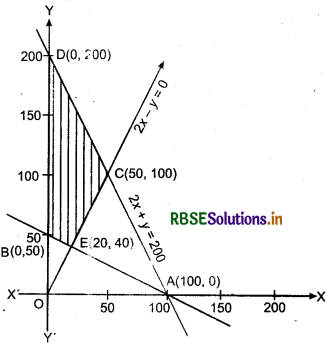
∴ The points of region x + 2y ≥ 100 lies on the line AB and above the line AB.

(ii) Graph of 2x - y ≤ 0 : Line 2x - y = 0, passes through points 0(0, 0) and C(50, 100).
Graph of 2x - y = 0 is line OC.
⇒ Putting x = 1, y = 0 in inequality 2x - y ≤ 0
2 ≤ 0 which is not true.
∴ The points of region 2x - y ≤ 0 lies on the line OC and above the line OC.

(iii) Graph of 2x + y < 200: Line 2x + y = 200 passes through points A (100, 0) and D(0, 200)
∴ Graph of 2x + y = 200 is line AD.
⇒ Putting x = 0, y = 0 in inequality 2x + y ≤ 200, 0 ≤ 200 which is true.
⇒ The points of region 2x + y ≤ 200 lies on the line AD and below the line AD.

(iv) The points of region x ≥ 0 lies on y-axis and right side of y-axis

(v) The points of region y ≥ 0 lies on x-axis and above x-axis.
∴ The feasible region of this problem is DBEC
Intersection point of line AB : x + 2y = 100 and line OC: 2x - y = 0 is L(20,40).
Now, objective function : Z = x + 2y
at point B(0,50), Z = 0 + 2 × 50 = 100
at point E(20, 40), Z = 20 + 2 × 40 = 20 + 80 = 100
at point C(50,100), Z = 50 + 2 × 100 = 50 + 200 = 250
at point D(0, 200), Z = 0 + 2 × 200 = 400
Hence, Maximum value of Z is 400 which is at point D(0,200) and minimum value of Z is 100 which is at all points of BE.

Question 9.
Maximize Z = - x + 2y, subject to the constraints: '
x ≥ 3, x + y ≥ 5, x + 2y ≥ 6, y≥ 0.
Answer:
Given that: objective function Z = - x + 2y and constraints :x ≥ 3, x + y ≥ 5, x + 2y ≥ 6 and y ≥ 0.
(i) Graph of x + y ≥ 5: Line x + y = 5 passes through the points A(5, 0) and B(0,5).
∴ Graph of x + y = 5 is line AB.
⇒ Putting x = 0, y = 0 is inequality x + y ≥ 5 0 ≥ 5, which is not true.
∴ The points of region x + y ≥ 5 lies on the line AB and above the line AB.

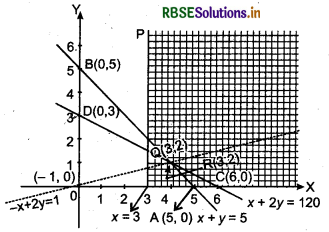
(ii) Graph of x + 2y ≥ 6 : Line x + 2y = 6, passes through the points C(6, 0) and D(0,3).
Graph of line x + 2y = 6 is line CD.
⇒ Putting x = 0, y = 0 in inequality x + 2y ≥ 6.
0 ≥ 6 which is not true.
The points of region x + 2y ≥ 6 lies on the line CD and above the line CD.

(iii) The points of region x ≥ 3 lies on line PQ : x = 3 and right side of it.

(iv) The points of region y ≥ 0 lies on x-axis and above x-axis.
∴ The feasible region of this problem is PQRCX.
Intersection point of line PQ : x = 3 and line AB : x + y = 5 is Q(3, 2)
Intersection point of line CD : x + 2y = 6 and line AB : x + y = 5 is R (4,1) :

Now, objective function:
Z = -x + 2y
At point Q(3,2),
Z = -3 + 2 × 2 = -3 + 4 = 1
At point R(4,1),
Z = -4 + 2 × 1 = -4 + 2 = -2
At point C(6,0),
Z = -6 + 2 × 0 = -6
Maximum value of Z is 1 but feasible region is unbounded if we consider - x + 2y ≥ 1 then many points are common in - x + 2y ≥ 1 and feasible region.
Hence, Z has no maximum value.

Question 10.
Maximize Z = x + y, subject to x - y ≤ - 1, -x + y ≤ 0, x,y ≥ 0.
Sol. Given that: objective function Z = x + y and
constraints : x - y ≤ -1, - x + y ≤ 0 and x, y ≥ 0.

(i) Graph of x - y ≤ - 1 : Line x - y = - 1 passes through points A(-1,0) and B(0,1) whose graph is line AB.
Putting x = 0, y = 0 in inequality x - y ≤ -1 0 ≤ -1 which is not true.
∴ The points of region x - y ≤ -1 lies on the line AB and above the line AB.

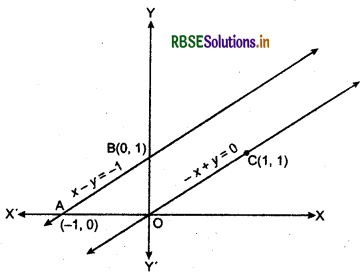
(ii) Graph of - x + y ≤ 0 : Line - x + y = 0, passes through the points 0(0,0) and (1, 1) putting x = 1, y = 0 in inequality - x + y ≤ 0
-1 ≤ 0 which is true.
⇒ The points of region - x + y ≤ 0 lies on the line OC and below the line OC towards (1,0)

(iii) The points of region x ≥ 0 lies on y-axis and right side of y-axis.

(iv) The points of region y ≥ 0 lies on x-axis and above x-axis.
Thus, there is no any feasible region of this problem.
Hence, Z has no maximum value.