RBSE Solutions for Class 12 Maths Chapter 11 Three Dimensional Geometry Ex 11.1
Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 11 Three Dimensional Geometry Ex 11.1 Textbook Exercise Questions and Answers.
Rajasthan Board RBSE Solutions for Class 12 Maths in Hindi Medium & English Medium are part of RBSE Solutions for Class 12. Students can also read RBSE Class 12 Maths Important Questions for exam preparation. Students can also go through RBSE Class 12 Maths Notes to understand and remember the concepts easily.
RBSE Class 12 Maths Solutions Chapter 11 Three Dimensional Geometry Ex 11.1
Question 1.
If a line makes angles 90°, 135°, 45° with the x, y and z-axis respectively, find its direction cosines.
Answer:
A line makes angles 90°, 135°, 45° with the x, y and z-axis respectively.
∴ cos α = cos 90° = 0,
cos β = cos 135° = - \(\frac{1}{\sqrt{2}}\),
cos γ = cos 45° = \(\frac{1}{\sqrt{2}}\)
∴ Direction cosines are 0, - \(\frac{1}{\sqrt{2}}\), \(\frac{1}{\sqrt{2}}\).
Question 2.
Find the direction cosines of a line which makes equal angles with the coordinate axis.
Answer:
Let α be the angle made by the line with the coordinate axis.
∴ Direction cosines of the line are cos α, cos α, cos α
But l2 + m2 + n2 = 1
cos2 α + cos2 α + cos2 α = 1
3 cos2α = 1
cos2 α = \(\frac{1}{3}\)
∴ cos α = ± \(\frac{1}{\sqrt{3}}\)
Hence, the direction cosines of the line are:
±\(\frac{1}{\sqrt{3}}\), ±\(\frac{1}{\sqrt{3}}\), ±\(\frac{1}{\sqrt{3}}\).

Question 3.
If a line has the direction ratios - 18, 12, - 4 then what are its direction cosines?
Answer:
Let a, b, c be the direction ratios, then direction cosines of the line are as follows
(where a = - 18, b = 12, c = - 4)

Question 4.
Show that the points (2, 3, 4), (- 1, - 2, 1) (5, 8, 7) are collinear.
Answer:
Let A(2, 3, 4), B(- 1, - 2, 1) and C(5, 8, 7) be the given points.

Hence A, B, C are collinear.
Hence proved.

Question 5.
Find the direction cosines of the sides of the triangle whose vertices are (3, 5, - 4), (- 1, 1, 2) and (- 5, - 5, - 2).
Answer:
Let A(3, 5, - 4), B(- 1, 1, 2) and C(- 5, - 5, - 2) be the vertices of ∆ABC.
(i) Direction ratios of AB:
x2 - x1, y2 - y1, z2 - z1
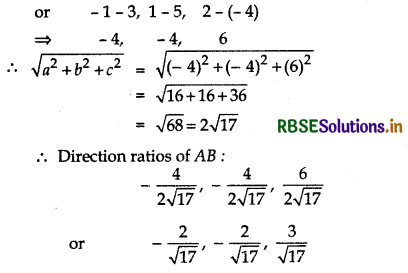
(ii) Direction ratios of BC:
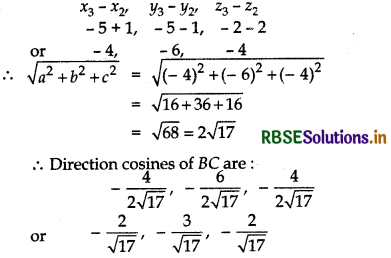
(iii) Direction ratios of CA:
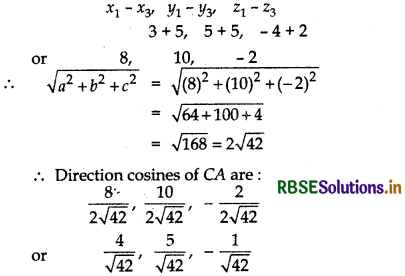

- RBSE Class 12 Maths Notes Chapter 13 Probability
- RBSE Class 12 Maths Notes Chapter 12 Linear Programming
- RBSE Class 12 Maths Notes Chapter 11 Three Dimensional Geometry
- RBSE Class 12 Maths Notes Chapter 10 Vector Algebra
- RBSE Class 12 Maths Notes Chapter 9 Differential Equations
- RBSE Class 12 Maths Notes Chapter 8 Application of Integrals
- RBSE Class 12 Maths Notes Chapter 7 Integrals
- RBSE Class 12 Maths Notes Chapter 6 Application of Derivatives
- RBSE Class 12 Maths Notes Chapter 5 Continuity and Differentiability
- RBSE Class 12 Maths Notes Chapter 4 Determinants
- RBSE Class 12 Maths Notes Chapter 3 Matrices