RBSE Solutions for Class 12 Maths Chapter 10 Vector Algebra Miscellaneous Exercise

Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 10 Vector Algebra Miscellaneous Exercise Textbook Exercise Questions and Answers.

RBSE Class 12 Maths Solutions Chapter 10 Vector Algebra Miscellaneous Exercise

Question 1.
Write down a unit vector in XY-plane, making an angle of 30° with the positive direction of X-axis.
Answer:
Let \(\overrightarrow{O P}\) is a unit vector in XY-plane which makes an angle of 30° with the positive direction.
Thus ∠XOP = 30°
PN is perpendicular on x-axis from point P of vector \(\overrightarrow{O P}\).

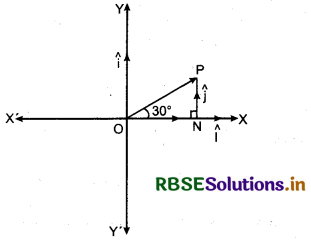
In right angled triangle ∆ONP,

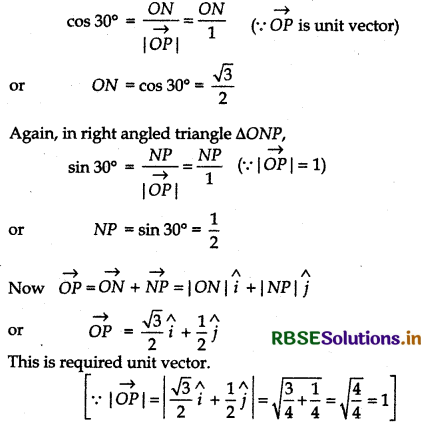
Question 2.
Find the scalar components and magnitude of the vector joining the points P(x₁, y₁, z₁) and Q(x₂, y₂, z₂).
Answer:
Let O be origin, then with respect to O,
Position vector of point

Question 3.
A girl walks 4 km towards west, then she walks 3 km in a direction 30° east of north and stops. Determine the girl’s displacement from her initial point of departure.
Answer:
Let the girl walks 4 km towards west OP from point O as shown in figure. Then vector \(\overrightarrow{O P}\) is represented by - 4î. Now, the girl walks 3 km in a direction 30° east of north PQ then she reach at point Q. PQ makes an angle 30° east of north and makes an angle 60° with OX’ axis, as shown in figure.

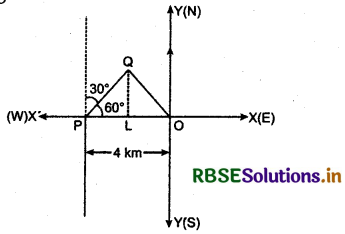
Question 4.
If \(\vec{a}=\vec{b}+\vec{c}\), then it is true |\(\vec{a}\)| = |\(\vec{b}\)| + |\(\vec{c}\)|?
Justify your answer.
Answer:

where θ is the angle betwen \(\vec{b}\) and \(\vec{c}\)

(i) If θ = 0, cos 0° = 1

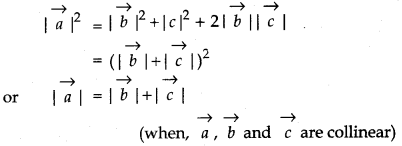
(ii) if θ ≠0, cos 0°≠ 1

Question 5.
Find the value of x for which x for which x(î + ĵ + k̂) is a unit vector.
Answer:
Given, x(î + ĵ + k̂) is a unit vector
∴ |xî + xĵ + xk̂| = 1
or √3x² = 1
or x√3 = ± 1
or x = ±\(\frac{1}{\sqrt{3}}\).

Question 6.
Find a vector of magnitude 5 units, and parallel to the resultant of the vectors \(\vec{a}\) = 2î + 3ĵ - k̂ and b = î - 2ĵ + k̂
Answer:
Let resultant of the vectors \(\vec{a}\) and \(\vec{b}\) is \(\vec{c}\).
Then \(\vec{c}\) = \(\vec{a}\) + \(\vec{b}\)

Question 7.
If \(\vec{a}\) = î + ĵ + k̂, \(\vec{b}\) = 2î - ĵ + 3k̂ and \(\vec{c}\) = î - 2ĵ + k̂, find a unit vector parallel to the vector 2\(\vec{a}\) - \(\vec{b}\) + 3\(\vec{c}\).
Answer:
Given,

Question 8.
Show that the points A(1, - 2, - 8), B(5, 0, - 2) and C(11, 3, 7) are collinear, and find the ratio in which B divides AC.
Answer:
Let O be origin, then with respect to O

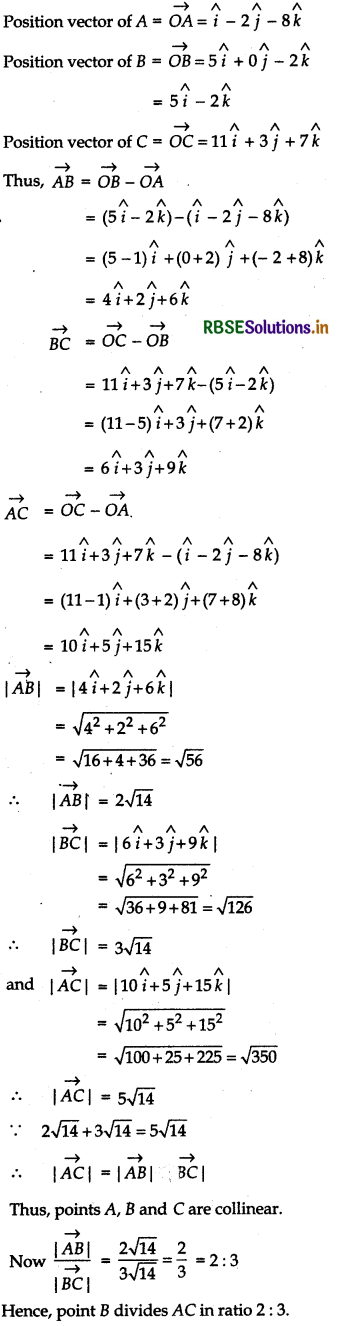
Question 9.
FInd the position vector of a point R which divides the line joining two points P and Q whose position vectors are P\((2 \vec{a}+\vec{b})\) and Q\((\vec{a}-3 \vec{b})\) externally in the ratio 1:2. Also, show that P is the mid point of the line segment RQ.
Answer:
Given position vectors of points P and Q are 2 \(\vec{a}+\vec{b}\) and \(\vec{a}-3 \vec{b}\) respectively.

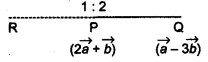
Point R, divides PQ externally in the ratio 1:2
∴ Position vector of R

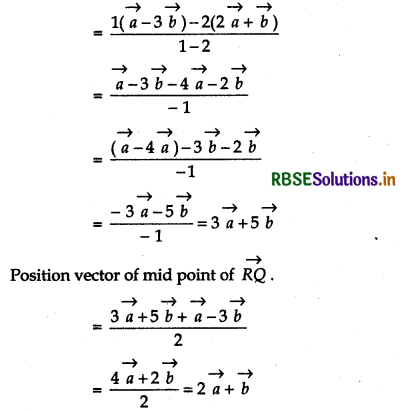
which is the position vector of P.
Thus, P is the mid point of the line segment RQ.

Question 10.
The two adjacent sides of a parallelogram are 2î - 4ĵ + 5k̂ and î - 2ĵ - 3k̂. Find the unit vector parallel to its diagonal. Also, find its area.
Answer:
Let two adjacent sides of the parallelogram are \(\vec{a}\) and \(\vec{b}\).

= [- 4 × (- 3) - (- 2) × 5]î - [2 × (- 3) - 1 × 5]ĵ + [2 × (- 2) - 1 × (- 2)]k̂
= (12 + 10)î - (- 6 - 5)ĵ + (- 4 + 4)k̂
= 22î + 11ĵ
Area of parallelogram = |\(\vec{a} \times \vec{b}\)|
= |22î + 11ĵ| = \(\sqrt{22^2+11^2}\)
= 11\(\sqrt{4+1}\) = 11√5
= 11√5 unit.

Question 11.
Show that the direction cosines of a vector equally inclined to the axes OX, OY and OZ are ± \(\left(\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\right)\).
Answer:
Let vector \(\overrightarrow{O P}\) is equally inclined to the axes OX, OY and OZ. Let this angle be α.
Then, direction cosines of \(\overrightarrow{O P}\) are
cos α, cos α, cos α.
But cos² α + cos² α + cos² α = 1. (∵ l² + m² + n² = 1)
or 3 cos² α = 1 ⇒ cos² α = \(\frac{1}{3}\) ⇒ cos α = \(\frac{1}{\sqrt{3}}\)
Hence, direction cosines of vector \(\overrightarrow{O P}\) are
\(\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\).

Question 12.
Let \(\vec{a}\) = î + 4ĵ + 2k̂, \(\vec{b}\) = 3î - 2ĵ + 7k̂ and \(\vec{c}\) = 2î - ĵ + 4k̂ Find a vector \(\vec{d}\) which is perpendicular to both \(\vec{a}\) and \(\vec{b}\), and \(\vec{c} \cdot \vec{d}\) = 15.
Answer:
Given, \(\vec{a}\) = î + 4ĵ + 2k̂,
\(\vec{b}\) = 3î - 2ĵ + 7k̂
\(\vec{c}\) = 2î - ĵ + 4k̂
Now, perpendicular vector on \(\vec{a}\) and \(\vec{b}\) = \(\vec{a} \times \vec{b}\)

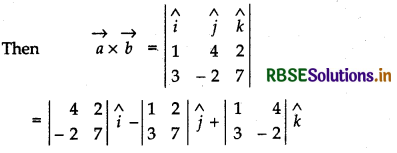
= [4 × 7 - (- 2) × 2]î - (1 × 7 - 3 × 2)ĵ +[1 × (- 2) - 3 × 4] k̂
= (28 + 4)î - (7 - 6)ĵ + (- 2 - 12)k̂
= 32î - ĵ - 14k̂

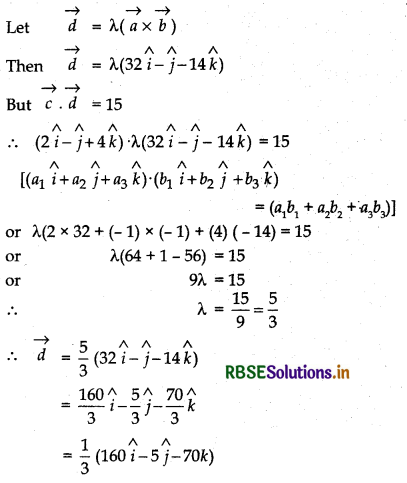
Question 13.
The scalar product of the vector î + ĵ + k̂ with a unit vector along the sum of vectors 2î + 4ĵ - 5k̂ and λî + 2ĵ + 3k̂ is equal to one. Find the value of A.
Answer:

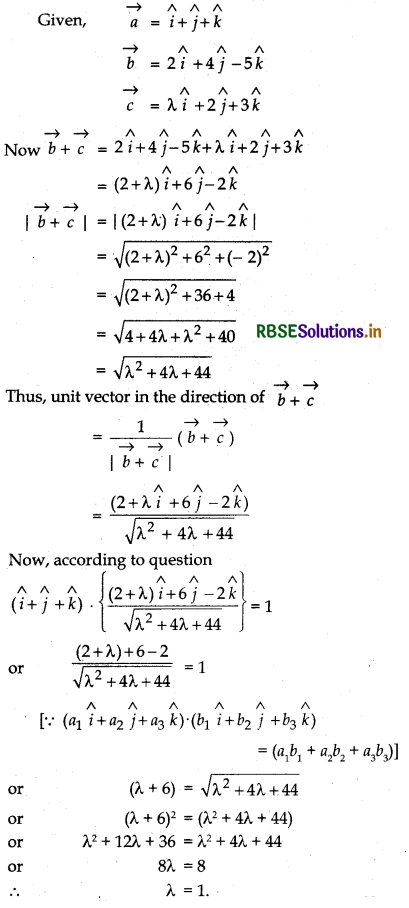
Question 14.
If \(\vec{a}, \vec{b}, \vec{c}\) are mutually perpendicular vectors of equal magnitudes, show that the vector \(\vec{a}+\vec{b}+\vec{c}\) is equally inclined to \(\vec{a}, \vec{b}\) and \(\vec{c}\).
Answer:

Question 15.
Prove that (\(\vec{a}+\vec{b}) \cdot(\vec{a}+\vec{b}\)) = \(|\vec{a}|^2+|\vec{b}|^2\), if and only if \(\vec{a}, \vec{b}\) are perpendicular given \(\vec{a}\) ≠ 0, \(\vec{b}\) ≠ 0.
Answer:

Question 16.
If θ is the angle between two vector \(\vec{a}\) and \(\vec{b}\), then \(\vec{a} \cdot \vec{b}\), then \(\vec{a} \cdot \vec{b}\) ≥ 0 only when
(A) 0 < θ < \(\frac{\pi}{2}\)
(B) 0 ≤ θ ≤ \(\frac{\pi}{2}\)
(C) 0 < θ < π
(D) 0 < θ < π
Answer:
We know that

Hence, option (B) is correct.

Question 17.
Let \(\vec{a}\) and \(\vec{b}\) be two unit vectors and θ is the angle between them. Then \(\vec{a}+\vec{b}\) is a unit vector if
(A) θ = \(\frac{\pi}{4}\)
(B) θ = \(\frac{\pi}{3}\)
(C) θ = \(\frac{\pi}{2}\)
(D) θ = \(\frac{2 \pi}{3}\)
Answer:
Given, \(\vec{a}\) and \(\vec{b}\) be two unit vectors and θ is the angle between them.

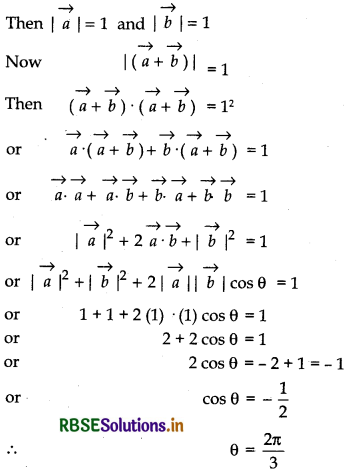
Hence, option (D) is correct.

Question 18.
The value of
î . (ĵ × k̂) + ĵ . (î × k̂) + k̂ . (î × ĵ) is:
(A) 0
(B) - 1
(C) 1
(D) 3
Answer:
Vectors î, ĵ and k̂ are mutually perpendicular and also unit vectors.
Now î . (ĵ × k̂) + ĵ . (î × k̂) + k̂ . (î × ĵ) = î . î + ĵ . (- ĵ) + k̂ . k̂ = 1 - 1 + 1
Hence. option (C) is correct.

Question 19.
If θ is the angle between ant two vectors \(\vec{a}\) and \(\vec{b}\), then |\(\vec{a} \cdot \vec{b}\)| = |\(\vec{a} \times \vec{b}\)| when θ is equal to:
(A) 0
(B) \(\frac{\pi}{4}\)
(C) \(\frac{\pi}{2}\)
(D) π
Answer:
Given angle between \(\vec{a}\) and \(\vec{b}\) is θ, then