RBSE Solutions for Class 12 Maths Chapter 10 Vector Algebra Ex 10.2

Rajasthan Board RBSE Solutions for Class 12 Maths Chapter 10 Vector Algebra Ex 10.2 Textbook Exercise Questions and Answers.

RBSE Class 12 Maths Solutions Chapter 10 Vector Algebra Ex 10.2

Question 1.
Compute the magnitude of the following vectors:
\(\vec{a}\) = î + ĵ + k̂
\(\vec{b}\) = 2î - 7ĵ - 3k̂
\(\vec{c}\) = \(\frac{1}{\sqrt{3}}î + \frac{1}{\sqrt{3}}ĵ - \frac{1}{\sqrt{3}}k̂\)
Answer:
(i) Magnitude of vector \(\vec{a}\) = |\(\vec{a}\)|

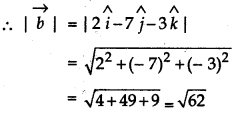
(ii) Magnitude of vector \(\vec{b}\) = |\(\vec{b}\)|

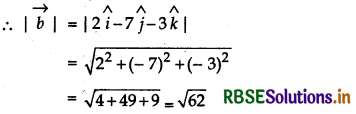
(ii) Magnitude of vector \(\vec{c}\) = |\(\vec{c}\)|

Hence, magnitude of given vectors are √3, √62 and 1 respectively.

Question 2.
Write two different vectors having same magnitude.
Answer:
Let vectors are

Thus, vectors \(\vec{a}\) and \(\vec{b}\) are two vectors having same magnitude. Numbers of such vectors are infinite.

Question 3.
Write two different vectors having same direction.
Answer:

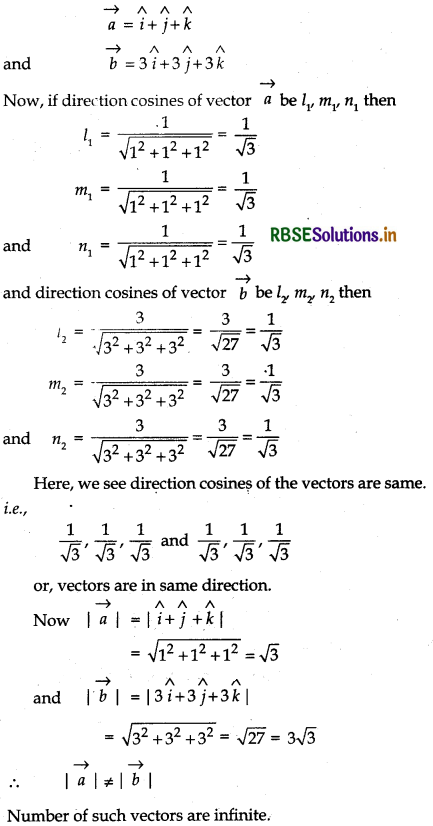
Question 4.
Find the values of x and y so that the vectors 2î + 3ĵ and xî + yĵ are equal.
Answer:
We know that two vectors are equal if their components are equal.
Let \(\vec{a}\) = 2î + 3ĵ and \(\vec{b}\) = xî + yĵ
Now \(\vec{a}\) = \(\vec{b}\) ⇒ 2î + 3ĵ = xî + yĵ
Then 2 = x and 3 = y
∴ x = 2 and y = 3.

Question 5.
Find the scalar and vector components of the vector with initial point (2, 1) and terminal point (- 5, 7).
Answer:
Let initial point and terminal point of the vector are A and B.
Then, co-ordinate of A (2, 1)
and, co-ordinate of B = (- 5, 7)
Now, from formula
\(\overrightarrow{A B}\) = (x₂ - x₁)î + (y₂ - y₁)ĵ + (z₂ - z₁)k̂
\(\overrightarrow{A B}\) = (- 5 - 2)î + (7 - 1)ĵ
= - 7î + 6ĵ
∴ Scalar components of \(\overrightarrow{A B}\) are - 7 and 6.
and vector components of \(\overrightarrow{A B}\) are - 7î and 6ĵ.

Question 6.
Find the sum of the vectors
\(\vec{a}\) = î - 2ĵ + k̂, \(\vec{b}\) = - 2î + 4ĵ + 5k̂ and \(\vec{c}\) = î - 6ĵ - 7k̂
Answer:
Sum of vectors \(\vec{a}, \vec{b}\) and \(\vec{c}\)
= \(\vec{a}+\vec{b}+\vec{c}\)
∴ \(\vec{a}+\vec{b}+\vec{c}\)
= (î - 2ĵ + k̂) + (- 2î + 4ĵ + 5k̂) + (î - 6ĵ - 7k̂)
= (1 - 2 + 1)î + (- 2 + 4 - 6)ĵ + (1 + 5 - 7)k̂
= - 4ĵ - k̂ is the required sum.

Question 7.
Find the unit vector in the direction of the vector \(\vec{a}\) = î + ĵ + 2k̂.
Answer:
Unit vector in the direction of vector \(\vec{a}\)

which is unit vector in the direction of the vector \(\vec{a}\).

Question 8.
Find the unit vector in the direction of the vector \(\overrightarrow{P Q}\), where P and Q are the points (1, 2, 3) and (4, 5, 6) respectively.
Answer:
Vector joining the points P and Q = \(\overrightarrow{P Q}\)
∴ \(\overrightarrow{P Q}\) = (x₂ - x₁)î + (y₂ - y₁)ĵ + (z₂ - z₁)k̂
= (4 - 1)î + (5 - 2)ĵ + (6 - 3)k̂
(∵ x₁ = 1, y₁ = 2, z₁ = 3 and x₂ = 4, y₂ = 5, z₂ = 6)
= 3î + 3ĵ + 3k̂

Question 9.
For given vectors \(\vec{a}\) = 2î - ĵ + 2k̂ and \(\vec{b}\) = - î + ĵ - k̂, find the unit vector in the direction of the vector \(\vec{a}\)+ \(\vec{b}\)

Answer:

which is the required unit vector.

Question 10.
Find a vector in the direction of vector 5î - ĵ + 2k̂ which has magnitude 8 units.
Answer:

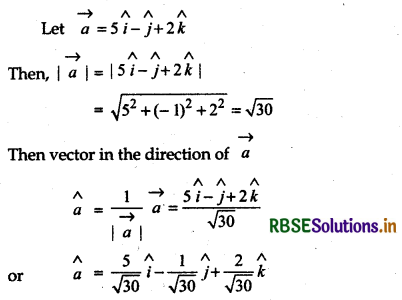
Now, the vector in the direction of \(\vec{a}\) and magnitude 8 units = 8\(\vec{a}\)

Question 11.
Show that the vectors 2î - 3ĵ + 4k̂ and - 4î + 6ĵ - 8k̂ are collinear.
Answer:
Let \(\vec{a}\) = 2î - 3ĵ + 4k̂
and \(\vec{b}\) = 4î + 6ĵ - 8k̂
= - 2(2î - 3ĵ + 4k̂)
∴ \(\vec{b}\) = - 2\(\vec{a}\) or \(\vec{a}\) = -\(\frac{1}{2}\vec{b}\)
Vectors \(\vec{a}\) and \(\vec{b}\) have the same direction, therefore they are collinear.

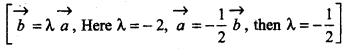
Hence Proved.

Question 12.
Find the direction cosines of the vector î + 2ĵ + 3k̂.
Answer:
Let vector \(\vec{r}\) = î + 2ĵ + 3k̂
If a, b, c are direction ratios of vector \(\vec{r}\) and l, m, and n are direction cosines, then,
a = 1, b = 2, c = 3

Hence, direction cosines of the given vector are \(\frac{\pm 1}{\sqrt{14}}, \frac{\pm 2}{\sqrt{14}}\) and \(\frac{\pm 3}{\sqrt{14}}\).

Question 13.
Find the direction cosines of the vector joining the points A(1, 2, -3) and B(-1,-2, 1), directed from A to B.
Answer:
We know that the direction cosines of the vector joining the points A(x₁, y₁, z₁) and B(x₂, y₂, z₂) directed from A to B = \(\overrightarrow{A B}\)
∴ \(\overrightarrow{A B}\) = (x₂ - x₁)î + (y₂ - y₁)ĵ + (z₂ - z₁)k̂
Given A(1, 2, -3) and B(- 1, - 2, 1)
∴ x₁ = 1, y₁ = 2, z₁ = - 3 .
and x₂ = - 1 , y₂ = - 2, z₂ = 1
∴ Vector directed from A to B = \(\overrightarrow{A B}\)
∴ \(\overrightarrow{A B}\) = (- 1 - 1)î + (- 2 - 2)ĵ + {1 - (- 3)}k̂
or \(\overrightarrow{A B}\) = - 2î - 4ĵ + 4k̂ ............. (i)
If direction ratios of \(\overrightarrow{A B}\) are a, b and c, then
\(\overrightarrow{A B}\) = aî + bĵ + ck̂
∴ From equations (1) and (2) a = - 2, b = - 4, c = 4
If direction cosines of \(\overrightarrow{A B}\) are l, m and n, then

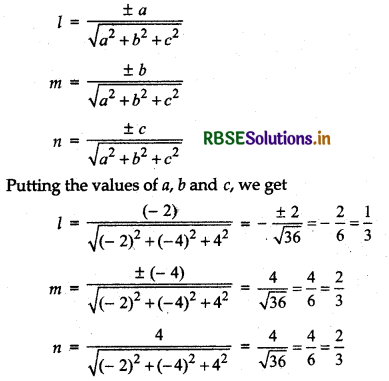
Hence the required direction cosines are \(\frac{-1}{3}, \frac{-2}{3}\) and \(\frac{2}{3}\).

Question 14.
Show that the vector î + ĵ + k̂ is equally inclined to the axes OX, OY and OZ.
Answer:
Let \(\vec{r}\) = î + ĵ + k̂
If direction ratios of vector r are a, b and c, then and
\(\vec{r}\) = aî + bĵ + ck̂
∴ a = 1, b = 1, c = 1
If direction cosines of vector \(\vec{r}\) are l, m and n, then

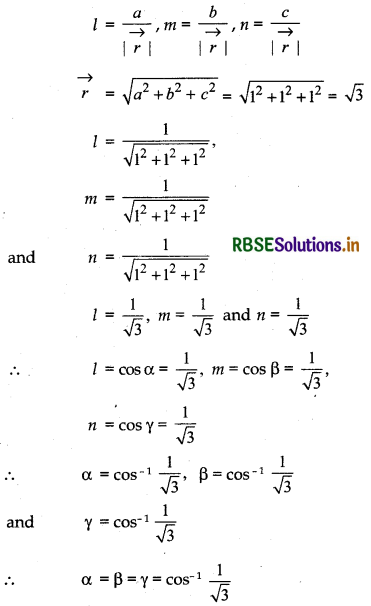
Hence, the given vector makes equal angle to the axes i.e., the given vector is equally inclined to the axes.

Question 15.
Find the position vector of a point R which divides the line joining two points P and Q whose position vectors are (î + 2ĵ - k̂) and (- î + ĵ + k̂) respectively, in the ratio 2 : 1
(i) internally
(ii) externally
Answer:
Let origin is O, then position vectors of points P, Q and R are \(\overrightarrow{O P}\), \(\overrightarrow{O Q}\) and \(\overrightarrow{O R}\).
Given, \(\overrightarrow{O P}\) = î + 2ĵ - k̂
and \(\overrightarrow{O Q}\) = - î + ĵ + k̂

(i) When point R divides the line joining the points P and Q in the ratio 2 : 1 internally, then

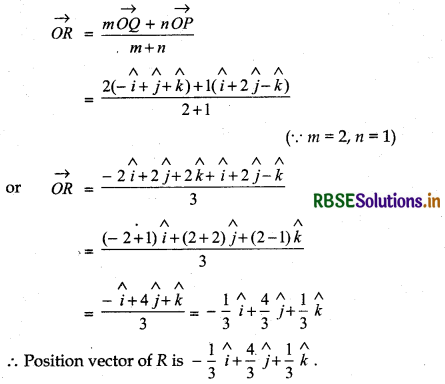
(ii) When point R divides the line joining the points P and Q in the ratio 2 :1 externally, then

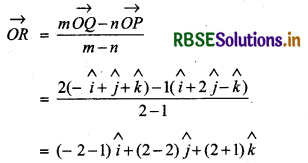
or \(\overrightarrow{O R}\) = - 3î + 3k̂
∴ Position vector of R is - 3î + 3k̂.

Question 16.
Find the position vector of the mid -point of the vector joining the points P(2, 3,4) and Q(4, 1, - 2).
Answer:
Let origin is o, then position vector of P and Q with respect to O are OP and OQ.
Now \(\overrightarrow{O P}\) = 2î + 3ĵ + 4k̂ and \(\overrightarrow{O P}\) = 4î + ĵ - 2k̂
Let R is the mid point of \(\overrightarrow{P Q}\), then

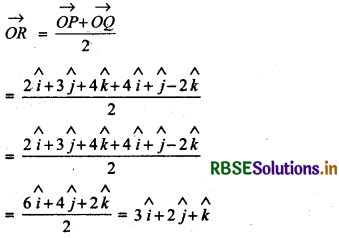
Hence, the required mid-points is 3î + 2ĵ + k̂.

Question 17.
Show that the points A, B and C, with position vectors,
\(\vec{a}\) = 3î - 4ĵ - 4k̂, \(\vec{b}\) = 2î - ĵ + k̂ and \(\vec{c}\) = î - 3ĵ - 5k̂
respectively form the vertices of a right angled triangle.
Answer:
Let O is origin, then


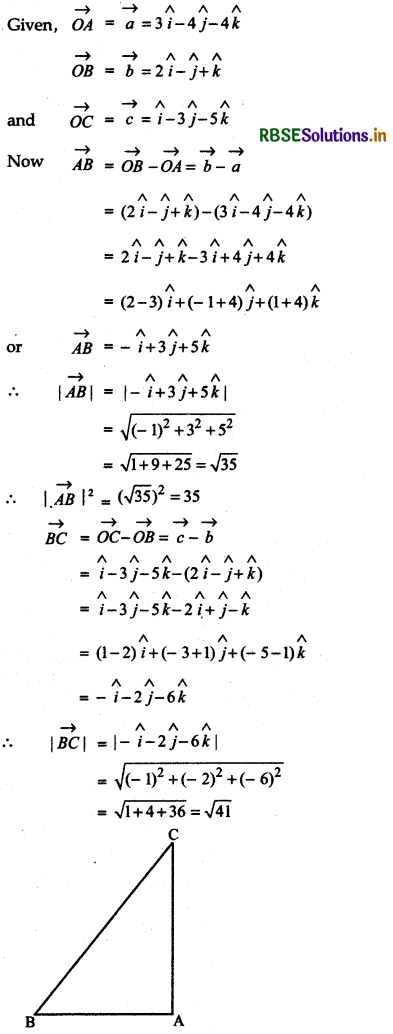
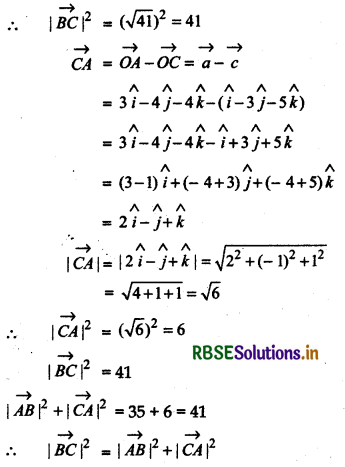
Hence, ∆ABC is right angled triangle i.e., points A, B and C form the vertices of a right angled triangle.
Hence Proved.

Question 18.
In triangle ABC (given figure), which of the following is not true.

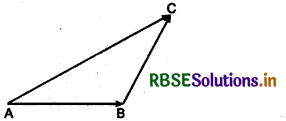
(A) \(\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{C A}=\overrightarrow{0}\)
(B) \(\overrightarrow{A B}+\overrightarrow{B C}-\overrightarrow{A C}=\overrightarrow{0}\)
(C) \(\overrightarrow{A B}+\overrightarrow{B C}-\overrightarrow{A C}=\overrightarrow{0}\)
(D) \(\overrightarrow{A B}-\overrightarrow{C B}-\overrightarrow{C A}=\overrightarrow{0}\)
Answer:
According to triangle law of addition of vectors,

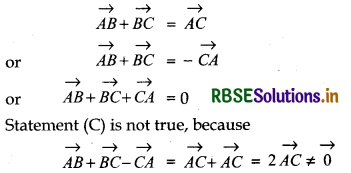
Hence, correct option is (C)

Question 19.
If \(\vec{a}\) and \(\vec{b}\) are two collinear vectors, then which of the following are incorrect:
(A) \(\vec{b}\) = λ\(\vec{a}\), for some scalarA
(B) \(\vec{a}\) = ±\(\vec{b}\)
(C) the respective components of \(\vec{a}\) and \(\vec{b}\) are not proportional.
(D) both the vectors a and b have same direction but different magnitudes.
Answer:
Given statements (A), (B) and (C) are correct.
Again, collinear vectors are parallel but it is not necessary direction is same or magnitude is same.
Thus, statement (D) is not correct
Hence, correct option is (D).