RBSE Solutions for Class 12 Chemistry Chapter 2 Solutions

Rajasthan Board RBSE Solutions for Class 12 Chemistry Chapter 2 Solutions Textbook Exercise Questions and Answers.

RBSE Class 12 Chemistry Solutions Chapter 2 Solutions

RBSE Class 12 Chemistry Solutions InText Questions and Answers

Question 2.1. 
Calculate the mass percentage of benzene (C₆H₆) and carbon tetrachloride (CCl₄) if 22 g of benzene is dissolved in 122 g of carbon tetrachloride. 
Answer:
Total mass of solution = Mass of benzene + Mass of CCl₄ 
= 22 g + 122 g = 144 g 

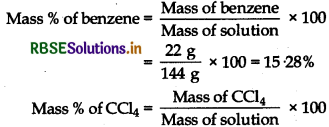
= \(\frac{122 \mathrm{~g}}{144 \mathrm{~g}} \times 100\)
= 84.72%
Alternatively, mass % of CCl₄
= 100 - Mass % of benzene
= 100 - 15 .28 = 84.72%

Question 2.2. 
Calculate the mole fraction of benzene in solution containing 30% by mass in carbon tetrachloride. 
Answer:
Let mass of solution = 100 g 
Thus mass of benzene = 30 g 
Mass of CCl₄ = 100 - 30 = 70 g 

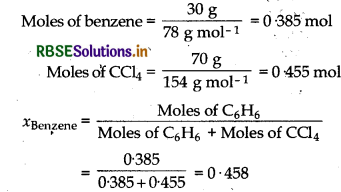
xCCl₄ = 1 - XBenzene 
= 1 - 0.458. = 0.542. 

 

Question 2.3. 
Calculate the molarity of each of the following solutions: 
(a) 30 g of CO(NO₃)₂.6H₂O in 4.3 L of solution 
(b) 30 mL of 0.5 M H₂SO₄ diluted to 500 mL. 
Answer:
(a) Molar mass of CO(NO₃)₂.6H₂O 
= 58.7 + 2(14 + 48) + 6 x 18 g mol^-1 
= 58.7 + 124 + 108 g mo^l-1 
= 290.7 g mol^-1 

Moles of CO(NO₃)₂.6H₂O
\(\frac{30 \mathrm{~g}}{290 \cdot 7 \mathrm{~g} \mathrm{~mol}^{-1}}\) = 0.103
Volume of Solution = 4.3 L

MV₁ =M₃V₂ 
0.5 x 30 = M₂ x 500 
M₂ = \(\frac{0.5 \times 30}{500}\)
= 0.03 M. 

Question 2.4. 
Calculate the mass of urea (NH₂CONH₂) required in making 2.5 kg of 0.25 molal aqueous solution. 
Answer:
Molality = 0.25 m 
i.e. O.25 moles of urea are present in 1000 g of solvent. 
Molar mass of solute (NH₂CONH₂) 
= 14 × 2 + 4 x 1 + 12 + 16 
= 60 g mol^-1 
Mass of solute = Number of moles x Molar mass 
= 0.25 x 60 = 15 g 
Total mass of solution = 1000 + 15 = 1015 g 
∴1015 g of solution contains urea = 15 g 
∴ 2500 g of solution contain urea = \(\frac{15}{1015}\)
= 37 g  

Question 2.5. 
Calculate (a) molality, (b) molarity and (c) mole fraction of KI if density of 20% (mass/mass) aqueous solution of KI is 1.202 g mL^-1.
Answer: 
Mass of solute, (WB) = 20 g 
Mass of solvent, (WA) = 100 - 20 = 80 g 
Molar mass of KI, (MB) = 39 + 127 
= 166 g mol^-1 
Mass (a) Molarity:Volume of solution (V(mL)) = 
= \(\frac{100}{1 \cdot 202} \mathrm{ml}\)
WB = 20 g 
MB = 166 g mol^-1

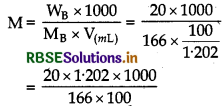
= 1. 44 mol L^-1 

(b) Molality (m) 
= \(\frac{W_B \times 1000}{M_B \times W_A}=\frac{20 \times 1000}{166 \times 80}\)

(c) Mole fraction of KI 

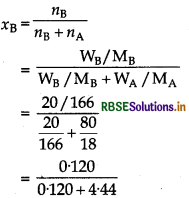
= 0·0263 

Question 2.6. 
H₂S a toxic gas with rotten egg like smell is used for qualitative anlaysis. If the solubility of H₂S in water at STP is 0.195 m. Calculate Henry's law constant. 
Answer:
Solubility of H₂S = 0.195 m 
i.e., Moles of H₂S. n_B = 0.195 mol 
Mass of solvent, M_A = 1000 g  
Molar mass of solvent (water), 
M_A = 18 g 
Moles of solvent (nH₂O) = \(\frac{1000}{18}\) = 55.5 mol 

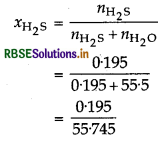
= 0.0035 
Pressure at STP (PH₂S) = 0.987 bar 
According to Henry's law, 
PH₂S = KH x xH₂S
\(\mathrm{K}_{\mathrm{H}}=\frac{p_{\mathrm{H}_2 \mathrm{~s}}}{x_{\mathrm{H}_2 \mathrm{~s}}}\)
or
\(\mathrm{K}_{\mathrm{H}}=\frac{0.987}{0.0035}\)
= 282 bar 

Question 2.7. 
Henry's law constant for CO₂ in water is 1.67 x 10⁸ Pa at 298 K. Calculate the quantity of CO₂ in 500 mL of soda water when packed under 2 : 5 atm CO₂ pressure at 298 K. 
Answer:
K_H = 1.67 x 108 Pa 
PCO₂ = 2.5 atm 
= 2.5 x 101325 Pa 
= 2.533 x 10⁵ Pa 
\(x_{\mathrm{CO}_2}=\frac{p_{\mathrm{CO}_2}}{\mathrm{~K}_{\mathrm{U}}}=\frac{2.533 \times 10^5}{1.670 \times 10^8}\)
= 1.517 x 10^-3 
But, 
\(x_{\mathrm{CO}_2}=\frac{n_{\mathrm{CO}_2}}{n_{\mathrm{CO}_2}+n_{\mathrm{H}_2 \mathrm{O}}}\)
Since 
nCO₂ < < nH₂O
Therefore, 
\(x_{\mathrm{CO}_2}=\frac{n_{\mathrm{CO}_2}}{n_{\mathrm{H}_2 \mathrm{O}}}\)
Volume of water = 500 mL 
Thus, Mass of water = 500 g (d =1.00 g mL^-1) 
\(n_{\mathrm{H}_2 \mathrm{O}}=\frac{500}{18}\) = 22.78 mol
Therefore, 
1.57 x 10^-3 = \(\frac{n_{\mathrm{CO}_2}}{22 \cdot 78}\)
or 
nCO₂ = 1.517 x 10^-3 x 22.78 
= 42.14 x 10^-3 mol 
Quantity of CO₂ = 42.14 x 10^-3 x 44 
= 1.854 g. 

Question 2.8. 
The vapour pressure of pure liquids A and B are 450 and 750 mm Hg at 350 K respectively. Find out the composition of the liquid mixture if total pressure is 600 mm Hg. Also find the composition of vapour phase. 
Answer:
Vapour pressure of A, pA° = 450 mm Hg 
Vapour pressure of B, PB° = 750 mm Hg 
Total vapour pressure p = PA + PB 
= PA° XA + PB° XB 
600 = 450 x xA + 750(1 - xA) 
600 = 450_xA + 750 - 750_xA 
350_xA = 150 
\(\frac{150}{300}\) = 0.5 
X_B = 1 - X_A 
= 1 - 0.5 = 0.5 
PA = 0.5 x 450 = 225 mm Hg 
PB = 0.5 × 750 = 375 mm Hg 
p = 225 + 375 = 600 mm Hg 
Mole fraction of A in vapour phase, 
\(x_{\mathrm{A}}=\frac{p_{\mathrm{A}}}{p}\)
= \(\frac{225}{600}\)
= 0.375 
Mole fraction of B in vapour phase, 
\(x_{\mathrm{B}}=\frac{p_{\mathrm{B}}}{p}\)

= \(\frac{375}{600}\)
= 0.625 

Question 2.9. 
Vapour pressure of pure water at 298 K is 23.8 mm Hg. 50 g of urea (NH₂CONH₂) is dissolved in 850 gof water. Calculate the vapour pressure of water for this solution and its relative lowering. 
Answer:
Mass of urea, W_B = 50 g 
Molar mass of urea, M_B = 60 g mol^-1 
Mass of water, W_A= 850 g 
Molar mass of water, M_A = 18 g mol^-1 
Vapour pressure of pure water, 
pA° = 23.8 mm Hg 
Vapour pressure of solution, 

= 0·017 
Relative lowering of vapour pressure = 0.017 

238 - P_A = 0.017 x 23.8 
23.8 - P_A= 0.4046 
P_A = 23.8 - 0.4046 
= 23.3954 mm Hg 

Question 2.10. 
Boiling point of water at 750 mm Hg is 99. 63°C. How much sugar is to be added to 500 g of water such that it boils at 100°C. 
Answer:
Molar mass of sucrose (C₁₂H₂₂O₁₁), 
M_B = 12 x 12 +1 x 22 + 16 x 11 
= 342 g mol^-1 
K_b (for water) = 0·52 K kg mol^-1
∆Tb = 100 - 99.63 
= 0.37°C = 0.37K 
Mass of water, W_A = 500 g 
Mass of sucrose, W_B = ? 

= 0.122 kg = 122 g 

Question 2.11. 
Calculate the mass of ascorbic acid (vitamin C, C₆H₈O₆) to be dissolved in 75 g of acetic acid to lower its melting point by 1.5°C. Kf = 3.9K kg mol^-1. 
Answer:
Mass of acetic acid, W_A = 75 g 
Mass of ascorbic acid, W_B = ? 
Molar mass of ascorbic acid (C₆H₈O₆), 
M_B = 12 x 6 + 1 + 8 + 16 x 6 
= 176 g mol^-1 
Kf = 3.9 K kg mol^-1 

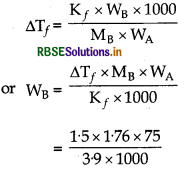
= 5.08 g.

Question 2.12. 
Calculate the osmotic pressure in Pascals exerted by a solution prepared by dissolving 1.0 g of polymer of molar mass 185,000 in 450 mL of water at 37°C.
Answer:
Mass of polymer, W_B =1.0g 
Molar mass of polymer, M_B = 185000 g mol^-1 
Temperature, T = 37 + 273 = 310 K 
Volume, V = 450 mL = 0·450 L 
Gas constant, R = 8.314 k pa LK^-1 mol^-1 
= 8.314 × 103 Pa LK^-1 mol^-1 
Osmotic pressure π = ? 

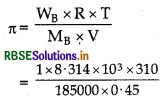
= 30.96 Pa. 

RBSE Class 12 Chemistry Solutions Textbook Questions and Answers

Question 2.1. 
Define the term solution. How many types of solutions are formed ? Write in brief about each type of solution with an example. 
Answer:
A homogeneous mixture of two or more than two components is called solution. A solution mainly has two components: 
(i) Solvent : The component which is present in the largest quantity is called solvent. Solvent determines the physical state in which solution exists. 
(ii) Solute : The component which is present in lesser quantity is called solute. A solution may contain one or more solutės. 
Types of solutions: Depending upon the physical state of solute and solvent, solutions may be classified in following categories:

Type of Solutions	Solute	Solvent	Examples
1. Gaseous Solutions	Gas	Gas	Mixture of nitrogen and oxygen gases Chloroform mixed with nitrogen gas Camphor in nitrogen gas
	Liquid	Gas
	Solid	Gas
2. Liquid Solutions	Gas	Liquid	Oxygen dissolved in water Ethanol dissolved in water Glucose dissolved in water
	Liquid	Liquid
	Solid	Liquid
3. Solid Solutions	Gas	Solid	Solution of hydrogen in palladium Amalgam of mercury with sodium Copper dissolved in gold
	Liquid	Solid
	Solid	Solid

Question 2.2. 
Suppose a solid solution is formed between two substances, one whose particles are very large and the other whose particles are very small. What kind of solid solution is this likely to be? 
Answer:
Interstitial solid solution

Question 2.3. 
Define the following terms: 
(i) Mole fraction, 
(ii) Molality, 
(iii) Molarity, 
(iv) Mass percentage. 
Answer:
Whe a liquid is alioweci to evaporate in a closed vessel, a part of the liquid evaporates and gets converted into vapours. Since the liquid is taken in a closed vessel, the evaporating molecules cannot escape to the atmosphere. Therefore, these vapours get collected in the available space over the liquid surface, As the evaporation proceeds, the number of molecules in the vapour phase gradually increases.

These molecules move randomly, some of these strike the surface of liquid and get condensed. Thus both evaporation and condensation processes occur simultaneously. Ultimately, a stage is reached when the rate of evaporation and rate condensation become equal to each other and an equilibrium is established between liquid and vapour phases. The pressure exerted by the vapours of the liquid at this stage is called vapour pressure. Thus, “the pressure exerted by the vapours of the liquid in equilibrium with the liquid at a given temperature is called vapour pressure.”

Factors affecting vapour pressure of a liquid Vapour pressure of a liquid depends upon the following factors:
(I) Nature of the liquid: Each liquid has a characteristic vapour pressure. Vapour pressure of a liquid depends on the magnitude of the intermolecular forces present between its molecules. The liquids, which have weaker intermolecular forces between their molecules, tend to escape readily into vapour phase and hence, have higher vapour pressure. On the other hand, if these forces are strong. then the molecules will not change into the vapours so readily and therefore, the vapour pressure will be low.

For example : Dimethyl ether and aIco1Q1 have higher vapour pressure than water at a given temperature because intermolecular forces in dimethyl ether and alcohol are weaker as compared to water.

(ii) Temperature of the liquid : The vpour pressure of a liquid increases with increase in temperature. It is because when the temperature is increased, the average kinetic energy of the molecu les in the liquid also increases. As a result, more molecules will escape from the surface of liquid into the vapour phase and consequently the vapour pressure of the liquid will increase. The effect of temperature on the vapour pressure of a liquid is expressed in terms of Clausius Clapeyron equation

Question 2.4. 
Concentrated nitric acid used in the laboratory work is 68% nitric acid by mass in aqueous solution. What should be molarity of such sample of acid if the density of solution is 1.504 g mL^-1? 
Answer:
Mass of solute (W_B) = 68 g 
Molar mass of solute (M_B) = 63 g/mol 
Density (d) = 1.504 g/ml 
Mass of solution = 100 g 

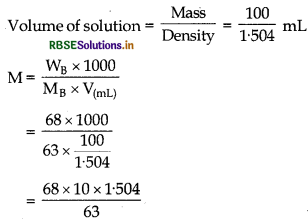
= 16.23 M 

Question 2.5. 
A solution of glucose in water is labelled as 10% w/W, what would be the molality and mole fraction of each component in the solution ? If the density of solution is 1.2 g mL^-1, then what shall be the molarity of the solution? 
Answer:
10% (w/W) aqueous solution of glucose means 10g of glucose is present in 100 g of solution i.e., 90g water is present in the solution. 

(i) Molality:Moles of glucose = 10/180 = 0.0556 
Mass of water = 90 g 

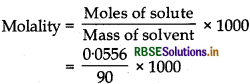
= 0.618 mL

(ii) Mole fraction: Moles of glucose = 0.0556 
Moles of water = \(\frac{90}{18}\)

Mole fraction of glucose = \(\frac{0.0556}{0.0556+5 \cdot 0}\)

= 0.01
Mole fraction of water = 1 - 0.01 = 0.99 

(iii) Molarity:Moles of glucose = 0.0556 

= 0.667 M 

Question 2.6. 
How many mL of 0.1 M HCl are required to react completely with 1 g mixture of Na₂CO₃ and NaHCO₃ containing equimolar amount of both? 
Answer:
Let x g of Na₂CO₃ is present in mixture: 
Amount of NaHCO₃ = (1 - x) g 
Molar mass of Na₂CO₃ = 2 x 23 + 12 +3 x 16 
= 106 g/mol 
Molar mass of NaHCO₃ = 23 + 1 + 12 +3 x 16 
= 84 g/mol 
Moles of Na₂CO₃ = x /106 
Moles of NaHCO₃ = \(\frac{1-x}{84}\)
Since number of moles of both in mixture are equal. 
\(\frac{x}{106}=\frac{1-x}{84}\)
= 0.558 g 
Moles of Na₂CO₃ = \(\frac{0 \cdot 558}{106}\) = 0.00526 
Moles of NaHCO₃ = \(\frac{1-0.558}{84}\)
= \(\frac{0.442}{84}\)
= 0.00526. 

Calculation of required moles of HCl: 
Na₂CO₃ + 2HCI → 2NaCl + H₂O + CO₂ 
NaHCO₃ + HCl → NaCl + H₂O + CO₂↑ 
According to the reaction 
1 mol of Na₂CO₃ requires HCl = 2 mol 
0.00526 mol of Na₂CO₃ requires HCl 
= 0.00526 x 2 mol 
= 0.01052 mol 
1 mol of NaHCO₃ requires HCl = 1 mol 
0.00526 mol of NaHCO₃ requires HCl
= 0 00526 x 1 mol 
= 0.00526 mol 
Total required moles of HCl = 0.01052 + 0.00526 
= 0.01578 mol. 

Calculation of volume of 0.1 M HCI: 
0.1 mol of 0.1 M HCl is present in = 1000 mL of HCl 
0.01578 mol of 0.1 M HCl is present in 
= \(\frac{1000}{0.1} \times 0.01578\)
= 157.8 mL of HCl.

Question 2.7. 
A solution is obtained by mixing 300 g of 25% solution and 400 g of 40% solution by mass. Calculate the mass percentage of the resulting solution. 
Answer:
Amount of solute in 300 g of 25% solution 
= 25/100 x 300 = 75 g 
Amount of solute in 400 g of 40% solution 
= 40/100 x 400
= 160 g 
Total amount of solute = 75 + 160 = 235 g 
 ∴ Total mass of solution = 300 + 400 = 700 g 
% of solute in final solution = 70 x 100 = 33.57% 
% of water in final solution = 100 - 33.57 = 66-43%. 

Question 2.8. 
An antifreeze solution is prepared from 222.6 g of ethylene glycol (C₂H₆O₂) and 200 g of water. Calculate the molality of the solution. If the density of the solution is 1.072 g mL^-1, then what shall be the molarity of the solution? 
Answer:
Mass of solute, (W_B) = 222.68 
Molar mass of solute (C₂H₄(OH₂)] (M_B) = 62 g/mol 
Mass of solvent (W_A) = 200 g 
Molar mass of solvent (M_A) = 18 g mol^-1 
 
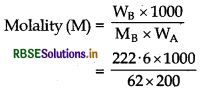
= 17.95 mol kg^-1 
density of solution = 1.072 g/ml 
mass of solution = 222.6 + 200 = 422.6g 

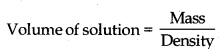
= \(\frac{422 \cdot 6}{1 \cdot 072}\)
= 394.2 ml
Molarity = \(\frac{W_B \times 1000}{M_B \times V_{(m L)}}\)
= \(\frac{222 \cdot 6 \times 1000}{62 \times 394 \cdot 2}\)
= 9.1 mol L 

Question 2.9. 
A sample of drinking water was found to be severely contaminated with chloroform (CHCl₃), supposed to be a carcinogen. The level of contamination was 15 ppm (by mass): (i) express this in percent by mass (ii) determine the molality of chloroform in the water sample. 
Answer:
15 ppm (by mass) means 15 g of CHCl₃ is present in 100 g of solution. 
(i) Percentage by mass =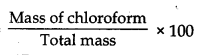 
= \(\frac{15}{10^6} \times 100\)
= 1.5 x 10-3 % 

(ii) Calculation of molality 
Mass of CHCl₃ = 15 g 
Molar mass of CHCl₃ = 12 + 1 + 35.5 x 3 = 119.5 
15 Moles of CHCl₃ = 15/110.5 = 0.1255 mol 
Mass of water = (106) - (15) = 106 

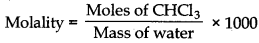
= \(\frac{0 \cdot 1255}{10^6} \times 1000\)
= 1.255 x 10^-4m 

Question 2.10. 
What role does the molecular interactions play in solution of alcohol and water? 
Answer:
There is strong hydrogen bonding between alcohol molecules as well as water molecules. On mixing alcohol and water, the molecular interactions become weak. Hence, they show positive deviation from ideal behaviour. Therefore, the solution will have higher vapour pressure and lower boiling point than that of alcohol and water. 

Question 2.11. 
Why do gases always tend to be less soluble in liquids as the temperature is raised ? 
Answer:
The dissolution of a gas in a liquid is exothermic process. Therefore, according to Le-Chatelier's principle, with increase in temperature, the equilibrium shifts in the backward direction. Hence, the solubility of gas in solution decreases with increase in temperature. 

Question 2.12. 
State Henry's law and mention some important applications. 
Answer:
Henry's law states that "the partial pressure of the gas in vapour phase (p) is proportional to the mole fraction of the gas. (x) in the solution.” 
p α x 
p = KH.X where, 
KH = Henry's constant 
x= Mole fraction of gas 
p= Pressure of the gas Applications of Henry's law: 
(i) To increase the solubility of CO₂ in soda water and soft drinks, the bottles are sealed under high pressure. 
(ii) At high altitudes, the pressure of oxygen is less than that at the ground level. Hence, the people living at high altitudes or climbers have low concentration of oxygen in the blood (As pressure decreases the solubility of gas decreases). Low blood oxygen causes climbers to become weak and unable to think clearly. These symptoms are called anoxia. Because of this reason climbers generally carry cylinders of oxygen in order to suppliment the deficiency of oxygen. 

Question 2.13. 
The partial pressure of ethane over a solution containing 6.56 x 10^-2 g of ethane is 1 bar. If the solution contains 5.00 x 10²g of the ethane, then what shall be the partial pressure of the gas? 
Answer:
According to Henry's law 
m = k x p 
Icondition: 6:56 x 10^-2 g = k + 1 bar 
k = 6:56 x 10^-2 g bar^-1 

II condition :5 00 10^-2g = (6.56 x 10^-2 g bar^-1) x p 
\(p=\frac{5.00 \times 10^{-2} \mathrm{~g}}{6.56 \times 10^{-2} \mathrm{~g} \mathrm{bar}^{-1}}\)
= 0.762 bar. 

Question 2.14. 
What is meant by positive and negative deviations from Raoult's law and how is the sign of AmixH related to positive and negative deviations from Raoult's law? 
Answer:
Positive deviation from Raoult's law occurs when the total vapour pressure of the solution is more than corresponding vapour pressure in case of ideal solution. 
P = PA + PB > PA° + PB°
Negative deviation from Raoult's law occurs when the total vapour pressure of the solution is less than corresponding vapour pressure in case of the ideal solution.
P = PA + PB < PA° + PB°
For positive deviation from Raoult's law, Δ _mix H has a positive sign.
For negative deviation from Raoult's law, Δ _mix H has a negative sign.

Question 2.15. 
An aqueous solution of 2% non-volatile solute exerts a pressure of 1.004 bar at the normal boiling point of the solvent. What is the molar mass of the solute? 
Answer:
Vapour pressure of pure water at boiling point 
(PÅ ) = 1 atm = 1 013 bar 
Vapour pressure of solution (p) = 1 004 bar 
Mass of solute (W_B) = 2 g 
Mass of solution = 100 g 
Mass of solvent (W_A) = 100 - 2 = 98 g 
Applying Raoult's law, 

= 41.0 g mol^-1. 

Question 2.16. 
Heptane and octane form an ideal solution. At 373 K, the vapour pressure of the two liquid components are 105.2 kPa and 46 : 8 kPa respectively. What will be the vapour pressure of a mixture of 26.0g heptane and 35 g of octane? 
Answer: 
Molar mass of heptane (C₇H₁₆) = 100 g mol^-1 
Molar mass of octane (C₃H₁₈) = 114 g mol^-1 
260 Moles of heptane = 26.0/100 = 0 .26 mol 
Moles of octane = \(\frac{35 \cdot 0}{114}\) = 0.31 

P(Heptane) = 0.456 x 105.2 kPa  = 47.97 kPa 
P(Octane) = 0.544 x 46.8 kPa  = 25.46 kPa 
Ptotal = 47.97 + 25. 46 
= 73. 43 kPa. 

Question 2.17. 
The vapour pressure of water is 12.3 kPa at 300 K.Calculate vapour pressure of 1 molal solution of a non-volatile solute in it. 
Answer: 
1 molal solution means 1 mol of solute is present in 1 kg of solvent. 
Moles of solvent = \(\frac{1000}{18}\) = 555 mol 
Mole fraction of solute (X_B) = \(\frac{1}{1+555}\)
= \(\frac{1}{56 \cdot 5}\)
= 0.0177 
According to Raoult's law, 
\(\frac{p_{\mathrm{A}}^{\circ}-p_{\mathrm{A}}}{p_{\mathrm{A}}^{\circ}}=x_{\mathrm{B}}\)
\(\frac{12 \cdot 3-p_{\mathrm{A}}^{\circ}}{12 \cdot 3}\) = 0.0177
12 3 - PÅ = 12.3 x 0.0177 
12.3 - pA° = 0.2177 
PA° = 12.08 kPa 
Vapour pressure of solution = 12.08 kPa
 
Question 2.18. 
Calculate the mass of a non-volatile solute (molar mass 40 g mol^{- 1}) which should be dissolved in 114 g of octane to reduce its vapour pressure to 80%. 
Answer:
Mass of solvent, (W_A) = 114 g 
Molar mass of solvent, (M_A) = 114 g 
Molar mass of solute, (M_B) = 40 g/mol 
Vapour pressure of octane, (pA°) = 100 mm 
Vapour pressure of solution, (PÅ )=80 mm 
Mass of solute, (W_B) = ? 

Mass of solute = 8 g.

Question 2.19. 
A solution containing 30 g of non-volatile solute exactly in 90 g of water has a vapour pressure of 2.8 kPa at 298 K. Further, 18 g of water is then added to the solution, the new vapour pressure becomes 2. 9 kPa at 298 K. Calculate: (i) molar mass of the solute (ii) vapour pressure of water at 298 K. 
Answer:
I case: Mass of solute, W_B = 30 g 
Molar mass of solvent, M_A = 18 g 
Mass of solvent, W_A = 90 g 
Vapour pressure of solution p= 2.8 kPa 
II case: (after adding water) 
Mass of solute, W_B = 30 g 
Molar mass of solvent, M_A = 18 g 
Mass of solvent, W_A = 90 + 18 
= 108 g 
Vapour pressure of solution, p = 2.9 kPa 
Since in both the cases, solute is same, hence molar mass of solute (M_B) will be same in both the cases. 

or
90(p° - 2.8) = 108(p° - 2.9) 
or
90p° - 90° x 2.8 = 108p° - 108 x 2.9 
or
90p° - 252 = 108p° - 3132 
or
108p° - 90p° = 313.2 - 252 
or
18p° = 61.2 
or 
p° = \(\frac{61 \cdot 2}{18}\)
p° = 3.4 kPa 
Vapour pressure of pure solvent = 3. 4 kPa 
Calculation of molar mass of solute (M_B) 

= 34 g/mol. 

Question 2.20. 
A 5% solution (by mass) of cane sugar in water has freezing point of 271 K. Calculate the freezing point of 5%glucose in water if freezing point of pure water is 273.15 K. 
Answer:
(i) For 5% solution of cane sugar 
Mass of solute, W_B = 5 g 
Mass of solvent, W_A = 100 - 5 = 95 g 
Molar mass of solute, M_B = 342 g/mol 
Freezing point of solution, T_s = 271 K 
Freezing point of pure water T = 273.15 K 
∆TF = Tp - Tf 
= 273.15 - 271 
= 2.15K 

Kf = 13 97 K kg mol^-1. 

(ii) For 5% glucose solution 
Mass of solute, W_B = 5g 
Molar mass of solute, M_B = 180 g mol^-1 
Mass of solvent, W_A = 95 g 
Kf = 13.97 K kg mol^-1 

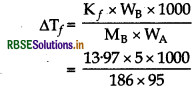
= 4.085 K 
Freezing point of 5% glucose solution 
= 273.15 - 4.085 = 269.065K 

Question 2.21. 
Two elements A and B form compounds having formula AB₂ and AB₄. When dissolved in 20 g of benzene (C₆H₆), 1 g of AB₂ lowers the freezing ponit by 2.3 K, whereas 1.0 g of AB₄ lowers it by 1.3 K. The molar depression constant for benzene is 5.1 kg mol^-7. Calculate atomic masses of A and B. 
Answer:
Mass of solvent, W_A = 20 g 
Mass of solute, W_B = 18 
∆TF= 23 K 
M_B = ? 
Kf = 5.1 K kg mol^-1 

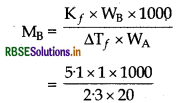
= 110.87 g/mol 
For compound AB₄, Mass of solvent, 
W_A = 20 g 
Mass of solute, W_B = 1 g 
∆Tf = 13K 
Kf = 5.1 K kg mol^-1 
M_B = ? 

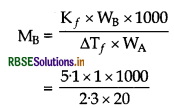
= 196·15 g/mol 

Molar mass of AB₂ = 110.87 g/mol 
Molar mass of AB₄ = 196.15 g/mol 
Let atomic mass of A is'd' and atomic mass of B is'b'. 
a + 2b = 110.87  ........ (i)
a + 4b = 196.15 ........... (ii)
Subtract eq (i) from (ii) 
or 
2b = 85.28 
b = 85.28/2 
Substituting the value of bin equation (i) 
a + 2b = 110.87 
or
a + 242.64 = 110.87 
a + 85.28 = 110.87 
a = 110.87 - 85.28 a 
= 25.59 g/mol 
Atomic mass of A = 25. 59 g mol^-1 
Atomic mass of B = 42. 64 g mol^-1. 

Question 2.22. 
At 300 K, 36 g of glucose present per litre in its solution has an osmotic pressure of 4. 98 bar. If the osmotic pressure of the solution is 1. 52 bar at the same temperature. What would be its concentration ? 
Answer: 
π = 4.98 bar 
T = 300 K 
V = 1L 
Mass of glucose, W_B = 36 g 
Molar mass of glucose, M_B = 6× 12 + 1 × 12 + 6 x 16 = 180 g mol^-1 
For first solution 
πV = nBRT 
\(\pi \mathrm{V}=\frac{\mathrm{W}_{\mathrm{B}}}{\mathrm{M}_{\mathrm{B}}} \times \mathrm{R} \times \mathrm{T}\)
4.98 x 1 = 36/180 x R x 300
= 0 083L bar/K mol 
For second solution, π = 1.52 bar 
C = ? 
R = 0.083 L bar K^-1 mol^-1 
T = 300 K 
V = 1L 
π = CRT 
\(\mathrm{C}=\frac{\pi}{\mathrm{RT}}\)
= \(\frac{1.52}{0.083 \times 300}\)
= 0.061 mol L. 

Question 2.23. 
Suggest the most important type of intermolecular attractive interaction in the following pairs: 
1. n-hexane and n-octane
2. 12 and CCl₄ 
3. NaClO₄ and water 
4. methanol and acetone: 
5. acetonitrile (CH₃CN) and acetone (C₃H₆O). 
Answer:

1. Both are non-polar, therefore, intermolecular attractive interactions in this pair are London forces. 
2. London forces. 
3. NaCl₄ dissociates in its aqueous solution to give Na⁺ and ClO₄^- ions and water is a polar molecule. Hence, intermolecular attractive interactions in this pair are ion dipole interactions. 
4. Both are polar molecules, therefore, intermolecular attractive interactions in this pair are dipole-dipole interactions. 
5. Dipole-dipole interactions. 

Question 2.24. 
Based on solute-solvent interactions, arrange the following in order of increasing solubility in n-octane and explain: cyclohexane, KCl, CH₃OH,CH₃CN. 
Answer:
(i) Cyclohexane and n-octane, both are non-polar compounds. Hence, they are completely miscible with each other in all proportions. 
(ii) KCl is an ionic compound, whereas n-octane is a non polar compound. Hence, KCl does not dissolve in n octane. 
(iii) CH₃OH and CH₃CN both are polar compounds but CH₃CN is less polar than CH₃OH. Since solvent is non polar, thus solubility of CH₃CN in n-octane is higher than that of CH₃OH. Hence, the order of solubility is as follows 
KCl < CH₃OH < CH₃CN < Cyclohexane 

Question 2.25. 
Amongst the following compounds, identify which are insoluble, partially soluble and highly soluble in water? 
1. phenol, 
2. toluene, 
3. formic acid, 
4. ethylene glycol, 
5. chloroform, 
6. pentanol. 
Answer:

1. Phenol is partially soluble in water because it has polar -OH group but it also has a non polar aromatic phenyl (- C₆H₅) group. Therefore, phenol is not highly soluble in water. 
2. Toluene is insoluble in water because it is a non polar compound and water is a polar solvent. 
3. Formic acid is highly soluble in water because formic acid forms hydrogen bond with water. 
4. Ethylene glycol is highly soluble in water because it forms hydrogen bond with water. 
5. Chloroform is insoluble in water because it is an organic liquid. 
6. Pentanol is partially soluble in water because it has polar -OH but large hydrocarbon part (C₅H₁₁) which is non-polar. 

Question 2.26. 
If the density of some lake water is 1.25 g mL^-1 and contains 92 g of Na⁺ ions per kg of water. Calculate the molality of Nations in the lake. 
Answer:
Mass of solute, W_B = 92 g 
Mass of solvent, W_A = 1 kg 
Molar mass of solute, M_B = 23 g mol^-1 
Molality, m = ? 

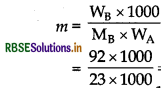
= 92/93
= 4 mol kg^-1 

Question 2.27. 
If the solubility product of CuS is 6 x 10^-16. Calculate the maximum molarity of Cus in aqueous solution. 
Answer:

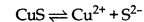
Ksp = [CU²⁺] [S^2-]
Ksp = s x s
ksp = s²
s = \(\sqrt{\mathrm{K}_{s p}}\)
= \(\sqrt{6 \times 10^{-16}}\)
= 2.45 x 10^-8 mol L^-1 
Molarity of CuS = 2.45 x 10^-8 mol L^-1 

Question 2.28. 
Calculate the mass percentage of aspirin (C₂H₂O₄) in acetonitrile (CH₃CN) when 6.5g of C₉H₂O₄ is dissolved in 450 g of CH₃CN. 
Answer:
Mass % of aspirin .

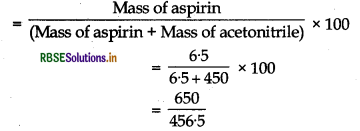
=1.424%. 
 
Question 2.29. 
 Nalorphene (C₁₉H₂₁NO₃), similar to morphine, is used to combat withdrawal symptoms in narcotic users. Dose of nalorphene generally given is 1.5 mg. Calculate the mass of 1.5 x 10^-3m aqueous solution required for the above dose. 
Answer: 
Mass of solute (W_B) = 1.5 mg = 1.5 10^-3g 
Molality (m) = 1.5 x 10^-3 mol kg^-1 
Molar mass of solute (M_B) = 19 x 12 + 21 x 1 + 14 + 3 x 16 
= 311 g mol^-1 
Mass of solvent Wa = ? 

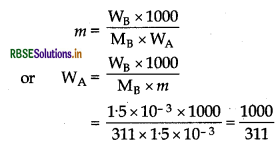
W_A = 3.21 g 
Mass of solution = Mass of solute + Mass of solvent = 0.0015 + 3.21 = 3. 2115 g. 

Question 2.30. 
Calculate the amount of benzoic acid (C₆H₅COOH) required for preparing 250 mL of 0. 15 M solution in methanol. 
Answer: 
Molarity = 0.15 M 
Volume = 250 ml 
Molar mass of solute M_B = 6 12 +5 1+12 + 2  x 16 + 1 = 122 g/mol 
Mass of solute, W_B = ? 

= 4.575 g. 

Question 2.31. 
The depression in the freezing point of water observed for the same amount of acetic acid, trichloroacetic acid and trifluoroacetic acid increases in the order given above. Explain briefly. 
Answer:
The acids may be arranged in the following order of depression in freezing point: 
acetic acid < trichloroacetic acid < trifluoroacetic acid 

This can be explained on the basis of degree of ionisation which depends on the strength of acid. Fluorine is highly electronegative, therefore, it is a strong electron 
withdrawing group. As a result, trifluoroacetic acid is strongest acid whereas acetic acid is weakest acid. Therefore, degree of ionisation of these acids will decrease as: 
trifluoroacetic acid > trichloroacetic acid > acetic acid 
Greater the degree of ionisation, greater will be number of ions produced and hence, greater is depression in freezing point. Hence, depression in freezing point will be highest for trifluoroacetic acid and lowest for acetic acid. 

Question 2.32. 
Calculate the depression in the freezing point of water when 10 g of CH₃ CH₂ CHClCOOH is added to 250 g of water Ka = 1.4 x 10^-3, Kf = 1.86 K kg mol^-1. 
Answer:
Molar mass of CH₃ CH₂ CHClCOOH (MB) 
= 4 × 12 + 7 x 1 + 355 + 2 x 16 
= 1225 g mol^-1 
Mass of solute, W_B = 10 g 
Mass of solvent, W_A = 250 g 
Molality (m) = \(\frac{W_B \times 1000}{W_A \times M_B}\)
= \(\frac{10 \times 1000}{122.5 \times 250}\)
= 0.3264 mol kg^-1. 
Let a is degree of dissociation 
\(\mathrm{CH}_3 \mathrm{CH}_2 \mathrm{CHClCOOH} \rightleftharpoons \mathrm{CH}_3 \mathrm{CH}_2 \mathrm{CHClCOO}++\mathrm{H}^{+}\)

Calculation of Van't Hoff factor 
α = \(\frac{i-1}{n-1}\)
n = 2 
α = i - 1 
or i = α + 1
i = 0065 +1 
i = 1065 
∆Tf = i Kfm 
= 1.065 x 186 x 0.3264 
= 0.65K 
Depression in freezing point = 0.65 K 

Question 2.33. 
19.5 g of CH₂FCOOH is dissoved in 500 g of water. The depression in the freezing point of water observed is 1.0°C. Calculate the Van't Hoff factor and dissociation constant of fluoroacetic acid. 
Answer: 
Mass of solute, W_B = 19.5g 
Mass of solvent, W_A = 500 g 
Depression in freezing point, ∆Tf = 1.0°C 
Molar mass of solute, MB = 78 g mol^-1 
Kf = 1.86 K kg mol^-1

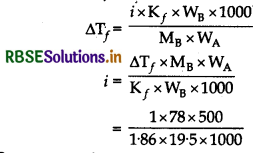
= 1.0753 
CH₂F COOH dissociates as 
\(\mathrm{CH}_2 \mathrm{FCOOH} \rightleftharpoons \mathrm{CH}_2 \mathrm{FCOO}^{-}+\mathrm{H}^{+}\)
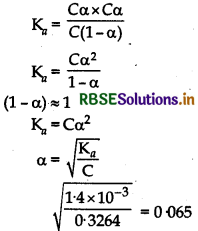
\(\begin{aligned} &\mathrm{K}_{\mathrm{a}}=\frac{\mathrm{C} \alpha \times \mathrm{C} \alpha}{\mathrm{C}(1-\alpha)} \\ &\mathrm{K}_a=\frac{\mathrm{C} \alpha^2}{1-\alpha} \end{aligned}\)
if 1 - α = 1
ka = Ca²

Kn = 2.835 x 10^-3. 

Question 2.34. 
Vapour pressure of water at 293 K is 17.535 mm Hg. Calculate the vapour pressure of water at 293 K, when 25g of glucore is dissolved in 450g of water. 
Answer:
Vapour pressure of water, p° = 17535 mm Hg 
Mass of solute, W_B = 25 g 
Mass of solvent, W_A = 450 g 
Molar mass of solute, M_B = 180 g mol^-1 
Molar mass of solvent, M_A = 18 g mol^-1 
Vapour pressure of solution, p = ?

or 
17.535 - p = 0.0974 
p = 17.535 - 0974 
= 17.4376 
= 17.44 mm Hg 
Vapour pressure of water in solution=17.44 mm Hg. 

Question 2.35. 
Henry's law constant for the molality of methane in benzene at 298 K is 4.27 x 10 mm Hg. Calculate the solubility of methane in benzene at 298 K under 760 mm Hg. 
Answer: 
KH = 4.27 x 10 mm Hg 
p = 760 mm Hg 
Applying Henry's law 
p = (K_H) (x_CH4)

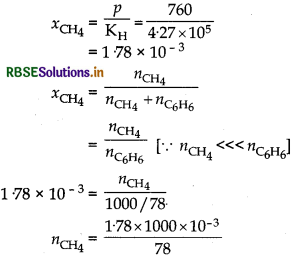
= 0.023 mol 
Solubility of methane in benzene in, mole fraction 
= 1.78 x 10^-3 

Question 2.36. 
100 g of liquid A (molar mass 140 g mol^-1) was dissolved in 1000 g of liquid B(molar mass 180 g mol^-1). The vapour pressure of pure liquid B was found to be 500 torr. Calculate the vapour pressure of pure liquid A and its vapour pressure in the solution if the total vapour pressure of the solution is 475 torr.
Answer:
Moles of liquid A (solute) = \(\frac{100 \mathrm{~g}}{140 \mathrm{~g} \mathrm{~mol}^{-1}}\)
= 5/7 mol 
Moles of liquid B (solvent) = \(\frac{1000 \mathrm{~g}}{180 \mathrm{~g} \mathrm{~mol}^{-1}}=\frac{50}{9} \mathrm{~mol}\)
Mole fraction of liquid A, X_A = \(\frac{5 / 7}{5 / 7+50 / 9}\)
= \(\frac{5 / 7}{395 / 63}\)
Mole fraction of liquid B, X_B = 1 - X_A 
= 1 - 0.114 = 0.886 
Given 
P_B = 500 torr 
Total vapour pressure, p = 475 torr 
p = P_A + P_B 
p = px_a + Px_b 
475 = pA° x 0114 + 500 x 0.886 
or
475 = 0.114pA° + 443
or 475 - 443 = 0.114 pA°
or 
\(\frac{32}{0 \cdot 114}=p_{\mathrm{A}}^{\circ}\) 
pA° = 280.7 torr
pA = pA° x_A
= 280.7 x 01.114 
= 32.0 torr
Vapour pressure of pure liquid A = 280.7 torr 
Vapour pressure of liquid A in solution = 32.0 torr. 

Question 2.37.
Vapour pressure of pure acetone and chloroform at 328 K are 741.8 mm Hg and 632.8 mm Hg respectively. Assuming that they form ideal solution over the entire range of composition, plot Ptotal, Pchloroform and Pacetone as a function of xacetone. The experimental data observed for different compositions of mixture is: 
Answer:

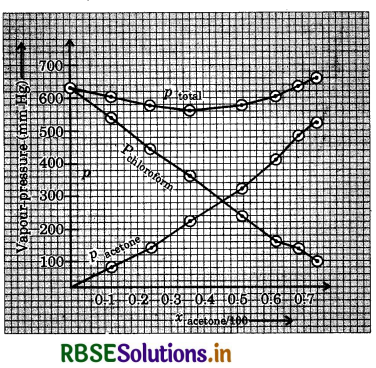
The plot for Ptotal dips downwards and therefore, the solution shows negative deviation from ideal behaviour. 

Question 2.38. 
Benzene and toluene form ideal solution over the entire range of composition. The vapour pressure of pure benzene and toluene at 300K are 50.71 mm Hg and 32.06 mm Hg respectively. Calculate the mole fraction of benzene in vapour phase if 80 g of benzene is mixed with 100 g of toluene. 
Answer:
Molar mass of benzene (C₆H₆)= 78 g mol^-1 
Molar mass of toluene (C₆H₅CH₃) = 92gmol^-1 
Moles of benzene = \(\frac{80 g}{78 \mathrm{~g} \mathrm{~mol}^{-1}}\)
= 1.026 mol 
Moles of Toluene = \(\frac{100 \mathrm{~g}}{92 \mathrm{~g} \mathrm{~mol}^{-1}}\)
= 1.087 mol 
Mole fraction of benzene x Benzene 
= im
Mole fraction of Toluene = \(\frac{1.026}{1.026+1.087}\)

= \(\frac{1 \cdot 026}{2 \cdot 113}\)
= 1 - 0.486 = 0514 
PBenzene = 50.71 mm Hg 
Proluene = 32 06 mmHg 
Applying Raoult's law 
Ptotal = P Benzene + PToluene 
Protal = PBenzene x XBenzene + PToluene x Toluene 
= 50.71 × 0.486 + 32.06 + 0.514 
= 24.645 + 16.479 
= 41 .24 mmHg 
In vapour phase 
PBenzene = Y Benzene x Ptotal 
P°Benzene x  XBenzene = YBenzene x Ptotal 
50.71 x 0.486 = Y Benzene x 41.124 
YBenzene = \(\frac{24 \cdot 645}{41 \cdot 124}\) = 0.599 
YToluene = 1 - 0.599 = 0.401 
Moles of benzene in vapour phase =0.599. 
 
Question 2.39. 
The air is a mixture of number of gases. The major components are oxygen and nitrogen with approximate proportion of 20% is to 79% by volume at 298 K. The water is in equilibrium with air at a pressure of 10 atm. Henry's law constants for oxygen and nitrogen at 298 K are 3.30 x 10⁷mm and 6.51 x 10⁷mm respectively. Calculate the composition of these gases in water. 
Answer: 
The vapour pressure of air over water = 10 atm 
Since the air contains 20% oxygen and 79% nitrogen by volume 
∴ Partial pressure of oxygen, 
PO₂ = imm = 2 atm 
= 2 x 760 mm = 1520 mm 
Partial pressure of nitrogen, 
PN₂ = imm 10 atm = 7.9 atm 
= 7.9 x 760 mm = 6004 mm 
KH(O₂) = 3.30 x 10⁷ mm 
KH(N₂) = 6.51 x 10⁷mm 
Applying Henry's law 
PO₂ = KH x xO₂ 

Mole fraction of O₂ = 4 61 × 10^-5 
Mole fraction of N₂ = 9.22 × 10^-5 

Question 2.40. 
Determine the amount of CaCl₂ (i = 2 .47) dissolved in 2 - 5 litre of water such that its osmotic pressure is 0.75 atm at 27°C. 
Answer:
For CaCl₂ i = 2.47 
π = iCRT = i nB/VRT 

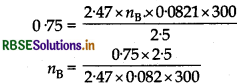
= 0.0308 mol 
Molar mass of CaCl₂ = 40 + 2 × 35.5
= 111 g mol^-1
Amount of CaCl₂ in grams 
= 111 × 0.0308 = 3.42g

Question 2.41 
Determine the osmotic pressure of a solution prepared by dossplving 25 mg of K₂SO₄ in 2 litre of water at 25°C assuming that it is completely dissociated.
Answer:
Amount of K₂SO₄,
W_B = 25 mg = 0.025g
Volume of Water V = 2L
T = 25°C = 25 + 273 = 298K
Molar mass of K₂SO₄ 
M_B = 2 x 39 + 32 + 4 x 16 
= 174 g mol-1
Since K₂SO₄ is completely dissociated
K₂SO₄ → 2K⁺ + SO₂^-4
Numbers of ions produced = 3
i = 3
π = iCRT
= i\(\frac{n_{\mathrm{B}}}{\mathrm{V}}\)RT
\(\begin{aligned} &=i \times \frac{\mathrm{W}_{\mathrm{B}}}{\mathrm{M}_{\mathrm{B}} \times \mathrm{V}} \times \mathrm{RT} \\ &=\frac{3 \times 0.025 \times 0.082 \times 298}{174 \times 2} \end{aligned}\)
= 5.27 x 10^-3 atm
Ostmotic pressure of K₂SO₄ = 5.27 x 10^-3 atm