RBSE Class 12 Chemistry Important Questions Chapter 2 Solutions

Rajasthan Board RBSE Class 12 Chemistry Important Questions Chapter 2 Solutions Important Questions and Answers.

RBSE Class 12 Chemistry Chapter 2 Important Questions Solutions

Multi Choice Questions (MCQ):

Question 1. 
The number of moles of NaOH required for the preparation of 5 litre 2m NaOH solution is:
(a) 10
(c) 5
(b) 1
(d) 2.5
Answer:
(a) 10

Question 2. 
A molal solution is that in which 1 mole solute is dissolved in:
(a) 1000 g solvent
(b) 1L solvent
(c) 1L solution
(d) 22.4 L solution 
Answer:
(a) 1000 g solvent

 

Question 3. 
The normality of 2M sulphuric acid is:
(a) 2N
(b) 4N
(c) N/2
(d) N/4
Answer:
(b) 4N

Question 4. 
The unit of molality is:
(a) mol L^-1
(b) mol g^-1
(c) mol Kg^-1
(d) mol ml^-1
Answer:
(c) mol Kg^-1

Question 5. 
The molality of water
(a) 18 mol L^-1
(b) 10^-7 mol L^-1
(c) 55.5 mol L^-1
(d) None of these
Answer:
(c) 55.5 mol L^-1

Question 6. 
Which of the following does not depend on temperature?
(a) Normality
(b) Molarity
(c) Formality
(d) Molality
Answer:
(d) Molality

Question 7. 
The number of milli-equivalents of solute in 60 mL of 4.0 M H₂SO₄ is:
(a) 240
(b) 480
(c) 24
(d) 48
Answer:
(b) 480

Question 8. 
How much water is added in 500 mL of 0.5 M NaOH so that the concentration of solution becomes 10 mg mL^-1?
(a) 100 mL
(c) 250 mL
(b) 200 mL
(d) 500 mL
Answer:
(d) 500 mL

Question 9. 
The mole fraction of one molal aqueous solution is:
(a) 0.031
(b) 0.042
(c) 0.018
(d) 0.026
Answer:
(c) 0.018

Question 10. 
10 L dilute solution is prepared from 1L of 0.5 M H₂SO₄, The molarity of solution obtained is:
(a) 1 M
(b) 0.05 M
(c) 0.5M
(d) 0.1 M
Answer:
(b) 0.05 M

Question 11. 
Which of the following is homogeneous system?
(a) Muddy water
(b) Bread
(c) Concrete
(d) Solution of sugar in water
Answer:
(a) Muddy water

Question 12. 
The solution of solute per kilogram solvent is called:
(a) Normality
(b) Molarity
(c) Mole fraction
(d) Molality
Answer:
(d) Molality

Question 13. 
The number of moles in per litre solvent is called:
(a) Molality
(b) Molarity
(c) Formality
(d) Normality
Answer:
(c) Formality

Question 14. 
If 250 ml solution of 0.25 M NaCl is diluted with water to make its volume 500 mL then the new concentration of solution is:
(a) 0.167 M
(b) 0.125 M
(c) 0.0833 M
(d) 0.0167 M
Answer:
(b) 0.125 M

Question 15. 
500 mL solution of glucose contains 6.02 x 10²² molecules. The concentration of solution will be:
(a) 0.1 M
(b) 1.0M
(c) 0.2M
(d) 2.0 M
Answer:
(a) 0.1 M

Question 16. 
Which is correct for benzene and toluene?
(a) ∆Hmix  ≠  0
(b) ∆Vmix ≠ 0
(c) ∆Hmix ≠ 0
(d) None of these
Answer:
(c) ∆Hmix ≠ 0

Question 17. 
The vapour pressure of liquid
(a) increases with increase in temperature 
(b) does not depend on temperature
(c) decreases with increase in temperature 
(d) None of the above
Answer:
(a) increases with increase in temperature 

Question 18. 
According to Raoult's law, the relative lowering of vapour pressure of solution of non-volatile solute is: 
(a) equal to precentage of solute
(b) equal to mole fraction of solute
(c) equal to mole fraction of solvent
(d) equal to mass precentage of solute
Answer:
(b) equal to mole fraction of solute

Question 19. 
Which of the solution represents positive deviation?
(a) Ethyl bromide + Methyl bromide
(b) Cyclohexane + Ethanol
(c) Chloroform + Acetone
(d) None of the above
Answer:
(b) Cyclohexane + Ethanol

Question 20. 
Which of the following represents negative devia- tion?
(a) Water + Hydrochloric acid
(b) Benzene + Toluene 
(c) CS₂ + Chloroform 
(d) None of the above
Answer:
(a) Water + Hydrochloric acid

Very Short Answer Type Questions:

Question 1. 
Give reason for the following. Aquatic animals are more comfortable in cold water than in warm water. 
Answer:
Oxygen is present in dissolved state in water. As per Henry's law when temperature rises solubility of a gas decreases in solvent, it means solubility of oxygen in warm water is less than cold water. This makes aquatic species respirate comfortably in cold water.

Question 2. 
Define the term mole fraction.
Answer:
The mole fraction of a component is the ratio of the number of moles of the component to the total number of moles of all the components present in the solution. Mole fraction of a component

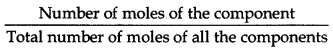
For a binary solution, mole fraction of component A,
\(\chi_{\mathrm{A}}=\frac{n_{\mathrm{A}}}{n_{\mathrm{A}}+n_{\mathrm{B}}}\)
Similarly, for B,
\(\chi_{\mathrm{B}}=\frac{n_{\mathrm{B}}}{n_{\mathrm{A}}+n_{\mathrm{B}}}\)
and
X_A + X_B^-1

Question 3. 
Explain the Henry's law about dissolution of a gas in a liquid.
Answer:
Henry's law states that, the partial pressure of the gas
in vapour phase (p) is directly proportional to the mole fraction of the gas (x) in the solution.
p = KH_xx
Here, KH = Henry's law constant.
Different gases have different Ky values at the same temperature.

Question 4. 
State the main advantage of molality over molarity as the unit of concentration.
Answer:
Molality does not change with change in temperature while, molarity decreases with rise in temperature.

Question 5. 
Define an ideal solution and write one of its charac- teristics.
Answer:
The solutions which obey Raoult's law over the entire range of concentration are known as ideal solutions.
For ideal solutions, ∆H (mixing) = 0 and ∆V (mixing) = 0, e.g. solution of n-hexane and n-heptane, bromóethane and chloroethane, etc.
Or
In these solutions (binary solutions), A-B type (i.e. solute-solvent) interactions are nearly equal to the A - A (solute-solute) and B - B type (solvent-solvent) interactions.

Question 6. 
Some liquids on mixing form azeotropes. What are azeotropes?
Answer:
Azeotropes are binary mixture having the same composition in liquid and vapour phase and boil at constant temperatures.

Question 7. 
State Raoult's law.
Or 
Define Raoult's law in its general form in refer- ences to solutions.
Or 
State Raoult's law for a solution of volatile liq- uids.
Answer:
Raoult's law For a solution of volatile liquids, the par- tial vapour pressure of each component of the solu- tion is directly proportional to its mole fraction present in solution. Thus, for any component, partial vapour pressure.
p_xx ⇒ p = p_ox
where, po is the vapour pressure of pure component and y is the mole fraction of that component.

Question 8. 
What are isotonic solutions?
Answer:
Two solutions having the same osmotic pressure at a given temperature are called isotonic solutions.

Question 9. 
Define the term osmotic pressure.
Answer:
Osmotic pressure is the extra pressure which is applied on the solution just to prevent the flow of solvent into the solution through a semipermeable membrane.

Question 10. 
What is meant by reverse osmosis?
Answer:
If a pressure higher than the osmotic pressure is ap- plied in the solution, the solvent will flow from the solution into the pure solvent through the semi-permeable membrane and the process is called reverse osmosis.

Question 11. 
Define the following terms.
1. Isotonic solutions 
2. van't Hoff factor
Answer:

1. Two solutions having the same osmotic pressure at a given temperature are called isotonic solutions.
2. van't Hoff factor It is defined as the ratio of the experimental value of colligative property to the cal- culated value of the colligative property and is used to find out the extent of dissociation or association. Math- ematically, it is represented as Observed colligative property Calculated colligative property.

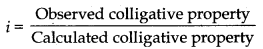
Question 12. 
Explain boiling point elevation constant for a solvent or ebullioscopic constant.
Answer:
The boiling point elevation constant is equal to the elevation in boiling point when I mole of a solute is dissolved in 1 kg of solvent. It is also called ebullioscopic constant.

Short Answer Type Questions:

Question 1. 
Give reason for the following:
(a) Aquatic species are more comfortable in cold water than warm water.
(b) At higher altitudes people suffer from anoxia re- sulting in inability to think.
Answer:
(a) Oxygen is present in dissolved state in water. As per Henry's law when temperature rises solubility of a gas decreases in solvent, it means solubility of oxygen in warm water is less than cold water. This makes aquatic species respirate comfortably in cold water.

(b) At higher altitude people suffer from anoxia be- cause at higher altitudes, the partial pressure of oxy- gen is less than that at ground level. This leads to low concentration of oxygen in the blood and tissues of people.

Question 2. 
Define the following terms:
(i) Mole fraction (x)
(ii) Molality of a solution (m)
Answer:
(i) The mole fraction of a component is the ratio of the number of moles of the component to the total number of moles of all the components present in the solution. Mole fraction of a component


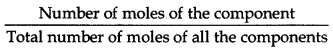
(ii) Molality of solution (m) Molality is defined as the number of moles of the solute per kilogram of the solvent. It is represented by m Number of moles of solute

Question 3. 
Calculate the molarity of 9.8% (w/w) solution of H₂SO₄, if the density of the solution is 1.02 g mL^-1. [Molar mass of H₂SO₄ = 98 gmol^-1]
Answer:
9.8% solution of H₂SO₄ means 9.8 g of H₂SO₄ is present in 100 g of the solution. Thus,
Mass of H₂SO₄ dissolved = 9.8 g 
Mass of solution = 100 g
Density of solution = 1.02 g mL^-1
Moles of H₂SO₄ = \(\frac{9.8 \mathrm{~g}}{98 \mathrm{~g} \mathrm{~mol}^{-1}}\)
= 0.1 mol

= \(\frac{100 \mathrm{~g}}{1.02 \mathrm{~g} \mathrm{~mL}^{-1}}=\frac{100}{1.02} \mathrm{~mL}\)
= 98.04 mL or 0.09804 L

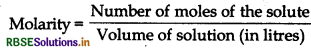
= \(\frac{0.1 \mathrm{~mol}}{0.09804 \mathrm{~L}}\)
= 1.019 mol L^-1 or 1.02 M.

Question 4. 
Differentiate between molarity and molality of a solution. How can we change molality value of a solution into molarity value?
Answer:
Molality is defined as the number of moles of the solute per kilogram of the solvent. It is represented by m.

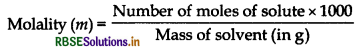
It does not change with change in temperature. Molarity is defined as the number of moles of solute dissolved in one litre or one cubic decimetre of the solution.

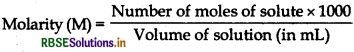
It decreases with increase in temperature (as V α T). We can change molality value of a solution into molarity value by using following relation:
Molality (m) = \(\frac{M \times 1000}{(1000 \times d)-\left(M \times M_2\right)}\)
where, M is the molarity and M₂ is the molar mass of component 2 (generally solute) and d is the density of solution (in gcm-³).

Question 5. 
A solution of glucose (CH₁₂O₆) in water is labelled as 10% by weight. What would be the molality of the solution? (Molar mass of glucose = 180 gmol^-1). 
Answer:
A 10% glucose solution by weight means that 10 g glucose is present in 100 g solution. Number of moles of 10 g glucose
= \(\frac{10}{180}\) =  0.0555 mol
Weight of glucose = 10 g
Weight of water = 90 g
= \(\frac{90}{1000} \mathrm{~kg}\)
= 0.09 kg

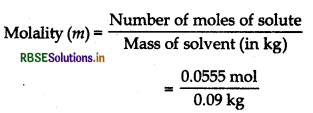
= 0.61 mol kg^-1 or 0.61 m

Question 6. 
If the density of water of a lake is 1.25 g mL^-1 and 1 kg of lake water contains 92 g of Na⁺ ions, calculate the molarity of Na⁺ ions in this lake water. (Atomic mass of Na = 23 g mol^-1).
Answer:
(i) Find volume of solution using the formula V = imm
(ii) While calculating volume of solution, total mass, i.e. mass of solute + mass of solvent is taken.
Given,
d = 1.25 g mL^-1, 
W₂ = 92g, 
W₁ = 1 kg or 1000 g, 
M₂ = 23 g mol^-1
We know that, density (d) of solution

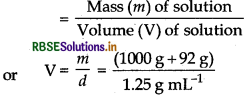
[Mass of solution = mass of solvent + mass of solute]
= \(\frac{1092}{1.25} \mathrm{~mL}\)
= 873.6 × 10^-3 L
Moles of solute = \(\frac{92 \mathrm{~g}}{23 \mathrm{~g} \mathrm{~mol}^{-1}}\) = 4 mol

= 4.579 mol L^-1 or 4.58 M

Question 7. 
Differentiate between molarity and molality for a solution. How does a change in temperature influence their values?
Answer:

Molality is defined as the number of moles of the solute per kilogram of the solvent. It is represented by m.

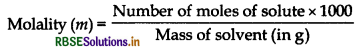
It does not change with change in temperature. Molarity is defined as the number of moles of solute dissolved in one litre or one cubic decimetre of the solution.

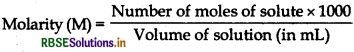
Question 8. 
State Henry's law and mention its two important applications.
Answer:
Henry's law states that, the partial pressure of the gas
in vapour phase (p) is directly proportional to the mole fraction of the gas (x) in the solution.
p = KH_xx
Here, KH = Henry's law constant.
Different gases have different Ky values at the same temperature.
Application of Henry's Law

1. To increase the solubility of CO₂ in soft drinks and soda water, the bottle is sealed under high pressure. 
2. To minimise the painful effects of bends or decom- pression sickness in deep sea divers oxygen diluted with less soluble helium gas is used as breathing gas. 

Question 9. 
What type of azeotropic mixture will be formed by a solution of acetone and chloroform? Justify on the basis of strength of intermolecular interactions that develop in the solution.
Answer:
The mixture of acetone and chloroform is an example of maximum boiling azeotrope. It shows negative deviation from Raoult's law because of increase in inter molecular forces of attraction between acetone and chloroform, since they form hydrogen bonds between them. 

Question 10. 
State Raoult's law for a solution containing volatile components. Write two characteristics of the solu- tion which obeys Raoult's law at all concentrations. 
Answer:
Raoul's law states that "at a given temperature, for a solution of volatile liquids, the partial vapour pres- sure of each component of the solution is directly proportional to its mole fraction present in the solution." The solutions which obey Raoult's law over the entire range of concentration are known as ideal solutions. The solutions which obey Raoult's law over the entire range of concentration are known as ideal solutions. The important properties of these solutions are:
(i) the enthalpy of mixing of pure components to form the solution of mixing of pure components to form the solution is zero, i.e. ∆mix H = 0.
(ii) the volume of mixing is also zero, i.e. ∆mix V = 0.

Question 11. 
Write two differences between an ideal solution and a non-ideal solution.
Answer:

Ideal solution	Non-ideal solution
The components of this solution obey Raoult's law at all temperatures and concentrations. i.e. pA : P°A XA and PB P A Xa On mixing. there is no enthalpy and volume change i.e.  ∆mix H = O and ∆mix V = 0	The components of this solution do not obey Raoult's positive and negative deviations from Raoults law. i.e. PA ≠ P°A xA  and PB ≠ PB XB On mixing, there is enthalpy and volume change i.e ∆mix H ≠ 0 and ∆mix V ≠ 0

 

Question 12. 
Define the following:
(i) Ideal solution
(ii) Molarity (M)
Answer:
(i) The solutions which obey Raoult's law over the entire range of concentration are known as ideal solutions.
For ideal solutions, ∆H (mixing) = 0 and ∆V (mixing) = 0, e.g. solution of n-hexane and n-heptane, bromóethane and chloroethane, etc.
Or
In these solutions (binary solutions), A-B type (i.e. solute-solvent) interactions are nearly equal to the A - A (solute-solute) and B - B type (solvent-solvent) interactions.

(ii) Molarity (M) Molarity is defined as the number of moles of solute dissolved in one litre or one cubic decimetre of the solution.

e.g. 0.25 mol L^-1 (or 0.25 M) solution of NaOH means that 0.25 mole of NaOH has been dissolved in one litre (or one cubic decimetre) of solution.

Question 13. 
1. Gas (A) is more soluble in water than gas (B) at the same temperature. Which one of the two gases will have the higher value of K (Henry's constant) and why?
2.  In non-ideal solution, what type of deviation shows the formation of maximum boiling azeotropes?
Answer:
Solubility is inversely proportional to KH i.e. Henry's .constant of the gas.

1.  Greater the value of KH, lower is the solubility of the gas. As gas (A) is more soluble in water than gas (A) has lower value of KH. In other words, gas (B) has higher value of K, than gas (A) at the same temperature.
2. In non-ideal solutions, the solutions that show large negative deviation from Raoult's law form maximum boiling azeotropes e.g. Mixture of nitric acid and water.

Question 14. 
What is meant by negative deviation from Raoult's law? Give an example. What is the sign of ∆mix H for negative deviation?
Or 
What is meant by positive deviation from Raoult's law? Give an example. What is the sign of ∆mix H for positive deviation?
Or 
Non-ideal solutions exhibit either positive or negative deviations from Raoult's law. What are these deviations and why are they caused? Explain with one example for each type.
Answer:
Positive deviation means A - B interactions are weaker than A - A and B - B interactions while, opposite is true in case of negative deviation. For non-ideal solutions, vapour pressure is either higher or lower than that predicted by Raoult's law. If it is higher, the solution exhibits positive deviation and if it is lower, it exhibits negative deviation from Raoult's law.

In case of positive deviation, A - B interactions are weaker than A - A and B - B interactions. Due to this, vapour pressure increases which results in positive deviation. e.g. Ethanol + Acetone and Chloroform + Acetone show positive deviation.

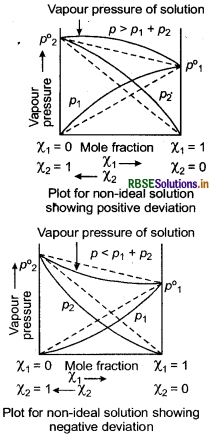
Question 15. 
Define azeotropes. What type of azeotrope is formed by negative deviation from Raoult's law? Give an example.
Or 
Define azeotropes. What type of azeotrope is formed by positive deviation from Raoult's law? Give an example.
Answer:
Azeotropes are binary mixture having the same composition in liquid and vapour phase and boil at constant temperatures.
Azeotropes showing negative deviation from Raoult's law form maximum boiling azeotropes at a specific composition, e.g. Azeotrope formed from nitric acid and water. Azeotropes showing positive deviation from Raoult's law form minimum boiling azeotropes at a specific composition e.g. Ethonol-water mixture.

Question 16. 
State Raoult's law for a solution containing volatile components. How does Raoult's law become a spe- cial case of Henry's law?
Or 
State Raoults law for the solution containing vola- tile components. What is the similarity between Raoult's law and Henry's law?
Answer:
Raoult's law as a special case of Henry's law In the solution of a gas in a liquid, one of the components is so volatile that it exists as a gas and its solubility is given by Henry's law which states that, p = KHx 
i.e. partial pressure of the volatile component (gas) is directly proportional to the mole fraction of that component (gas) in the solution. When the equations of Raoult's law and Henry's law are compared, it can be seen that the partial pressure of the volatile component or gas is directly proportional to its mole fraction in solution. Only the proportionality constant Ky differs from p°.

Hence, Raoult's law and Henry's law has been idential except that their proportionality constants are different. Therefore, Raoult's law becomes a special case of Henry's law in which KH becomes equal to vapour pressure of pure component po.

Question 17. 
Explain why a solution of chloroform and acetone shows negative deviation from Raoult's law?
Answer:
Negative deviation means A-B interactions are stron- ger than A - A and B - B interactions. A mixture of chloroform and acetone forms a solution which shows negative deviation from Raoult's law because chloroform molecule forms H-bonding with acetone molecule. Here, solute is chloroform and sol- vent is acetone. As a result of this, A-B interaction becomes stronger than A-A and B-B interactions. This decreases the escaping tendency of molecules for each component which leads to the decrease in vapour pressure and resulting in negative deviation from Raoult's law.

Question 18. 
Calculate the freezing point of a solution containing 60 g of glucose (Molar mass = 180 g mol^-1) in 250 g of water.
(Kf of water = 1.86 K kg mol^-1) 
Answer:
Molality of solution,
\(m=\frac{\mathrm{W}_{\mathrm{B}}}{\mathrm{M}_{\mathrm{A}}} \times \frac{1000}{\mathrm{~W}_{\mathrm{A}}}\)
where, weight of glucose
W_B = 60 g
molar mass of glucose
M_A = 180 g mol^-1
weight of solvent (water) W_A = 250 g
Putting values in Eq. (i)
= \(\frac{60 \mathrm{~g}}{180 \mathrm{~g} \mathrm{~mol}^{-1}} \times \frac{1000 \mathrm{~g}}{250 \mathrm{~g} \mathrm{~mol}^{-1}}\)
Molality m = 1.33
Now,
∆Tf = 1.86K kg mol^-1 x 1.33 m
∆Tf = 2.47 K
Freezing point of pure water = 273.15 K
∴ freezing point of solution = 273.15 - 2.47 K
= 270.68 K

Question 19. 
Give reasons for the following:
(i) Measurement of osmotic pressure method is preferred for the determination of molar masses of macro molecules such as proteins and polymers.
(ii) Elevation of boiling point of 1 M KCl solution is nearly double than that of 1 M sugar solution.
Answer:
(i) The osmotic pressure method has the advantage over other methods as pressure measurement is around the room temperature and the molarity of the solution is used instead of molality.

(ii) Elevation in boiling pont is directly proportional to 'i'. ∆Tb α i. Now as given in the question, elevation of boiling point of 1 M KCl solution is nearly double than that of 1 M sugar solution. It is because KCl being ionic, dissociates into K⁺ and Cl and therefore it's van't Hoff factor, i is 2 whereas for sugar van't Hoff factor is 1 as it does not undergoes such a dissociation.

Question 20. 
Define the following terms:
(i) Colligative properties 
(ii) Molality (m)
Answer:
(i) Colligative properties The properties of solutions which depends only on the number of solute particle, irrespective of their naure relative to the total number of particle present in the solution.
There are four important colligative properties.

1. Relative lowering of vapours pressure. 
2. Elevation of boiling point
3. Depression of freezing point
4. Osmosis and osmotic pressure

(ii) Molality (m) It is defined as the number of moles of the solute per kilogram of the solvent.
\(x = {-b \pm \sqrt{b^2-4ac} \over 2a}\)
e.g. 1.00 mol kg^-1 (or 1.00 m) solution of KCl means that 1 mole (74.5 g) of KCl is dissolved in 1 kg of water. 

Question 21. 
Define the following terms:
(i) Abnormal molar mass
Answer:
(i) Abnormal molar mass Molar mass that is either lower or higher than the expected or normal value is called abnormal molar mass, e.g. all the molecules of ethanoic acid associate in benzene, then ∆Tb or ∆Tf for ethanoic acid will be half of the normal value.

Question 22. 
(i) On mixing liquid X and liquid Y, volume of the resulting solution decreases. What type of deviation from Raoult's law is shown by the resulting solution? What change in temperature would you observe after mixing liquids X and Y?
(ii) What happens when we place the blood cell in water (hypertonic solution)? Give reason.
Answer:
(i) On mixing two liquids X and Y, the decreate in volume of the resulting solution suggests that a non- ideal solution is formed which shows negative devia- tion from Raoult's law. In case of the solutions showing negative deviations from Raoult's law, AH mixing (i.e. enthalpy of mixture) is negative. Thus, on mixing X and Y liquids evolution of heat takes places, i.e. the temperature of the resulting solution increases.

(ii) When we placed the blood cell in hypertonic solution then due to osmosis, water will flow out of the cell and it would shrink.

Question 23. 
Why does a solution containing non-volatile solute have higher boiling point than the pure solvent? Why is elevation of boiling point a colligative property? 
Answer:
The boiling point of the solution containing a non - volatile solute is always higher than that of the pure solvent because the vapour pressure of such a solution is lower than that of the pure solvent and vapour pressure increases with increase in temperature. Hence, the solution has to be heated more to make the vapour pressure equal to the atmospheric pressure. Elevation of boiling point is a colligative property because it depends upon the number of solute particles present in a solution.

Question 24. 
Calculate the mass of compound (molar mass = 256 g mol^-1) to be dissolved in 75 g of benzene to lower its freezing point by 0.48 K(Kf = 5.12 K kg mol^-1).
Answer:
Given that
Kf = 5.12K kg mol^-1, WB = 75 g,
∆Tf =  0.48 K, M_A = 256 g mol^-1

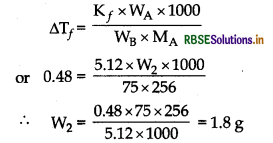
Question 25. 
How is the vapour pressure of a solvent affected when a non-volatile solute is dissolved in it?
Answer:
When a non-volatile solute is added to a solvent, its vapour pressure decreases because some of the sur- face sites are occupied by solute molecules. Thereby, the fraction of the surface covered by the solvent molecules gets reduced. Thus, less space is available for the solvent molecules to vaporise. Therefore, vapour pressure is also reduced. 

Question 26. 
An aqueous solution of sodium chloride freezes be- low 273 K. Explain the lowering in freezing point of water with the help of a suitable diagram.
Answer:
When a non-volatile solute is added to a solvent, the freezing point of the solution is always lower than that of pure solvent as the vapour pressure of the solvent decreases in the presence of non-volatile solute. Plot for the lowering in freezing point of water when NaCl is added to it is shown below.

Question 27. 
18 g glucose, C₆H₁₂O₆ (molar mass = 180 g mol^-1) is dissolved in 1 kg of water in a sauce pan. At what temperature, will this solution boil?
(Kb for water = 0.52K kg mol¹, boiling point of pure water = 373.15K).
Answer:
(i) First, find out ∆Tb by using formula.
\(\Delta \mathrm{T}_b=\frac{\mathrm{K}_b \times \mathrm{W}_b \times 1000}{\mathrm{M}_b \times \mathrm{W}_a}\)

(ii) Then, find out the value of Tb by using formula. ∆T2 = Tb - Tb
Given,
Wa = weight of H₂O (solvent) = 1 kg = 1000 g
W_b = weight of C₆H₁₂O₆ = 18 g
T_b = 373.15 K
K_b = 0.52K kg mol^-1 (glucose-solute)
Mb = Molar mass of solute = 180 g mol^-1 (glucose)

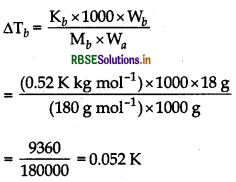
As we know that,
∆Tb = Tb - Tb
⇒ 0.052 = Tb - 373.15 
Tb = 373.15 + 0.052
= 373.202 K
= 373.20 K (approx)

Question 28. 
A 1.00 molal aqueous solution of trichloroacetic acid (CCI₃COOH) is heated to its boiling point. The solution has the boiling point 100.18°C. Determine the van't Hoff factor for trichloracetic acid. (Kb for water = 0.512K kg mol^-1).
Answer:
Consider the relation,
∆Tb = iKpn
Molar of solution
m = 1.00 m
Boiling point solution,
Tb = 100C = 373.18 K
Boiling point of water (solvent)
Tb = 100.18°C  = 373 K
∆Tb = Tb - Tb =  373.18 K - 373 K
= 0.18 K
∆Tb = iKbm
0.18K = i x 0.512 Kkg mol^-1 x 1mol kg^-1
\(i=\frac{0.18 \mathrm{~K}}{0.512 \mathrm{~K} \mathrm{~kg} \mathrm{~mol}^{-1} \times 1 \mathrm{~mol} \mathrm{~kg}^{-1}}\)
i = 0.35

Question 29.
Define the terms: Osmosis and osmotic pressure. Is the osmotic pressure of a solution a colligative prop- erty? Explain.
Answer:
Osmosis The movement of solvent molecules from less concentrated solution to more concentrated solution through semipermeable membrane is called osmosis. Osmotic pressure is the minimum pressure which needs to be applied to a solution to prevent the inward flow of its pure solvent across a semipermeable membrane.

yes, Osmotic pressure is a colligative property because it depends upon the number of solute particles but not on their nature, e.g. 0.1 molar KCl and NaCl have the same osmotic pressure under same conditions of tem- perature and pressure.

Question 30. 
What is van't Hoff factor? What possible values can it have if the solute molecules undergo dissociation?
Answer:
The Van't Hoff factor can be defined as the ratio of the concentration of particles formed when a substance is dissolved to the concentration of the substance by mass. The extent to which a substance associates or dissociates in a solution is described by the Van't Hoff factor.

In dissociation, observed molar mass has lesser value than normal, so i is more than one (i > 1). e.g. The value of i for ethanoic acid in benzene is nearly 0.5 while for aqueous KCl, NaCl and MgSO₄ solution, it is nearly 2. The value of i for K₂SO₄ is nearly 3. 

Question 31. 
The molecular masses of polymers are determined by osmotic pressure method and not by measuring other colligative properties. Give two reasons.
Answer:
The osmotic pressure method has the advantage over other colligative properties because

1. pressure measurement is around the room tempera- ture and the molarity of the solution is used instead of molality.
2. its magnitude is large as compared to other colli- gative properties even for every dilute solution.

Question 32. 
Find the boiling point of a solution containing 0.520 g of glucose (C₆H₁₂O₆) dissolved in 80.2 g of water. [Given, K, for water = 0.52 Km^-1].
Answer:
Given Kb = 0.52 Km^-1
Mass of solute (W₂) = 0.520 g (glucose)
Mas of solvent (W₁) = 80.20G (water)
Molar mass of solute (M₂) = 180 g mol^-1

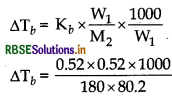
∆Tb = 0.0187 = 0.019
Boiling point Tb = 373 + 0.019 = 373.019 K
Hence b.p = 373.02 K

Question 33. 
Find the freezing point of a solution containing 0.520g glucose (C₆H₁₂O₆) dissolved in 80.2 g of water [Given, K,for water = 1.86 Km^-1]
Answer:
(i) First, find out ∆T,by using the formula
\(\Delta \mathrm{T}_f=\frac{\mathrm{K}_f \times \mathrm{W}_2 \times 1000}{\mathrm{M}_2 \times \mathrm{W}_1}\)

(ii) Then, find out the value of T, by using formula,
∆Tf = Tf - Tf
Given, W₂ (glucose) = 0.520 g
W₁ (H₂O) = 80.2 g
Kf (H₂O) = 1.86 k m^-1
M2 of C₆H₁₂O₆ (glucose) = 180 g mol^-1

= 0.0669 K
Freezing point of solution
Tf = 273 K - 0.0669 k
= 272. 933K

Question 34. 
Outer hard shells of two eggs are removed. One of the egg is placed in pure water and the other is placed in saturated solution of sodium chloride. What will be observed and why?
Answer:
The egg placed in pure water will swell because the concentration of protein is high inside the egg as com- pared to water. Therefore, water diffuses through the semi-permeable membrane of egg and egg swells. On the other hand, the egg placed in sodium chloride solution will shrink due to osmosis, water will move out of the egg membrane, thereby, shrinking the egg. 

Question 35. 
A solution of glucose (molar mass = 180 g mol^-1) in water is labelled as 10% (by mass). What would be the molality and molarity of the solution? (Density of solution = 1.2 g mL^-1).
Answer:m = lOOg
W2 = 10g (solute);
W1 = 90g (solvent)
M2 (glucose) = 180 g mo1^-1
Density of solution = 1.2 g mL^-1
Volume of solution,

= 0.617 M

Question 36. 
The partial pressure of ethane over a saturated solution containin 6.56 x 10^-2 g of ethane is 1 bar. If the solution contains 5.0 x 10^-2 of ethane, then what will be the partial pressure of the gas?
Answer:
According to Henry's law,
\(m=\mathrm{K}_{\mathrm{H}} \times p\)
In Ist case,
6.56 x 10^-2 g = KH x 1 Bar
or
KH = 6.56 x 10^-2 g bar^-1
In II nd case,
5.00 x 10^-2 g = (6.56 x 10^-2 g bar^-1) x p
or
\(p=\frac{5.00 \times 10^{-2} \mathrm{~g}}{6.56 \times 10^{-2} \mathrm{~g} \mathrm{bar}^{-1}}\)
= 0.762 bar

Question 37. 
If N₂ gas is bubbled through water at 293 K, how many millimoles of N₂ gas would dissolve in 1 L of water? Assume that N₂ exerts a partial pressure of
0.987 bar. Given that Henry's law constant for N₂ at 293 K is 76.48 K bar.
Answer:
According to Henry's law,
PN₂ = K_H x xN₂
\(\chi_{\mathrm{N}_2}=\frac{p_{\mathrm{N}_2}}{\mathrm{~K}_{\mathrm{H}}}=\frac{0.987}{.76480}\)
= 1.29 x 10^-5
If n moles of N₂ are present in 1L or 1000 g of water

n = 1.29 x 10^-5 x 55.5 moles 
= 71.595 x 10^-5 moles
= 0.716 m moles

Question 38.
The vapour pressure of pure liquids A and B are 450 mm and 700 mm of Hg respectively at 350 K. Find out the composition of the liquid mixture if total vapour pressure is 600 mm of Hg. Also, find the com- position in the vapour phase.
Answer:
Given
P_A = 450 mm of Hg
P_B = 700 mm of Hg;
PTotal = 600 mm of Hg; X_A =?
According to Raoult's law,
P_A = X_A x P_A
p_B = X_B x P_B
= x_A x P_A + (1 - X_A)P_B
= P_B + (P_A - P_B)P_A
On substituting the given values into the above equa- tion, we get
600 = 700 + (450 - 700)X_A 
100 = 250X_A
=> \(\frac{100}{250}\)
= 0.40
Thus, the composition of the liquid mixture will be
X_A = 0.40
X_B = 1 - 0.40 = 0.60
Calculation of composition in the vapour phase,
P_A = X_A x PA
= 0.40 x 450 mm of Hg 
= 180 mm of Hg
P_B = X_B x P_B
= 0.60 x 700 mm of Hg 
= 420 mm of Hg
Mole fraction of A in the vapour phase
\(=\frac{p_{\mathrm{A}}}{p_{\mathrm{A}}+p_{\mathrm{B}}}=\frac{180}{180+420}\)
Mole fraction of B in vapour phase
= 1 - 0.30 = 0.70

Question 39. 
At 300 K, 30 g of glucose present in a litre of its solution has an osmotic pressure of 4.98 bar. If the os- motic pressure of a glucose solution is 1.52 bar at the same temperature, what would be its concentration? 
Answer:
Given
Mass of glucose (wg) = 30 g
Volume of solution (Vi) = 1000 mL (= 1L) 
Osmotic pressure (initial) (πi) = 4.98 bar 
Osmotic pressure (final) (πf) = 1.52 bar 
Molar mass of glucose (MB) = 180 g mol^-1 
(i.e. of C₆H₁₂O₆)
∴ Osmotic pressure (π) = CRT
(where, C = concentration)
\(\pi=\frac{W_B}{M_B} \frac{\mathrm{RT}}{\mathrm{V}}\)
and mass of glucose is same for both the solutions i.e. for πi and πf
or 4.98 x 1 = 152 x Vf
∴ Vf = 4.98/1.52 = 3.28 L^-1
Therefore concentration of final solution (Cf) is
Ci x vi = Cf x Vf
(where C is intial concentration)

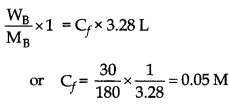
Hence concentration of final solution = 0.05 M

Question 40.
A 40% solution (w/w) of sucrose (M = 342 g mol^-1) in water has a frezing point of 271.15K. Calculate the freezing point of 5% glucose (M = 180 g mol^-1) in water.
(Given freezing point of pure water = 273.15 K)
Answer:
4 % solution (w/w of sucrose is given 
⇒ w₂ = 4g: M₂ = 342 g/ mol and w₁ = 96 g
∴ Molality of surcose
\(=\frac{w_2 \times 1000}{M_2 \times w_1}=\frac{4 \times 1000}{342 \times 96}\)

= 0.122 mol Kg-1 or 0.122 m
∆Tf = Tf - tF
= 273.15 - 271.15
=2K
∆Tf = Kf x m ⇒ Kf = \(\frac{\Delta \mathrm{T}_f}{m}=\frac{2}{0.122}\)
Now, molality of glucose solutionGiven, w₂ = 5g; M₂ = 180 g/mol; w₁ = 95 g
∴ Molality of glucose = \(\frac{w_2 \times 1000}{\mathrm{M}_2 \times w_1}=\frac{5}{180} \times \frac{1000}{95}\)
= 0.292 m
∴ ∆Tf(glucose) = kf  x M_{2 
\(=\frac{2}{0.122} \times 0.292=4.8\)}
∴ freezing point of glucose solution
= 273.15 - 4.8
= 268.35 K

Question 41.
Calculate the freezing point of an aqueous solution containing 10.5 g of magnesium bromide in 200 g of water, assuming complete dissociation of magnesium bromide.(Molar mass of magnesium bromide = 184 g mol^-1, Kf for water = 1.86 K kg mol^-1)
Answer:
Apply van,t Hoff equation, ∆Tf = iKfm, 
where i = number of ions produced by MgBr₂.
Given, W₂ = 10.50g,
W₁ = 200g
M₂(MgBr₂) = 184 g mol^-1
Kf = 1.86 K kg mol^-1
MgBr₂(aq) → Mg²⁺(aq) + 2Br^-(aq)
i = 3
∆Tf = iKfm

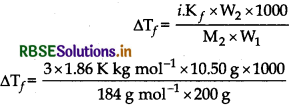
= 1.592 K
Freezing point of solution= 273 K - 1.592 K
Tf = 271.408 K₂SO₄

Question 42.
A 10% solution (by mass) of sucrose in water has freezing point of 269.15 K. Calculate the freezing point of 10% glucose in water, if frezing point of pure water is 273.15 K.
[Given : molar mass of sucrose = 342 g mol^-1 and molar mass of glucose = 180 g mol^-1]
Answer:
First, calculate the value of molality(m) of sucrose, using m = \(\frac{\mathrm{W}_{\text {solute }} \times 1000}{\mathrm{M}_{\text {solute }} \times \mathrm{W}_{\text {solvent }}}\)
then, calculate the cryoscopic constant (Kf) by using depression in freezing point, ∆Tf = Kfm. Finally calculate the ∆Tf of glucose solution followed by freezing point of glucose solution Tf = 273.15 - ∆Tf.
For sucrose solution,
10% solution by mass means 10g of sucrose disolved in 90g of water.
Molality of sucrose solution = \(\frac{W_2 \times 1000}{M_2 \times W_1}\)
Given W₂ = 10 g
W₁ = 90 g
M₂ = 342 g mol^-1
Molality (m) = \(\frac{10}{342} \times \frac{1000}{90}\)
= 0.324 mol kg^-1
∆Tf for sucrose solution= 273.15 K - 269.15 K
= 4K
∆Tf = Kfm
Kf = imm
For glucose solution
W2 = 10g, W1 = 90g,
M2 = 180 g mol-1
Molality (m) = \(\frac{4}{0.324}\)
= 0.617 mol kg^-1
∆Tf = Kfm
∴ ∆Tf(glucose) = \(\frac{4}{0.324} \times 0.617\)
= 7.617 k
Hence, feezing point of glucose solution
= 273.15 K - 7.617 K
= 265.53 k

Question 43.
Calculate the boiling point of solution when 4g of MgSO₄ (M = 120 g mol^-1) was dissolved in 100g of water, if freezing point of pure water is 273.
Answer:
Given weight of solute,
W₂ = 4g
Weight of solvent W₁ = 100g
i = 2 (as MgSO₄ dissociates completely into 2 ions)

Kb = 0.52 K kg mol^-1
M₂ = 120 g mol^-1
∴The elevation in boiling point of solution

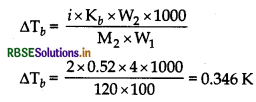
Boiling point of pure water is 100° C or 373 K.
Tb = ∆Tb + Tb
∴ Tb = 373 + 0.376
= 373.346 K

Question 44. 
Calculate the mass of NaCl (molar mass = 58.5 g mol^-1) to be dissolved in 37.2 g of water to lower the freez- ing point by 2° C, assuming that NaCl undergoes complete dissociation. (K, for water = 1.86 K kg mol^-1)
Or
What mass of NaCl must be dissolved in 65.0 g of water to lower the freezing point of water by 7.50°C? The freezing point depression constant (K) for water is 1,86°C/m. Assume van't Hoff factor for NaCl is 1.87. (Molar mass of NaCl = 58.5 g ).
Answer:
(i) Apply the formula,
\(\Delta \mathrm{T}_f=\frac{i \mathrm{~K}_f \times \mathrm{W}_2 \times 1000}{\mathrm{M}_2 \times \mathrm{W}_1}\) and find W₂.

(ii) Since, NaCl and KCl are strong electrolytes,
∴ a = 1 and i = 2 (if not given in question)
Given,
W₁ = 37.2 g, ∆Tf = 2°C = 2K
∵ ∆Tf = Tf  - T°f
∆Tf = 2°C - 0°C = 2°C
or 
∆Tf =275K - 273K = 2K 
Kf = 1.86 K log mol^-1,i = 2

[for complete dissociation]
M₂(NaCl) = 23 + 35.5 = 58.5 g mol^-1

W₂ = 1.17 g
Refer to the above method to obtain the weight of NaCl. 

Question 45. 
45 g of ethylene glycol (C₂H₆O₂) is mixed with 600 g of water. Calculate
(i) the freezing point depression and 
(ii) the freezing point of the solution. (Given, K, of water = 1.86 K kg mol^-1)
Answer:
(i) Apply the formula, \(\Delta \mathrm{T}_f=\frac{\mathrm{K}_f \times \mathrm{W}_2 \times 1000}{\mathrm{M}_2 \times \mathrm{W}_1}\)
calculate ∆Tf

(ii) ∆T = Tf - Tf, from then calculate Tf
Given,
W1 = 600 g, K = 1.86 K kg mol-1,
W2 = 45 g, M2 = 62.0 g mol-1,
Tf (water)=273 K
(i)

∆Tf = 2.25 K

(ii)  ∆Tf = Tf  - Tf
Tf = 273 K - 2.25K = 270.75 K

Question 46. 
A 5% solution (by mass) of cane sugar (M. W 342 g mol^-1) is isotonic with 0.877% solution of substance X. Find the molecular weight of X.
Answer:
Isotonic solutions have same osmotic pressure at a given temperature.
Given,
W(cane sugar) = 5g 
W(X) = 0.8778
M(cane sugar) = 342 g mol^-1
(cane sugar) = π(X)
[ ∵ solution is isotonic]

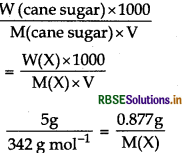
M(X) = 59.9 g mol^-1

Question 47. 
3.9 g of benzoic acid dissolved in 49 g of benzene shows a depression in freezing point of 1.62 K. Calculate the van't Hoff factor and predict the nature of solute (associated or dissociated). (Given: Molar mass of benzoic acid = 122 g mol^-1, Kf for benzene = 4.9K kg mol^-1)
Answer:
Calculate 'i' using formula
∆Tf = \(\frac{i \mathrm{~K}_1 \mathrm{~W}_2 \times 1000}{\mathrm{M}_2 \times \mathrm{W}_1}\)
Given, W₂ = 3.9 g, W₁ = 49 g; ∆T = 1.62 K M₂ (benzoic acid) = 122 g mol^-1
Kf(benzene) = 4.9K kg mol^-1

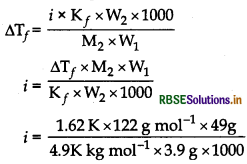
i = 0.50
Since, i < 1, therefore solute (benzoic acid)
undergoes association.

Question 48. 
A solution is prepared by dissolving 10 g of non - volatile solute in 200 g of water. It has a vapour pressure of 31.84 mm of Hg at 308 K. Calculate the molar mass of the solute. (Vapour pressure of pure water at 308 K = 32 mm Hg)
Answer:
Apply formula,
\(\frac{p^{\circ}-p}{p^{\circ}}=\frac{n_2}{n_1}\)
Given,
W₂ = 10g, 
W₁ = 200 g, 
p = 31.84 mm of Hg 
p° = 32 mm of Hg; 
M₁ = 18 g mol^-1

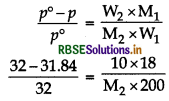
M₂ = 180 g mol^-1.

Question 49. 
Calculate the boiling point elevation for a solution prepared by adding 10 g of CalCl₂ to 200 g of water. (Kb for water = 0.512K kg mol^-1, molar mass of CaCl₂ = 111 g mol^-1).
Answer:
Mass of CaCl₂ (W₂) = 10 g 
Ma of water (W₁) = 200 g
Kb = 0.512K kg mol^-1
i for CaCl₂ = 3

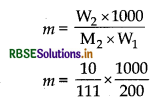
m = 0.450 kg mol^-1,
∆Tb = iKb x m
= 3 x 0.512 K kg mol^-1 x 0.450 m = 0.6912 K

Question 50. 
Some ethylene glycol, HOCH₂CH₂OH, is added to your car's cooling system along with 5 kg of water. If the freezing point of water-glycol solution is -15.0°C, what is the boiling point of the solution?
(Kb = 0.52 K kg mol^-1 and Kf = 1.86 K kg mol^-1 for water)
Answer:
(i) First, find out m by using, ∆Tf = Km.
(ii) Then, find out ∆Tb by using, ∆Tb = Km. 
(iii) Then, find out the value of T, by using formula, Tb = ∆Tb + Tb
Given,
∆Tf = Tf - Tf
= 0 - (-15.0°C) = 15°C
∆Tf = 15.0°C
Kf = 1.86K/m = 1.86°C/m

Molality (m) of HOCH₂CH₂OH = \(\frac{\Delta \mathrm{T}_f}{\mathrm{~K}_f}\)
\(\frac{15.0^{\circ} \mathrm{C}}{1.86^{\circ} \mathrm{C} / \mathrm{m}}\) = 8.06 m
∆Tb = Kb x molality (m) of HOCH₂CH₂OH
= 0.52°C/m x 8.06 m = 4.19°C

Boiling point of pure water is 100°C.
Tb = ∆Tb + Tb
Tb = 4.19°C + 100.00°C
= 104.19°C

Question 51. 
Determine the osmotic pressure of a solution pre- pared by dissolving 2.5 x 10^-2 g of K₂SO₄ in 2L of water at 25°C, assuming that it is completely dissoci- ated. (R = 0.0821 L atm K^-1 mol^-1, molar mass of K₂SO₄ = 174 kg mol^-1).
Answer:
Apply 
\(i \frac{W_b R T}{M_b \mathrm{~V}}\)
to find out the value of л.
Given,mass of K₂SO₄, W_b = 2.5 × 10^-2g
Molar mass of K₂SO₄, Mb = 174 g mol^-1
i = n = 3
(for complete dissociation, i = n) V = 2L, T = 25°C = 298 K R = 0.0821 L atm K^-1 mol^-1
We know, osmotic pressure

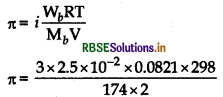
π = 52.7 x 10 atm

Question 52. 
1.00 g of a non - electrolyte solute when dissolved in 50 g of benzene lowered the freezing point of benzene by 0.40 K. Find the molar mass of the solute. (K for benzene = 5.12K kg mol^-1).
Answer: 
Given,
W₂ = 1.00 g, 
W₁ = 50 g, 
Kf = 5.12K kg mol^-1

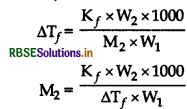
=256 g mol^-1

Question 53. 
A 5% solution (by mass) of cane-sugar in water has freezing point of 271 K. Calculate the freezing point of 5% solution (by mass) of glucose in water if the freezing point of pure water is 273.15 K.
[Molecular masses glucose (C₆H₁₂O₆) = 180 amu org mol-1 and cane-sugar (C₁₂H₂₂O₁₁) 342 amu or g mol^-1]
Answer:
Freezing point of pure water is 273.15 K (0°C) and that of given solution is 271 K; i.e. we have depression in freezing point. So,
(i) apply the formula of depression in freezing point for cane sugar and find the value of K
(ii) use this value of Ky to find out the value of AT, for glucose solution. Then find the freezing point of solution.
Given, 5% cane-sugar solution meant,
W₂ = 5g W₁ = 95g M₂ = 342 g mol^-1
Similarly, for 5% glucose solution,
W₂ (glucose) = 5g; W₁ = 95g M₂ (glucose) 180g mol^-1
∆Tf (cane sugar) = 273.15K - 271K = 2.15K
∆Tf(cane sugar) = \(\frac{\mathrm{K}_f \times \mathrm{W}_2 \times 1000}{\mathrm{M}_2 \mathrm{~W}_1}\) \(\)
\(\begin{aligned} 2.15 &=\frac{\mathrm{K}_f \times 5 \times 1000}{342 \times 95} \\ \mathrm{~K}_f &=\frac{2.15 \times 342 \times 95}{5 \times 1000} \end{aligned}\)
= 13.9707

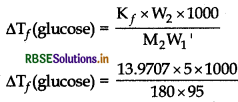
= 4.085 K
∴ Freezing point of the solution
= 273.15 K - 4.085 K
= 269.065 K

Question 54. 
At 25°C, the saturated vapour pressure of water is 3.165 kPa (23.75 mm Hg). Find the saturated vapour pressure of a 5% aqueous solution of urea (carbam- ide) at the same temperature. (Molar mass of urea = 60.05 g mol^-1).
Answer:
Apply formula, 
\(\begin{aligned} &\frac{p^{\circ}-p}{p^{\circ}}=\frac{n_2}{n_1} \\ &\frac{p^{\circ}-p}{p^{\circ}}=\chi_2=\frac{n_2}{n_1} \end{aligned}\)
[for a dilute solution, n₂ << n₁]
\(\frac{p^{\circ}-p}{p^{\circ}}=\frac{W_2 \times \mathrm{M}_1}{\mathrm{M}_2 \times \mathrm{W}_1}\)
Given,
p° = 3.165 kPa. 
W₂ = 5g, 
W₁ = 95g
M₂ = 60.05g mol^-1 
M₁ = 18g mol^-1
\(\frac{3.165-p}{3.165}=\frac{5 \times 18}{60.05 \times 95}\)
= 0.0158
p = 3.115k Pa

Question 55. 
15.0 g of unknown molecular materials is dissolved in 450 g of water. The resulting solution freezes at - 0.34°C. What is the molar mass of the material? (K, for water = 1.86 K kg mol^-1)
Answer:
Given,
mass of solute (W2) = 150 g
Mass of solvent
(water) (W1) = 450 g
∆Tf = 0°C - (-0.34°C)
= 0.34°C = 0.34K
Kf = 1.86 K kg mol-1
To find molar mass of solute (M2)=?

= 182.35 g mol^-1

Question 56. 
A solution of glycerol (C₃H₂O₃) in water was pre- pared by dissolving some glycerol in 500 g of water. This solution has a boiling point of 100.42°C, what mass of glycerol was dissolved to make this solution?
(K, for water = 0.512 K kg mol^-1)
Answer:
Given, Mass of solute (glycerol) (W₂) = ? 
Molar mass of solute (M₂) = 92 mol^-1 
Mass of solvent (water) (W) = 500g.
∆Tb = 100.42°C - 100°C
= 0.42°C = 0.42 K 
Kb = 0.512K kg mol^-1
∵ ∆Tb = Kb x \(\frac{W_2}{M_2} \times \frac{1000}{W_1}\)
\(W_2=\frac{0.42 \times 92 \times 500}{0.512 \times 1000}\)
W₂ = 37.738
Hence, mass of glycerol dissolved = 37.73g

Question 57. 
Calculate the boiling point of a solution prepared by adding 15.00 g of NaCl to 250.00 g of water. (K, for water = 0.512 K kg mol¹, molar mass of NaCl = 58.44 gmol^-1).
Answer:
Apply van't Hoff equation, ∆Tb = iKb m, 
where i = number of ions produced by NaCl.
Given,
W₂ = 15.00 g,
W₁ = 250.0g
M₂ = 58.44 g mol^-1,
K_b = 0.512K kg mol^-1
NaCl(aq) → Na⁺ (aq) + Cl⁻ (aq)
i = 2
∆Tb = iKb.m
\(\Delta \mathrm{T}_b=\frac{i \times \mathrm{K}_b \times \mathrm{W}_2 \times 1000}{\mathrm{M}_2 \times \mathrm{W}_1}\)
\(\Delta \mathrm{T}_b^{\prime}=\frac{2 \times 0.512 \mathrm{~K} \mathrm{~kg} \mathrm{~mol}^{-1} \times 15.0 \mathrm{~g} \times 1000}{58.44 \mathrm{~g} \mathrm{~mol}^{-1} \times 250 \mathrm{~g}}\)
Boiling point of solution
= 373 K + 1.051 K
= 374.051 K

Question 58. 
What would be the molar mass of a compound if 6.21 g of it is dissolved in 24.0 g of chloroform form a solution that has a boiling point of 68.04°C. The boil- ing point of pure chloroform is 61.7°C and the boiling point elevation constant, K, for chloroform is 3.63% C/m.
Answer:
Given,
W₂ = 6.21g, W₁ = 24.0g Tb = 68.04°C
Tb = 61.7°C
Kb = 3.63°C/m;

∆Tb = Tb - Tb
= 68.04°C - 61.7°C = 6.34°C
\(\mathrm{M}_2=\frac{3.63^{\circ} \mathrm{C} \mathrm{m}^{-1} \times 6.21 \mathrm{~g} \times 1000}{6.34^{\circ} \mathrm{C} \times 24.0 \mathrm{~g}}\)
= 148.15 g mol^-1

Question 59. 
A solution prepared by dissolving 8.95 mg of a gene fragment in 35.0 mL of water has an osmotic pres- sure of 0.335 torr at 25°C. Assuming the gene frag- ment is non-electrolyte, determine its molar mass. W₂ = 8.95 mg 8.95 × 10^-3 g
Answer:
Given
W₂ = 8.95 mg = 8.95 x 10^-3 g,
V = 35.0 Ml = \(\frac{35}{1000} \mathrm{~L}\)
 π = 0.335 torr =\(\frac{0.335}{760} \mathrm{~atm}\) (∵ 1 atm = 760 torr)
 T= 273 + 25 = 298 K
 πV = nRT or πV = \(\frac{\mathrm{W}_2 \mathrm{RT}}{\mathrm{M}_2}\)
 ⇒ \(\mathrm{M}_2=\frac{\mathrm{W}_2 \mathrm{RT}}{\pi \mathrm{V}}\)
\(=\frac{8.95 \times 10^{-3} \mathrm{~g} \times 0.082 \mathrm{~L} \mathrm{~atm} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \times 298 \mathrm{~K}}{\frac{0.335}{760} \mathrm{~atm} \times \frac{35.0}{1000} \mathrm{~L}}\)
M₂ = 14174 g mol^-1
M₂ = 1.4174 x 104 g mol^-1

Question 60. 
A 0.561 m solution of unknown electrolyte depresses the freezing point of water by 2.93°C. What is van't Hoff factor for this electrolyte? The freezing point depression constant (K) for water is 1.86°C kg mol^-1. m = 0.561, ∆Tf = 2.93°C and Kf = 1.86°C kg mol^-1 ∆Tf = iKfm
Answer:
Given,
m = 0.561, ∆Tf = 2.93°C and
Kf = 1.86°C kg mol^-1
\(i=\frac{\Delta \mathrm{T}_f}{\mathrm{~K}_f m}\)
= 2.807

Question 61. 
Phenol associates in benzene to a certain extent to form a dimer. A solution containing 20 g of phenol in 1.0 kg of benzene has its freezing point lowered by 0.69 K. Calculate the fraction of phenol that has dimerised.
(Given, K, for benzene = 5.1 K kg mol^-1).
Answer:
(1) Find calculate M₂ (observed) by using the formula,
\(\mathrm{M}_2=\frac{\mathrm{K}_f \times \mathrm{W}_2 \times 1000}{\Delta \mathrm{T}_f \cdot \mathrm{W}_1}\)

(ii) Calculate 'i' by using the formula,
\(i=\frac{\mathrm{M}_2 \text { (Calculate) }}{\mathrm{M}_2 \text { (Observed) }}\)

(iii) Calculate a by using the formula,
\(\alpha=\frac{i-1}{\left(\frac{1}{n}-1\right)}\)

Here, n = 2 because phenol forms dimer on association.
Given,
W₂ = 20g, W₁ = 1 kg = 1000 g ∆Tf = 0.69 K, Kf =  5.1K kg mol^-1 

M₂ (Observed) = 147.82 g mol^-1
M₂ (Calculated) C₆H₅OH = 6 × 12 + 6 x 1 + 16
= 94 g mol^-1

Question 62. 
An aqueous solution containing 12.48 g of barium chloride in 1.0 kg of water boils at 373.0832 K. Calculate the degree of dissociation of barium chloride.
(Given, Kb for H₂O = 0.52 K kg mol^-1, molar mass of BaCl₂ = 208.34 g mol^-1)
Answer:
(i) First calculate M₂ (observed) by using the formula,
\(\mathrm{M}_2=\frac{\mathrm{K}_b \times \mathrm{W}_2 \times 1000}{\Delta \mathrm{T}_b \times \mathrm{W}_1}\)

(iii) Calculate i by using the formula,
\(i=\frac{\mathrm{M}_2 \text { (Calculated) }}{\mathrm{M}_2 \text { (Observed) }}\)

(iii) Calculate a by using the formula, α = \(\frac{i-1}{m-1}\)
Given,
and
W₂ = 12.48 g,
W₁ = 1.0 kg = 1000g
Tb (solution) = 373.0832 K 
Kb for H₂O = 0.52 Km^-1 
M₂(BaCl₂) = 208.34 g mol^-1
∆Tb = Tb - Tb
= 373.0832 K - 373 K
= 0.0832 K
M₂ (Observed) = \(\frac{\mathrm{K}_b \times \mathrm{W}_2 \times 1000}{\Delta \mathrm{T}_{\mathrm{b}} \times \mathrm{W}_1}\)
\(=\frac{0.52 \mathrm{Km}^{-1} \times 12.48 \mathrm{~g} \times 1000}{0.0832 \mathrm{~K} \times 1000 \mathrm{~g}}\)
M₂ (Observed) = 78 g mol^-1

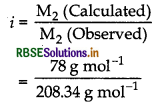
= 0.374
For BaCl₂, m = 3 as it gives 3 ions on dissociation.

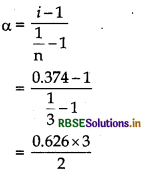
α = 93.9%

Question 63. 
At 300 K, 36 g of glucose, C₆H₁₂O₆ present per litre in its solution has an osmotic pressure of 4.98 bar. If the osmotic pressure of another glucose solution is 1.52 bar at the same temperature, calculate the con- centration of the other solution.
Answer:
Apply π = CRT and

For both solution, R, T and V are constant.
For first solution,
\((4: 98 \mathrm{bar})=\frac{(36 \mathrm{~g}) \times \mathrm{R} \times \mathrm{T}}{\left(180 \mathrm{~g} \mathrm{~mol} \mathrm{~mol}^{-1}\right) \times \mathrm{V}}\) ... (i)
For second solution,
\((1.52 \mathrm{bar})=\frac{\mathrm{W}_2 \times \mathrm{R} \times \mathrm{T}}{\mathrm{M}_2 \times \mathrm{V}}\)
On dividing Eq. (ii) by Eq. (i), we get

= 0.0610 mol

Question 64. 
Calculate the boiling point of one molar aqueous solution. Density of KBr solution is 1.06 gmL^-1 (Kb for H₂O = 0.52 kg mol¹, atomic mass of K = 39, Br = 80).
Answer:
(i) Calculate the molality of solution by using the formula.
\(m=\frac{\mathrm{M} \times 1000}{(1000 \times d)-\left(\mathrm{M} \times \mathrm{M}_2\right)}\)
(where, M = molarity, d = density and M₂ = molar mass of solute)

(ii) Calculate ∆T, by using the formula, ∆T_b = iKbn 
(iii) Calculate T, (boiling point of solution) by using
the formula, T_b + ∆T_B = T_B
Given, concentration of the solution 1 molar Density of the solution = 1.06 g mL^-1
M2, molar mass of KBr = 39 + 80 = 119 g mol^-1 
KB for H₂O = 0.52 K kg mol^-1
KBr → K⁺ + Br^-
i.e. ions produced = 2
∴ i  = 2
Molality,

= 1.0626 mol kg1
∆T_b = iKbm
= 2 × 0.52 K kg mol^-1 x 1.0626 mol kg^-1
∆T_b = 1.105 K
T_b = 373 K + 1.105 K = 374.105 K

Question 65. 
A solution prepared by dissolving 1.25 g of oil of win- tergreen (methyl salicylate) in 99.0 g of benzene has a boiling point of 80.31°C. Determine the molar mass of this compound. (Boiling point of pure benzene = 80.10°C and K, for benzene = 2.53°C kg mol^-1)
Answer:
Given, W₂ = 1.25 g
W₁ = 99.0 g
K_b = 2.53°C kg mol^-1
∆T_b = T_b - T_b = 80.31 - 80.10
= 0.21°C
Using the formula,

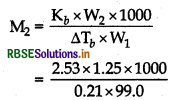
M₂ = 152 g mol^-1 approx.

Question 66. 
What mass of ethylene glycol (molar mass 62.0 g mol^-1) must be added to 5.50 kg of water to lower the freezing point of water from 0°C to - 10.0°C?
(K for water = 1.86 K kg mol^-1)
Answer:
Given, M₂ (ethylene glycol) = 62 g mol^-1. 
W₁ = 5.50 kg = 5500 g, 
∆T_f = 10 K [0°C- (-10°C) = 10°C = 10K]
and 
K_f = 1.86 K kg mol^-1

W₂ = 1833.33 g = 1.833 kg

Question 67. 
0.1 mole of acetic acid was dissolved in 1 kg of benzene. Depression in freezing point of benzene was determined to be 0.256 K. What conclusion can you draw about the state of the solute in solution? (Given, K, for benzene = 5.12 K kg mol^-1)
Answer:
(i) First find M₂ (observed) by using the formula,
\(\mathrm{M}_2=\frac{\mathrm{K}_f \times \mathrm{W}_2 \times 1000}{\Delta \mathrm{T}_f \times \mathrm{W}_1}\)

(ii) Compare it with calculated M₂ to know whether it is associated or dissociated.
Given, W₁ = 1kg = 1000 g (benzene)
∆T_f = 0.256 K, K_f =  5.12 K kg mol^-1
0.1 mol of acetic acid = 0.1 x 60 = 6.08 (W₂) 
[ ∵ Molar mass of CH₃COOH = 60 g mol^-1]

The value of i is 0.5. It is less than 1. Hence, the solute, i.e. CH₃COOH gets dimerise in benzene.

Question 68. 
Calculate the mass of ascorbic acid (C₆H₈O₆) to be dissolved in 75 g of acetic acid to lower its melting point by 1.5°C (K_f for acetic acid is 3.9K kg mol^-1) 
Answer:
Lowering in melting point (∆Tf) = 1.5°C
Mass of solvent (CH₃COOH), W₁ = 75g
Molar mass of solvent (CH₃COOH),
M₁ = 60 g mol^-1
[as CH₃COOH = 12 + 3 + 12 + 16 + 16 + 1 = 60 g mol^-1] 
Molar mass of solute (C₆H₈O₆),
M₂ = 72 + 8 + 96 = 176 g mol^-1
[as, CH₂O₆ = 6 x 12 + 8 x 1 + 6 x 16 = 176]
For acetic acid, K = 3.9K kg mol^-1
Applying the formula,

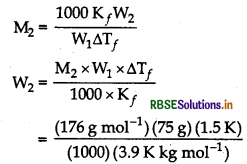
= 5.077 g

Long Answer Questions:

Question 1. 
(i) Calculate the freezing point of solution when 1.9g of MgCl₂ (M = 95 g mol^-1) was dissolved in 50 g of water, assuming MgCl₂ undergoes complete ionisatin.
(Kf for water = 1.86 K kg mol^-1)
(ii) (a) Out of 1 M glucose and 2 M glucose, which one has a higher boiling point and why?
(b) What happens when the external pressure applied becomes more than the osmotic pressure of the solution?
Answer:
(i) MgCl₂ → Mg²⁺ + 2Cl^-
1 mol of MgCl₂ gives 3 moles of particles.
Given,
i = 3
∆Tf = iKfm
WA = Weight of H₂O (solvent) = 50 g
WB = Weight of MgCl₂ (solute) = 1.9 g
T = 273.15 K
K =  1.86K kg mol^-1
MB = Molar mass of solute 
= 95 g mol^-1
\(\Delta \mathrm{T}_f=\frac{i \mathrm{~K}_f \times 1000 \times \mathrm{W}_b}{\mathrm{M}_{\mathrm{B}} \times \mathrm{W}_a}\)

\(=\frac{3 \times 1.86 \mathrm{~K} \mathrm{~kg} \mathrm{~mol}^{-1} \times 1000 \times 1.9 \mathrm{~g}}{95 \mathrm{~g} \mathrm{~mol}^{-1} \times 50 \mathrm{~g}}\)
∆Tf = 2.232 K
Also,
∆Tf = Tf - Tf
Tf = Tf - ∆T
= 273.15 - 2.232
= 270.918 K

(ii) (a) 2M glucose has higher boiling point because more the concentration, more is the elevation in boiling point.
(b) When the external pressure applied becomes more than the osmotic pressure of solution, reverse osmosis takes place.

Question 2. 
(i) When 2.56 g of sulphur was dissolved in 100 g of CS₂, the freezing point gets lowered by 0.383 K. Calculate the formula of sulphur (S_x).
(K, for CS₂ = 3.83 K kg mol¹, Atomic mass of sulphur = 32 g mol^-1)
(ii) Blood cells are isotonic with 0.9% sodium chloride solution. What happens if we place blood cells in a solution containing.
(i) 1.2% sodium chloride solution?
(ii) 0.4% sodium chloride solution?
Answer:
(i) Given, Weight of solvent (WA) = 100 g
Weight of solute (WB) = 2.56 g
∆Tf = 0.383 K
Kf = 3.83 K kg mol-1 
∆Tf = Kf x m

M = 256 g/mol
Molecular Mass = n x Atomic mass
∴ Formula of sulphur is Sg.

(ii) (a) If we place the blood cells in a solution contain- ing more than 0.9% (mass/volume) sodium chloride solution, water will flow out of the cells and they would shrink. This process is called plasmolysis.
(b) If the salt concentration is less than 0.9% (mass/ volume), then the water will flow into the cells and they would swell.

Question 3. 
On dissolving 3.24 g of sulphur in 40 g of benzene, boiling point of solution was higher than that of benzene by 0.81 K. K, value for benzene is 2.53 K kg mol^-1. What is the molecular formula of sulphur? (Atomic mass of sulphur 32 g mol^-1). '
Answer:
Molecular mass of sulphur,
\(\mathrm{M}_{\mathrm{B}}=\frac{\mathrm{K}_b \times \mathrm{W}_{\mathrm{B}} \times 1000}{\mathrm{~W}_{\mathrm{A}} \times \Delta \mathrm{T}_b}\)
Kb = 253 K kg mol^-1, 
WB = 324 g 
WA = 408 
∆Tb = 0.81 K
\(\mathrm{M}_{\mathrm{B}}=\frac{2.53 \times 3.24 \times 1000}{40 \times 0.81}\)
= 253 g mol^-1
Let the molecular formula of sulphur = Sx 
Atomic mass of sulphur = 32
Molecular mass = 32 x x
253 = 32x
or
\(x=\frac{253}{32}\)
= 7.91 = 8
Molecular formula of sulphur = Sg.

Question 4. 
In winter, the normal temperature in a Himalayan's valley was found to be - 10°C. Is a 30% by mass of aqueous solution of ethylene glycol (molar mass = 62) suitable for car radiator? (K, for water = 1.86K/m)
Answer:
\(\Delta \mathrm{T}_f=\frac{\mathrm{K}_f \times \mathrm{W}_{\mathrm{B}} \times 1000}{\mathrm{M}_{\mathrm{B}} \times \mathrm{W}_{\mathrm{A}}}\)
Kf = 1.86K/m, WB = 30g, MB = 62 
WA = 100 - 30 = 70 g
\(\Delta \mathrm{T}_f=\frac{1.86 \times 30 \times 1000}{62 \times 70}\)
= 12 86 K or 12.86°C
Tf = Tf - ∆T
= 0°C - 12 86°C  = -12 86°C
It is suitable because water freezes at -12.86°C. 

Question 5. 
Two aqueous solutions containing respectively 7.5 gurea (molar mass = 60) and 42.75 g substance X in 100 g of water freeze at the same temperature. Cal- culate the molecular weight of X.
Answer:
\(\Delta \mathrm{T}_f=\frac{\mathrm{K}_f \times \mathrm{W}_{\mathrm{B}} \times 1000}{\mathrm{M}_{\mathrm{B}} \times \mathrm{W}_{\mathrm{A}}}\)
Both the solutions freeze at the same temperature. Therefore, ∆T, (urea) = ∆Tf(X).
For urea: WB = 75g, MB = 60 g mol^-1, WA = 100 g 
For substance: WB = 42.75 g, MB = ? WA = 100g

= 342 g mol^-1

Question 6. 
A solution of an organic compound is prepared by dissolving 34.2g in 500 g of water. Calculate the molecular mass of the compound and freezing point of the solution. Given that Kb for water = 0.52 K m^-1, boiling point of solution = 100.14°C, K, for water = 1.87 Km^-1.
Answer:
∆Tb = Tb - Tb
= 100.14°C - 100°C = 0·14°C or 0.14K
\(\mathrm{M}_{\mathrm{B}}=\frac{\mathrm{K}_b \times \mathrm{W}_{\mathrm{B}} \times 1000}{\Delta \mathrm{T}_b \times \mathrm{W}_{\mathrm{A}}}\)
Kb = 0.52 Km^-1, WB = 34.2g WA = 500 g, ∆Tb = 0.14 K
\(M_B=\frac{0.52 \times 34.2 \times 1000}{0.14 \times 500}\)
= 342 g mol^-1
\(\Delta \mathrm{T}_f=\frac{\mathrm{K}_f \times \mathrm{W}_{\mathrm{B}} \times 1000}{\mathrm{M}_{\mathrm{B}} \times \mathrm{W}_{\mathrm{A}}}\)
Kf = 187 Km^-1, WB = 34.2 g, MB = 342 g mol^-1
WA = 500 g
\(\Delta \mathrm{T}_f=\frac{1.87 \times 34.2 \times 1000}{342 \times 500}\)
= 0.374 K or 0 374 °C
Tf = Tf - ∆T
= 0°C - 0374°C  = 0.374°C.

Question 7.
Osmotic pressure of a solution containing 2 g dis- solved protein per 300 cm³ of solution is 20 mm of Hg at 27°C. Calculate the molecular mass of protein (R = 0.0821 L atm K^-1 mol^-1).
Answer:
\(\mathrm{M}_{\mathrm{B}}=\frac{\mathrm{W}_{\mathrm{B}} \times \mathrm{R} \times \mathrm{T}}{\pi \times \mathrm{V}}\)
π  = 20 mm Hg = \(\frac{20}{760}\) atm
T = 27°C = 27 + 273 = 300 K
WB = 2g
\(\frac{300}{1000}\)L
R = 00821 L atm K^-1 mol^-1 
\(\begin{aligned} \mathrm{M}_{\mathrm{B}} &=\frac{2 \times 0.0821 \times 300}{\frac{20}{760} \times \frac{300}{1000}} \\ &=\frac{2 \times 0.0821 \times 300 \times 760 \times 1000}{20 \times 300} \end{aligned}\)
= 6239.6 g mol^-1

Question 8. 
The osmotic pressure of blood is 8.21 atm at 37°C. How much glucose should be used per litre for an intravenous injection that is isotonic with blood?
Answer:
\(\pi=\frac{W_B \times R \times T}{M_B \times V}\)
π = 821 atm, W = ?, R = 0.0821 L atm K^-1 mol^-1 
V = 1 L, MB = 180 g mol¹, T = 37 + 273 = 310 K
\(\begin{aligned} 8.21 &=\frac{W_B \times 0.0821 \times 310}{180 \times 1} \\ W_B &=\frac{8.21 \times 180}{0.0821 \times 310} \\ &=\frac{1477 \cdot 8}{25.451}=58.06 \mathrm{~g} \end{aligned}\)

Question 9. 
What osmotic pressure would 1.25 molal sucrose solution exhibit at 25°C? The density of the solution is 1.34 g/mL.
Answer:
1.25 molal solution means that 1 25 moles of solute are present in 1000 g of solvent.
Mass of solvent 1000 g
Molar mass of solute (Sucrose) = 342 g mol^-1
Mass of solute = 125 × 342 = 427 5 g
Mass of solution = 1000 + 427 5 = 1427 5 g
Mass
Volume of solution = \(\frac{\text { Mass }}{\text { Density }}=\frac{1427.5}{1.34}\)
= 1065 3 mL
Molarity of solution (M)
\(=\frac{\text { Moles of solute }}{\text { Volume of solution in } \mathrm{mL}} \times 1000\)
= \(\frac{125}{10653}\) × 1000 = 1.173 mol L^-1
π = CRT
C = 1·173 mol L^-1,
R = 0.0821 Latm K^-1 mol^-1
T = 25°C = 25 + 273 = 298 K
л = 1 - 173 × 0 0821 × 298 = 28.7 atm

Question 10. 
1.5 g of Ba(NO₃)₂ dissolved in 100 g of water shows a depression in freezing point equal to 0.28°C. What is the percentage dissociation of the salt (Kb for water = 1.86 K/m and molar mass of Ba(NO₃)₂ = 261).
Answer:
Observed molar mass can be calculated as
\(\mathrm{M}_{\mathrm{B}}=\frac{\mathrm{K}_f \times \mathrm{W}_{\mathrm{B}} \times 1000}{\Delta \mathrm{T}_f \times \mathrm{W}_{\mathrm{A}}}\)
Kf  = 186 Km-1, WB = 15g WA = 100 g 
∆Tf = 0.28°C or 0.28 K
\(\mathrm{M}_{\mathrm{B}}=\frac{1.86 \times 1.5 \times 1000}{0.28 \times 100}\)

Normal molar mass = 261
\(\begin{aligned} i &=\frac{\text { Normal molar mass }}{\text { Observed molar mass }} \\ &=\frac{261}{99 \cdot 64}=262 \end{aligned}\)
Ba(NO₃)₂ dissociates as

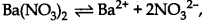
n = 2 + 1 = 3
\(\begin{aligned} \alpha &=\frac{i-1}{n-1} \\ &=\frac{2 \cdot 62-1}{3-1} \\ &=\frac{1 \cdot 62}{2} \end{aligned}\)
= 0.81
Percentage dissociation = 81%.

COMPETITION CORNER:

Question 1. 
Which pair of liquid represents positive deviation from Raoult's law?
(a) Water-Hydrochloric acid
(b) Benzene-Methanol 
(c) Water-Nitric acid
(d) Acetone-Chloroform
Answer:
(b) Benzene-Methanol 

Question 2. 
If the concentration of SO₂ in atmosphere is 0.12 ppm by volume then the pH value of rain water is:
(a) 12.5
(b) 5.6
(c) 5.4
(d) 2.0
Answer:
(c) 5.4

Question 3. 
Conc. aqueous sulphuric acid contains 98% H₂SO₄ by weight and its density in 1.80 g mL¹. The volume of acid required to make one litre 0.1 M H₂SO₄ is:
(a) 5.55 mL
(b) 11.10 mL
(c) 16.65 mL
(d) 22.20 mL
Answer:
(a) 5.55 mL

Question 4. 
The unit of elevation constant is
(a) K (molality)^-1
(b) mol kg Kor K^-1 (molality)
(c) Kg mol^-1Kor K^-1 (molality)^-1
(d) K mol kg^-1 or K (molality)
Answer:
(a) K (molality)^-1

Question 5. 
How much volume (in litre) of 3M NaOH (atomic mass of Na = 23) can be obtained from 80 g NaOH?
(a) 2.67
(b) 1.34
(c) 0.67
(d) 0.33
Answer:
(c) 0.67

Question 6. 
An aqueous solution boils at 100.2°C. It will freeze at what temperature? (Kb = 0.5°C/m, Kf = 1.9°C/m)
(a) +0.76
(b) -0.76
(c) - 0.38
(d) +0.38
Answer:
(b) -0.76

Question 7. 
A beaker contains solution of substance'A'. On adding some more amount of substance 'A' in this solution, substance 'A' gets precipitated. So, solution is
(a) Concentrated
(b) Saturated
(c) Unsaturated
(d) Supersaturated
Answer:
(d) Supersaturated

Question 8. 
4L of aqueous solution of 0.02 M NaCl is diluted by adding 1L of water. What will be molarity of this resultant solution?
(a) 0.004
(b) 0.008
(c) 0.012
(d) 0.016
Answer:
(d) 0.016

Question 9. 
The molarity of solution obtained by adding, 800 mL 0.5 M HCl and 200 mL 1M HCl in each other, will be:
(a) 0.8 M
(b) 0.6M
(c) 0.4 M
(d) 0.2M
Answer:
(b) 0.6M

Question 10. 
68.5 g of sucrose (molecular mass 342 g mol^-1) is dissolved in 1000 g water to make a solution. The freezing point of this solution is (Kf (water Kkg mol^-1)
(a) -0.570°C
(b) -0.372°C
(c) -0.520°C
(d) +0.372°C
Answer:
(b) -0.372°C

Question 11. 
Which of the following dilute solution has the value of van't Hoff factor equal to 2?
(a) K₂SO₄
(b) NaHSO₄
(c) Sugar
(d) MgSO₄
Answer:
(d) MgSO₄

Question 12. 
0.1 m NaCl and 0.1 m CH₃COOH are placed in two separate beakers. If their pressures are p₁ and p₂ respectively, then which of the following statement is correct?
(a) P₁  > P₂
(b) P₁ = P₂
(c) P₁ < P₂
(d) P₁ = P₂ = 0 atom
Answer:
(a) P₁  > P₂

Question 13. 
2.65 g of Na₂CO₃ is dissolved in 250 mL solution. If 10 mL of this solution is diluted upto 1L. then, what is the concentration of resultant solution? (molecular weight of Na₂CO₃ = 106)
(a) 0.1 M
(b) 0.001 M
(c) 0.01 M
(d) 104M
Answer:
(b) 0.001 M

Question 14. 
The molarity of 0.2 N Na₂CO₃ is:
(a) 0.1 M
(b) OM
(c) 0.4 M
(d) 0.2M
Answer:
(a) 0.1 M

Question 15. 
8.1 g of HBr is dissolved in 100 g of water to make a solution. What will be the freezing point of solution if acid is 90% ionised in solution?
(K, of water = 1.86 K kg mol^-1)
(a) 0.85°C
(b) -3.53°C
(c) 0°C
(d) -0.35°C
Answer:
(b) -3.53°C