RBSE Solutions for Class 12 Physics Chapter 5 Magnetism and Matter

Rajasthan Board RBSE Solutions for Class 12 Physics Chapter 5 Magnetism and Matter Textbook Exercise Questions and Answers.

RBSE Class 12 Physics Solutions Chapter 5 Magnetism and Matter

RBSE Class 12 Physics Magnetism and Matter Textbook Questions and Answers

Question 5.1.
Answer the following questions regarding earth’s magnetism:
(a) A vector needs three quantities for its specification. Name the three independent quantities conventionally used to specify the earth’s magnetic field.
(b) The angle of dip at a location in southern India is about 18°. Would you expect a greater or lesser dip angle in Britain?
(c) If you make a map of magnetic field lines at Melbourne in Australia, would the lines seen to go into the ground or come out of the ground?
(d) In which direction would a compass free to move in the vertical plane point to, if located right on the geomagnetic north or south pole?
(e) The earth’s field is claimed roughly approximately, the field due to a dipole magnetic moment 8 x 10²² JT^-1 located at the center. Check your order of magnitude of this number same way.
(f) Geologists claim that besides the main magnetic NS poles, there are several local poles on the earth’s surface oriented in different directions. How is such a thing possible at all?
Answer:
(a) Magnetic declination, angle of dip, horizontal component of earth’s magnetic field.

(b) Greater angle of dip in Britain, (it is about 70°), because Britain is closer to the magnetic north pole.

(c) Field lines of B due to the earth’s magnetism would seem to come out of the ground.

(d) A compass is free to move in a horizontal plane, while the earth’s field is exactly vertical at the magnetic poles. So the compass can point in any direction there.

(e) Use the formula for field B on the normal bisector of a dipole of magnetic moment m.
B = \(\frac{\mu_0}{4 \pi} \frac{\mathrm{M}}{r^3}\)
Take M = 8 x 10²² JT^-1
r = 6.4 x 10⁶ m;
one gets B = 0.3 G
which checks with the order of magnitude of the observed field on the earth.

(f) The earth’s field is only approximately a dipole field. Local N-S poles may arise due to, for instance, magnetized mineral deposits.

Question 5.2.
Answer the following questions:
(a) The earth’s magnetic field varies from point to point in space. Does it also change with time? If so, on what time scale does it change appreciably?
(b) The earth’s core is known to contain iron. Yet geologists do not regard this as a source of earth’s magnetism. Why?
(c) The charged currents in the outer conducting regions of the earth’s core are thought to be responsible for earth’s magnetism. What might be the ‘battery’ (i.e. the source of energy) to sustain these currents?
(d) The earth may have ever reversed the direction of its field several times during its history of 4 to 5 billion years. How can geologists know about the earth’s field in such distant past?
(e) The earth’s field departs from its dipole shape substantially at large distances (greater than about 30,000 km). What agencies may be responsible for this distortion?
(f) Interstellar space has an extremely weak magnetic field of the order of 10^-12 T. Can such a weak field of any significant consequence? Explain.
Note: Exercise 5.2 is meant mainly to arouse your curiosity. Answer to some questions above are tentative or unknown. Brief answers wherever possible are given at the end. For detail, you should consult a good text on geomagnetism.
Answer:
(a) Yes, it does change with time. Time scale for appreciable change is roughly a few hundred years. But even on a much smaller scale of a few years, its variations are not completely negligible.

(b) Because molten iron (which is the phase of iron at the high temperatures of the core) is not ferromagnetic.

(c) One possibility is the radioactivity in the interior of the earth.

(d) Earth’s magnetic field gets weakly ‘recorded’ in certain rocks during solidification. Analysis of this rock magnetism offers clues to geomagnetic history. 

(e) At large distances, earth’s field gets modified due to the field of ions in motion (in the earth’s ionosphere). The latter is sensitive to extraterrestrial disturbances such as, for example, the solar wind.

(f) From the relation R = mv/eB, an extremely minute field bends charged particles in a circle of very large radius. Over a small distance, the deflection due to the circular orbit of such large R may not be noticeable, but over the gigantic interstellar distances, the deflection can significantly affect the passage of e.g. cosmic rays.

Question 5.3.
A short bar magnet placed with its axis at 30° with a uniform external magnetic field of 0.25 T experiences a torque of magnitude equal to 4.5 x 10^-2 J. What is the magnitude of magnetic moment of the magnet?
Answer:
Given
θ = 30°, B = 0.25 T
τ = 4.5 x 10^-2 J, M = ?
Since τ = MB sin θ
∴ M = \(\frac{\tau}{B \sin \theta}=\frac{4.5 \times 10^{-2}}{0.25 \times \sin 30^{\circ}}\)
or M = \(\frac{4.5 \times 2}{25}\)
= 0.36 JT^-1

Question 5.4.
A short bar magnet of magnetic moment M = 0.32 JT^-1 is placed in a uniform external magnetic field of 0.15 T. If the bar is free to rotate in the plane of the field, which orientations would correspond to its (i) stable and (ii) unstable equilibrium? What is the potential energy of the magnet in each case?
Answer:
Given
M = 0.32 JT^-1, B = 0.15 T
U =?
θ = 0°
(i) If \(\overrightarrow{\mathrm{M}} || \overrightarrow{\mathrm{B}}\), then we have stable equilibrium and 
U = -MB cos 0° = -0.32 x 0.15 T
= -4.8 x 10^-2 J

(ii) If \(\overrightarrow{\mathrm{M}}\) is anti-parallel to \(\overrightarrow{\mathrm{B}}\), we have unstable equilibrium and θ = 180°
U = -MB cos 180°
= 0.32 x 0.15 T
= 4.8 x 10^-2 J

Question 5.5.
A closely wound solenoid of 800 turns and area of cross-section 2.5 x 10^-4 m² carries a current of 3.0 A. Explain the sense in which the solenoid acts like a bar magnet. What is its associated magnetic moment?
Answer:
Given N = 800, A = 2.5 x 10^-4 m²
I = 3.0 A, M =?
Since M = NIA
∴ M = 800 x 3 x 2.5 x 10^-4
or M = 0.60 JT^-1 along the axis of the solenoid.

Question 5.6.
If the solenoid in Exercise 5.5 is free to turn about the vertical direction and a uniform horizontal magnetic field of 0.25 T is applied, what is the magnitude of the torque on the solenoid when its axis makes an angle of 30° with the direction of the applied field?
Answer:
Given
B = 0.25 T
θ = 30°
Since τ = MB sin θ
= 0.6 x 0.25 x sin 30°
= 7.5 x 10^-2 Nm.

Question 5.7.
A bar magnet of magnetic moment 1.5 JT^-1 lies aligned with the direction of a uniform magnetic field of 0.22 T.
(a) What is the amount of work required by an external torque to turn the magnet so as to align its magnetic moment?
(i) normal to the field direction,
(ii) opposite to the field direction?
(b) What is the torque on the magnet in cases (i) and (ii)?
Answer:
M =    1.5 JT^-1
B =    0.22 T    
W =    ∫dW = \(\int_{\theta_1}^{\theta_2} \tau d \theta\)
or W = \(\int_{\theta_1}^{\theta_2} \mathrm{MB} \sin \theta d \theta\) (∵ τ = MB sin θ)
= MB \([-\cos \theta]_{\theta_1}^{\theta_2}\)
W = -MB (cos θ₂ - cos θ₁)
(a) (i) In this case θ₁ = 0
θ₂ = \(\frac{\pi}{2}\) radian
∴ W = -MB (cos \(\frac{\pi}{2}\) - cos 0°)
= MB (0 -1) = MB
= 1.5 x 0.22 = 0.33 J

(ii) θ₁ = 0, θ₂ = π radian
W = -MB (-1 - 1) = 2 MB
= 2 x 0.33 = 0.66 J

(b) (i) Torque, τ = MB sin \(\frac{\pi}{2}\) = MB x 1
= 0.33 N
(ii) Torque, τ = MB sin π
= MB x 0 = 0

Question 5.8.
A closely wound solenoid of 2000 turns and area of cross-section 1.6 x 10^-4 m², carrying a current of 4.0 A, is suspended through its center allowing it to turn in a horizontal plane.
(а) What is the magnetic moment associated with the solenoid?
(b) What is the force and torque on the solenoid if a uniform horizontal magnetic field of 7.5 x 10^-2 T is set up at an angle of 30° with the axis of the solenoid?
Answer:
(a) Magnetic dipole moment,
M = nIA
= 2000 x 4.0 x 1.6 x 10^-4
= 1.28 JT^-1

(b) Net force = 0
Torque, τ = MB sin θ
= 1.28 x 7.5 x 10^-2 x sin 30°
= 1.28 x 7.5 x 10^-3 x \(\frac{1}{2}\)
= 4.8 x 10^-2 Nm.

Question 5.9.
A circular coil of 16 turns and radius 10 cm, carrying a current of 0.75 A rests with its plane normal to an external field of magnitude 5.0 x 10^-2 T. The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with frequency of 2.0 s^-1. What is the moment of inertia of the coil about its axis of rotation?
Answer:
Magnetic dipole moment of the coil.
M = NIA

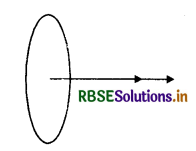
or M = 16 x 0.75 x π(10)² Am²
= 0.377 Am²
Time period of oscillation is given by
T = 2π \(\sqrt{\frac{\mathrm{I}}{\mathrm{MB}}}\)
T = \(\frac{1}{2 \pi} \sqrt{\frac{\mathrm{MB}}{\mathrm{I}}}\) ...........................(1)
∴ Moment of inertia of the coil about the axis of rotation.
I = \(\frac{\mathrm{MB}}{4 \pi^2 v^2}\)
or I = \(\frac{0.377 \times 5.0 \times 10^{-2}}{4 \times(3.14)^2 \times 2^2}\)
= 1.194 kg m²
= 1.2 kg m²

Question 5.10.
A magnetic needle free to rotate in a vertical plane to the magnetic meridian has its north tip pointing down at 22° with the horizontal. The horizontal component of the earth’s magnetic field at the place is known to be 0.35 G. Determine the magnitude of the earth’s magnetic field at the place.
Answer:
Given
δ = 22°, H = 0.35 G
R = ?
Since H = R cos δ
∴ R = \(\frac{\mathrm{H}}{\cos \delta}=\frac{0.35}{\cos 22^{\circ}}\)
or H = \(\frac{0.35}{0.9272}\) = 0.38 G.

Question 5.11.
At a certain location in Africa, a compass point 12° west of the geographical north. The north tip of the magnetic needle of a dip circle placed in the plane of the magnetic meridian points 60° above the horizontal. The horizontal component of the earth’s field is measured to be 0.16 G. Specify the direction and magnitude of the earth’s field at the location.
Answer:
Dip,    δ = 60°
V = 0.16 G = 0.16 x 10^-4 T
Since V = R cos δ
0.16 x 10^-4 = R. cos δ
= R x \(\frac{1}{2}\)
= R = 2 x 0.16 x 10^-4
= 0.32 x 10^-4 T
The earth’s field lies in a vertical plane 12° west of the geographical meridian making an angle of 60° (upwards) with the horizontal (magnetic south to magnetic north) direction.

Question 5.12.
A short bar magnet has a magnetic moment of 0.48 JT^-1. Give the direction and magnitude of the magnetic field produced by the magnet at a distance of 10 cm from the center of the magnet on (i) the axis (ii) the equatorial line (normal bisector) of the magnet.
Answer:
Given M = 0.48 JT^-1,  d = 10 cm B = ?
(i) Along the axis (for a short dipole)
B = \(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{M}}{d^3}\) = 10^-7 x \(\frac{2 \times 0.48}{10^{-3}}\)
= 0.96 x 10^-4 T = 0.96 G along S-N direction
(ii) Along equatorial line (for a short dipole)
B = \(\frac{\mu_0}{4 \pi} \frac{\mathrm{M}}{d^3}\) = 0.48 G along N-S direction.

Question 5.13.
A short bar magnet placed in a horizontal plane has its axis aligned along the magnetic north-south direction. Null points are found on the axis of the magnet at 14 cm from the center of the magnet. The earth’s magnetic field at the place is 0.36 G and the angle of dip is zero. What is the total magnetic field on the normal bisector of the magnet at the same distance as the null point (i.e. 14 cm from the center of the magnet)?
Answer:
Given, d = 14 cm = 14 x 10^-2 m
H = 0.36 G = 0.36 x 10^-4 T
Since null points are found on the axis of the magnet, so, we have
\(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{M}}{d^3}\) = H
or \(\frac{\mu_0}{4 \pi} \frac{\mathrm{M}}{d^3}=\frac{\mathrm{H}}{2}\) ........................(1)
Now on equatorial line at same distance d, we have
B₁ = \(\frac{\mu_0}{4 \pi} \frac{\mathrm{M}}{d^3}\)
Using Eq. (1).
B₁ = \(\frac{\mathrm{H}}{2}=\frac{0.36 \times 10^{-4}}{2}\)
= 0.18 x 10^-4 T
∴ Total magnetic field at this point on the equatorial line will be
B = B₁ + H = 0.18 x 10^-4 + 0.36 x 10^-4
= 0.54 x 10^-4 T
or B = 0.54 G  in the direction of earth's field.

Question 5.14.
If the bar magnet in Exercise 5.13 is turned around by 180°, where will the new null point be located?
Answer:
If the bar magnet is turned around 180°, the neutral point would be on equatorial line and we have
\(\frac{\mu_0}{4 \pi} \frac{\mathrm{M}}{d_1^3}\) = H ........................(1)
In exercise 5.13, we have
\(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{M}}{d^3}\) = H .....................(2)
From (1) and (2)
\(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{M}}{d^3}=\frac{\mu_0}{4 \pi} \frac{\mathrm{M}}{d_1^3}\)
or d₁³ = \(\frac{d^3}{2}\)
or d₁ = d x 2^-1/3
or d₁ = 14 x 2^-1/3
≅ 11.1 m on the normal bisector.

Question 5.15.
A short bar magnet of magnetic moment 5.25 x 10^-2 JT-1 is placed with its axis perpendicular to the earth’s field direction. At what distance from the center of the magnet the resultant field is inclined at 45° with earth’s field on
(i) its normal bisector
(ii) its axis.
Magnitude of the earth’s field at the place is given to be 0.42 G. Ignore the length of the magnet in comparison to the distances involved.
Answer:
M = 5.25 x 10-2 JT^-1, H = 0.42, G = 0.42 x 10^-4 T
(i) Since the resultant makes an angle of 45° with the earth’s field, the magnetic field due to the magnet must be equal to that of the earth.
\(\frac{\mu_0}{4 \pi} \frac{\mathrm{M}}{r_1^3}\) = 0.42 x 10^-4
∴ \(\frac{10^{-7} \times 5.25 \times 10^{-2}}{r_1^2}\) = 0.42 x 10^-4
r₁ = 5 x 10^-2 m = 5 cm
 
(ii) For a point on its axis, magnetic field is given by
H = \(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{M}}{r_2^2}\)
(for small magnet) 
∴ \(\frac{\mu_0}{4 . \tau} \frac{2 \mathrm{M}}{\left(r_2\right)^2}\) = 0.42 x 10^-4
on solving r₂ = 6.3 cm.

ADDITIONAL EXERCISES

Question 5.16.
Answer the following questions:
(a) Why does a paramagnetic sample display greater magnetization (for the same magnetizing field) when cooled?
(b) Why is diamagnetism, in contrast, almost independent of temperature?
(c) If a toroid used bismuth for its core, will the field in the core be (slightly) greater or (slightly) less than when the core is empty?
(d) If the permeability of a ferromagnetic material independent of the magnetic field? If not is it more for lower or higher fields?
(e) Magnetic field lines are always nearly normal to the surface of a ferromagnet at every point.
(f) Would the maximum possible magnetization of a paramagnetic sample be of the same order of magnitude as the magnetization of a ferromagnet?
Answer:
(a) The tendency to disrupt the alignment of dipoles (with the magnetizing field) arising from random thermal motion is reduced at lower temperatures.

(b) The induced dipole moment in a diamagnetic sample is always opposite to the magnetizing field, no matter what the internal motion of the atoms is.

(c) Slightly less, since bismuth is diamagnetic.

(d) No, it is evident from the magnetization curve. From the slope of the magnetization curve, it is clear that µ is greater for lower fields.

(e) Proof of the important fact ( of much practical use) is based on boundary conditions of magnetic fields (B and H) at the interface of two media (when one of the media has µ >> 1, the field lines meet this medium nearly normally).

(f) Yes. Apart from minor differences in the strength of the individual atomic dipoles of two different materials, a paramagnetic sample with saturated magnetization will have the same order of magnetization. But of course, saturation requires impractically high magnetizing fields.

Question 5.17.
Answer the following questions:
(a) Explain qualitatively on the basis of domain picture the irreversibility in the magnetization curve of a ferromagnet.
(b) The hysteresis loop of a soft iron piece has a much smaller area than that of a carbon steel piece. If the material is to go through repeated cycles of magnetization, which piece will dissipate greater heat energy?
(c) ‘A system displaying a hysteresis loop such as a ferromagnet is a device for storing memory.’ Explain the meaning of this statement.
(d) What kind of ferromagnetic material is used for coating magnetic tapes in a cassette player, or for building memory stores in a modern computer?
(e) A certain region of space to be shielded from magnetic fields. Suggest a method.
Answer:
(a) Since, in a ferromagnetic substance the magnetic properties are due to alignment of domains, therefore on withdrawing the magnetizing field the original domain formation does not take place.

(b) Carbon steel piece, because heat lost per cycle is proportional to the area of the hysteresis loop.

(c) Magnetisation of a ferromagnet is not a single-valued function of the magnetizing field. Its value for a particular field depends both on the field and also on the history of magnetization (i.e. how many cycles of magnetization it has gone through etc.) In other words, the value of magnetization is a record or ‘memory’ of its cycle of magnetization. If information bits can be made to correspond these cycles, the system displaying such a hysteresis loop can act as a device for storing information.

(d) Ceramics (specially treated barium iron oxides) also called ferrites.

(e) Surround the region by soft iron rings. Magnetic field lines will be drawn into the rings, and the enclosed space will be free of magnetic fields. But this shielding is only approximate, unlike the perfect electric shielding of a cavity in a conductor placed in an external electric field.

Question 5.18.
A long straight horizontal cable carries a current of 2.5 A in the direction 10° south of west to 10° north of east. The magnetic meridian of the place happens to be 10° west of the geographical meridian. The earth’s magnetic field at the location is 0.33 G and angle of dip is zero. Locate the line of neutral points. (Ignore the thickness of the cable).
Answer:
Current = 2.5 A
Earth’s field R = 0.33 x 10^-4 T
Angle of dip, δ = 0°
Horizontal component H = R cos δ
H = R cos 0° = R 
= 0.33 x 10^-4 T
Vertical component V = 0.
At neutral point, intensity of magnetic field = H

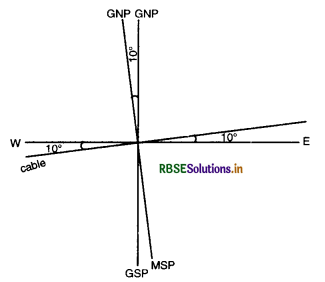
∴ \(\frac{\mu_0 I}{2 r}\) = H
r = \(\frac{\mu_0 \mathrm{I}}{2 \times \mathrm{H}}\)
or r = \(\frac{4 \pi \times 10^{-7} \times 2.5}{2 \times .33 \times 10^{-4}}\)
= 47.59 x 10^-3 m
r = 4.75 cm
The locus of the neutral points is a straight line parallel to the cable at a perpendicular distance of 4.75 cm above the plane of the paper.

Question 5.19.
A telephone cable at a place has four long straight horizontal wires carrying a current of 1.0 A in the same direction east to west. The earth’s magnetic field at the place is 0.39 G and angle of dip is 35°. The magnetic declination is nearly zero. What are the resultant magnetic field at point 4.0 cm below and above the cable?
Answer:
Magnetic field due to current

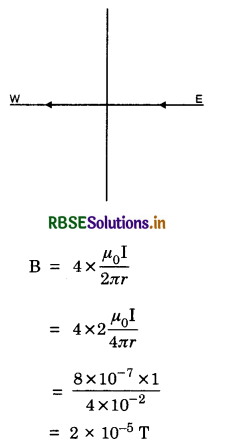
Horizontal component of the earth's magnetic field.
H = B cos δ
= 0.39 x 10^-4 x cos 35° [∵ 1G = 10^-4 T]
= 0.319 x 10^-4 T 
= 3.19 x 10^-5 T

(i) At 4 cm below the field due to current and due to earth’s magnetic field (\overrightarrow{\mathrm{H}}) in the same direction.
Total horizontal magnetic field, in horizontal direction
H₁ = (2 + 3.19) x 10^-5 T
or H₁ = 5.19 x 10^-5 T
Vertical component V = R sin 35°
= 0.39 x 10^-4 x sin 35°
= 2.2 x 10^-5 T
Resultant R = \(\sqrt{\mathrm{V}^2+\mathrm{H}_1^2}\)
= \(\sqrt{\left(2.2 \times 10^{-5}\right)^2+\left(5.19 \times 10^{-5}\right)^2}\)
= 5.54 x 10^-5 T

(ii) On the upper side the magnetic field due to the current and the earth are in the opposite directions.
Effective horizontal component 
∴ H₂ = 3.19 x 10^-5 - 2 x 10^-5
=    1.19 x 10^-5 T
V = 2.2 x 10^-5 T
∴ Resultant magnetic field, R₂ = \(\sqrt{\mathrm{H}_2^2+\mathrm{V}^2}\)
= \(\sqrt{\left(1.19 \times 10^{-5}\right)^2+\left(2.2 \times 10^{-5}\right)^2}\)
= 2.5 x 10^-5 T

Question 5.20.
A compass needle free to turn in horizontal plane is placed at the center of a circular coil of 30 turns and radius 12 cm. The coil is in a vertical plane making an angle of 45° with the magnetic meridian. When the current in the coil is 0.35 A, the needle points west to east.
(a) Determine the horizontal component of the earth’s magnetic field at the location.
(b) The current in the coil is reversed and the coil is rotated about its vertical axis by an angle of 90° in the anticlockwise sense looking from above. Predict the direction of the needle. Take the magnetic declination at the place to be zero.
Answer:
(a) The magnetic field at the center of the coil is given by
B = \(\frac{\mu_0 \mathrm{IN}}{2 r}\)
Component of magnetic field parallel to meridian.
= \(\frac{\mu_0 \mathrm{IN}}{2 r}\)cos 45°
Component of magnetic field in the magnetic meridian should be equal and opposite to that of the coil
-\(\frac{\mu_0 \mathrm{IN}}{2 r}\)cos 45° + H = 0
or H = \(\frac{\mu_0 \mathrm{IN}}{2 r}\)cos 45°
or H = \(\frac{4 \pi \times 10^{-7} \times 30 \times 0.35}{2 \times .12} \times \frac{1}{\sqrt{2}}\)
= 0.39 x 10^-4 T.

(b) East to west (i.e. the needle will reverse its original direction).

Question 5.21.
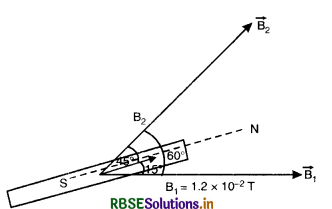
A magnetic dipole is under the influence of two magnetic fields. The angle between the field direction is 60°, and one of the fields has a magnitude of 1.2 x 10^-2 T. If the dipole comes to stable equilibrium at an angle of 15° with this field, what is the magnitude of the other field?
Answer:
In equilibrium, torque exerted by each field on the dipole are equal.
τ₁ = τ₂
MB₁ sin 15° = MB₂ sin 45°
B₂ = \(\frac{B_1 \sin 15^{\circ}}{\sin 45^{\circ}}\)
= \(\frac{1.2 \times 10^{-2} \sin 15^{\circ}}{\sin 45^{\circ}}\)
= 4.4 x 10^-3 T

Question 5.22.
A monoenergetic (18 kV) electron beam initially in the horizontal direction is subject to a horizontal magnetic field of 0.40 G normal to the initial direction. Estimate the up or down deflection of the beam over a distance of 30 cm (mg = 9.11 x 10^-31 kg, e = 1.60 x 10¹⁹ C).
Note. (Data in this exercise are so chosen that the answer will give you an idea of the effect of earth’s magnetic field on the motion of electron beam from the electron gun to the screen in a TV set). 

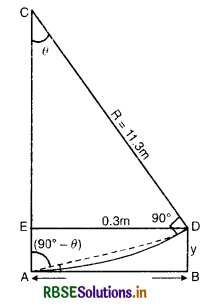
Answer:
E = 18 kV = 18000 V
B = 0.40 G = 0.40 x 10^-4 T
Since = \(\frac{m e v^2}{r}\) = Bqv
r = \(\frac{m e v}{\mathrm{~B} q}=\frac{\sqrt{2 m e \mathrm{E}}}{\mathrm{B} q}\)
or, r = \(\frac{\sqrt{2 \times 9.1 \times 10^{-31} \times 18 \times 10^3 \times 1.6 \times 10^{-19}}}{0.40 \times 10^{-4} \times 1.6 \times 10^{-19}}\)
= 11.3 m
The deflection according to Fleming’s left-hand rule will be up or down.
From right angled triangle CED,
\(\frac{\mathrm{CE}}{\mathrm{CD}}\) = cos θ
CE = CD cos θ
CE = R cos θ
AE = CA - CE
AE = R - R cos θ = R (1 - cos θ)
∴ y = R (1 - cos θ)
From ∆CED
sin θ = \(\frac{0.3}{11.3}\)
∴ θ = 1.52
∴ cos θ = 0.9996
∴ y = 11.3 (1 - 0.9996) = 3.98 x 10-3 m
or y = 3.98 mm
= 4 mm.

Question 5.23.
A sample of paramagnetic salt contains 2.0 x 10²⁴ atomic dipoles each of dipole moment 1.5 x 10^-23 JT-1. The sample is placed under a homogeneous magnetic field of 0.84 T and cooled to a temperature of 4.2 K. The degree of magnetic saturation achieved is equal to 15.8. What is the total dipole moment of the sample for a magnetic field of 0.98 T and a temperature of 2.8 K? (Assume Curie’s law).
Answer:
Initially total dipole moment
= 0.15 x 1.5 x 10^-23 x 2.0 x 10²⁴
= 4.5 JT^-1
Using Curie’s law

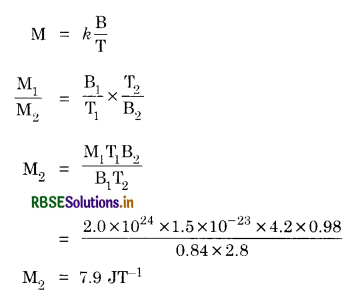
Question 5.24.
A Rowland ring of mean radius 15 cm has 3500 turns of wire wound on a ferromagnetic core of relative permeability 800. What is the magnetic field (B) in the core for a magnetizing current of 1.2 A?
Answer:

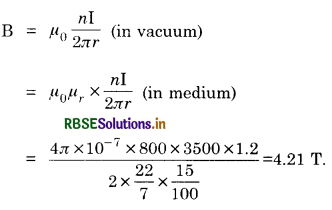
Question 5.25.
The magnetic moment vector µs and µl associated with the intrinsic spin angular momentum S and orbital angular momentum l respectively of an electron are predicted by quantum theory (and verified experimentally to a high accuracy) to be given by
µ_s = -(e/m)s
µ_l = -(e/2m)l
Which of these relations is in accordance with the result expected ‘classically’? Outline the derivation of the classical result.
Answer:
Of the two, relation µ_l = -(e/2m) l is in accordance with classical physics. It follows easily from the definitions of µ_l and l:
µ_l = IA = \(\left(\frac{e}{\mathrm{~T}}\right)\)πr² ...................(1)
l = mvr = \(\frac{2 m \pi r^2}{\mathrm{~T}}\) ....................(2) (∵ v = 2πr/T)
where r is the radius of the circular orbit which the electron of mass m and charge (-e) completes in a time T.
Clearly \(\frac{\mu_l}{l}=\frac{e}{2 m}\)
Since charge of the electron is negative (= -e), it is easily seen that µ_l and l are antiparallel, both normal to the plane of the orbit. Therefore 
\(\vec{\mu}_l=-\frac{e}{2 m} \vec{l}\)
Note µ_s is constant and is e/m, i.e., twice the classically expected value. This latter a classical result (verified experimentally) is an outstanding consequence of modern quantum theory.

SELECTED EXEMPLAR PROBLEMS

MCQ I (with one correct option)

Question 5.1.
A toroid of n turns, mean radius R and cross-sectional radius a carry current I. It is placed on a horizontal table taken as x-y plane. Its magnetic moment \(\vec{m}\).
(a) is non-zero and points in the z-direction by symmetry.
(b) points along the axis of the toroid (\(\vec{m}\) = m \(\hat{\phi}\) )
(c) is zero, otherwise, there would be a field falling as \(\frac{1}{r^3}\) at large distance outside the toroid.
(d) is pointing radially outwards.
Answer:
(c) In a toroid, the magnetic field is only confined inside the body of the toroid in the form of concentric magnetic lines of force and outside the toroid, the magnetic field is zero, so magnetic moment of the toroid is zero.

Question 5.2.
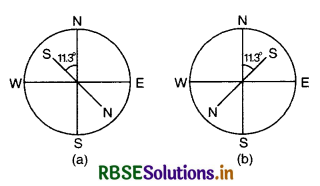
The magnetic field of Earth can be modeled by that of a point dipole placed at the center of the Earth. The dipole axis makes an angle of 11.3° with the axis of Earth. At Mumbai, declination is nearly zero. Then,
(a) the declination varies between 11.3° W to 11.3°E.
(b) the least declination is 0°.
(c) the plane defined by dipole axis and Earth axis passes through Greenwich.
(d) declination averaged over Earth must be always negative.
Answer:
(a) As the magnetic dipole placed at the center of earth at 11.3°, so there are two possibilities as shown ahead:

Question 5.3.
In a permanent magnet at room temperature
(а) magnetic moment of each molecule is zero.
(b) the individual molecules have non-zero magnetic moments which are all perfectly aligned.
(c) domains are partially aligned.
(d) domains are all perfectly aligned.
Answer:
(c) At room temperature thermal agitation do not allow the domains to have complete alignment, hence the domains are partially aligned.

Question 5.4.
Consider the two idealized systems:
(i) a parallel plate capacitor with large plates and small separation and (ii) a long solenoid of length L >> R radius of cross-section. In (i) E is ideally treated as a constant between plates and zero outside. In (ii) magnetic field is constant inside the solenoid and zero outside. These idealized assumptions, however, contradict fundamental laws as below:
(а) case (i) contradicts Gauss’s law for electrostatic fields.
(b) case (ii) contradicts Gauss’s law for magnetic fields.
(c) case (i) agrees with \(\oint \overrightarrow{\mathrm{E}} . \mathrm{d} \vec{l}\) = 0
(d) case (ii) contradicts \(\oint \overrightarrow{\mathrm{H}} . \mathrm{d} \vec{l}\) = I_cn
Answer:
(b) According to Gauss’s law in magnetism
\(\oint \overrightarrow{\mathrm{B}} . \mathrm{d} \vec{S}\) = 0
It contradicts in case of (ii) i.e. magnetic field is constant inside the solenoid and zero outside.

Question 5.5.
A paramagnetic sample shows a net magnetization of 8 Am^-1 when placed in an external magnetic field of 0.6T at a temperature of 4K. When the same sample is placed in an external magnetic field of 0.2T at a temperature of 16K, the magnetization will be: 
(a) \(\frac{32}{3}\) Am^-1
(b) \(\frac{2}{3}\) Am^-1
(c) 6 Am^-1
(d) 2.4 Am^-1
Answer:
(b) Given, I₁ = 8 Am^-1, B₁ = 0.6T, T₁ = 4K
I₂ = ?, B₂ = 0.2T, T₂ = 16K
From Curie's law,

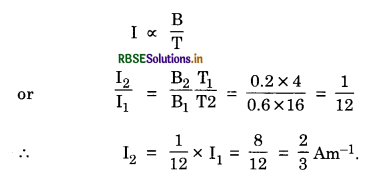
MCQII. [with more than one correct options]

Question 5.6.
S is the surface of a lump of magnetic material.
(a) Lines of B are necessarily continuous across S.
(b) Some lines of B must be discontinuous across S.
(c) Lines of H are necessarily continuous across S.
(d) Lines of H cannot all be continuous across S.
Answer:
(a), (d)
Magnetic field lines for magnetic induction \(\vec{B}\) are necessarily continuous across S.
The magnetic intensity (\(\vec{H}\)) outside the lump is given by, H = \(\frac{\mathrm{B}}{\mu_0}\) and inside the lump H = \(\frac{\mathrm{B}}{\mu_0 \mu_r}\), hence H cannot be continuous across S.

Question 5.7.
The primary origin(s) of magnetism lies in
(a) atomic currents. 
(b) Pauli exclusion principle.
(c) polar nature of molecules.
(d) intrinsic spin of electron.
Answer:
(a), (d), The electrons revolve and spin about the nucleus of an atom and give rise to current and hence magnetism.

Question 5.8.
A long solenoid has 1000 turns per meter and carries a current of 1 A. It has a soft iron core µ_r = 1000. The core is heated beyond the Curie temperature Tc.
(а) The H field in the solenoid is (nearly) unchanged but the B field decreases drastically.
(b) The H and B fields in the solenoid are nearly unchanged.
(c) The magnetization in the core reverses direction.
(d) The magnetization in the core diminishes by a factor of about 108.
Answer:
(a), (d)
Since H = nI = 1000 x 1 = 1000 Am = constant
And B = µ₀µ_rnI = µ₀(nl)µ_r = µ₀ (constant) µ_r
As µ_r changes, so B changes
Also \(\frac{\chi_{\text {ferro }}}{\chi_{\text {Para }}}=\frac{10^3}{10^{-5}}\) = 10⁸

Question 5.9.
Essential difference between electrostatic shielding by a conducting shell and magnetostatic shielding is due to
(a) electrostatic field lines can end on charges and conductors have free charges.
(b) lines of \(\vec{B}\) can also end but conductors cannot end them.
(c) lines of \(\vec{B}\) cannot end on any material and perfect shielding is not possible.
(d) shell of high permeability materials can be used to divert lines of \(\vec{B}\) from the interior region.
Answer:
(a), (c), (d)
Electric field inside a conductor is zero, but magnetic field lines cannot end on any material but prefer to pass through the material having high permeability.

Question 5.10.
Let the magnetic field on earth be modeled by that of a point magnetic dipole at the center of earth. The angle of dip at a point on the geographical equator.
(a) is always zero.
(b) can be zero at specific points.
(c) can be positive or negative.
(d) is bounded.
Answer:
(b), (c), (d)
Here angle of dip satisfies all these three options.

Very Short Answer Type Questions

Question 5.11.
A proton has spin and magnetic moment just like an electron. Why then its effect is neglected in magnetism of materials?
Answer:
Magnetic moment

or M_p << M_e.

Question 5.12.
A permanent magnet in the shape of a thin cylinder of length 10 cm has M = 10⁶A/m. Calculate the magnetization current I_M.
Answer:
Given l = 10 cm = 0.1 m, M = 10⁶ Am^-1
Since M = \(\frac{\mathrm{I}_m}{l} \)
or I_m = M x l = 10⁶ x 0.1 = 10⁵ A.

Question 5.13.
Explain quantitatively the order of magnitude difference between the diamagnetic susceptibility of N₂ (~5 x 10^-9) (at STP) Cu (~10^-5).
Answer:
Since χ = \(\frac{\mathrm{I}}{\mathrm{H}}=\frac{\mathrm{M} / \mathrm{V}}{\mathrm{H}}=\frac{\mathrm{M}}{\mathrm{HV}}=\frac{\mathrm{M} \rho}{\mathrm{H} m}\)
or χ ∝ ρ
\(\frac{\chi_{\mathrm{N}_2}}{\chi_{\mathrm{Cu}}}=\frac{\rho_{\mathrm{N}_2}}{\rho_{\mathrm{C}}}\) = 1.6 x 10^-4
So we can say that difference in susceptibility of N₂ and Cu is due to their densities.

Question 5.14.
From molecular viewpoint, discuss the temperature dependence of susceptibility for diamagnetism, paramagnetism, and ferromagnetism.
Answer:
Diamagnetism is due to orbital motion of electrons in an atom developing magnetic moments opposite to the applied field and hence diamagnetism is independent of temperature.
Paramagnetism and ferromagnetism is due to alignment of atomic magnetic moments in the direction of applied field. When the temperature of such substance is increased, this alignment is disturbed and hence susceptibility of both the substances decreases.

Question 5.15.
A ball of superconducting material is dipped in liquid nitrogen and placed near a bar magnet, (i) In which direction will it move? (ii) What will be the direction of its magnetic moment? 
Answer:
Both the superconducting material and liquid nitrogen are diamagnetic in nature. When ball of superconducting material is dipped in liquid nitrogen, it behaves as diamagnetic material near a bar magnet, hence it moves away froth the magnet.
As a diamagnetic substance gets magnetized in a direction opposite to the applied magnetic field, so the magnetic moment of the ball of diamagnetic material is opposite to the direction of magnetic field of the bar magnet.

Short Answer Type Questions

Question 5.17.
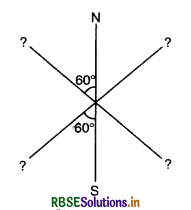
Three identical bar magnets are riveted together at center in the same plane as shown in Fig. EP 5.17 (a). This system is placed at rest in a slowly varying magnetic field. It is found that the system of magnets does not show any motion. The north-south poles of one magnet is shown in the Fig. Determine the poles of the remaining two.

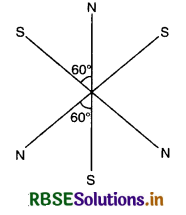
Answer:
When the given set of magnets does not show any motion in a slowly varying magnetic field, means that the system is in equilibrium position and net torque on the system is zero. So only possibility is that the poles of remaining magnets are as shown in the figure EP 5.17 (b).

Question 5.18.
Suppose we want to verify the analogy between electrostatic and magnetostatic by an explicit experiment. Consider the motion of (i) electric dipole \(\overrightarrow{\mathbf{p}}\) in an electrostatic field E and (ii) magnetic dipole \(\overrightarrow{\mathbf{M}}\) in a magnetic field \(\overrightarrow{\mathbf{B}}\). Write down a set of conditions on \(\overrightarrow{\mathbf{E}}\), \(\overrightarrow{\mathbf{B}}\), \(\overrightarrow{\mathbf{p}}, \overrightarrow{\mathbf{m}}\) so that the two motions are verified to be identical. (Assume identical initial conditions.)
Answer:
Let θ be angle between p and E for electric dipole and also between m and B for magnetic dipole so we have
τ_e = pE sin θ
τ_m = MB sin θ
Two motions will be identical, if
τ_e = τ_m
i.e. pE sin θ = mB sin θ
pE = MB
or E = \(\frac{\mathrm{M}}{p}B\)
As    E    =    cB
∴ p c B = MB
or p = \(\frac{\mathrm{M}}{\mathrm{c}}\)

Question 5.20.
Use (i) the Ampere’s law for H and (ii) continuity of lines of B, to conclude that inside a bar magnet, (a) lines of H run from the N pole to S pole, while (b) lines of B must run from the S pole to N pole.
Answer:
Consider a bar magnet and a line of \(\vec{B}\) through the magnet, it must be a closed line as shown in the figure.

If c be amperian loop, then
\(\int_{\mathrm{Q}}^{\mathrm{P}} \mathrm{H} d \mathrm{I}=\int \frac{\overrightarrow{\mathrm{B}}}{\mu_0} \cdot \overrightarrow{d l}>0\)
[∵ angle between \(\overrightarrow{\mathrm{B}}\) and \(\overrightarrow{\mathrm{dl}}\) is less than 90°]
Thus magnetic field line B must run from S to N inside the bar magnet.
According to Ampere’s law
\(\oint \overrightarrow{\mathrm{H}} \cdot \overrightarrow{d l}\) = 0
or \(\oint \cdot \overrightarrow{\mathrm{H}} \cdot \overrightarrow{d l}=\int_{\mathrm{P}}^{\mathrm{Q}} \overrightarrow{\mathrm{H}} \cdot \overrightarrow{d l}+\int_{\mathrm{Q}}^{\mathrm{P}} \overrightarrow{\mathrm{H}} \cdot \overrightarrow{d l}=0\)
As \(\int_0^{\mathrm{P}} \overrightarrow{\mathrm{H}} \cdot \overrightarrow{d l}>0, so \int_{\mathrm{P}}^{\mathrm{Q}} \overrightarrow{\mathrm{H}} \cdot \overrightarrow{d l}<0\)
It will be so if angle between \(\overrightarrow{\mathrm{H}}\) and \(\overrightarrow{\mathrm{dl}}\) is more than 90° thus lines from \(\overrightarrow{\mathrm{H}}\) must run from N to S pole, inside the magnet.

Long Answer Type Questions

Question 5.22.
What are the dimensions of χ, the magnetic susceptibility? Consider an H-atom. Guess an expression for χ, up to a constant by constructing a quantity of dimensions of χ, out of parameters of the atom: e, m, v, R and µ₀. Here, m is the electronic mass, v is electronic velocity, R is Bohr radius. Estimate the number so obtained and compare with the value of |χ| ~ 10-5 for many solid materials.
Answer:
Since χ = \(\frac{\mathrm{I}}{\mathrm{H}}\)
So χ is dimensionless [∴ I & H have the same units and dimension]
Since χ is related to e, m, v, R and µ₀ and dimensions of µ₀ = [MLQ ^-2]
From Biot-Savart’s law
dB = \(\frac{\mu_0}{4 \pi} \frac{\mathrm{I} d l \sin \theta}{r^2}\)
or µ₀ = \(\frac{4 \pi r^2 d \mathrm{~B}}{\mathrm{I} d l \sin \theta}=\frac{4 \pi r^2}{\mathrm{I} d l \sin \theta} \cdot \frac{\mathrm{F}}{q v \sin \theta}\)
∴ Dimensions of µ₀ = \(\frac{\left[\mathrm{L}^2\right]\left[\mathrm{MLT}^{-2}\right]}{\left[\mathrm{QT}^{-}\right][\mathrm{L}][\mathrm{Q}]\left[\mathrm{LT}^{-1}\right][1]}\)
[Q has the dimensions of charge]
Since χ has no dimensions, so it has no involvement of charge Q in the dimensional formula. It will be so if µ₀ and e together should have value µ₀e².
Let χ = µ₀e² m^a v^b R^c ...........................(i)
writing dimensional formulae of all physical quantities on both sides of equation (i), we get
[M⁰L⁰T⁰Q⁰] = [MLQ^-2] [Q²] [M]^a [LT^-1]^b [L]^c
= [M^{a + 1} L^{b + c + 1} T^-b Q⁰]
Equating the powers of M, L and T on both sides we get :
a + 1 = 0
a = -1

b + c + 1 = 0
0 + c + 1 = 0
c = -1

-b = 0
b =0

So. eqn.(i) becomes
χ = µ₀e² m^-1 v²R^-1 = \(\frac{\mu_0 e^2}{m \mathrm{R}}\)
= \(\frac{4 \pi \times 10^{-7} \times 1.6 \times 10^{-19}}{9.1 \times 10^{-31} \times 10^{-10}}\) = 10^-4
∴ \(\frac{\chi}{\chi_{\text {given solid }}}=\frac{10^{-4}}{10^{-5}}\) = 10

Question 5.24.
Consider the plane S formed by the dipole axis and the axis of earth. Let P be point on the magnetic equator and in S. Let Q be the point of intersection of the geographical and magnetic equators. Obtain the declination and dip angles at P and Q.
Answer:
(i) From the figure EP 5.24, we find that the point P is on the magnetic equator, so declination at P = 0.
And angle of dip at P = 0

(ii) Point Q is on magnetic equator, so angle of dip = 0, because the angle of dip at the equator is zero. As earth tilted on its axis by 11.3°, so the declination of Q is 11.3°.

Question 5.25.
There are two current-carrying planar coils made each from identical wires of length L. C₁ is circular (radius R) and C₂ is square (side a). They are so constructed that they have the same frequency of oscillation when they are placed in the same uniform \(\overrightarrow{\mathbf{B}}\) and carry the same current. Find an in terms of R.
Answer:
Given circular coil of radius R and length L.
∴ No, of turns per unit length n₁ = \(\frac{\mathrm{L}}{2 \pi \mathrm{R}}\)

For square of side a.
n₂ = \(\frac{\mathrm{L}}{4 a}\)
Magnetic moment of C₁
M₁ = n₁IA₁ = \(\frac{\mathrm{LI} \pi \mathrm{R}^2}{2 \pi \mathrm{R}}=\frac{\mathrm{LIR}}{2}\)
Magnetic moment of C₂

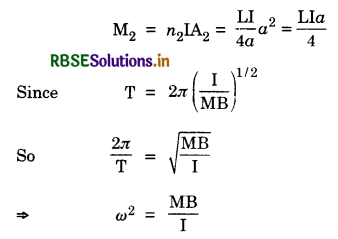
For circular coil C₁
ω₁ = \(\frac{\mathrm{M}_1 \mathrm{~B}}{\mathrm{I}_1}\)
For coil C₂
ω₂ = \(\frac{\mathrm{M}_2 \mathrm{~B}}{\mathrm{I}_2}\)
According to question
ω₁ = ω₂
∴ \(\frac{\mathrm{M}_2}{\mathrm{M}_1}=\frac{\mathrm{I}_2}{\mathrm{I}_1}\)
or \(\frac{\mathrm{LI} a}{4} \times \frac{2}{\mathrm{LIR}}=\frac{\frac{\mathrm{Ma} a^2}{12}}{\frac{\mathrm{MR}^2}{2}}\)
or \(\frac{a}{2 \mathrm{R}}=\frac{a^2}{6 \mathrm{R}^2}\)
or 3R = a
This is the value of 3R.