RBSE Solutions for Class 12 Physics Chapter 4 Moving Charges and Magnetism

Rajasthan Board RBSE Solutions for Class 12 Physics Chapter 4 Moving Charges and Magnetism Textbook Exercise Questions and Answers.

RBSE Class 12 Physics Solutions Chapter 4 Moving Charges and Magnetism

RBSE Class 12 Physics Moving Charges and Magnetism Textbook Questions and Answers

Question 4.1.
A circular coil of wire consisting of 100 turns, each of radius 8.0 cm carries a current of 0.40 A. What is the magnitude of the magnetic field \(\overrightarrow{\mathbf{B}}\) at the center of the coil?
Answer:
Given n = 100, r = 8.0 cm = 8 x 10^-2, I = 0.4 A, B = ?
At the center of circular coil
B = \(\frac{\mu_0 n I}{2 r}=\frac{4 \pi \times 10^{-7} \times 100 \times 0.4}{2 \times 8 \times 10^{-2}}\) = π x 10⁴ T
≃ 3.14 x 10^-4 T

Question 4.2.
A long straight wire carries a current of 35 A. What is the magnitude of the field \(\overrightarrow{\mathbf{B}}\) at a point 20 cm from the wire?
Answer:
Given I = 35 A, a = 20 cm = 0.2 m?
B = \(\frac{\mu_0 2 \mathrm{I}}{4 \pi a}\)
∴ B = \(\frac{10^{-7} \times 2 \times 35}{0.2}\)
or B = 3.5 x 10^-5 J

Question 4.3.
A long straight wire in the horizontal plane carries current of 50 A in north to south direction. Given the magnitude and direction of  \(\overrightarrow{\mathbf{B}}\)  at a point 2.5 m east of the wire.
Answer:
Given I = 50 A, B = ?, a = 2.5 m
B = \(\frac{\mu_0 2 \mathrm{I}}{4 \pi a}\)
or B = \(\frac{10^{-7} \times 2 \times 50}{2.5} \) = 4 x 10^-6 T

Question 4.4.
A horizontal overhead power line carries a current of 90 A in east to west direction. What is the magnitude and direction of the magnetic field due to the current 1.5 m below the line?
Answer:
I = 90 A, a = 1.5 m, B = ?

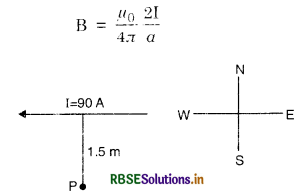
or B = \(\frac{10^{-7} \times 2 \times 90}{1.5}\)
= 1.2 x 10^-5 T (Towards north)

Question 4.5.
What is the magnitude of magnetic force per unit length on a wire carrying a current of 8 A and making an angle of 30° with the direction of a uniform magnetic field of 0.15 T?
Answer:
I = 8A. θ = 30°, B = 0.15 T
\(\frac{\mathrm{F}}{l}\) = ?
Since \(\frac{\mathrm{F}}{l}\) = BI sin θ = 0.15 x 8 x 1 x sin 30°
or F = 0.6 Nm^-1

Question 4.6.
A 3.0 cm wire carrying a current of 10 A is placed inside a solenoid perpendicular to its axis. The magnetic field inside the solenoid is given to be 0.27 T. What is the magnetic force on the wire?
Answer:
Given l = 3.0 cm = 3 x 10^-2 m, I = 10 A
θ = 90°, B = 0.27 T, F = ?
Since F = BI l sinθ
∴ F = 0.27 x 10 x (3 x 10^-2) sin 90°
= 8.1 x 10^-2 N

Question 4.7.
Two long and parallel straight wires A and B carrying currents of 8.0 A and 5.0 A in the same direction are separated by a distance of 4.0 cm. Estimate the force on a 10 cm section of wire A.
Answer:
Given I₁ = 8.0 A, I₂ = 5.0 A, r = 4.0 cm = 0.04 m, l = 10 cm = 0.01 m
Since F = \(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{I}_1 \mathrm{I}_2 l}{r}=\frac{10^{-7} \times 2 \times 8 \times 5 \times 0.01}{0.04}\)
= 2 x 10^-5 N
(direction is given by Fleming's left-hand rule)
So we find that the current in the two parallel wires is flowing in the same direction, so the force will be normal to A towards B.

Question 4.8.
A closely wound solenoid 80 cm long has 5 layers of windings of 400 turns each. The diameter of the solenoid is 1.8 cm. If the current carried is 8.0 A, estimate the magnitude B inside the solenoid near its center.
Answer:
Given l = 80 cm = 0.8 m
Total number of turns, N = 5 x 400 = 2000
∴ No.of turns per unit length
n = \(\frac{\mathrm{N}}{l}=\frac{2000}{0.8}\) = 2500
D = 1.8 cm, I = 8.0 A
∴ Magnetic field inside the solenoid near its center
B = µ₀nI = 4π x 10^-7 x 2500 x 8
= 2.5 x 10^-2 T

Question 4.9.
A square coil of side 10 cm consists of 20 turns and carries a current of 12 A. The coil is suspended vertically and the normal to the plane of the coil makes an angle of 30° with the direction of a uniform horizontal magnitude of torque experienced by the coil?
Answer:
Given l = 10 cm = 10^-1 m
So A = 10^-2 m², n = 20, θ = 30°, B = 0.80 T, τ = ?, I = 12 A
since τ = n I AB sin θ
= 20 x 12 x 10^-2 x 0.8 x sin 30°
= 0.96 Nm

Question 4.10.
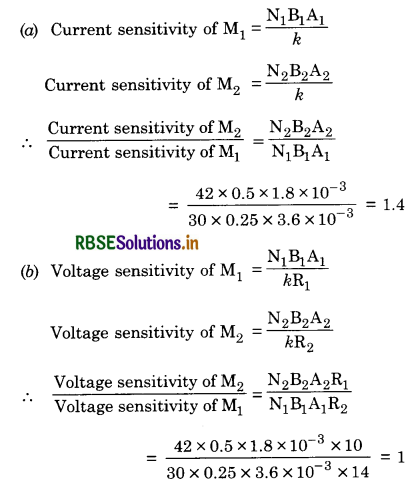
Two moving coil meters, M₁ and M₂ have the following particulars:
R₁ = 10 Ω, N₁ = 30, A₁ = 3.6 x 10^-3 m², B₁ = 0.25 T
R₂ = 14 , N₂ = 42, A₂ = 1.8 x 10^-3 m², B₂ = 0.50 T
(The spring constants are identical for the two meters). Determine the ratio of (a) current sensitivity and (b) voltage sensitivity of M₂ and M₁.
Answer:
Given
R₁ = 10 Ω, N₁ = 30, A₁ = 3.6 x 10^-3 m², B₁ = 0.25 T
R₂ = 14 Ω, N₂ = 42, A₂ = 1.8 x 10^-3 m², B₂ = 0.50 T

Question 4.11.
In a chamber, a uniform magnetic field of 6.5 G (1 G = 10^-4 T) is maintained. An electron is shot into the field with a speed of 4.8 x 10⁶ ms^-1 normal to the field. Explain why the path of the electron is a circle. Determine the radius of the circular orbit, (e = 1.5 x 10^-19 C, m_e = 9.1 x 10^-31 kg).
Answer:
Given B = 6.5 G = 6.5 x 10^-4 J, v = 4.8 x 10⁶ ms^-1
r = ?, e = 1.6 x 10^-19 C, m_e = 9.1 x 10^-31 kg
Since v ⊥ B and necessary centripetal force is provided by magnetic field.

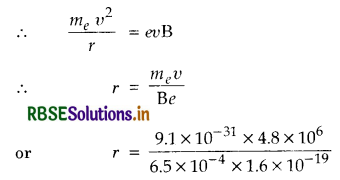
= 4.2 x 10^-2 m = 4.2 cm

Question 4.12.
In exercises 4.11, obtain the frequency of revolution of the electrons in its circular orbit. Does the answer depend on the speed of the electron? Explain.
Answer:
Since frequency of revolution is given by
v = \(\frac{\mathrm{Be}}{2 \pi m_e}=\frac{6.5 \times 10^{-4} \times 1.6 \times 10^{-19}}{2 \times 3.142 \times 9.1 \times 10^{-31}}\) = 18.18 x 10⁶ Hz
= 18 MHz.
The frequency is independent of speed of electron.

Question 4.13.
(a) A circular coil of 30 turns and radius 8.0 cm carrying a current of 6.0 A is suspended vertically in a uniform horizontal magnetic field of magnitude 1.0 T. The field lines make an angle of 60° with the normal to the coil. Calculate the magnitude of the counter torque that must be applied to prevent the coil from turning.
(b) Would your answer change if the circular coil in (a) were replaced by a planner coil of some irregular shape that encloses the same area?
(All other particulars are also unaltered).
Answer:
(a) n = 30, r = 8 cm
∴ A = π(8)² cm², I = 6A, B = 1 T, θ = 60°
Torque τ = n BIA sinθ
= 30 x 1 x 6 x \(\frac{\pi(8)^2}{100 \times 100}\) x sin 60°
= 3.133 Nm.

(b) No, the answer is unchanged because the formula τ = NIA x B is true for a planar loop of any shape.

ADDITIONAL EXERCISES

Question 4.14.
Two concentric circular coils X and Y of radii 16 cm and 10 cm respectively lie in the same vertical plane containing the north-south direction. Coil X has 20 turns and carries a current of 16A; coil Y has 25 turns and carries a current of 18 A. The sense of the current in X is anticlockwise and in Y clockwise, for an observer looking at the coil facing west. Given the magnitude and direction of the net magnetic field due to the coils at their center.
Answer:
For coil X,
I = 16A, n = 20, r = \(\frac{16}{100}\)m
Magnetic field at the center of coil X
B = \(\frac{\mu_0}{4 \pi} \frac{2 \pi n \mathrm{I}}{r}\) = 10^-7 x \(\frac{2 \pi \times 20 \times 16}{\frac{16}{100}}\)
= \(\frac{10^{-7} \times 2 \pi \times 100 \times 16 \times 20}{16}\)
= 4π x 10^-4 T
By right-hand rule, the direction of magnetic field is towards west.
For coil Y,
I = 18A, n = 25, r = \(\frac{10}{100}\)
B = \(\frac{\mu_0}{4 \pi} \times \frac{2 \pi n \mathrm{I}}{r}\) = 10^-7 x \(\frac{2 \pi \times 18 \times 25}{\frac{10}{100}}\)
= 10^-7 x \(\frac{2 \pi \times 25 \times 18 \times 100}{10}\)
= 9π x 10^-4 T
By right-hand rule, the direction of magnetic field is towards east.
Net magnetic field = 9π x 10^-4 T - 4π x 10^-4 T
= 5π x 10^-4 
= 1.6 x 10^-3 T (towards west)

Question 4.15.
A magnetic field of 100G? (1G = 10^-4 T) is required which is uniform in a region of linear dimension about 10 cm and area of cross-section about 10^-3 m². The maximum current carrying capacity of a given coil of wire is 15 A and the number of turns per unit length that can be wound around a core is at most 1000 turns m^-1. Suggest some appropriate design particulars of a solenoid for the required purpose. Assume the core is not ferromagnetic.
Answer:
Since B = µ₀ n I
So I = \(\frac{\mathrm{B}}{\mu_0 n}=\frac{100 \times 10^{-4}}{4 \pi \times 10^{-7} \times 1000}\)
or I = 7.96 n = 8 A.
Similarly, if we take n = 800 per m
I = \(\frac{\mathrm{B}}{\mu_0 n}=\frac{100 \times 10^{-4}}{4 \pi \times 10^{-7} \times 800}\) = 9.95 A
or I = 10 A
So we can produce B = 100 G in a number of ways.

Question 4.16.
(a) For a circular coil of radius R and N turns, carrying current I, the magnitude of magnetic field at a point on its axis at a distance x from its center is given by
B = \(\frac{\mu_0 \mathrm{IR}^2 \mathrm{~N}}{2\left(x^2+\mathrm{R}^2\right)^{3 / 2}}\)
Show that this reduces to the familiar result for field at the center of the coil.
(b) Consider two parallel co-axial circular coils of equal radius R, and number of turns N, carrying equal currents in the same direction and separated by a distance R. Show that the field on the axis around the mid-point between coils is uniform over a distance that is small as compared to R and it is given by
B = 0.72\(\frac{\mu_0 \mathbf{N I}}{\mathbf{R}}\) approximately.
(Such an arrangement to produce a nearly uniform magnetic field over a small region is known as Helmholtz coils)
Answer:
(a) Biot-Savart's law. It states that the magnetic field dB at a point P due to a current element Idl is given by
dB ∝ \(\frac{I d l \sin \theta}{r^2}\)
or dB = \(\frac{\mu_0}{4 \pi} \frac{\mathrm{Id} l \sin \theta}{r^2}\)
Magnetic Field at a Point on the Axis of a Circular Coil
I = Current length the coil
Let, O be the center of coil
R = Radius of the circular coil
x = Distance of point P on the axis from the center of the coil
AB = an element of the coil
dl = Length of the element AB
CP = r
According to Biot-Savart's law, magnetic field dB at the point P due to element \(\overrightarrow{d l}\) is given by

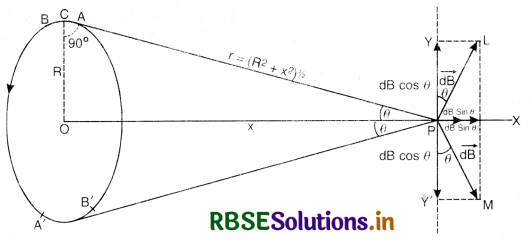
\(\overrightarrow{d \mathrm{~B}}=\frac{\mu_0}{4 \pi} \frac{\mathrm{I} \overrightarrow{d l} \times \vec{r}}{r^3}\)
The direction \(\overrightarrow{d B}\) at point will be along PL i.e. perpendicular to CP and in the plane of paper. As angle between \(I\overrightarrow{d l} \) and \(\vec{r}\) is 90°, the magnitude of magnetic field due to small element at point P is given by
dB = \(\frac{\mu_0}{4 \pi} \cdot \frac{\mathrm{I} d l}{r^2}\) ............................(1)

If we calculate the magnitude \overrightarrow{d B} due to an equal current element A'B' lying just opposite to the current element AB, it will be found that the magnitude of the magnetic field in this case also is the same as given by equation (1) but it acts along PM. The components of two magnetic fields along YY' will cancel out as they are equal in magnitude and opposite in direction. Same will happen for the current element taken in similar pairs.

Therefore, component of magnetic field due to a current element along PX is dB sinθ. The magnetic field due to the whole coil at P is obtained by integrating dB sinθ over the circumference of the coil i.e.
Magnetic field at P due to the circular coil is given by
B = ∫ dB sinθ
= ∫\(\frac{\mu_0}{4 \pi} \cdot \frac{\mathrm{I} d l}{r^2}\) sinθ
= \(\frac{\mu_0}{4 \pi} \cdot \frac{I}{r^2}\) sinθ ∫dl
Now, ∫dl = circumference of the coil of radius
R, = 2πR
∴ B = \(\frac{\mu_0}{4 \pi} \cdot \frac{\mathrm{I}}{r^2}\) sinθ 2πR
= \(\frac{\mu_0}{4 \pi} \cdot \frac{2 \pi \mathrm{IR}}{r^2}\) sinθ
From right-angled ∆OPC, we have 

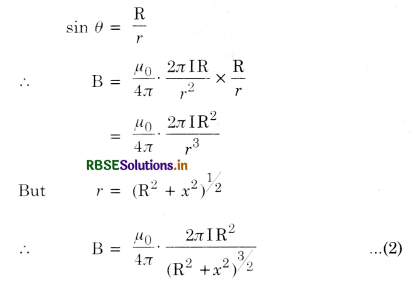
The direction of magnetic field is along PX.
If the coil consists of n turns, the strength of magnetic field at point P due to the coil will become n times that given by equation (2) i.e.
B = \(\frac{\mu_0}{4 \pi} \cdot \frac{2 \pi n \mathrm{IR}^2}{\left(\mathrm{R}^2+x^2\right)^{3 / 2}}\)
Special cases
1. Magnetic field at the center of the coil. 
When point P lies at the center of the coil, x = 0. Then, setting x = 0 in equation (2), we have 
B = \(\frac{\mu_0}{4 \pi} \cdot \frac{2 \pi \mathrm{I}}{\mathrm{R}}\)  .......................(3)
For a coil of n turns, the expression will be 
B = \(\frac{\mu_0}{4 \pi} \cdot \frac{2 \pi n \mathrm{I}}{\mathrm{R}}\) ....................(4)
2. When observation point lies far away from the center of the coil. In case, observation point P lies at a distance very large as compared to the radius of the coil i.e. if x >>R, then, R² can be neglected as compared to x² in equations (2) and (3).
Therefore, for a coil of single turn,
B = \(\frac{\mu_0}{4 \pi} \times \frac{2 \pi \mathrm{I} R^2}{x^2}\) .....................(5)
But πR² = A, the area of coil, Therefore, 
B = \(\frac{\mu_0}{4 \pi} \times \frac{2 \mathrm{IA}}{x^3}\) .............................(6)

(b) Magnetic field at p due to coil
B = \(\frac{\mu_0}{4 \pi} \frac{2 \pi \mathrm{IR}^2 \mathrm{~N}}{\left(\mathrm{R}^2+x^2\right)^{3 / 2}}\)
= \(\frac{\mu_0}{4 \pi} \times \frac{2 \pi \mathrm{INR}^2}{\left(\mathrm{R}^2+\frac{\mathrm{R}^2}{4}\right)^{3 / 2}}=\frac{\mu_0 \mathrm{INR}^2}{2\left(\frac{5}{4}\right)^{\frac{3}{2}} \mathrm{R}^3}\)
or B = \(\frac{\mu_0}{2} \frac{\mathrm{NI}}{\mathrm{R} \times 1.4}\)

Similarly magnetic field due to coil Y is
B = 0.36\(\frac{\mu_0 \mathrm{NI}}{\mathrm{R}}\)
∴ Total magnetic field = 0.72\(\frac{\mu_0 \mathrm{NI}}{\mathrm{R}}\) tesla at P due to coil X and Y.

Question 4.17.
A toroid has a core (non-ferromagnetic) of inner radius 25 cm and outer radius 26 cm, around which 3500 turns of wire are wound. If the current in the wire is 11A, what is the magnetic field (a) outside the toroid, (b) inside the core of the toroid, (c) in the empty space surrounded by the toroid?
Answer:
The magnetic field for toroid is 
B = µ₀nI = µ₀ x \(\frac{\mathrm{N}}{2 \pi r}\)I [∵ n = \(\frac{\mathrm{N}}{2 \pi r}\)]
(a) The magnetic field is zero outside the toroid.
(b) Inside the toroid magnetic field is 
B = \(\frac{4 \pi \times 10^{-7}}{2 \pi} \times \frac{3500 \times 11}{\frac{25.5}{100}}\)

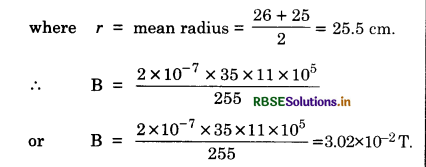
(c) Magnetic field in empty space surrounded by the toroid is zero.

Question 4.18.
Answer the following questions:
(ignore gravity throughout).
(a) A magnetic field that varies in magnitude from point but has a constant direction (east to west) is set up in a chamber. A charged particle enters the chamber and travels undeflected along a straight path with constant speed. What can you say about the initial velocity of the particle?
(b) A charged particle enters an environment of a strong and non-uniform magnetic field varying from point to point both in magnitude and direction and comes out of it following a complicated trajectory. Would its final speed equal the initial speed if it suffers no collisions with the environment.
(c) An electron traveling west to east enters a chamber having a uniform electrostatic field in a north-to-south direction. Specify the direction in which a uniform magentic field should be set up to prevent the electron from deflecting from its straight line path.
Answer:
(a) Initial velocity v is either parallel or antiparallel to B.
(b) Yes, because magnetic force can change the direction of v, not its magnitude.
(c) B should be in a vertically downward direction.

Question 4.19.
An electron emitted by a heated cathode and accelerated through a potential difference of 2.0 k V enters a region with uniform magnetic field of 0.15 T. Determine the trajectory of the electron if the field is transverse to its initial velocity, (a) is transverse to its initial velocity, (b) makes an angle of 30° with the initial velocity.
Answer:
(a) Since magnetic field is perpendicular to initial velocity of electron, therefore, the electron will move in circular path.
B = 0.15 T
P.D. = 200 V
K.E. of electron = eV = \(\frac{1}{2}\)mv²
∴ v² = \(\frac{2 e \mathrm{~V}}{m}\)
(m = mass of electron, e = charge of electron)

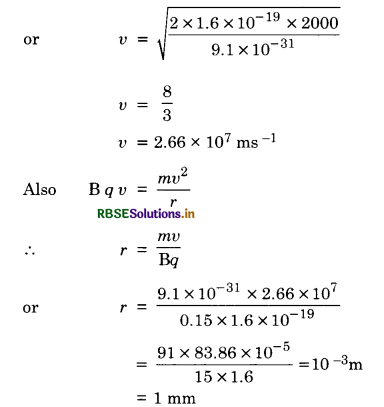
(b) When magnetic field makes an angle 30° with the initial velocity i.e. θ = 30°.
Then v' = v sinθ = \(\sqrt{\frac{2 e \mathrm{~V}}{m}}\) sin 30°
or v' = \(\frac{8}{3} \times 10^7 \times \frac{1}{2}=\frac{4}{3} \times 10^7\) ms^-1
The radius of the helical path is 
r = \(\frac{m v^{\prime}}{\mathrm{Be}}=\frac{9 \times 10^{-31} \times\left(\frac{4}{3} \times 10^7\right)}{0 \cdot 15 \times 1 \cdot 6 \times 10^{-19}}\) = 0.5 mm

Question 4.20.
A magnetic field set up using Helmholtz coils (described in Exercise 4.16) is uniform in a small region and has a magnitude of 0.75 T. In the same region, a uniform electrostatic field is maintained in a direction normal to the common axis of the coils. A narrow beam of (single-species) charged particles all accelerated through 15 kV enters this region in a direction perpendicular to both the axis of the coils and the electrostatic field. If the beam remains undeflected when the electrostatic field is 9.0 x 10⁵ Vm^-1, make a simple guess as to what the beam contains. Why is the answer not unique?
Answer:
B = 0.75 T, E = 9 x 10⁶ Vm^-1
v = \(\frac{\mathrm{E}}{\mathrm{B}}\) (∵ beam is undeflected)
= \(\frac{9 \times 10^5}{0.75}\) = 12 x 10⁶ ms^-1 (∵ eE = ev B)
K>E> of charged particle = \(\frac{1}{2}\)mv² = eV
or \(\frac{e}{m}=\frac{v^2}{2 \mathrm{~V}}=\frac{144 \times 10^{12}}{2 \times 15000}\) = 4.8 x 10⁷ C kg^-1
Particle is deuteron; the answer is not unique because only the ratio of charge to mass is determined. Other possible answers are He⁺⁺, Li⁺⁺, etc.
∴ He⁺⁺ and Li⁺⁺ also have the same value of e/m \(\left[\because \frac{e}{m}=\frac{2 e}{2 m}=\frac{3 e}{3 m}\right]\)

Question 4.21.
A straight horizontal conducting rod of length 0.45 m and mass 60 g is suspended by two vertical wires at its ends. A current of 5.0 A is set up in the rod through the wires.
(a) What magnetic field should be set up normally to the conductor in order that the tension in the wires is zero?
(b) What will be the total tension in the wires if the direction of current is reversed, keeping the magnetic field the same as before? (Ignore the mass of the wire) g = 9.8 ms^-2.
Answer:
(a) Rod carrying current placed in uniform magnetic field experiences a force BI l which is balanced by weight of rod
F = BI l
mg = BI l
B = \(\frac{m g}{\mathrm{I} l}=\frac{60 \times 9.8}{1000 \times 0.45 \times 5}\)
B = 0.26 T
A horizontal magnetic field of magnitude 0.26 normal to the conductor in such a direction that Fleming's left-hand rule gives a magnetic force upwards.
(b) Force due to magnetic field = BI l
= 0.26 x 5 x 0.45 = 5.85 N
Weight of rod = \(\frac{60}{1000}\) x 9.8 = 0.588 N
Total force = 0.588 + 0.585 = 1.173 N.

Question 4.22.
The wires which connect the battery of an automobile to its starting motor carry a current of 300A (for a short time). What is the force per unit length between the wires if they are 70 cm long and 1.5 cm apart? Is the force attractive or repulsive?
Answer:
F = \(\frac{\mu_0}{4 \pi} \times \frac{2 \mathrm{I}_1 \mathrm{I}_2}{r}\)
= 10^-7 x \(\frac{2 \times 300 \times 300 \times 100}{1.5 \times 10^{-2}}\)
= 1.2 Nm (Repulsive force)

Question 4.23.
A uniform field equal to 1.5 T exists in a cylindrical region of radius 10.0 cm, its direction parallel to the axis along east to west. A wire-carrying current of 7.0 A in the north-to-south direction passes through this region. What is the magnitude and direction of the force on the wire if
(a) the wire intersects the axis,
(b) the wire is turned from N-S to northeast-northwest direction,
(c) the wire in the N-S direction is lowered from the axis by a distance of 6.0 cm?
Answer:
(a) F = BI l sin 90° = 1.5 x 7 x \(\frac{20}{100}\) or F = 2.1 N acting vertically downwards.
(b) Now θ = 45°, and length of the wire in the cylindrical region of the magnetic field is l₁ (say) and is given by
l = l₁ sin 45°
or l₁ = \(\frac{l}{\sin 45^{\circ}}=\frac{l}{1 / \sqrt{2}}=\sqrt{2}\) l
So force F₁ = BIl₁ sin 45°
= 1.5 x 7.0 x \(\sqrt{2} l \times \frac{l}{\sqrt{2}}\) = 10.5 x 2.0
= 2.1 N.
(c) When the wire is lowered through a distance 6.0 cm, and reaches at CD then length of the wire in the magnetic field,
l₂ = 2x
and x is given by
x.x = 4 x (10 + 6)
or x = 8 cm
∴ l₂ = 8 x 2 = 16 cm

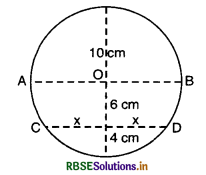
= \(\frac{16}{100}\) m
∴ Force on the wire
F₂ = BI l₂ = \(\frac{1 \cdot 5 \times 7 \times 16}{100}\)
= 1.68 N ( vertically downwards)

Question 4.24.
A uniform magnetic field of 3000 G is established along the positive Z-direction. A rectangular loop of sides 10 cm and 5 cm carries a current of 12 A. What is the torque on the loop in the different cases shown in the figures? What is the force in each case? Which case corresponds to stable equilibrium?

Answer:
(a) Torque = 3000 G = 300 x 10^-4 T.
Area A = 10 x 5 = 50 cm² = \(\frac{50}{100 \times 100}\) m²
τ = BIA = \(\frac{3}{10} \times 12 \times \frac{50}{100 \times 100}\)
= 18 x 10^-2 Nm along Y-axis.

(b) Same as in (a)

(c) 1.8 x 10^-2 Nm along X-direction.

(d) 1.8 x10^-2 Nm at an angle 240° with the +x direction.

(e) Zero.

(f) Zero.
Force is zero in each case. Case (e) corresponds to stable and case (f) to unstable equilibrium.

Question 4.25.
A circular coil of 20 turns and radius 10 cm is placed in a uniform magnetic field of 0.10 T normal to the coil. If the current in the coil is 5.0 A, what is the
(a) total torque on the coil, (b) total force on the coil, (c) average force on each electron in the coil due to the magnetic field? (The coil is made of copper wire of cross-sectional area 10^-5 m² and the free electron density in copper is given to be about 10²⁹ m^-3)
Answer:
n = 20, r = 10 cm, B = 0.10 T, I = 5.0 A
(a) Torque acting on the coil = n BIA sin θ = 0
(b) Force acting on the coil = BI l sin θ = 0
(c) Force on each electron
where n = number of electrons per unit volume.
and A = Area of cross-section of wire.
F = Bev = \(\frac{\mathrm{BI}}{n \mathrm{~A}}\) (I = ven A)
= \(\frac{0.1 \times 5}{10^{29} \times 10^{-5}}\)
= 5 x 10^-25 N.

Question 4.26.
A solenoid 60 cm long and of radius 4.0 cm has 3 layers of winding of 300 turns each. A 2.0 cm long wire of mass 2.5 g lies inside the solenoid near its center normal to its axis; both the wires and the axes of the solenoid are in the horizontal plane. The wire is connected through two leads parallel to the axis of the solenoid to an external battery which supplies a current of 6.0 A in the wire. What value of current (with appropriate sense of circulation) in the windings of the solenoid can support weight of the wire? g = 9.8 ms^-2.
Answer:
The wire lies in the magnetic field of solenoid.
The force due to field balances the weight.
Let I = Current needed through the solenoid
∴ B = µ₀nI
The force acts on the wire normal to its length

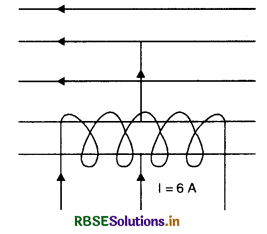
∴ F = BI'l
= µ₀ nI I'l and n = \(\frac{\frac{900}{60}}{100}\) = 1500
∴ µ₀nII'l = mg
I = \(\frac{2.5 \times 9.8}{1000 \times 4 \pi \times 10^{-7} \times 1500 \times 6 \times \frac{1}{15}}\)
= 108 A
The direction of current in the solenoid is as shown in the above Fig. TBQ 4.26.

Question 4.27.
A galvanometer coil has resistance of 12 Ω and the meter shows full-scale deflection for a current of 3mA. How will you convert the galvanometer into a voltmeter of range of to 18 V?
Answer:
Here G = 12 Ω, I_g = 3mA = 3 x 10^-3 A.
V = 18 V
Since R = \(\frac{\mathrm{V}}{\mathrm{I}_g}\) - G
∴ R = \(\frac{18}{3 \times 10^{-3}}\) - 12 = (6000 - 12) Ω
or R = 5988 Ω

Question 4.28.
A galvanometer coil has a resistance of 15 Ω and the meter shows full-scale deflection for a current of 4 mA. How will you convert the galvanometer into an ammeter of range 0 to 6A?
Answer:
Here G = 15 Ω, I_g = 4 mA = 0.004 A, I = 6A
Since = \(\frac{\mathrm{I}_g \mathrm{G}}{\mathrm{I}-\mathrm{I}_g}=\frac{0.004 \times 15}{6-0.004}=\frac{0.06}{5.996}\)
≃ 10 x 10^-3 Ω = 10 m Ω
So shunt resistance = 10 m Ω

SELECTED EXEMPLAR PROBLEMS

MCQ I. (with one correct option)

Question 4.1.
Two charged particles traverse identical helical paths in a completely opposite sense in a uniform magnetic field B = B₀\(\widehat{\boldsymbol{k}}\).
(a) They must have equal z-components of momenta.
(b) They must have equal charges.
(c) They necessarily represent a particle-antiparticle pair.
(d) The charge-to-mass ratio satisfy :
\(\left(\frac{e}{m}\right)_1+\left(\frac{e}{m}\right)_2\) = 0
Answer:
(d) For a charged particle of charge q and mass m having pitch d, we have
\(\frac{q}{m}=\frac{2 \pi v \cos \theta}{d m}\)
i.e. \(\frac{q}{m}\) = constant
As the charged particles traverse identical helical paths in a completely opposite sense in a uniform magnetic field, so they must have the same and opposite sign
∴ \(\left(\frac{e}{m}\right)_1+\left(\frac{e}{m}\right)_2\) = 0
Note: Take e instead of q for solution

Question 4.2.
Biot-Savart's law indicates that the moving electrons (velocity v) produce a magnetic field B such that
(a) B ⊥ v
(b) B ||v.
(c) it obeys inverse cube law.
(d) it is along the line joining the electron and point of observation.
Answer:
(a) From Biot-Savart's law, we have 
\(\overrightarrow{\mathrm{B}}=\frac{\mu_0}{4 \pi} \frac{\mathrm{I} d \vec{l} \times \vec{r}}{r^3}\)
or \(\overrightarrow{\mathrm{B}}=\frac{\mu_0}{4 \pi} \frac{q\left(\frac{\overrightarrow{d l}}{t} \times \vec{r}\right)}{r^3}\)
or \(\overrightarrow{\mathrm{B}}=\frac{\mu_0}{4 \pi}\left[\frac{\vec{v} \times \vec{r}}{r^3}\right]\)
The direction of \(\overrightarrow{\mathrm{B}}\) is along (\(\overrightarrow{\mathrm{v}}\) x \(\overrightarrow{\mathrm{r}}\)) i.e. perpendicular to the plane of \(\overrightarrow{\mathrm{v}}\)  and \(\overrightarrow{\mathrm{r}}\)

Question 4.3.
An electron is projected with uniform velocity along the axis of a current carrying long solenoid. Which of the following is true?
(a) The electron will be accelerated along the axis
(b) The electron path will be circular about the axis.
(c) The electron will experience a force at 45° to the axis and hence execute a helical path.
(d) The electron will continue to move with uniform velocity along the axis of the solenoid.
Answer:
(d) The Lorentz force is given by
F = -e vB sinθ
As θ = 0, so F = 0 and hence the electron will continue to move with uniform velocity along the axis of the solenoid.

Question 4.4.
In a cyclotron, a charged particle:
(a) undergoes acceleration all the time.
(b) speeds up between the dees because of the magnetic field.
(c) speeds up in a dee.
(d) slows down within a dee and speeds up between dees.
Answer:
(a) The charged particle accelerates between the dees and inside the dees, it has centripetal acceleration due to perpendicular magnetic field. Hence, it accelerates all the time.

Question 4.5.
A circular current loop of magnetic moment M is in an arbitrary orientation in an external magnetic field B. The work done to rotate the loop by 30° about an axis perpendicular to its plane is:
(a) MB
(b) \(\sqrt{3} \frac{M B}{2}\)
(c) \(\frac{\text { MB }}{2}\)
(d) Zero.
Answer:
(d) Since, work done, W = MB [cos θ₁ - cos θ₂]
As θ₁ = θ₂
∴ W = 0

MCQ II (with more than one correct option)

Question 4.6.
The gyro-magnetic ratio of an electron in an H-atom, according to Bohr model, is:
(a) independent of which orbit it is in.
(b) negative.
(c) positive.
(d) increases with the quantum number n.
Answer:
(a) , (b) 
Since gyro-magnetic ratio = \(\frac{e}{2 m}\) = constant.
As the value of e is negative, so gyro-magnetic ratio is negative.

Question 4.7.
Two identical current-carrying coaxial loops, carry current I in an opposite sense. A simple amperian loop passes through both of the n once. Calling the loop as C,
(a) \(\oint_c \overrightarrow{\mathbf{B}} \cdot \overrightarrow{d l}=\mp 2 \mu_0 \mathbf{I}\)
(b) the value of \(\oint_c \overrightarrow{\mathbf{B}} \cdot \overrightarrow{d l}\) is independent of sense of C.
(c) there may be a point on C where  \(\overrightarrow{\mathbf{B}}\)  and  \(\overrightarrow{d l}\) are perpendicular.
(d) \(\overrightarrow{B}\) vanishes everywhere on C.
Answer:
(b), (c)
From Ampere ciruital law
\(\oint_c \overrightarrow{\mathrm{B}} \cdot \overrightarrow{d l}\) = µ₀ (I - I) = 0 [because current is in opposite sense]
Hence  \(\oint_c \overrightarrow{\mathrm{B}} \cdot \overrightarrow{d l}\) is independent of sense of C.
Also, there may be a point c where \(\overrightarrow{\mathrm{B}} \perp \overrightarrow{d l}\) and hence
\(\oint_c \overrightarrow{\mathrm{B}} \cdot \overrightarrow{d l}\) = 0

Question 4.8.
A charged particle would continue to move with a constant velocity in a region wherein,
(a) E = 0, B ≠ 0
(b) E ≠ 0, B ≠ 0
(c) E ≠0, B = 0
(d) E = 0, B = 0
Answer:
(a), (b) and (d) 
Since  \(\overrightarrow{\mathrm{F}}_e=q \overrightarrow{\mathrm{E}}\)  and  \(\overrightarrow{\mathrm{F}}_m=q(\vec{v} \times \overrightarrow{\mathrm{B}})\)
Now Fe = 0, if E = 0 and Fm = 0 if θ = 180° or 0°
hence B ≠ 0
Aslo E = 0 and B = 0
And, the resultant force q \(\overrightarrow{\mathrm{E}}+q(\vec{v} \times \overrightarrow{\mathrm{B}})\) = 0, if E ≠ 0, B ≠ 0.

Very Short Answer Type Questions

Question 4.9.
Verify that the cyclotron frequency ω = \(\frac{e \mathrm{~B}}{m}\) has the correct dimensions of [T]^-1.
Answer:

Question 4.10.
Show that a force that does not work must be a velocity-dependent force.
Answer:
Since work done
W = \(\overrightarrow{\mathrm{F}} \cdot \overrightarrow{d l}=\overrightarrow{\mathrm{F}} \cdot \vec{v}\) d t = 0
or \(\overrightarrow{\mathrm{F}} \cdot \vec{v}\) = 0
or Fv cos θ = 0
or θ = 90°
So force \(\vec{F}\) that does no work be velocity dependent if θ = 90°. If \(\vec{v}\) changes (direction), the (direction) F should also change so that above condition is satisfied.

Question 4.11.
The magnetic force depends on v which depends on the inertial frame of reference. Does then the magnetic force differ from inertial frame to frame? Is it reasonable that the net acceleration has a different value in different frames of reference?
Answer:
Magnetic force
F_m = qv B sin θ
Thus, Fm is velocity dependent and it differs from one inertial frame to another.
The net acceleration which comes into existence out of this is however, frame-independent (non-relativistic physics) for inertial frames.

Question 4.12.
Describe the motion of a charged particle in a cyclotron if the frequency (rf) field were doubled.
Answer:
Tn cyclotron, the frequency of revolution
v = \(\frac{1}{\mathrm{~T}}=\frac{\mathrm{B} q}{2 \pi m}\)
If v' = 2v, then
T' = \(\frac{1}{v^{\prime}}=\frac{1}{2 v}=\frac{\pi m}{\mathrm{~B} q}\) = constant
Thus the particle will accelerate and deaccelerate alternately. So the radius of path in the Dee's will remain unchanged.

Question 4.13.
Two long wires carrying current I₁ and I₂ are arranged as shown in Fig. EP 4.13. The one carrying current I₁ is along the x-axis. The other carrying current I₂ is along a line parallel to the y-axis given by x = 0 and z = d. Find the force exerted at O₂ because of the wire along the x-axis.

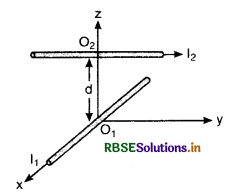
Answer:
Direction of magnetic field at O₂ due to wire carrying current is 
B₁ = \(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{I}_1}{d}\)
The direction of B₁ is along Y-axis.
The second wire is along y-axis and hence the force is zero.

Short Answer Type Questions

Question 4.14.
A current carrying loop consists of an identical quarter circle of radius R, lying in the positive quadrants of the x-y, y-z and z-x planes with their centers at the origin, joined together. Find the direction and magnitude of B at the origin.
Answer:
Since magnetic field at the center of an arc subtending an angle θ carrying current I is given by 

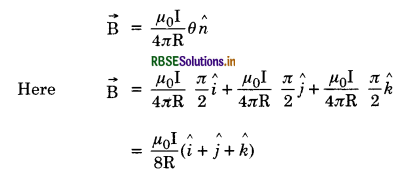
Question 4.15.
A charged particle of charge e and mass m is moving in an electric field E and magnetic field B. Construct dimensionless quantities and quantities of dimension [T]^-1.
Answer:
There is no dimensionless quantity
And [T]^-1 = [ω] = [\(\frac{e \mathrm{~B}}{m}\)]

Question 4.16.
An electron enters with a velocity into a cubical region (faces parallel to coordinate planes) in which there are uniform electric and magnetic fields. The orbit of the electron is found to spiral down inside the cube in plane parallel to the x-y plane. Suggest a configuration of fields E and B that can lead to it.
Answer:
Since the orbit of the electron is spiral down inside the cube in plane parallel to x-y plane, so, B must be along z-axis i.e. \(\overrightarrow{\mathrm{B}}=\mathrm{B}_0 \hat{k}\) and electric field should be along x-axis i.e. \(\overrightarrow{\mathrm{E}}=\mathrm{E}_0 \hat{i}\), when E₀ > 0.

Question 4.17.
Do magnetic fields obey Newton's third law? Verify for two current elements \( \overrightarrow{d l_1}=d l \hat{i}\)  located at the origin and \(\overrightarrow{d l_2}=d l \hat{j}\) located at (0, R, 0). Both carry current I.
Answer:
Since the current element I\(\overrightarrow{d l_1}\) is lying at origin along X-axis and I\(\overrightarrow{d l_2}\) is lying at a distance R from I\(\overrightarrow{d l_1}\) and directed along y-axis.
So magnetic field due to I\(\overrightarrow{d l_1}\), will be along z-axis

Now magnetic field of I\(\overrightarrow{d l_2}\) at the location of current element I\(\overrightarrow{d l_1}\) is zero, so force on I\(\overrightarrow{d l_1}\) sue to I\overrightarrow{d l_2} is zero.
So, magnetic forces do not obey Newton's third law

Question 4.18.
A multirange voltmeter can be constructed by using a galvanometer circuit as shown in Fig. EP 4.18. We want to construct a voltmeter that can measure 2V, 20V and 200V using a galvanometer of resistance 10 Ω and that produces maximum deflection for current of 1 mA. Find R₁, R₂ and R₃ that have to be used.

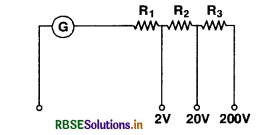
Answer:
Given G = 10 Ω, I_g = 1mA = 10^-3 A
(i) For V = 2 volt, Rg = ?
∴ R₁ = \(\frac{\mathrm{V}}{\mathrm{I}_g}\) - G
= \(\frac{2}{10^{-3}}\) - 10 = 1990 Ω = 2 kΩ

(ii) For V = 20 volt, R₂ = ?
R₁ + R₂ = \(\frac{\mathrm{V}}{\mathrm{I}_0}\) - G
= \(\frac{20}{10^{-3}}\) - 10 = 20,000 - 10 = 20000 = 20 kΩ
∴ R₂ = 20 - R₁ = 20 - 2 = 18 kΩ

(iii) For 200V, R₃ = ?
R₁ + R₂ + R₃ = \(\frac{200}{10^{-3}}\) - 10 = 200 kΩ
∴ R₃ = 200 - 20 = 180 kΩ

Question 4.19.
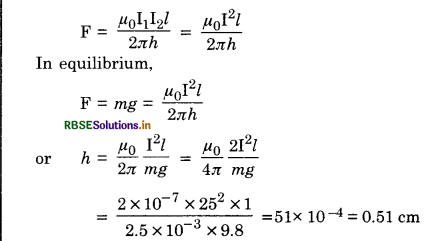
A long straight wire carrying current of 25A rests on a table as shown in Fig. EP 4.19. Another wire PQ of length 1m, mass 2.5 g carries the same current but in the opposite direction. The wire PQ is free to slide up and down. To what height will PQ rise?

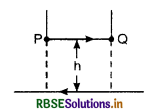
Answer:
Given I₁ = I2 = 25A, l = 1m, m = 2.5g = 2.5 x 10^-3 kg
Magnetic field due to straight current carrying wire at distance h is given by
B = \(\frac{\mu_0 I_1}{2 \pi h}\)
And magnetic force on small conductor

Long Answer Type Questions

Question 4.20.
A 100 turn rectangular coil ABCD (in XY plane) is hung from one arm of a balance Fig. EP 4.20. A mass 500 g is added to the other arm to balance the weight of the coil. A current 4.9A passes through the coil and a constant magnetic field of 0.2T acting inward (in xz plane) is switched on such that only arm CD of length 1 cm lies in the field. How much additional mass 'm' must be added to regain the balance?

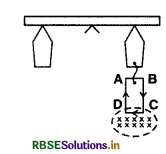
Answer:
When the magnetic field is off, Στ = 0 i.e. moment of force in both arms of the balance is the same 
i.e. Mgl = W_coil.l
or W_coil = Mg .....................(i)
When magnetic field is switched on, let additional mass m be placed to regain the balance of the beam.
Then Mgl + mgl = W_coil l + IBL sin 90°.l
or Mg + mg = W_coil + IBL
or Mg + mg = Mg + IBL
Using eqn. (i), we get
m = \(\frac{\text { IBL }}{g}=\frac{4.9 \times 0.2 \times 1 \times 10^{-2}}{9.8}\) = 10^-3 kg = 1g

Question 4.21.
An electron and a positron are released from (0, 0, 0) and (0, 0, 1.5R) respectively, in a uniform magnetic field B = B0\hat{i}, each with an equal momentum of magnitude p = e Br. Under what conditions on the direction of momentum will the orbits be non-intersecting circles?
Answer:
Since  \(\overrightarrow{\mathrm{B}}\left(=\mathrm{B}_0 \hat{i}\right)\)  is along x-axis. For circular orbit the momentum of electron and positron are in X-Y-plane. Let  \(\vec{p}_1\)  and \vec{p}_2 be the momentum of the electron and positron respectively. As the momentum of both the particles is the same, they define circles of opposite sense. Let  \(\vec{p}_1\)   make an angle θ with y-axis and  \(\vec{p}_2\)  also makes the same angle θ with y-axis. The centers of respective the circles must be perpendicular to the moment and at a distance R. Let the center of the electron be at C_e and that of positron at C_p.

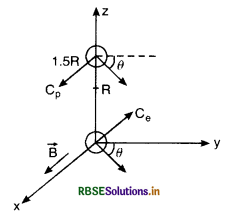
The coordinates of C_e is (0, -R sin θ, R cos θ)
The coordinates of C_p is (0, -R sin θ, \(\frac{3}{2}\)R - R cos θ).
The two circles will not overlap if the distance between the two centers are greater than 2R.
Let d be the distance between C_p and C_e
Then d² = (2R sin θ)² + (\(\frac{3}{2}\)R - 2R cos θ)²
= 4R² sin² θ + \(\frac{9}{4}\) R² - 6R² cos θ + 4R2 cos² θ
= 4R² + \(\frac{9}{4}\) R² - 6R² cos θ
Since d has to be greater than 2R
So d² > 4R²
∴ 4R² + \(\frac{9}{4}\) R² - 6R² cos θ > 4R²
or \(\frac{9}{4}\) > 6 cos θ
or cos θ < \(\frac{3}{8}\)

Question 4.22.
A uniform conducting wire of length 12a and resistance R is wound up as a current-carrying coil in the shape of (i) an equilateral triangle of side a; (ii) a square of sides a and, (iii) a regular hexagon of sides a. The coil is connected to a voltage source V₀. Find the magnetic moment of the coils in each case.
Answer:
(i) For an equilateral triangle n = 4

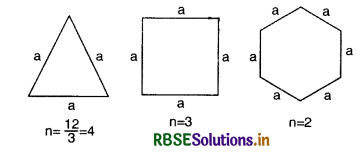
∴ Magnetic moment of the coil
M₁ = nI A = 4I \(\left(\frac{\sqrt{3}}{4} a^2\right)\) [∵ A = \(\frac{1}{2}\) x a x a sin 60° = \(\sqrt{\frac{3}{4}}\)a²]
or M₁ = Ia²\(\sqrt{3}\)

(ii) For a square, n = 3
∴ Magnetic moment of the coil
M₂ = nIA = 3I (a²) = 3Ia²

(iii) For a regular hexagonal of sides a, n = 2
Magnetic moment of the coil
M₃ = nI A = 2I \(\left(\frac{6 \sqrt{3}}{4} a^2\right)\) [∵ A = 6 x \(\frac{a}{2} \times \frac{a}{2} \tan 60^{\circ}=\frac{6 \sqrt{3}}{4} a^2\)]
or M₃ = 3\(\sqrt{3}\)Ia²

Question 4.23.
Five long wires A, B, C, D and E, each carrying current I are arranged to form edges of a pentagonal prism as shown in Fig. EP 4.23. Each carries current out of the plane of paper.
(a) What will be magnetic induction at a point on the axis O? Axis is at a distance R from each wire.
(b) What will be the field if current in one of the wires (say A) is switched off?
(c) What if current in one of the wires (say) A is reversed?

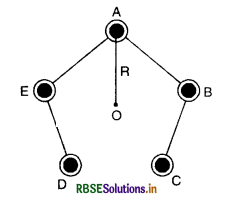
Answer:
(a) Let five wires A, B, C, D and E be perpendicular to the plane of paper at locations as shown in the figure.
Thus the magnetic induction at point O due to five wires will be represented by various sides of the closed pentagon in one order, lying in the plane of paper. So its resultant value is zero.

(b) Total magnetic field induction due to currents in wires B, C, D and E is equal and opposite to the magnetic field induction at O due to current through A
∴ Magnetic field induction at O due to current through wire A is B = \(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{I}}{\mathrm{R}}\) perpendicular to AO towards right.
When current of one wire is switched off.
B = \(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{I}}{\mathrm{R}}\) perpendicular to AO towards left.
(c) When current in wire (say) A is reversed, total magnetic induction at O
= Magnetic field induction due to wire A + magnetic field induction due to wire B, C, D and E
= \(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{I}}{\mathrm{R}}\) (⊥ to AO towards  left) + \(\frac{\mu_0}{4 \pi} \frac{2 \mathrm{I}}{\mathrm{R}}\) (⊥ to AO towards  left) 
= \(\frac{\mu_0 \mathrm{I}}{\pi \mathrm{R}}\) (⊥ to AO towards  left)