RBSE Solutions for Class 8 Maths Chapter 6 Square and Square Roots Intext Questions

Rajasthan Board RBSE Solutions for Class 8 Maths Chapter 6 Square and Square Roots Intext Questions Textbook Exercise Questions and Answers.

RBSE Class 8 Maths Solutions Chapter 6 Square and Square Roots Intext Questions

(Try These Page No: 90)

Class 8 Maths Square And Square Roots Try These Solutions Question 1.
Find the perfect square numbers between
(i) 30 and 40
Answer:
5 × 5 = 25, 6 × 6 = 36 and 7 × 7 = 49
Hence 36 is a perfect square number lying between 30 and 40.

(ii) 50 and 60
Answer:
7 × 7 = 49 and 8 × 8 = 64
Hence there is no perfect square number lying between 50 and 60.

(Try These Page No: 90)

Class 8 Maths Try These Solutions Chapter 6 Question 1.
Can we say whether the following numbers are perfect squares? How do we know?
(i) 1057
(ii) 23453
(iii) 7928
(iv) 222222
(v) 1069
(vi) 2061
Write five numbers which you can decide by looking at their one’s digit that they are not square numbers.
Answer:
(i) Here 1057 ends with 7 and we know that perfect square never ends with 2, 3, 7 or 8. Lie at unit’s places. So 1057 is not a perfect square.

(ii) Here 23453 ends with 3, which is not one of 0, 1, 4, 5, 6 or 9. Therefore 23453 cannot be a perfect square.

(iii) 7928 does not end with 0, 1, 4, 5, 6 or 9, so it cannot be a perfect square.

(iv) Since 222222 does not end with 0, 1, 4, 5, 6 or 9, so it cannot be a perfect square.

(v) ∵ 1069 ends with 9, so it may or may not be a perfect square. But
32² = 32 × 32 = 1024
and 33² = 33 × 33 = 1089
∴ 1069 is not a perfect square.

(vi) 2061 ends with 1, so it may or may not be a perfect square number.
45² = 45 × 45 = 2025
and 46² = 46 × 46 = 2116
∴ 2061 is not a perfect square. Five numbers which we can decide by looking at their one’s digit that they are not square numbers are 22, 43, 57, 268 and 193.

Class 8 Maths Ch 6 Try These Question 2.
Write five numbers which you cannot decide just by looking at their unit’s digit (or one’s place) whether they are square numbers or not.
Answer:
Five numbers, which we cannot decide whether they are square numbers just by looking at the unit’s digit are 1331, 2744, 3375, 17576 and 24389.

(Page No: 91)

Question 1.
Which of 123², 77², 82², 161², 109² would end with digit 1?
Answer:
We know that if a number has 1 or 9 in the unit’s place then its square ends in 1. Therefore 161² and 109² would end with digit 1.

(Try These Page No: 91)

Try These Solutions Class 8 Chapter 6 Question 1.
Which of the following numbers would have digit 6 at unit place.
(i) 19²
Answer:
Since 19 does not end with 4 or 6, so 19² will not have 6 in it’s unit’s place.

(ii) 24²
Answer:
Since 24 ends with 4, so 24² will have 6 in it’s unit’s place.

(iii) 26²
Answer:
Since 26 ends with 6, so 26² will have 6 in its unit’s place.Square and Square Roots

(iv) 36²
Answer:
Since 36 ends with 6, so 36² will have 6 in its unit’s place.

(v) 34²
Answer:
Since 34 ends with 4, so 34² will have 6 in its unit’s place.

(Page No: 92)

Class 8 Maths Chapter 6 All Try These Solutions Question 1.
What will be the “one’s digit” in the square of the following numbers?
(i) 1234
(ii) 26387
(iii) 52698
(iv) 99880
(v) 21222
(vi) 9106
Answer:

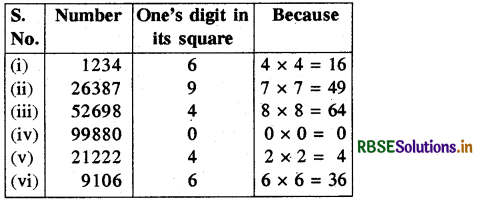
(Page No: 92)

Try These Solutions Class 8 Maths Chapter 6 Question 1.
The square of which of the following numbers would be an odd number/an even number? Why?
(i) 727
Answer:
Since 727 is an odd number, so its square is also odd.

(ii) 158
Answer:
Since 158 is an even number, so its square is also even.

(iii) 269
Answer:
Since 269 is an odd number, so its square is also odd.

(iv)1980
Answer:
(iv) Since 1980 is an even number, so its square is also even.

Question 2.
What will be the number of zeros in the square of the following numbers?
(i) 60
Answer:
60² will have two zeros.

(ii) 400
Answer:
400² will have four zeros.

(Try These Page No: 94)

Class 8 Ch 6 Try These Question 1.
How many natural numbers lie between 9² and 10² ? Between 11² and 12²?
Answer:
There are 18 (i.e. 2 × 9) natural" numbers between 9² and 10².
There are 22 (i.e. 2 × 11) natural numbers between 11² and 12².

Class 8 Maths Chapter 6 Try These Page No 94 Question 2.
How many non square numbers lie between the following pairs of numbers.
(i) 100² and 101²
(ii) 90² and 91²
(iii) 1000² and 1001²
Answer:
(i) There are 200 (i.e. 2 × 100) non-square numbers between 100² and 101².
(ii) There are 180 (i.e. 2 × 90) non-square numbers between 90² and 91².
(iii) There are 2000 (2 × 1000) non-square numbers between 1000² and 1001².

(Try These Page No: 94)

Find whether each of the following numbers is a perfect square or not?
(i) 121
(ii) 55
(iii) 81
(iv) 49
(v) 69
Answer:
(i) Given number is 121. We subtract 1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21 from it successively.
121 - 1 = 120; 120 - 3 = 117; 117 - 5= 112; 112 - 7 = 105; 105 - 9 = 96; 96 - 11 = 85; 85 - 13 = 72; 72 - 15 = 57; 57 - 17 = 40; 40 - 19 = 21; 21 - 21 = 0 This shows, 121 = 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15 + 17 + 19 + 21
∴ 121 is a perfect square.

(ii) We subtract 1, 3, 5, 7, 9, 11, 13, 15 from 55 successively.
55 - 1 = 54; 54 - 3 = 51; 51 - 5 = 46; 46 - 7 = 39; 39 - 9 = 30; 30 - 11 = 19; 19 - 13 = 6; 6 - 15 = - 9
This shows that 55 cannot be expressed as the sum of odd numbers starting from 1.

(iii) We subtract 1, 3, 5, 7, 9, 11, 13, 15, 17 from 81 successively.
81 - 1 = 80; 80 - 3 = 77; 77 - 5 = 72; 72 - 7 = 65; 65 - 9 = 56; 56 - 11 = 45; 45 - 13 = 32; 32 - 15 = 17; 17 - 17 = 0
This shows 81 = 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15 + 17 ;
∴ 81 is a perfect square.

(iv) We subtract 1, 3, 5, 7, 9, 11, 13 from 49 successively.
49 - 1 = 48; 48 - 3 = 45; 45 - 5 = 40; 40 - 7 = 33; 33 - 9 = 24; 24 - 11 = 13; 13 - 13 = 0
This means, 49 = 1 + 3 + 5 + 7 + 9+11 + 13
∴ 49 is a perfect square.

(v) We subtract 1, 3, 5, 7, 9, 11, 13, 15, 17, 19 from 69 successively.
69 - 1 = 68; 68 - 3 = 65; 65 - 5 = 60; 60 - 7 = 53; 53 - 9 = 44; 44 - 11 = 33; 33 - 13 = 20; 20 - 17 = 3; 3 - 19 = - 16
This shows that 69 cannot be expressed as the sum of odd numbers starting from 1.
Therefore 69 is not a perfect square.

(Page No: 95)

Class 8 Maths Chapter 6 Try These Solutions Question 1.
Express the following as the sum of two consecutive integers.
(i) 21²
Answer:
(i) We know that
n² = \(\frac{n^{2}-1}{2}+\frac{n^{2}+1}{2}\)
∴ 21² = 441

(ii) 13²
Answer:
13² = 169
= \(\frac{13^{2}-1}{2}+\frac{13^{2}+1}{2}\)
= \(\frac{169-1}{2}+\frac{169+1}{2}\)
= 85 + 84

(iii) 11²
Answer:
Similarly
11² = 121 = 60 + 61

(iv) 19²
Answer:
19² = 361 = 180 + 181

Class 8 Maths Chapter 6 Try These Question 2.
Do you think the reverse is also true; i.e., is the sum of any two consecutive positive integers is perfect square of a number? Give example to support your answer.
Answer:
No, the reverse is not always true.
4 + 5 = 9 = 3²
but 5 + 6 = 11 is not a perfect square.

(Try These Page No: 95)

Squares And Square Roots Class 8 Try These Solutions Question 1.
Write the square, making use of the pattern given below :
1² = 1
11² = 1 2 1
111² = 1 2 3 2 1
1111² = 1 2 3 43 2 1
11111² = 1 2 3 4 5 4 3 2 1
11111111² = 1 2 3 4 5 6 7 8 7 6 5 4 3 2 1
Answer:
(i) 111111² = 1 2 3 4 5 6 5 4 3 2 1
(ii) 1111111² = 1 2 3 4 5 6 7 6 5 4 3 2 1

(Page No: 95)

Chapter 6 Maths Class 8 Try These Solutions Question 1.
Can you find the square of the following numbers using the pattern given below :
(i) 6666667²
(ii) 66666667²
Pattern—
7² = 49
67² = 4489
667² = 444889
6667² = 44448889
66667² = 4444488889
666667² = 444444888889
Answer:
(i) 6666667² = 44444448888889
(ii) 66666667² = 4444444488888889

(Try These Page No: 97)

Square And Square Roots Class 8 Try These Solutions Question 1.
Find the squares of the following numbers containing 5 in unit’s place.
(i) 15
Answer:
15² = (1 × 2) Hundreds + 25
= 200 + 25 = 225

(ii) 95
Answer:
95² = (9 × 10) Hundreds + 25
= 9000 + 25 = 9025

(iii) 105
Answer:
105² = (10 × 11) Hundreds + 25
= 11000 + 25 = 11025

(iv) 205
Answer:
205² = (20 × 21) Hundreds + 25
= 42000 + 25 = 42025

(Try These Page No: 99)

Square And Square Roots Class 8 Try These Question 1.
(i) 11² = 121. What is the square root of 121?
(ii) 14² = 196. What is the square root of 196?
Answer:
(i) ∵ 11² = 121, ∴ Square root of 121 is 11.
(ii) Square root of 196 is 14.

(Think, Discuss And Write Page No: 99)

Class 8 Square And Square Roots Try These Question 1.
(-1)² = 1. Is - 1 a square root of 1?
Answer:
Yes. - 1 is a square root of 1.

Class 8 Chapter 6 Try These Question 2.
(- 2)² = 4. Is - 2 a square root of 4?
Answer:
Yes, - 2 is a square root of 4.

Try These Square And Square Roots Class 8 Question 3.
(- 9)² = 81. Is - 9 a square root of 81?
Answer:
Yes, - 9 is a square root of 81.

(Try These Page No: 100)

Question 1.
By repeated subtraction of odd numbers starting from 1, find whether the following numbers are perfect squares or not? If the number is a perfect square then find its square root.
(i) 121
(ii) 55
(iii) 36
(iv) 49
(v) 90
Answer:
(i) From 121, we subtract successive odd numbers starting from 1, as below :
121 - 1 = 120
120 - 3 = 117
117 - 5 = 112
112 - 7 = 105
105 - 9 = 96
96 - 11 = 85
85 - 13 = 72
72 - 15 = 57
57 - 17 = 40
40 - 19 = 21
21 - 21 = 0
We obtain 1 to 11th step, and were able to express 121 as the sum of consecutive odd numbers starting from 1. So 121 is a perfect square and √121 = 11.

(ii) From 55, we subtract successive odd numbers starting from 1, as below :
55 - 1 = 54
54 - 3 = 51
51 - 5 = 46
46 - 7 = 39
39 - 9 = 30
30 - 11 = 19
19 - 13 = 6
6 - 15 =- 9
This shows that we are not able to express 55 as the sum of consecutive odd numbers starting from 1.
∴ 55 is not a perfect square.

(iii) From 36, we subtract successive odd numbers starting from 1, as below :
36 - 1 = 35
35 - 3 = 32
32 - 5 = 27
27 - 7 = 20
20 - 9 = 11
11 - 11 =0
We obtain 1 to 6th step and were able to express 36 as the sum of consecutive odd numbers starting from 1. So 36 is a perfect square and √36 = 6.

(iv) From 49, we subtract successive odd numbers starting from 1, as below :
49 - 1 = 48
48 - 3 = 45
45 - 5 = 40
40 - 7 = 33
33 - 9 = 24
24 - 11 = 13
13 - 13 = 0
We obtain 1 to 7th step and were able to •express 49 as the sum of consecutive odd numbers starting from 1. So 49 is a perfect square and √49 = 7.

(v) From 90, we subtract successive odd numbers starting. from 1, as below :
90 - 1 = 89
89 - 3 = 86
86 - 5 = 81
81 - 7 = 74
74 - 9 = 65
65 - 11 = 54
54 - 13 = 41
41 - 15 = 26
26 - 17 = 9
9 - 19 = -10
This shows that we are not able to express 90 as die sum of consecutive numbers starting from 1. So, 90 is not a perfect square.

(Think, Discuss and Write Page No: 103)

Question 1.
Can we say that if a perfect square is of m-digits, then its square root will have \(\frac{n}{2}\) digits if n is even or \(\frac{(n+1)}{2}\) if n is odd?
Answer:
Yes, it is true.
For Example:
(i) 529 Here n = 3 (odd)
∴ \(\frac{n+1}{2}\) = \(\frac{3+1}{2}\) = 2
and we know that
√529 = 23 (2 digits)

(ii) 1296 Here n = 4 (even)
∴ \(\frac{n}{2}\) = \(\frac{4}{2}\) = 2
and we know that
√1296 = 36 (2 digits)

(Try These Page No: 105)

Question 1.
Without calculating square roots, find the number of digits in the square root of the following numbers.
(i) 25600
(ii) 100000000
(iii) 36864
Answer:
(i) Since 25600 consists of 5 digits, so the number of digits in √25600 is \(\frac{5+1}{2}\), i.e. 3 digits.
(ii) Since 100000000 consists of 9 digits, so its square root will have \(\frac{9+1}{2}\), i.e. 5 digits.
(iii) Since 36864 consists of 5 digits, so its square root will have \(\frac{5+1}{2}\), i.e. 3 digits.

(Try These Page No: 107)

Question 1.
Estimate the value of the following to the nearest whole number.
(i) √80
(ii) √1000
(iii) √350
(iv) √500
Answer:
(i) √80
We know that 64 < 80 < 81
⇒ 8 < √80 < 9
∵ 81 is much closer to 80 than 64
∴ √80 is nearer to 9.

(ii) √1000
We know that 31² = 961 and 32² = 1024
∴ 31 < √1000 < 32
∵ 1000 is much closer to 1024 than 961.
∴ √1000 is nearer to 32.

(iii) √350
We know that 182 = 324 and 192 = 361
∴ 18 < √350 < 19
Since 350 is much closer to 361 than 324
∴ √350 is nearer to 19.

(iv) √500
We know that 222 = 484 and 232 = 529
∴ 22 < √500 < 23
∵ 500 is much closer to 484 than 529
∴ √500 is nearer to 22.