RBSE Solutions for Class 8 Maths Chapter 15 Introduction to Graphs Ex 15.1

Rajasthan Board RBSE Solutions for Class 8 Maths Chapter 15 Introduction to Graphs Ex 15.1 Textbook Exercise Questions and Answers.

RBSE Class 8 Maths Solutions Chapter 15 Introduction to Graphs Ex 15.1

Question 1.
The following graph shows the temperature of a patient in a hospital, recorded every hour.

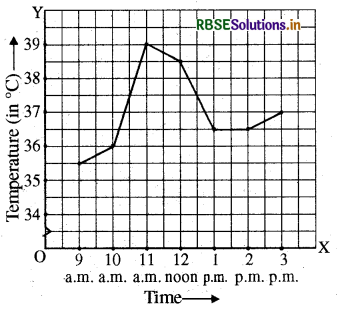
(a) What was the patient’s temperature at 1 p.m. ?
Answer:
In the graph, the time are represented on the x-axis and the temperature (in °C) are represented on the y-axis. The temperature at any time can be read from the graph exactly in the same way as we read the coordinates of a point.

From the graph, we observe that:
The patient’s temperature at 1 p.m. was 36.5°C.

(b) When was the patient’s temperature 38.5°C?
Answer:
The temperature of patient was 38.5°C at 12 noon.

(c) The patient’s temperature was the same two times- during the period given. What were these two times?
Answer:
The patient’s temperature was same at 1 p.m. to 2 p.m.

(d) What was the temperature at 1.30 p.m.? How did you arrive at your answer?
Answer:
The temperature at 1.30 p.m. was 36.5°C because the temperature between 1 p.m. and 2 p.m. is same. Also, graphs between 1 p.m. and 2 p.m. is a straight line parallel to jc-axis. Similarly, the point on the y-axis, between 36°C and 37°C will represent 36.5°C.

(e) During which periods did the patients’ temperature showed an upward trend?
Answer:
During the periods 9 a.m. to 10 a.m., 10 a.m. to 11 a.m. and 2 p.m. to 3 p.m. the patient’s temperature showed on upward trend.

Question 2.
The following line graph shows the yearly sales figures for a manufacturing company.

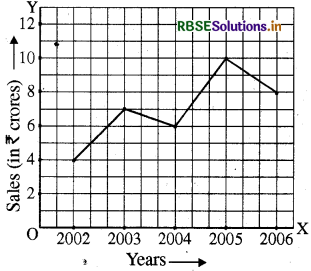
(a) What were the sales in (i) 2002 (ii) 2006?
Answer:
(i) Sales in 2002 = 4 crores
(ii) Sales in 2006 = 8 crores

(b) What were the sales in (i) 2003 (ii) 2005?
Answer:
(i) Sales in 2003 = 7 crores
(ii) Sales in 2005 = 10 crores

(c) Compute the difference between the sales in 2002 and 2006.
Answer:
The difference between the sale of 2002 and 2006 = 8 - 4 = 4 crores.

(d) In which year was there the greatest difference between the sales as compared to its previous year?
Answer:
The greatest difference between the sales of two consecutive years was 2004 and 2005. i.e. 2005.

Question 3.
For an experiment in Botany, two different plants, plant A and plant B were grown under similar laboratory conditions. Their heights were measured at the end of each week for 3 weeks. The results are shown by the following graph.

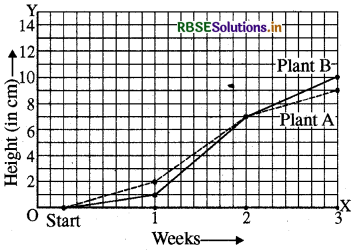
(a) How high was Plant A after (i) 2 weeks
(ii) 3 weeks?
Answer:
(i) Height of Plant A after 2 weeks = 7 cm
(ii) Height of Plant A after 3 weeks = 9 cm

(b) How high was Plant B after (i) 2 weeks (ii) 3 weeks?
Answer:
(i) Height of Plant B after 2 weeks = 7 cm
(ii) Height of Plant B after 3 weeks =10 cm

(c) How much did Plant A grow during the 3rd week?
Answer:
The plant A grows during the 3rd week = 9 - 7=2 cm

(d) How much did Plant B grow from the end of the 2nd week to the end of the 3rd week?
Answer:
The plant B grows from the end of the 2nd week to the end of the 3rd week
= 10 cm - 7 cm = 3 cm.

(e) During which week did Plant A grow most?
Answer:
2nd week

(f) During which week did Plant B grow least?
Answer:
First week

(g) Were the two plants of the same height during any week-shown here? Specify.
Answer:
Both the plants have shown the same height at the end of 2nd week.

Question 4.
The following graph shows the temperature forecast and the actual temperature for each day of a week.

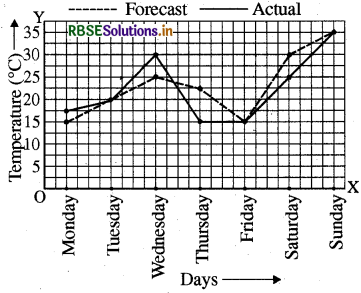
(a) On which days was the forecast temperature the same as the actual temperature?
Answer:
Tuesday, Friday and Sunday.

(b) What was the maximum forecast temperature during the week?
Answer:
Sunday (35°C)

(c) What was the minimum actual temperature during the week?
Answer:
15°C

(d) On which day did the actual temperature differ the most from the forecast temperature?
Answer:
The actual temperature differed die most from the forecast temperature on Thursday.

Question 5.
Use the tables below to draw linear graphs.
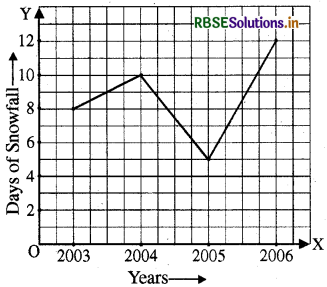
(a) The number of days a hill side city received snow in different years.

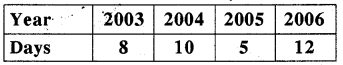
Answer:
To draw the required graph, we represent years on the x-axis and the days on the y-axis. We plot the ordered pairs (2003, 8), (2004, 10), (2005, 5) and (2006, 12) as points and then join them by line segments as shown below :

(b) Population (in thousands) of men and women in a village in different years.

Answer:
To draw the required graph, we represent years on the x-axis and the population (in thousands) on the y-axis. The dotted line shows the population (in thousands) of men and the solid line shows the population (in thousands) of women.

First, we plot the ordered pairs (2003, 12), (2004, 12.5), (2005, 13), (2006, 13.2) and (2007, 13.5) and then join diem by the dotted line as shown to get the graph representing the number of men. Furthem, we plot (2003, 11.3), (2004, 11.9), (2005, 13), (2006, 13.6) and (2007, 12.8) and then join them by line segments as shown to get the graph representing the graph of number of women. Hence, we get the required graph.

Question 6.
A courier-person cycles from a town to a neigh-bouring suburban area to deliver a parcel to a merchant. His distance from the town at different times is shown by the following graph :

(a) What is the scale taken for the time axis?
Answer:
4 small division = 1 hour let the 4 small divisions = 1 cm
1 cm = 1 hour
i.e., 4 units = 1 hour

(b) How much time did the person take for the travel?
Answer:
3 hours 30 minutes or 3.30 hours

(c) How far is the place of the merchant from the town?
Answer:
22 km

(d) Did the person stop on his way? Explain.
Answer:
Yes; this is indicated by the horizontal part of the graph (10 a.m.-10.30 a.m.)

(e) During which period did he ride fastest? 
Answer:
Between 8 a.m. to 9 a.m.

Question 7.
Can there be a time-temperature graph as follows? Justify your answer.
(i)

Answer:
Yes, this represents a time- temperature graph because it represents a smooth rise in temperature and is represented by a line graph.

(ii)

Answer:
Yes, this represents a time-temperature graph because it represent a smooth fall in temperature and is represented by a line graph.

(iii)

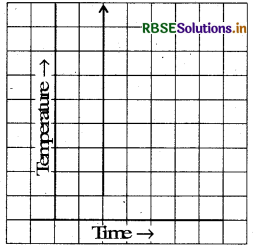
Answer:
No, there are infinite number of temperature at the same time which is not possible.

(iv)

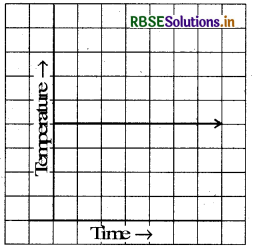
Answer:
Yes, temperature can be made for a period of time.
Thus, case (iii) does not represent a time- temperature graph.