RBSE Solutions for Class 7 Maths Chapter 8 Comparing Quantities Intext Questions

Rajasthan Board RBSE Solutions for Class 7 Maths Chapter 8 Comparing Quantities Intext Questions Textbook Exercise Questions and Answers.

RBSE Class 7 Maths Solutions Chapter 8 Comparing Quantities Intext Questions

(Think, Discuss and Write - Page 157)

Class 7 Maths Comparing Quantities Try These Solutions Question 1.
An ant can carry 50 times its weight. If a person can do the same, how much would you be able to carry?
Answer:
I would carry 50 times my weight.

(Try These - Page 158)

Class 7 Comparing Quantities Try These Question 1.
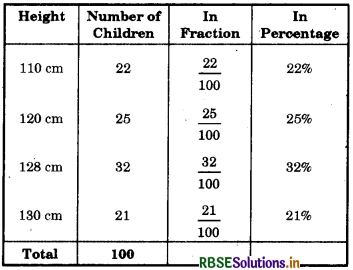
Find the percentage of children of different heights for the following data.
 
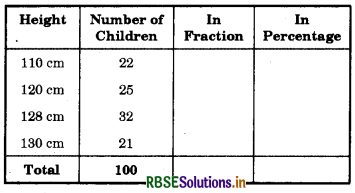
Answer:

Class 7 Maths Ch 8 Try These Question 2.
A shop has the following number of shoe pairs of different sizes :
Size 2 : 20
Size 3 : 30
Size 4 : 28
Size 5 : 14
Size 6 : 8
Write this information in tabular form as done earlier and find the percentage of each shoe size available in the shop.
Answer:

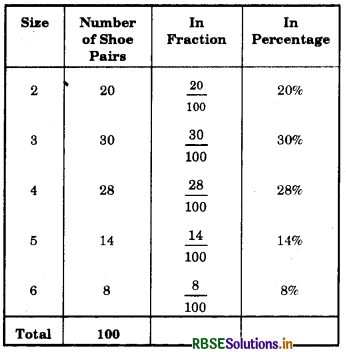
(Try These - Page 159-160)

Try These Solutions Class 7 Chapter 8 Question 1.
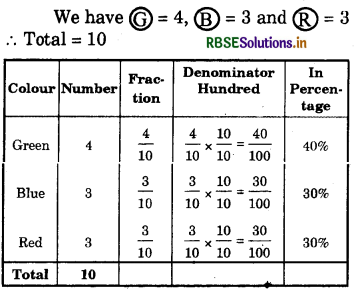
A collection of 10 chips with different colours is given.

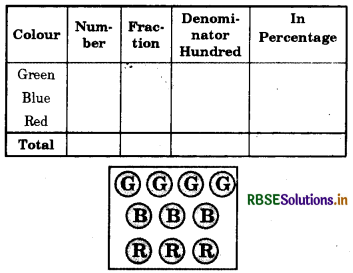
Fill the table and find the percentage of chips of each colour.
Answer:

Class 7 Maths Chapter 8 Try These Question 2.
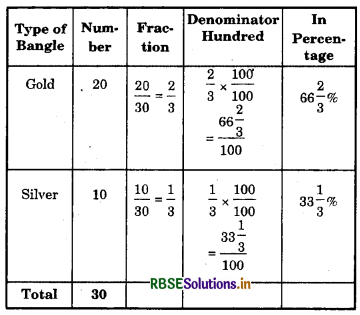
Mala has a collection of bangles. She has 20 gold bangles and 10 silver bangles. What is the percentage of bangles of each type? Can you put it in the tabular form as done in the above example?
Answer:
We have :

(Think, Discuss and Write - Page 160)

Try These Comparing Quantities Class 7 Question 1.
Look at the examples below and in each of them discuss which is better for comparison.
1. In the atmosphere 1 g of air contains:

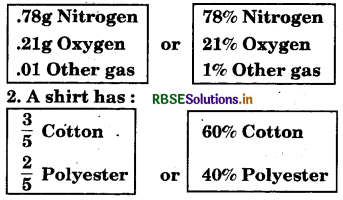
Answer:
Percentage is better for comparison in both the cases.

(Think, Discuss and Write - Page 161)

Class 7 Maths Chapter 8 Try These Solutions Question 1.
(i) Can you eat 50% of a cake? Can you eat 100% of cake? Can you eat 150% of a cake?
(ii) Can a price of an item go up by 50%? Can a price of an item go up by 100%? Can a price of an item go up by 150%?
Answer:
(i) Yes, we can eat 50% of a cake.
Yes, we can eat 100% of a cake. 
No, we cannot eat 150% of a cake,

(ii) Yes, price of an item can go up by 50%.
Yes, price of an item can go up by 100%.
Yes, price of an item can go up by 150%.

(Try These - Page 161)

Question 1.
Convert the following to percen tages:
(a) \(\frac{12}{16}\)
Answer:
\(\frac{12}{16}=\frac{12}{16}\) × 100% = 75%

(b) 3.5
Answer:
3.5 = 3.5 × 100% = \(\frac{35}{10}\) × 100% = 350%

(c) \(\frac{49}{50}\)
Answer:
\(\frac{49}{50}\) = \(\frac{49}{50}\) × 100 = 98%

(d) \(\frac{2}{2}\)
Answer:
\(\frac{2}{2}\) = \(\frac{2}{2}\) × 100 = 100%

(e) 0.05
Answer:
0.05 = 0.05 × 100% = \(\frac{5}{100}\) × 100 = 5%

Question 2.
(i) Out of 32 students, 8 are absent. What percent of the students are absent?
Answer:
\(\frac{8}{32}\) × 100% = 25% are absent.

(ii) There are 25 radios, 16 of them are out of order. What percent of radios are out of order?
Answer:
\(\frac{16}{25}\) × 100% = 64% out of order.

(iii) A shop has 500 items, out of which 5 are defective. What percent are defective?
Answer:
\(\frac{5}{500}\) × 100% = 1% are defective. 

(iv) There are 120 voters, 90 of them voted yes. What percent voted yes?
Answer:
\(\frac{90}{120}\) × 100 = 75% voted yes.

(Try These - Page 162)

Try These Solutions Class 7 Comparing Quantities Question 1.
35% + _______________ % = 100%,
64% + 20% + _______________% = 100%
45% = 100% - _______________%,
70% = _______________ % - 30%
Answer:
35% + 65% = 100% [∵ 100 - 35 = 65]
64% + 20% + 16% = 100% [∵ 64 + 20 = 84 and 100 - 84 = 16]
45% = 100% - 55% [∵ 100 - 45 = 65]
70% = 100% - 30% [∵ 70 + 30 = 100]

Comparing Quantities Class 7 Try These Solutions Question 2.
If 65% of students in a class have a bicycle, what percent of the student do not have bicycles?
Answer:
100% - 65% = 35% of students do not have bicycles.

Maths Class 7 Chapter 8 Try These Question 3.
We have a basket full of apples, oranges and mangoes. If 50% are apples, 30% are oranges, then what percent are mangoes?
Answer:
100% - (50% + 30%) = 100% - 80%
= 20% are mangoes.

(Try These - Page 163)

Class 7 Chapter 8 Try These Question 1.
What percent of these figures are shaded?

You can make some more figures yourself and ask your friends to estimate the shaded parts.
Answer:
(i) Fraction of shaded part 
= \(\frac{1}{4}+\frac{1}{4}+\frac{1}{4}=\frac{3}{4}\)
Percentage of shaded part
= \(\frac{3}{4}\) × 100% = 75%

(ii) Fraction of shaded part
= \(\frac{1}{4}+\frac{1}{8}+\frac{1}{8}=\frac{2+1+1}{8}=\frac{1}{2}\)
Percentage of shaded part
= \(\frac{1}{2}\) × 100% = 50%

(Try These - Page 164)  

Question 1.
Find:
(a) 50% of 164
Answer:
50% of 164 = \(\frac{50}{100}\) × 164 = 82

(b) 75% of 12
Answer:
75% of 12 = \(\frac{75}{100}\) × 12 = 9

(c) 12\(\frac{1}{2}\)% of 64
Answer:
12\(\frac{1}{2}\)% of 64 = \(\frac{25}{2}\)% of 64
= \(\frac{25}{2 \times 100}\) × 64 = 8

Question 2.
8% children of a class of 25 like getting wet in the rain. How many children like getting wet in the rain?
Answer:
Number of children who like wetting in rain = 8% of 25
= \(\frac{8}{100}\) × 25 = 2 children

(Try These - Page 164)

Question 1.
9 is 25% of what number?
Answer:
Let the required number be x; Then 25% of x = 9
⇒ \(\frac{25}{100}\) × x = 9
⇒ \(\frac{x}{4}\) = 9
⇒ x = 9 × 4
⇒ x = 36
∴ The required number is 36.

Question 2.
75% of what number is 15?
Answer:
Let the required number be x. 
Then 75% of x = 15
⇒ \(\frac{75}{100}\) × x = 15
⇒ \(\frac{3 x}{4}\) = 15
⇒ 3x = 4 × 15 ⇒ 3x = 60
⇒ x = \(\frac{60}{3}\) ⇒ x = 20
∴ The required number is 20.

(Try These - Page 166)

Question 1.
Divide 15 sweets between Manu and Sonu so that they get 20% and 80% of them respectively.
Answer:
Ratio of the shares of Manu and Sonu = 20 : 80 = 1 : 4
Sum of parts = 1 + 4 = 5
Share of Manu = \(\frac{1}{5}\) × 15 = 3 sweets
Share of Sonu = \(\frac{4}{5}\) × 15 = 12 sweets

Question 2.
If angles of a triangle are in the ratio 2:3:4. Find the value of each angle.
Answer:
The sum of measures of the three angles of a triangle is 180°.
Ratios of angles = 2 : 3 : 4 
∴ Sum of parts = 2 + 3 + 4 = 9
First angle = \(\frac{2}{9}\) × 180° = 40°
Second angle = \(\frac{3}{9}\) × 180° = 609
Third angle = \(\frac{4}{9}\) × 180° = 80°
The value of each angle is 40°, 60°, 80°.

(Try These - Page 167)

Class 7 Maths Chapter 8 Try These Solutions Page 167 Question 1.
Find percentage of increase or decrease:
(i) Price of shirt decreased from ₹ 280 to ₹ 210.
(ii) Marks in a test increased from 20 to 30.
Answer:
(i) Percentage decrease
= \(\frac{\text { Amount of change }}{\text { Original-amount }}\) × 100%
= \(\frac{280-210}{280}\) × 100%
= \(\frac{70}{280}\) × 100% = 25%

(ii) Percentage increase
= \(\frac{\text { Amount of change }}{\text { Original amount }}\) × 100%
= \(\frac{30-20}{20}\) × 100% = \(\frac{10}{20}\) × 100% = 50%

Question 2.
My mother says, in her childhood petrol was ₹ 1 per litre. It is ₹ 52 per litre today. By what percentage has the price gone up?
Answer:
Percent increase
= \(\frac{\text { Amount of change }}{\text { Original amount }}\) × 100%
= \(\frac{52-1}{1}\) × 100% = \(\frac{51}{1}\) × 100 = 5100% 

(Try These - Page 169)

Try These Solutions Of NCERT Maths Class 7 Chapter 8 Page 169 Question 1.
A shopkeeper bought a chair for ₹ 375 and sold it for ₹ 400. Find the gain percentage.
Answer:
CP = ₹ 375
SP = ₹ 400
Profit = ₹ 400 - ₹ 375 = ₹ 25 Profit
Profit Percent = \(\frac{\text { Profit }}{\text { Cost Price }(\mathrm{CP})}\) × 100%
= \(\frac{25}{375}\) × 100% = 6\(\frac{2}{3}\)%

Class 7 Maths Try These Solutions Chapter 8 Question 2.
Cost of an item is ₹ 50. It was sold with a profit of 12%. Find the selling price.
Answer:
Profit = 12% of CP = 12% of ₹ 50
= \(\frac{12}{100}\) × 50 = ₹ 6
Selling Price = Cost Price + Profit
= ₹ 50 + ₹ 6 = ₹ 56

Class 7 Chapter 8 Maths Try These Question 3.
An article was sold for ₹ 250 with a profit of 5%. What was its cost price?
Answer:
SP = CP + Profit 
⇒ 250 = CP + 5% of CP
⇒ 250 = CP + \(\frac{5}{100}\) × CP 
⇒ 250 = CP + \(\frac{1}{20}\)CP
⇒ 250 = \(\frac{21}{20}\)CP
⇒ CP = \(\frac{250 \times 20}{21}\) = ₹ 238.10
∴ Cost price of an article is ₹ 238.10.

Comparing Quantities Try These Class 7 Question 4.
An item was sold for ₹ 540 at a loss of 5%. What was its cost price?
Answer:
SP = CP - Loss
⇒ 540 = CP - 5% of CP
⇒ 540 = CP - \(\frac{5}{100}\) × CP 
⇒ 540 = (1 - \(\frac{5}{100}\))CP
⇒ 540 = (1 - \(\frac{1}{20}\))CP = \(\frac{19}{20}\)CP
⇒ CP = \(\frac{540 \times 20}{19}\) = 568 \(\frac{8}{19}\)
∴ The cost price of the item is ₹ 568 \(\frac{8}{19}\)

(Try These - Page 170)

Try These Solutions Class 7 Maths Chapter 8 Question 1.
₹ 10,000 is invested at 5% interest rate p.a. Find the interest at the end of one year.
Answer:
Given, Principal (P) = ₹ 10,000
Rate (R) = 5% p.a.
Interest at the end of one year 
= \(\frac{P R}{100}=\frac{10,000 \times 5}{100}\) = ₹ 500

Chapter 8 Maths Class 7 Try These Solutions Question 2.
₹ 3,500 is given at 7% p.a. rate of interest. Find the interest which will be received at the end of two years.
Answer:
Given, P = ₹ 3,500, R = 7% p.a.,
T = 2 years
∴ Interest at the end of two years
PRT 3500x7x2 „
" 100 “ 100 -?490

Try These Solutions Class 7 Question 3.
₹ 6,050 is borrowed at 6.5% rate of interest p.a. Find the interest and the amount to be paid at the end of 3 years.
Answer:
Given, P = ₹ 6,050, R = ₹ 6.5% p.a.,
T = 3 years.
The interest to be paid at the end of 3 years
= \(\frac{P R T}{100}=\frac{6,050 \times 6.5 \times 3}{100}\) = 1,179.75
Amount to be paid at the end of 3 years
= ₹ 6,050 + ₹ 1,179.75 = ₹ 7,229.75

Try These Solutions Class 7 Ch 8 Question 4. 
₹ 7,000 is borrowed at 3.5% rate of interest p.a. borrowed for 2 years. Find the amount to be paid at the end of the second year.
Answer:
Given, P = ₹ 7,000, R = ₹ 3.5% p.a., T = 2 years.
Interest to be paid at the end of 2nd year
= \(\frac{P R T}{100}=\frac{7,000 \times 3.5 \times 2}{100}\) = ₹ 490
∴ Amount to be paid after 2nd year = ₹ 7,000 + ₹ 490 = ₹ 7,490

(Try These - Page - 171)

Question 1.
You have ₹ 2,400 in your account and the interest rate is 5%. After how many years would you earn ₹ 240 as interest ?
Answer:
Given, Principal (P) = ₹ 2400 Rate (R) = 5% p.a.
Simple Interest = ₹ 240
∵ S.I. = \(\frac{P \times R \times T}{100}\)
∴ 240 = \(\frac{2,400 \times 5 \times T}{100}\)
⇒ T = \(\frac{240 \times 100}{2,400 \times 5}\) = 2 Years
Hence, Interest of ₹ 240 will be obtained after 2 years.

Question 2.
On a certain sum the interest paid after 3 years is ₹ 450 at 5% rate of interest per annum. Find the sum.
Answer:
Given, Rate (R)= 5% p.a., Time (T) = 3 years, Interest = ₹ 450
∴ Interest = \(\frac{P \times R \times T}{100}\)
∴ 450 = \(\frac{P \times 5 \times 3}{100}\)
or P = \(\frac{450 \times 100}{5 \times 3}\) = 3,000
∴ The required sum (Principal) is ₹ 3000.