RBSE Solutions for Class 7 Maths Chapter 11 Perimeter and Area Intext Questions

Rajasthan Board RBSE Solutions for Class 7 Maths Chapter 11 Perimeter and Area Intext Questions Textbook Exercise Questions and Answers.

RBSE Class 7 Maths Solutions Chapter 11 Perimeter and Area Intext Questions

(Try These Page No: 205)

Question 1.
What would you need to find area or perimeter to answer the following?
1. How much space does a blackboard occupy?
Answer:
Area of blackboard.

2. What is the length of a wire required to fence a rectangular flower bed?
Answer:
Perimeter of flower bed.

3. What distance would you cover by taking two rounds of a triangular park?
Answer:
2 × Perimeter of park.

4. How much plastic sheet do you need to cover a rectangular swimming pool?
Answer:
Area of swimming pool.

(Try These Page No: 206)

Question 1.
Experiment with several such shapes and cut-outs. You might find it useful to draw these shapes on squared sheets and compute their areas and perimeters.
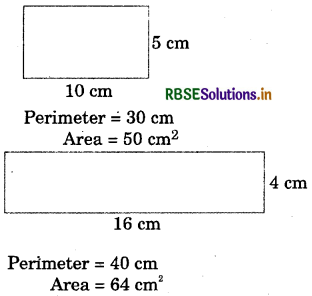
You have seen that increase in perimeter does not mean that area will also increase.
Answer:

Perimeter = 30 cm
Area = 56 cm²

From the above calculation of area and perimeter we find that increase of perimeter does not lead to increase in area.

Question 2.
Give two examples where the area increase as the perimeter increases.
Answer:
(i)

(ii)

Question 3.
Give two examples where the area does not increase when perimeter increases.
Answer:

(Try These Page No: 210)

Question 1.
Each of the following rectangles of length 6 cm and breadth 4 cm is composed of congruent polygons. Find the area of each polygon.
Answer:
Area of all rectangles = l × b = 6 × 4 cm² = 24 cm². Since, area of all congruent polygon are equal to each other.

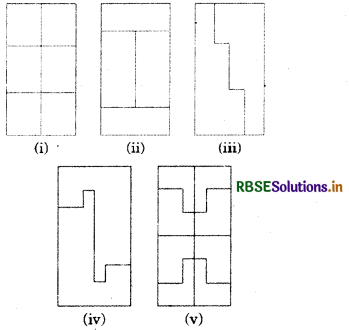
(i) Area of each polygon = (24 ÷ 6) cm²
= 4 cm²

(ii) Area of each polygon = (24 ÷ 4) cm²
= 6 cm²

(iii) Area of each polygon = (24 ÷ 2) cm²
= 12 cm²

(iv) Area of each polygon = (24 ÷ 2) cm²
= 12 cm²

(v) Area of each polygon = (24 ÷ 8) cm²
= 3 cm²

(Try These Page No: 212)

Question 1.
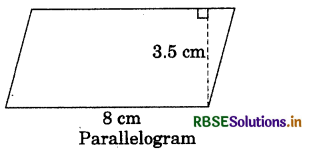
Find the area of following parallelograms:
(i)

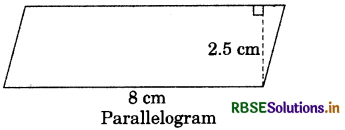
(ii)

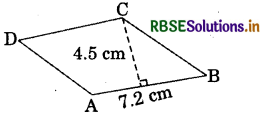
(iii) In parallelogram ABCD, AB 7.2 cm and the perpendicu1ar from C on AB is 4.5 cm.
Answer:

(i) Area of ||gm = base × height
= 8 × 3.5 = 28 cm²

(ii) Area of ||gm = base × height
= 8 × 2.5 = 20 cm²

(iii) Base = 7.2 cm, height = 4.5 cm,
∴ Area of the parallelogram ABCD
= base × height = 7.2 × 4.5 = 32.40 cm²

(Try These: Page No: 219)

Question 1.
(a) Which square has the larger perimeter (in fig.)?
(b) Which is larger, perimeter of smaller square or the circumference of the circle (in fig.)?

Answer:
(a) The outer square has the larger perimeter.
(b) The circumference of the circle is larger than the perimeter of smaller square.

(Try These: Page No: 222)

Question 1.
Draw circles of different radii on a graph paper. Find the area by counting the number of squares. Also find the area by using the formula. Compare the two values.
Answer:
(i)

Area by counting the number of squares = 16 cm²

Note:
Count 1 for half or more than half square.
Count 0 for less than half square.
Area by using the formula = \(\frac{22}{7}\) × (2.3)²
= 16.62 cm²
(Area of circle = πr²)

(ii)

Area by counting the number of squares = 60 cm²
Area by using the formula = \(\frac{22}{7}\) × (4.50)²
= 63.64 cm²
(Area of circle = πr²)

(Try These: Page No: 225)

Question 1.
Convert the following:
(i) 50 cm² in mm²
Answer:
50 cm² in mm²
1 cm² = 1 cm × 1 cm
= 10 mm × 10 mm
= 100 mm²
∴ 50 cm² = 100 × 50 mm²
= 5000 mm²

(ii) 2 ha in m²
Answer:
2 ha in m²
1 ha = 10000 m²
∴ 2 ha = 10000 × 2 m²
= 20000 m²

(iii) 10 m² in cm²
Answer:
10 m² in cm²
1 m² = 1 m × 1 m
= 100 cm × 100 cm
= 10000 cm²
∴ 10 m² = 10000 × 10 cm²
= 100000 cm²

(iv) 1000 cm² in m²
Answer:
1000 cm² in m²
1 cm² = \(\frac{1}{10000}\) m²
∴ 1000 cm² = 1000 × \(\frac{1}{10000}\)
= \(\frac{1}{10}\) = 0.1 m².