RBSE Solutions for Class 7 Maths Chapter 11 Perimeter and Area Ex 11.1

Rajasthan Board RBSE Solutions for Class 7 Maths Chapter 11 Perimeter and Area Ex 11.1 Textbook Exercise Questions and Answers.

RBSE Class 7 Maths Solutions Chapter 11 Perimeter and Area Ex 11.1

Question 1.
The length and the breadth of a rectangular piece of land are 500 m and 300 m respectively. Find:
(i) its area.
(ii) the cost of the land, if 1 m² of the land costs 10,000.
Answer:
(i) Length of rectangular piece of land = 500m
Breadth of rectangular piece of land = 300 m
Area = l × b = 500 × 300 m = 1,50,000 m²

(ii) Cost of 1 m² land = 10,000
Cost of 1,50,000 m² land
= ₹ 10000 × 1,50,000 = 1,50,00,00,000
So, the area of land is 150000 m² and its cost is ₹ 1,50,00,00,000.

Question 2.
Find the area of a square park whose perimeter is 320 m.
Answer:
Perimeter of square park = 320 m
Side = \(\frac{\text { Perimeter }}{4}\) = \(\frac{320 \mathrm{~m}}{4}\) = 80 m
Area of square park = (side)² = (80 m)²
= 6400 m²
So, the area of square park is 6400 m².

Question 3.
Find the breadth of a rectangular plot of land, if its area is 440 m² and the length is 22 m. Also find its perimeter.
Answer:
Area of rectangular land = 440 m2
Length = 22 m
Breadth = \(\frac{\text { Area }}{l}\) = \(\frac{440}{22}\) m = 20 m
Perimeter = 2 × (l + b)
= 2 × (22 + 20)
= 2 × 42 m = 84 m

So, the perimeter of land is 84 m.

Question 4.
The perimeter of a rectangular sheet is 100 cm. If the length is 35 cm, find its breadth. Also find the area.
Answer:
Perimeter of rectangular sheet = 100 cm
∵ Perimeter = 2 (length + breadth)
⇒ 100 = 2 (35 + 6)
⇒ 100 = 70 + 26
⇒ 26 = 100 - 70 = 30
∴ b = \(\frac{30}{2}\) = 15 cm
Area = l × b
= 35 × 15 cm (Length = 35 cm)
= 525 cm²
So, the breadth of sheet is 15 cm and its area is 525 cm².

Question 5.
The area of a square park is the same as of a rectangular park. If the side of the square park is 60 m and the length of the rectangular park is 90 m, find the breadth of the rectangular park.
Answer:
Side of square park = 60 m
Area = (Side)²
= (60 m)²
= 3600 m²
Since, it is given that area of square park is equal to the area of rectangular park.
Area of rectangular park = 3600 m²
Length of park = 90 m
b = \(\frac{\text { Area }}{l}\) = \(\frac{3600}{90}\) = 40 m
(∵ Length of park = 90 m)

Question 6.
A wire is in the shape of a rectangle. Its length is 40 cm and breadth is 22 cm. If the same wire is rebent in the shape of a square, what will be the measure of each side. Also find which shape encloses more area?
Answer:
Length of rectangle = 40 m
Breadth of rectangle = 22 m
Perimeter of rectangle = 2 × (l + b)
= 2 × (40 + 22) m
= 2 × 62 m = 124 m
Since, the wire is rebent to form a square
∴ Perimeter of square = 124 m
Side = \(\frac{\text { Perimeter }}{4}\) = \(\frac{124}{4}\) m = 31 m
∴ Area of rectangle = l × b
= 40 × 22 m² = 880 m²
Also, Area of square = (Side)²
= (31 m)²
= 961 m²
Since, 961 m² > 880 m²
∴ Square encloses more area.

Question 7.
The perimeter of a rectangle is 130 cm. If the breadth of the rectangle is 30 cm, find its length. Also find the area of the rectangle.
Answer:
Perimeter of rectangle = 130 cm
b = 30 cm
l = \(\frac{p}{2}\) - b = \(\frac{130}{2}\) - 30
= 65 - 30 cm = 35 cm
Area of rectangle = l × b
= 35 × 30 cm² = 1050 cm²
So, the area of rectangle is 1050 cm².

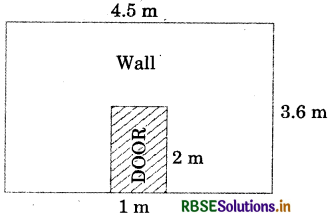
Question 8.
A door of length 2 m and breadth 1 m is fitted in a wall. The length of the wall is 4.5 m and the breadth is 3.6 m. Find the cost of whitewashing the wall, if the rate of whitewashing the wall is ₹ 20 per m².
Answer:
Length of door = 2 m
Breadth of door = 1 m
∴ Area of door = 1 × b
= (2 × 1) m² = 2 m²
Length of wall = 4.5 m
Breadth of wall = 3.6 m
Area of wall = l × b
= 4.5 x 3.6 m = 16.20 m²
Area of the wall to be whitewashed
= Area of wall - Area of door
= 16.20 m² - 2 m² = 14.2 m²

∵ Cost of whitewashing 1 m² = ₹ 20
∴ Cost of whitewashing 14.2 m²
= 14.2 × 20 = ₹ 284
So, the cost of whitewashing the wall is ₹ 284.