RBSE Solutions for Class 11 Maths Chapter 9 Sequences and Series Miscellaneous Exercise

Rajasthan Board RBSE Solutions for Class 11 Maths Chapter 9 Sequences and Series Miscellaneous Exercise Textbook Exercise Questions and Answers.

RBSE Class 11 Maths Solutions Chapter 9 Sequences and Series Miscellaneous Exercise

Question 1.
Show that sum of (m + n)^th and (m - n)^th terms.of an AP. is equal to twice the m^th term
Answer:
Let a be the first term of A.P. and d be the common difference.
m^th term of series T_m = a + (m - 1)d ............. (1)
(m + n)^th term of series T_{m + n} = a + (m + n - 1)d .............. (2)
(m - n)^th term of series T_{m - n} = a + (m - n - 1)d ................. (3)
Adding equation (2) and (3)
T_{m + n} + T_{m - n}
= a + (m + n - 1)d + a + (m - n - 1)d
= a + a + [m + n - 1 + m - n - 1]d
= 2a + (2m - 2)d
= 2a + 2(m - 1)d
= 2 [a + (m - 1)d]
= 2 × T_m [From equation (1)]
= 2 × m^th terms
Thus, sum of (m + n)^th term and (m - n)^th terms
= 2 × sum of m^th terms
Hence proved.

Question 2.
If the sum of three numbers in A.P. is 24 and their product is 440, find the numbers.
Answer:
Let three numbers of A.P.be a - d, a, a + d.
We have sum of number = 24
⇒ a - d + a + a + d = 24
⇒ 3a = 24
∴ a = 8
Now, (a - d) . (a) . (a + d) = 440
⇒ (8 - d) × 8 × (8 + d) = 440
⇒ 8(64 - d²) = 440
⇒ 64 - d² = \(\frac{440}{8}\) = 55
⇒ - d² = 55 - 64
⇒ - d² = - 9
⇒ d² = 9
∴ d = ±3
So,d = 3 or d = - 3
When a = 8 and d = 3 then
First number = (a - d) = 8 - 3 = 5
Second number = a = 8
and third number = (a + d) = 8 + 3 = 11
Thus, numbers are 5, 8, 11
When a = 8 and d = - 3 then numbers
8 - (- 3), 8, 8 + (- 3)
⇒ 8 + 3, 8, 8 - 3
⇒ numbers will be 11, 8, 5

Question 3.
Let sum of n, 2n and 3n terms of an A.P. be S₁, S₂ and S₃ respectively, then prove that S₃ = 3(S₂ - S₁)
Answer:
Let the first term of A.P. be a and common difference is d.

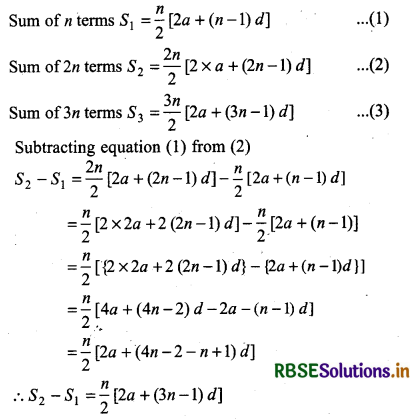
Multiplying both sides by 3.
3(S₂ - S₁) = \(\frac{3 n}{2}\)[2a + (3n - 1)d]
= S₃ [From equation (3)]
Thus, 3(S₂ - S₁) = S₃
Hence proved.

Question 4.
Find the sum of all. numbers between 200 and 400 which are divisible by 7.
Answer:
Between 200 and 400, number divisible by 7 is 203
First term of series, a = 203
Since after 200 and 203 is number which is divisible by 7
\(\frac{200}{7}\) = 28 \(\frac{4}{7}\) or 29 and
29 × 7 = 203
and last number divisible by 7, between 200 and 400 = 399
Since \(\frac{400}{7}\) = 57\(\frac{1}{7}\) or 57
and 57 × 7=399
Thus last term of sequence l = 399
Thus, between 200 and 400, numbers divisible by 7
203, 210, 217, 224 .................. 399 are in A.P.
Here a = 203, common difference d = 210 - 203 = 7
let total numbers be n.
Then n^th number = 399
⇒ a + (n - 1)d = 399
[Formula nth term = last term = a + (n - 1)d]
⇒ 203 + (n - 1) × 7 399
⇒ 7n - 7 = 399 - 203
⇒ 7n - 7 = 196
⇒ 7n = 196 + 7
⇒ 7n = 203
⇒ n = \(\frac{203}{7}\)
⇒ n = 29

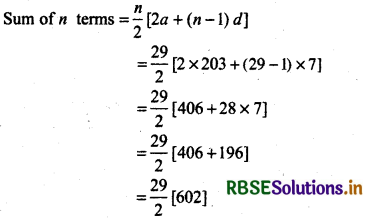
= 29 × 301
= 8729
Thus, sum of numbers between 200 and 400 and divisible by 7 = 8729

Question 5.
Find the sum of integers from 1 to 100 that are divisible by 2 or 5.
Answer:
Integers from 1 to 100 which are divisible by 2 are
2, 4, 6, 8, 10, ................... 98, 100
and integers from 1 to 100 which are divisible by 5 but not by 2 are
5, 15, 25, ..................... 95
Sum of integers from 1 to 100 which are divisible by 2 or 5
= (2 + 4 + 6 + 8 + 10 + .................. + 100) + (5 + 15 + 25 + ................. + 95)
= 2(1 + 2 + 3 + 4 + ................. 50) + 5 (1 + 3 + 5 + 7 + ................... + 19)
= 2\(\frac{50(50+1)}{2}\) + 5\(\frac{10}{2}\) [1 + 19]
[∵ sum of n terms of A.P. = \(\frac{n}{2}\) (a + l)]
= 50 × 51 + 5 × 5 [1 + 19]
[Since, numbers which are divisible by 5 = 10]
= 2550 + 25 × 20
= 2550 + 500
= 3050
Thus, required sum = 3050

Question 6.
Find the sum of all two digit numbers which when divided by 4 yield 1 as remainder.
Answer:
First two-digit number = 10
And last tWo-digit number = 99
Two-digit first number when divided by 4 yields remainder 1 = 13
And two-digit last number when divided by 4 yields remainder 1 = 97
Since, number less than 97 is 96 which is completely divisible by 4,
So, required numbers are 13, 17, 21, 25, ................. 97
Let number of terms in sequence be n.
Now, n^th term = 97 ............... (1)
Here a = 13, common difference d = 17 - 13 = 4
So, n^th term = a + (n - 1)d
= 13 + (n - 1) × 4
And 13 + (n - 1) × 4 = 97 [By equation (1)]
⇒ 13 + 4n - 4 = 97
⇒ 4n = 97 + 4 - 13
⇒ 4n = 101 - 13
⇒ 4n = 88
∴ n = 22
So, number 6f terms ¡n sequence = 22
Sum of all numbers = 13 + 17 + 21 + ................. + 97
Here, a = 13, d = 4, n = 22
Since, S_n = \(\frac{n}{2}\)[2a + (n - 1)d]
S₂₂ = \(\frac{22}{2}\) [2 × 13 + (22 - 1) × 4]
= 11 × [26 + 84]
= 11 × 110
= 1210
Thus, sum of two-digit numbers which yield remainder 1 when divide by 4 = 1210

Question 7.
If f is a function satisfying f(x + y) = f(x) f(y) for ail x, y ∈ N such that f(1) = 3 and \(\sum_{x=1}^n\) f(x) = 120, find the value of n.
Answer:
Given, f(x + y) = f(x)f(y)
and f(1) = 3
For x = 1 and y = 1
f (1 + 1) = f(1) × f(1)
⇒ f(2) = 3 × 3 = 9
⇒ f(2) = 9
Now, for x = 1 and y = 2
f(1 + 2) = f(1) × f(2)
f (3) = 3 × 9
f (3) = 27
And for x = 1 and y = 3
f(1 + 3) = f(1) × f(3)
⇒ f (4) = 3 × 27
f (4) = 81
Similarly, for x = 1 and y = 4
f (1 + 4) = f (1) × f (4)
⇒ f(5) = 3 ⇒ 81
⇒ f(5) = 243
Similarly, we can obtain next terms.
Again \(\sum_{x=1}^n f(x)\) = 120
So, f(1) + f(2) + f(3) + ............ + f(n) = 120
or 3 + 9 + 27 + 81 + 243 + ............. + n terms = 120
Above sequence is a G.P.,
whose first term a = 3, common ratio r = 3

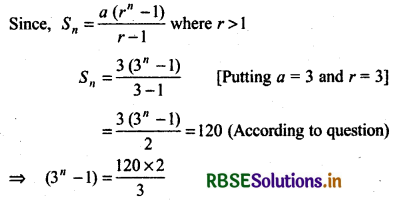
⇒ 3ⁿ - 1 = 80
⇒ 3ⁿ = 80 + 1
⇒ 3ⁿ = 81
⇒ (3)ⁿ = (3)⁴
Comparing powers on both sides
n = 4
Thus, required value of n = 4

Question 8.
The sum of some terms of GP. is 315 whose first term and the common ratio are S and 2 respectively. Find the last term and the number of terms.
Answer:
Let n be the number of terms in GP.
Given, first term a = 5
and common ratio r = 2
Given, sum of n terms = 315
Since, S_n = a\(\frac{\left(r^n-1\right)}{r-1}\), where r > 1
∴ \(\frac{5\left(2^n-1\right)}{2-1}\) = 315 (∵ s_n = 315)
⇒ 5(2ⁿ - 1) = 315
⇒ 2ⁿ - 1 = \(\frac{315}{5}\)
⇒ 2ⁿ - 1 = 63
⇒ 2ⁿ = 63 + 1
⇒ 2ⁿ = 64
⇒ 2ⁿ = (2)⁶
Comparing powers on both sides
n = 6
Now, n^thterm = ar^{n - 1}
= 5 × 2^{6 - 1}
= 5 × 2⁵
= 5 × 32 = 160
Thus, last term of series = 160
and number of terms = 6

Question 9.
The first term of a G.P. is 1. The sum of the third term and fifth term is 90. Find the common ratio of G.P.
Answer:
Let common ratio of GP. is r.
Here, first term a = 1
Third term a₃ = ar^{3 - 1} [∵ nth term a_n = ar^{n - 1}]
= ar²
= 1 × r²
= r²
Fifth term a₅ = ar^{5 - 1}
= ar⁴ = 1 × r⁴ = r⁴
According to question, a₃ + a₅ = 90
So, r² + r⁴ = 90
⇒ r⁴ + r² - 90 = 0
⇒ r⁴ + 10r² - 9r² - 90 = 0
⇒ r² (r² + 10) - 9 (r² + 10) = 0
⇒ (r² + 10) (r² - 9) = 0
⇒ r² + 10 = 0 or r² - 9 = 0
⇒ r² = - 10 or r² = 9
But r² ≠ - 10
[Because this will be imaginary number]
Since r = √- 10 (Impossible)
Now, from r² = 9
⇒ r = ±3
Thus, the common ratio of series = ± 3

Question 10.
The sum of three numbers in G.P. is 56. If
we subtract 1, 7, 21 from these numbers in that order, we obtain an arithmetic progression. Find the numbers.
Answer:
Let first term of GP. is a and common ratio is r
Then three numbers of series a, ar, ar² are in GR
According to question
a + ar + ar² = 56
or a(1 + r + r²) = 56 ................... (1)
Now, subtracting 1, 7, 21 from a, ar, ar² respectively.
Numbers (a - 1), (ar - 7), (ar² - 21) are in A.P.
∴ 2(ar - 7) = (a - 1) + (ar² - 21)
[∵ If a, b, c are in A.P. then zb = a + c]
⇒ 2ar - 14 = a + ar² - 1 - 21 .
⇒ 2ar - 14 = a + ar² - 22
⇒ a + ar² = 2ar - 14 + 22
⇒ a + ar² = 2ar + 8
⇒ a - 2ar + ar² = 8
⇒ a(1 - 2r + r²) = 8 .............. (2).
Dividing equation (1) by (2), we get

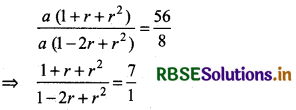
⇒ 1 + r + r² = 7 - 14r + 7r²
⇒ 7r² - r² - 14r - r + 7 - 1 = 0
⇒ 6r² - 15r + 6 = 0
⇒ 6r² - (12 + 3)r + 6 = 0
⇒ 6r² - 12r - 3r + 6 = 0
⇒ 6r(r - 2) - 3(r - 2) = 0
⇒ (6r - 3) (r - 2) = 0
Either 6r - 3 = 0 or r - 2 = 0
⇒ r = \(\frac{3}{6}\) = \(\frac{1}{2}\) or r = 2
⇒ r = 2 or \(\frac{1}{2}\)
Taking r = 2 from equation (1)
a + ar + ar² = 56
⇒ a + 2a + 4a = 56
⇒ 7a = 56 .
a = 8
⇒ ar = 8 × 2 = 16 and ar² = 8 × 2² = 32
Thus, number are a, ar, ar² = 8, 16, 32
When r = \(\frac{1}{2}\), from equation (1), we have
∴ a + \(\frac{a}{2}\) + \(\frac{a}{4}\) = 56
⇒ \(\frac{4 a+2 a+a}{4}\) = 56
⇒ 7a = 56 × 4
⇒ a = \(\frac{56 \times 4}{7}\)
∴ a = 32
The numbers a, ar, ar² = 32, 16, 8
So, numbers are 8, 16 and 32
Thus, required numbers are 8, 16, 32 or 32, 16, 8

Question 11.
A G.P. consists of an even number of terms. If the sum of all the terms is 5 times the sum of terms occupying odd places, then find its common ratio.
Answer:
Let the first term of GP. be a and the common ratio be r and number of terms 2n.
So, sequence will be a, ar, ar², ar³, ................. ar^{2n - 1}.
S_2n = \(\frac{a\left(r^{2 n}-1\right)}{r-1}\), where r > 1 ................ (1)
Now, number of terms placed at odd places and their common ratio = r²
Since sequence formed by terms at odd places will be a, ar², ar⁴, ....................

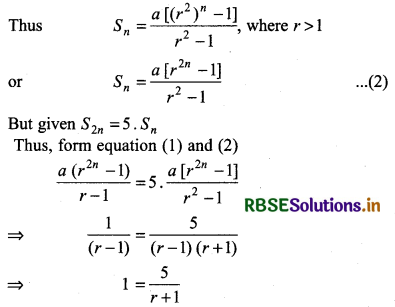
⇒ r + 1 = 5
⇒ r = 5 - 1
∴ r = 4
Thus, required common ratio of given series is 4.

Question 12.
The sum of first four terms of an A.P. is 56. The sum of the last four terms is 112. If Its first. term is 11, then find the number of terms.
Answer:
Let the number of terms in A.P. be n and the common difference be d.
We have I^st term = a =11
II^nd term = a + d = 11 + d
III term = a + 2d = 11 + 2d
and IVth term = a + 3d = 11 + 3d
Sum of first four terms
a + a + d + a + 2d + a + 3d = 56
⇒ 11 + 11 + d + 11 + 2d + 11 + 3d = 56
⇒ 44 + 6d = 56
⇒ 6d = 56 - 44
⇒ 6d = 12
⇒ d = 2
Now, sequence consist of n terms
Then, last four terms will be (n - 3), (n - 2), (n - 1) and
Then (n - 3)^th term
= a + (n - 3 - 1) × d
= 11 + (n - 4) × 2
= 11 + 2n - 8
= 2n + 3
(n - 2)^th term = a + (n - 2 - 1) × d
= 11 + (n - 3) × 2
= 11 + 2n - 6
= 2n + 5
(n - 1)^th term = a + (n - 1 - 1)d
= 11 + (n - 2) × 2
= 11 + 2n - 4
= 2n + 7
So, nth term = a + (n - 1) d
= 11 + (n - 1) × 2
= 11 + 2n - 2
= 2n + 9
Then last four terms of sequence will be
(2n + 3), (2n + 5), (2n + 7), (2n + 9)
According to the question,
Sum of last four terms = 112
⇒ 2n + 3 + 2n + 5 + 2n + 7 + 2n + 9 = 112
⇒ 8n + 24 = 112
⇒ 8n = 112 - 24
⇒ 8n = 88
⇒ n = 11
Thus, number of terms in sequence is 11.

Question 13.
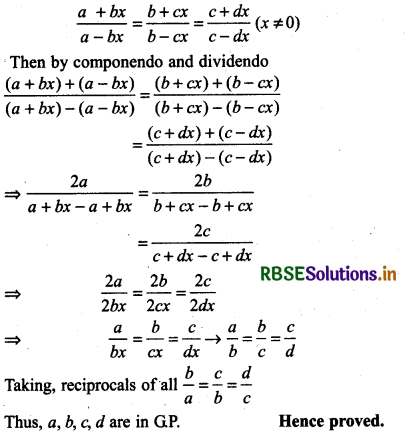
If \(\frac{a+b x}{a-b x}=\frac{b+c x}{b-c x}=\frac{c+d x}{c-d x}\), (x ≠ 0) then show that a, b, c and d are in G.P.
Answer:
We have,

Question 14.
Let S be the sum, P the product and R the sum of reciprocals of n terms in a G.P. Prove that P² Rⁿ = Sⁿ
Answer:
Let first term of GP. = a
Common ratio = r
Then n terms of GP. will be a, ar, ar², ar³, ........................,
We have,
a + ar + ar² + ar³ + ....... + ar^{n - 1} = S
⇒ \(\frac{a\left(r^n-1\right)}{r-1}\) = S ................... (1)
Product of terms
a × (ar) × (ar²) × (ar³) × ............... × ar^{n - 1} = P (given)
⇒ aⁿ r^{(1 + 2 + 3 + .............. n - 1)} terms = P

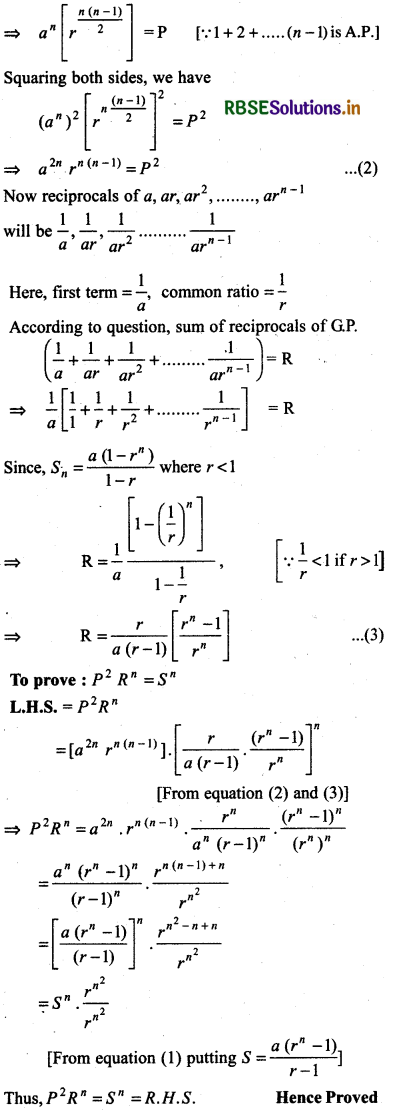
Question 15.
If p, q and r terms of an AP. are a, b, c respectively, then prove that
(q - r)a + (r - p)b + (p - q)c = 0
Answer:
Let first term of series = A
Then we have
pth term of series = A + (p - 1) × d = a ................ (1)
qth term of series = A + (q - 1) × d = b .............. (2)
rth term = A + (r - 1) × d = c .............. (3)
Multiplying equation (1) by (q - r) equation (2) by (r - p) and equation (3) by (p - q) and then adding
a(q - r) + b(r - p) + c(p - q)
= A(q - r) + A(r - p) + A(p - q) + (p - 1)d × (q - r) + (q - 1)d × (r - p) + (r - 1) × d(p - q)
= A(q - r + r - p + p - q) + d [(p - 1) (q - r) + (q - 1) (r - p) + (r - 1) (p - q)]
= A × 0 + d[pq - pr - q + r + qr - qp - r + p + rp - rq - p + q]
= A × 0 + R × 0 = 0
Thus, a(q - r) + b(r - p) + c(p - q) = 0
or (q - r)a + (r - p)b + (p - q)c = 0
Hence Proved.

Question 16.
If a\(\left(\frac{1}{b}+\frac{1}{c}\right)\), b\(\left(\frac{1}{c}+\frac{1}{a}\right), c\left(\frac{1}{a}+\frac{1}{b}\right)\) are in A.P. then prove that a, b, c are in A.P.
Answer:
We have

Question 17.
If a, b, c, d are in G.P., then prove that (aⁿ + bⁿ), (bⁿ + cⁿ), (cⁿ + dⁿ) are in G.P.
Answer:
Given a, b, c, d are in GP.

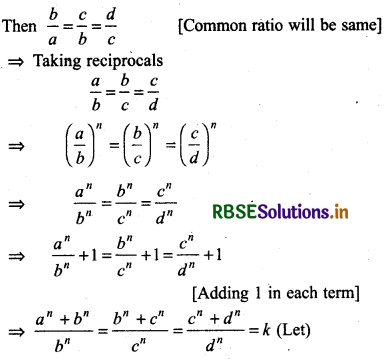
Then aⁿ + bⁿ = kbⁿ ................. (1)
bⁿ + cⁿ = kcⁿ ......................... (2)
cⁿ + dⁿ = kdⁿ ....................(3)
Then by equation (1) and (3)
(aⁿ + bⁿ) (cⁿ + dⁿ) = k² bⁿ dⁿ .........(4)
Since, \(\frac{b}{c}=\frac{c}{d}\) (Given)
Then bd = c²
⇒ bⁿdⁿ = c²ⁿ ........... (5)
Thus, (aⁿ + bⁿ) (cⁿ + dⁿ) = k² c²ⁿ [From equation (4)]
⇒ (aⁿ + bⁿ) (cⁿ + dⁿ) = (k cⁿ)²
⇒ (aⁿ + bⁿ) (cⁿ + dⁿ) = (bⁿ + cⁿ)² [From equation (2)]
⇒ (aⁿ + bⁿ) (cⁿ + dⁿ) = (bⁿ + cⁿ)²
⇒ (aⁿ + bⁿ), (bⁿ + cⁿ), (cⁿ + dⁿ) are in GP.
Hence proved.

Question 18.
If a and b are roots of x² - 3x + p = 0 and c and d are roots of x² - 12x + q = 0 where a, b, ç, d form a G.P. Prove that (q + p):(q - p) = 17:15
Answer:
We have, a and b are roots of equation x² - 3x + p = 0
Sum of roots = a + b = - (- 3) = 3
⇒ a + b = 3 ..................... (1)
Product of roots = ab = p ................... (2)
Root of equation x² - 12x + q are c and d
= c + d = 12 ........................ (3)
Product of roots cd = q ...................... (4)
Now q + p = cd + ab or ab + cd
q - p = cd - ab
So, \(\frac{q+p}{q-p}=\frac{a b+c d}{c d-a b}\) .................... (5)
Again, a, b, c, d are in G.P. (given)

So, d = 4b ....................... (6)
Again a + b = 3 and c + d = 12
[From equation (1) and (3)]
Then 4a + 4b = 12 = c + d
⇒ 4a + 4b = c + d
⇒ 4a + d = c + d
[From equation (6) Putting]
⇒ c = 4a
c = 4a, d = 4b
Then cd = 4a ⇒ 4b
⇒ cd = 16ab ..................... (7)
Putting value of cd in equation (5), we have
\(\frac{q+p}{q-p}=\frac{a b+c d}{c d-a b}=\frac{(a b+16 a b)}{(16 a b-a b)}\)
= \(\frac{a b(1+16)}{a b(16-1)}=\frac{17}{15}\)
Thus (q + p) :(q - p) = 17 : 15
Hence Proved.

Question 19.
The ratio of the A.M. and GM. of two positive numbers a and b is m : n. Show that
a : b = (m + \(\sqrt{m^2-n^2}\)) : (m - \(\sqrt{m^2-n^2}\))
Answer:
A.M. of numbers a and b
A = \(\frac{a+b}{2}\)
and GM. of numbers a and b
G = √ab
We have A : G = m : n

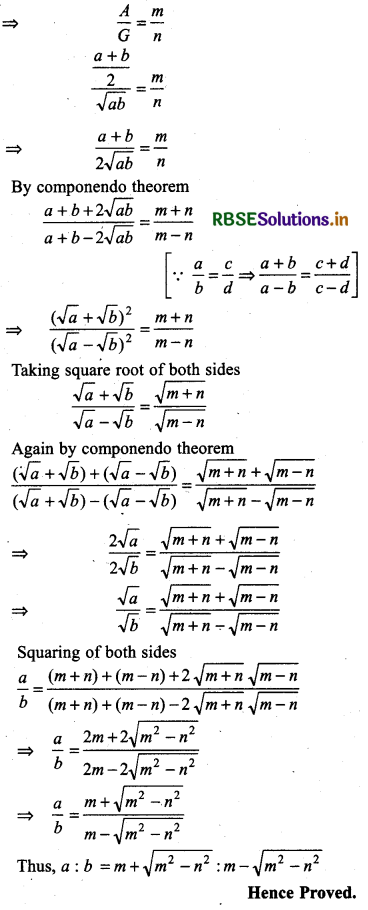
Question 20.
If a, b, c are in AP. b, c, d are In G.P. and \(\frac{1}{c}, \frac{1}{d}, \frac{1}{e}\) are in A.P., prove that a, c, e are in G.P.
Answer:
We have, a, b, c are in AP.

Question 21.
Find sum of the following series upto n terms:
(i) 5 + 55 + 555 + ..............
(ii) 6 + 66 + 666 + ..................
Answer:
(i) Given series 5 + 55 + 555 + ........................ n terms
Let the sum of n terms be S_n.
Now S_n = 5 + 55 + 555 + .......................... n terms
= 5[1 + 11 + 111 + ........................ n terms]
Multiplying Numerator and Denominator by 9

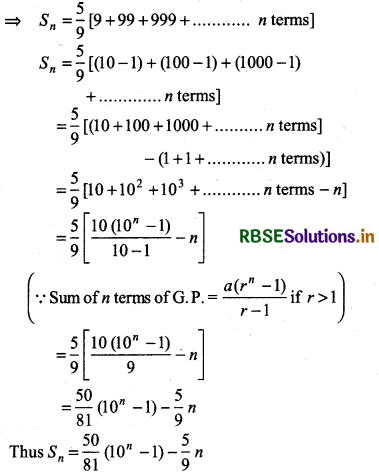
(ii) Let S_n = . 6 + .66 + .666 + .................. n terms
= 6[.1 + . 11 + . 111 + .............. n terms]
Multiplying numerator and denominator by 9

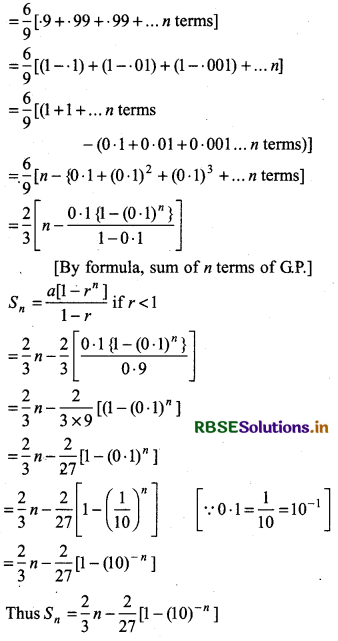
Question 22.
Find 20th term of the series
2 × 4 + 4 × 6 + 6 × 8 + ........... + n terms
Answer:
Given sequence
= 2 × 4 + 4 × 6 + 6 × 8 + ................. + n terms
20th terms of this sequence
= (2, 4, 6, ....................... 20th terms) × (4, 6, 8 20th terms)
= [2 + (20 - 1) × 2] × [4 + (20 - 1) × 2]
= [2 + 19 × 2] × [4 + 19 × 2] .
= 40 × 42
= 1680
Thus, 20th term of given series = 1680

Question 23.
Find sum of first n terms of the series:
3 + 7 + 13 + 21 + 31 + ...............
Answer:
Let S be the sum of n terms of given sequence 3 + 7 +
13 + 21 + 31 + ................ S_n and n^th terms is t_n and (n - 1)^th term be t_{n - 1}.
Now, S_n = 3 + 7 + 13 + 21 + 31 + .......... + t_n
S_n = 3 + 7 + 13 + 21 + t_{n - 1} + t_n
On subtracting
0 = 3 + 4 + 6 + 8 + ..................... - t_n
N0w, t_n = 3 + 4 + 6 + 8 + ............ n term
t_n = 3 + [4 + 6 + 8 + ............. (n - 1) term]

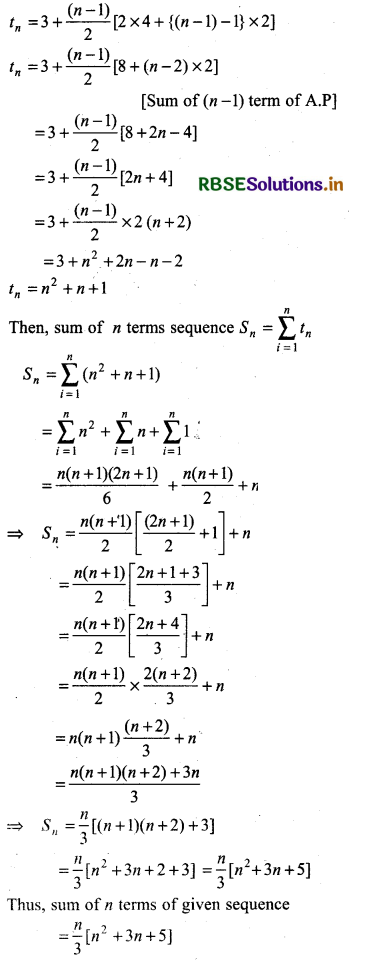
Question 24.
If S₁, S₂, S₃ are sum of first n natural numbers, their squares and their cubes respectively show that:
9S²₂ = S₃ (1 + 8S₁)
Answer:
We have

Question 25.
Find the sum of the following series upto n terms.
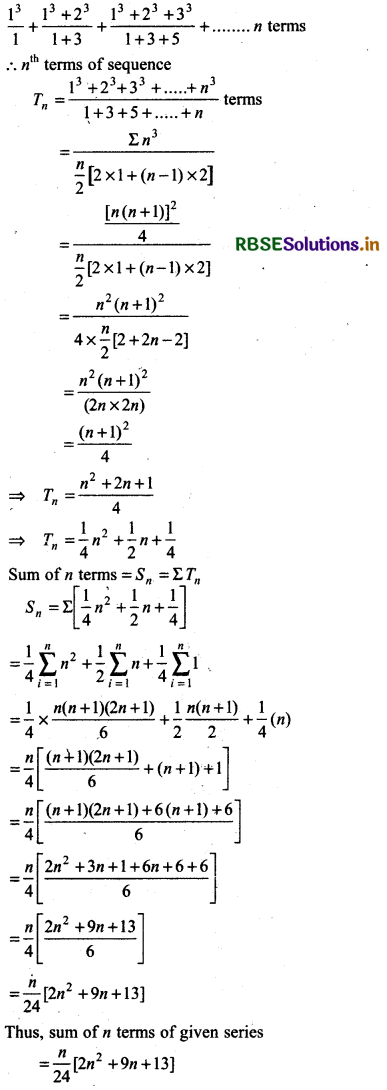
\(\frac{1^3}{1}+\frac{1^3+2^3}{1+3}+\frac{1^3+2^3+3^3}{1+3+5}+\ldots \ldots\)
Answer:
Given sequence

Question 26.
Show that
\(\frac{1 \times 2^2+2 \times 3^2+\ldots \ldots+n(n+1)^2}{1^2 \times 2+2^2 \times 3+\ldots \ldots n^2 \times(n+1)}=\frac{3 n+5}{3 n+1}\)
Answer:
L.H.S.

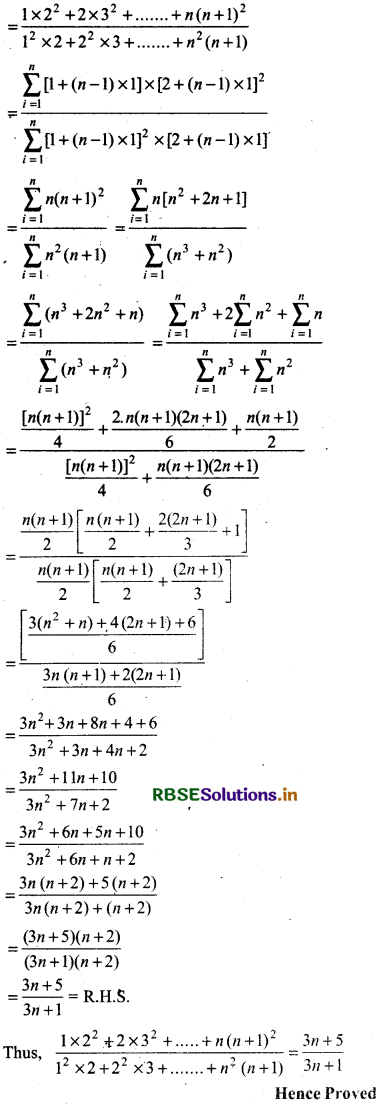
Question 27.
A farmer buys a used tractor for ₹ 12000. He pays ₹ 6000 cash and agrees to pay the balance in annual instalments of ₹ 500 plus 12% interest on the unpaid amount. How much will the tractor cost him?
Answer:
We have,
C.P of Tractor = ₹ 12,000
Cash payment = ₹ 6,000
Balanced amount on which interest have to paid
= ₹ 12,000 - ₹ 6,000
= ₹ 6,000
Annual instalment = ₹ 500
Number of instalment = \(\frac{₹ 6,000}{₹ 500}\) = 12
According to question,
Including interest on balance amount first instalment have to be paid
So with 1^st instalment, principal for interest = ₹ 6,000
Interest = \(\frac{6,000 \times 12 \times 1}{100}\) = ₹ 720 (Interest on 1^st instalment)
After 1st instalment, balance amount
= ₹ 6,000 - ₹ 500 = ₹ 5,500
So, along with 2^nd instalment, principal for interest = ₹ 5,500
Interest on second installment = \(\frac{5,500 \times 12 \times 1}{100}\) = ₹ 660
Balance amount = ₹ 5,500 - ₹ 500
= ₹ 5,000
So, along with 3^rd instalment, principal for interest = ₹ 5,000
Interest on 3^rd installment = \(\frac{5,000 \times 12 \times 1}{100}\) = ₹ 600
Remaining amount = ₹ 5, 000 - ₹ 500
= ₹ 4500
So, along with 4^th instalment, principal for interest = ₹ 4500
Interest on 4^th installment = \(\frac{4,500 \times 12 \times 1}{100}\) = ₹ 4500
.............................
.............................
Similarly, we have to find upto 12^th instalment.
Total amount of interest
= 720 + 660 + 600 + 540 + .......... + interest of 12^th instalment which makes A.P., whose
First term = a = 720
Common difference = d = 660 - 720 = - 60
Number of terms = 12 (no. of instalments)
Since, S = \(\frac{n}{2}\) [2a + (n - 1)d]
Total interest = \(\frac{12}{2}\) [2 × 720 + (12 - 1) × (- 60)]
= 6[1,440 - 660]
= 6 × [780]
= ₹ 4680
Total amount to be paid
= ₹ 12,000 + ₹ 4,680
= ₹ 16,680
Thus, total cost of tractor = ₹ 16,680

Question 28.
Shamshad All buys a scooter for ₹ 22000. He pays ₹ 4000 cash and agrees to pay the balance In annual instalment of ₹ 1000 pIus 10% interest on the unpaid amount. How much will the scooter cost him?
Answer:
We have
Cost price of scooter = ₹ 22,000
Cash payment = ₹ 4,000
Balance amount on which interest have to be paid
= ₹ 22,000 - ₹ 4,000
= ₹ 18,000
Annual instalment = ₹ 1,000
Number of instalment = ₹ \(\frac{18,000}{1,000}\) = 18
Along with 1 st instalment principal for interest = ₹ 18,000
Interest for first instalment = ₹ \(\frac{18,000 \times 10 \times 1}{100}\)
= \(\frac{18,000 \times 10 \times 1}{100}\)
= \(\frac{18,000 \times 10 \times 1}{100}\)
Remaining amount after first installment
= ₹ 18,000 - ₹ 1,000
= ₹ 17,000
Interest for second = ₹ \(\frac{17,000 \times 10 \times 1}{100}\)
= ₹ 1,700
After 2^nd instalment, Remaining amount
= ₹ 17,000 - ₹ 1,000
= ₹ 16,000
Along with third instalment, principal for interest
= ₹ 16,000
Interest for third instalment = ₹ \(\frac{16,000 \times 10 \times 1}{100}\)
= ₹ 15,000
Thus, along with four instalment, principal for interest
= ₹ \(\frac{15,000 \times 10 \times 1}{100}\)
= ₹ 1,500
................................
...............................
Total amount of interest
= 1,800 + 1,700 + 1,600 + 1,500 + ........ +
Interest upto 18^th instalment which form a A.P. whose
First term a = 1,800
Common difference d = 1,700 - 1,800 = - 100
Number of terms = 18 (total number of instalments)
Since, S_n = \(\frac{n}{2}\) [2a + (n - 1) d]
Total interest = \(\frac{18}{2}\) [2 × 1,800 + (18 - 1) (- 100)]
= 9[3,600 + 17 (- 100)]
= 9[3600 - 1,700]
= 9 × 1, 900
= ₹ 17,100
Total amount to be paid
= ₹ 22,000 + ₹ 17,100
= ₹ 39,100
Thus, Shamshad will be paid ₹ 39,100 for scooter

Question 29.
A person writes a letter to four of his friends. He asks each one of them to copy the letter and mail to four different persons with instruction that they move the chain similarly. Assuming that the chain is not broken and that it costs 50 paise to mall one lefter. Find the amount spent on the postage when 8^th set of letter is mailed.
Answer:
According to question,
1 st person writes 4 letters and send to four persons
All four persons also write 4 letters ans send to 16 persons, since I person sends 4 letters.
In this way at second level, number of letters = 4 × 4 = 16
Now, 16 persons also write 4-4 letters.
So, at third level, number of letters = 64
This repeats upto eight level.
In this way, we get 4, 16, 64 ............... which is GP.
Its first term a = 4

Total amount spent on postage at the rate of 50 p per letter = 50 × 87380 p
= 4369000 p
= ₹ \(\frac{43,69,000}{100}\) = ₹ 43,690
Thus, total postage = ₹ 43,690

Question 30.
A man deposited ₹ 10,000 in a bank at the rate of 5% simple Interest annually. Find the amount in 15^th year. Since, he deposited the amount and also calculate the total amount after 20 years.
Answer:
We have,
Total money deposited in bank = ₹ 10,000
Interest rate = 5% annually
In 1st year, amount deposited in bank = ₹ 10,000
Interest in 1 year = \(\frac{10,000 \times 5 \times 1}{100}\) = ₹ 500
In 2^nd year, amount deposited in bank
= ₹ 10,000 + ₹ 500 = ₹ 10,500
Interest after 2^nd year = \(\frac{10,000 \times 5 \times 1}{100}\)
= ₹ 500
In 3^rd year, amount deposite in bank
= ₹ 10, 500 + ₹ 500
= ₹ 11,000
In this way, money deposite in each year
₹ 10,000, ₹ 10,500, ₹ 11,000, ₹ 11,500 .....
which forms A.P. in which
First term a = ₹ 10,000
Common difference d = 10,500 - 10,000 = 500
Number of terms =15
In 15^th year, money deposited in account to a person
Since, Tⁿ = a + (n - 1) d
Thus T₁₅ = 10,000 + (15 - 1) × 500
= 10,000 + 14 × 500
R₁₅ = 10,000 + 7,000 = ₹ 17,000
In 15^th year, money deposited in account of a person
= ₹ 17,000
After 20 years, i.e. in 21st year total deposited amount
T_n = a + (n - 1)d
T₂₁ = 10,000 + (21 - 1) × 500
= 10,000 + 20 × 500
= 10,000 + 10,000
= ₹ 20,000
Thus, after 20 years total amount will be ₹ 20,000

Question 31.
A manufacturer reckon that the value of a machine, which cost him ₹ 15625 will depreciate each year by 20%. Find the estimated value at the end of 5 years.
Answer
We have,
Present value of machine = ₹ 15,625
Rate of depreciation = 20% per year
= \(\frac{20}{100}=\frac{1}{5}\) part
After depreciation per year cost = \(\left(1-\frac{1}{5}\right)=\frac{4}{5}\) = part
Now, initial value of machine a = ₹ 15,625
Common ratio r = \(\frac{4}{5}\)
Number of terms n = 6 [time (after 5 years) 6^th year]
Cost 8 machine after 5 years (in 6^th year)
= ar^{n - 1}

= 5 × 4 × 4 × 4 × 4 × 4
= 5 × 104
= ₹ 5,120

Alternative Method:
We have present value of machine = 15,625
Rate of depreciation = 20% per year
Cost of Machine of after 1 year
Cost of Machine of after 1 year
= (15625 - 15625 × \(\frac{20}{100}\))
= ₹ 15, 625 - ₹ 3,125
= ₹ 12,500
Cost of machine after 2 years
= (12,5oo - 12,5oo × \(\frac{12}{100}\))
= 12,500 - 2,500
= ₹ 10,000
Cost of machine after 3 years
= (1o,ooo - 1o,ooo × \(\frac{20}{100}\))
= 10,000 - 2,000
= ₹ 8000
Cost of machine in sequence form
₹ 12,500, ₹ 10,000, ₹ 8,000, ...........
So, sequence is in GP. whose first term
a = ₹ 12,500

Question 32.
150 workers were engaged to finish a job in a certain number of days. 4 workers dropped out on second day, 4 more workers dropped out on third day anti so on. It took 8 more days to finish the work. Find the number of days in which the work was completed.
Answer:
Let work will be completed in n days
We have, number of workers who work on first day = 150
Number of workers who work on second day
= 150 - 4 = 156
Number of workers who work on third day = 150 - 4 × 2
= 150 - 8 = 142
Number of workers who work on fourth day
= 150 - 3 × 4
= 150 - 12
= 138
Similarly, number-of workers who work on nth day
= 150 + (n - 1) × (- 4)
= 150 + 4 - 4n
= 154 - 4n
In this way, number of workers who work on 1^st day, 2^nd day 3^rd day, ............ n^th day
150, 146, 142, 138......... (154 - 4n)
Clearly, number of workers forms a A.P. Whose first ferm = 150
Common difference = 146 - 150 = - 4
Last term = l = 154 - 4n = nth term
Total number of workers who work
S = \(\frac{n}{2}\)(a + l)
= \(\frac{n}{2}\) (150 + 154 - 4n)
= \(\frac{n}{2}\) (304 - 4n)
= n(152 - 2n)
= 152n - 2n²
If 150 workers works continuously, then work finishes before 8 days, i.e., in (n - 8) days.
Then number of workers who work for 1 day
= 150(n - 8)
= 150n - 1200
Here, 152n - 2n² = 150n - 1200
⇒ 2n² + 150n - 152n - 1200 = 0
⇒ 2n² - 2n - 1200 = 0
⇒ n² - n - 600 = 0
⇒ n² - (25 - 24)n - 600 = 0
⇒ n² - (25 - 24)n - 600 = 0
⇒ n² - 25n + 24n - 600 = 0
⇒ n(n - 25) + 24(n - 25 = 0
⇒ (n - 25) (n + 24) = 0
n = 25 or n = - 24
Rut n ≠ - 24 because number of days cannot be negative
So, required number of days = 25