RBSE Solutions for Class 11 Maths Chapter 9 Sequences and Series Ex 9.3

Rajasthan Board RBSE Solutions for Class 11 Maths Chapter 9 Sequences and Series Ex 9.3 Textbook Exercise Questions and Answers.

RBSE Class 11 Maths Solutions Chapter 9 Sequences and Series Ex 9.3

Question 1.
Find the 20^th and n^th terms of G.P.
\(\frac{5}{2}, \frac{5}{4}, \frac{5}{8} \ldots \ldots\)
Answer:

Question 2.
Find 12^th term of G.P. whose 8^th term is 192 and common ratio is 2.
Answer:
Let the first term of the progression = a
Since, T_n = ar^{n - 1}
Then 8^th term of progression = ar⁷ = 192
⇒ a.2⁷ = 192 (∵ r = 2)
⇒ a × 128 = 192
⇒ a = \(\frac{192}{128}\)
So, first term of progression a = \(\frac{3}{2}\)
Now, 12^th term of progression = ar¹¹
= \(\frac{3}{2}\) × 2¹¹
= 3 × 2¹⁰ (∵ 2¹⁰ = 1024)
= 3 × 1024
= 3072
Thus, 12^th term of progression is 3072.

Question 3.
The 5^th, 8^th and 11^th terms of a G.P. are p, q and s respectively show that q² = ps.
Answer:
Let first term of given GP. is a and the common ratio is r.
5^th term of progression = ar^{5 - 1} = ar⁴ = p ............. (1)
8^th term of progression = ar^{8 - 1} = ar⁷ = q ................. (2)
and 11th term of progression = ar^{11 - 1} = ar¹⁰ = s ................. (3)
[By formula T_n = ar^{n - 1}]
Multiplying equation (1) and (3) .
ar⁴ × ar¹⁰ = p × S
⇒ a² r¹⁴ = ps
⇒ (ar⁷)² = ps [From equation (2)]
Thus, q² = ps
Hence Proved

Question 4.
The 4^th term of a G.P. is square of its second term, and the first term is - 3. Determine its 7^th term.
Answer:
Let the common ratio of GP. is r.
Here, first term a = - 3
Second term of progression = ar^{2 - 1}
= ar = - 3r
Fourth term of progression = ar^{4 - 1} = ar³ = (- 3) r³
According to the question
Fourth term of progression = (second term of progression)²
- 3r³ = (- 3r)²
⇒ - 3r³ = 9r²
⇒ r = - 3
Now, 7^th term of progression = ar^{7 - 1} = ar⁶
= -3 × 729
=- 2187
Thus, 7^th term = - 2187

Question 5.
Which term of the following sequences
(a) 2, 2, √2, 4, ................ is 128?
Answer:
Given, sequence is 2, 2√2, 4, .......................
Here, first term a = 2

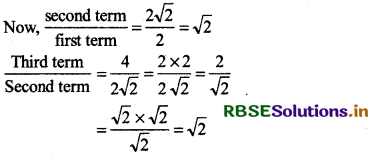
So, common ratio = √2
Given sequence is in GP.
Let p^th term of the sequence is 128.
Now p^th term = ar^{p - 1} = 128
⇒ 2(√2)^{p - 1} = 128
⇒ (√2)^{p - 1} = 64
⇒ (√2)^{p - 1} = 2⁶
⇒ (√2)^{p - 1} = [(√2)²]⁶
⇒ (√2)^{p - 1} = (√2)¹²
Comparing powers on both sides,
p - 1 = 12
or p = 12 + 1
∴ p = 13
Thus, 13^th term is 128.

(b) √3, 3, 3√3, ..................‚ is 729?
Answer:
Here, first term a = √3.
Second term = 3
Third term = 3√3

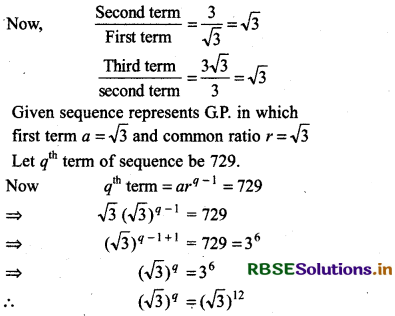
On comparing powers of both sides
q = 12
Thus, 12^th term of sequence is 729

(c) \(\frac{1}{3}, \frac{1}{9}, \frac{1}{27}, \ldots \ldots . \text { is } \frac{1}{19683}\)?
Answer:

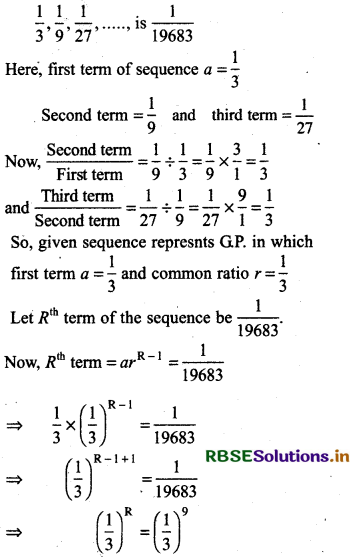
Comparing powers on both sides,
R = 9
Thus, 9th term of sequence is \(\frac{1}{19683}\).

Question 6.
For what values of x, the numbers - \(\frac{2}{7}\), x, - \(\frac{7}{2}\) are in G.P.?
Answer:

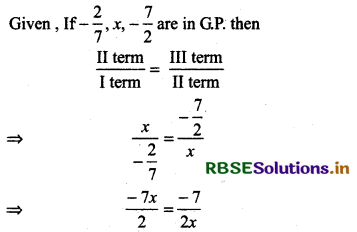
⇒ 14x² = - 14
⇒ x² = 1
⇒ x = ±1
∴ x = ±1
Thus, value of x = ±1

Find the sum to Indicated number of terms in each of the geometric progressions in exercises 7 to 10.

Question 7.
0.15, 0.015, 0.0015, ................. 20 terms.
Answer:
Given GP.
0.15, 0.015, 0.0015, .................
I term = a₁ = 0.15
II term = a₂ = 0.015

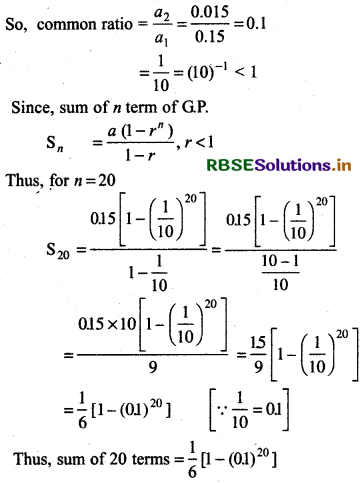
Question 8.
√7, √21, 3√7, ...................... n terms.
Answer:

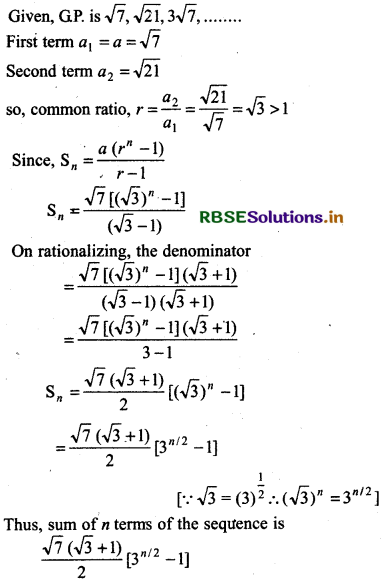
Question 9.
1, - a, a², - a³, ........ n terms (if a ≠ - 1)
Answer:
Given, G.M. is 1, - a, a², - a³, .................... n terms
First term a₁ = a = 1
Second term a₂ = - a

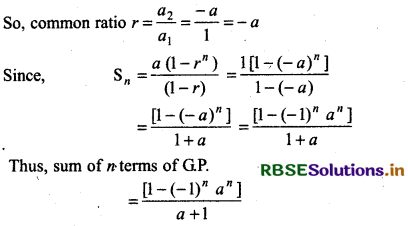
Question 10.
x³, x⁵, x⁷, ................. terms (if x ≠ ± 1).
Answer:
Given, GP. is. x³, x⁵, x⁷, ................. [Where as x ≠ ± 1]
1^st term a₁ = x³
2^nd term a₂ = x⁵

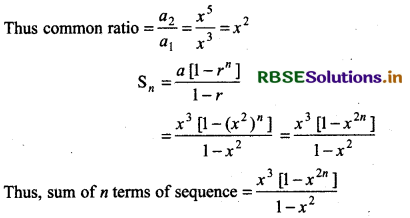
Question 11.
Evaluate \(\sum_{k=1}^{11} (2 + 3k)\)
Answer:
\(\sum_{k=1}^{11}\) (2 + 3^k) + (2 + 3¹) + (2 + 3²) + (2 + 3³) + (2 + 3⁴) + (2 + 3⁵) + (2 + 3⁶) + ...................... + (2 + 3¹¹)
[Putting k = 1, 2, 3 .............. - 11 and adding]
= (2 + 2 + 2 + ......... + 11 terms) + (3¹ + 3² + 3³ + .................... + 3¹¹)
= 2 × 11 + (3 + 3² + 3³ + ................. + 3¹¹)

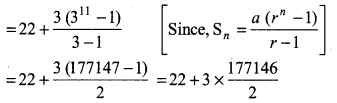
= 22 + 3 × 88573 = 22 + 265719
= 265741
Thus \(\sum_{k=1}^{11}\)(2 + 3^k) = 265741

Question 12.
The sum of first three terms of a GP. is \(\frac{39}{10}\) and their product is 1. Find the common ratio and the terms.
Answer:
Let three terms of GP. are \(\frac{a}{r}\), a, ar.
Product of these terms = \(\frac{a}{r}\) × a × ar, a³
According to question, product of three terms =1
∴ a³ = 1
⇒ a = 1
And \(\frac{a}{r}\) + a + ar = \(\frac{39}{10}\)
According to question, sum of three terms = \(\frac{39}{10}\)
⇒ \(\frac{1}{r}\) + 1 + r = \(\frac{39}{10}\) (∵ a = 1)
⇒ \(\frac{1+r+r^2}{r}=\frac{39}{10}\)
⇒ 10(r² + r + 1) = 39r
⇒ 10r² + 10r + 10 = 39r
⇒ 10r² + 10r - 39r + 10 = 0
⇒ 10r² - 29r + 10 = 0
⇒ 10r² - (25 + 4)r + 10 = 0
⇒ 10r² - 25r - 4r + 10 = 0
⇒ 5r(2r - 5) - 2(2r - 5) = 0
⇒ (2r - 5) (5r - 2) = 0

Question 13.
How many terms of G.P. 3, 3², 3³ .................. are needed to give the sum 120?
Answer:
Given, GM. = 3, 3², 3³ ..................
First term a₁ = 3
Second term a₂ = 3²
Common ratio r = \(\frac{a_2}{a_1}=\frac{3^2}{3}\) = 3
Let p terms of GP. are taken so that their sum becomes 120.
Since, S_p = \(\frac{a\left(r^p-1\right)}{r-1}\)
According to question S_p = \(\frac{3\left(3^p-1\right)}{3-1}\)
⇒ \(\frac{3\left(3^p-1\right)}{2}\) = 120
⇒ 3(3^p - 1) = 120 × 2
⇒ 3(3^p - 1) = 240
⇒ 3^p - 1 = \(\frac{240}{3}\) = 80
⇒ 3^p = 80 + 1
⇒ ^p = 81
⇒ 3^p = 3⁴
Comparing powers on both sides
p = 4
Thus, number of terms = 4

Question 14.
The sum of first three terms of a G.P. is 16 and the sum of the next three terms is 128.
Determine the first term, the common ratio and the sum to n terms of the GP.
Answer:
Let a be the first term of GP. and r be the common ratio, then GP.
a, ar, ar², ar³, ar⁴, ar⁵
Here, first three terms of GP. be a, ar, ar² and next three terms will be ar³, ar⁴, ar⁵.
Sum of first three terms = 16 = a + ar + ar²
⇒ 16 = a (1 + r + r²)
Sum of next three terms = 128 = ar³ + ar⁴ + ar⁵
⇒ 128 = ar³ (1 + r + r²)
Dividing equation (2) by (1)
\(\frac{128}{16}=\frac{a r^3\left(1+r+r^2\right)}{a\left(1+r+r^2\right)}\)
⇒ 8 = r³
⇒ r³ = 8
⇒ r³ = 2
Comparing powers on both sides
r = 2
Putting r = 2 in equation (1),
16 = a(1 + 2 + 2²)
⇒ 16 = a (7)
∴ a = \(\frac{16}{7}\) (first term)
Common ratio = 2

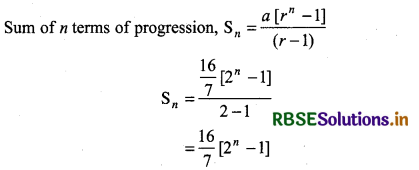
Thus, first term of progression is \(\frac{16}{7}\), common ratio r = 2 and sum of n terms S_n = \(\frac{16}{7}\)[2ⁿ - 1]

Question 15.
Given a GP. with a = 729 and 7^th term 64 Determine S₇.
Answer:
Let common ratio of GP. be r.
Here, first term a = 729, 7^th term = 64
Now, 7th term = ar^{7 - 1} ⇒ ar⁶ = 64 [∵ T_n = ar^{n - 1}]
⇒ 729 × r⁶ = 64

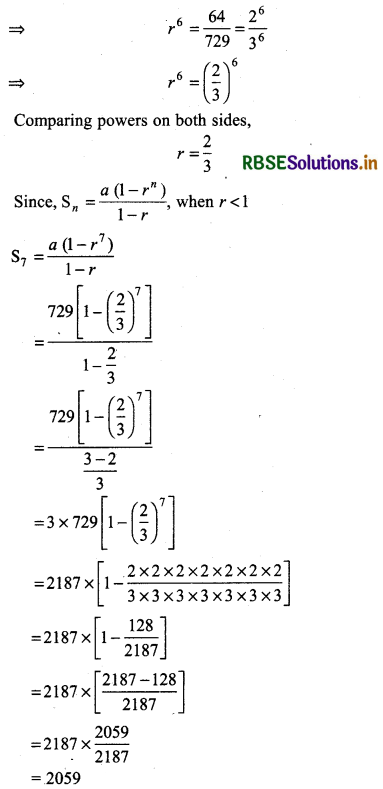
= 2059
Thus, sum of 7^th terms of G.P. is 2059.

Question 16.
Find a G.P. for which sum of the first two terms is - 4 and the fifth term is 4 times the third term.
Answer:
Let the first term of GP. be a and the common ratio be r.
So, progression will be a, ar, ar², ar³
Now, second term of progression = ar
Sum of first two terms = a + ar = - 4
⇒ a(1 + r) = - 4 .................... (1)
Third term of progression = ar^{3 - 1} = ar²
Fifth term of progression = ar^{5 - 1} = ar⁴
We have,
Fifth term = 4 × third term
ar⁴ = 4 × ar²
⇒ \(\frac{a r^4}{a r^2}\) = 4 ⇒ r² = 4
⇒ r = ±2
Taking r = 2,
From equation (1), a(1 + 2) = - 4

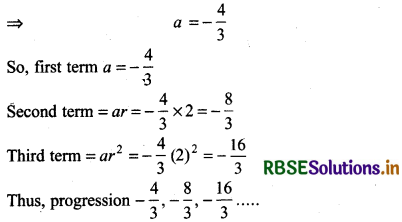
Where first term à = - \(\frac{4}{3}\) and common ratio r = 2
Taking r = - 2,
From equation (1),
a (1 - 2) = - 4
⇒ - a = - 4
∴ a = 4
So, if r = - 2, then first term a =4
then second term = ar
= 4 × (- 2) = - 8
If r = - 2, then third term
= ar² = 4(- 2)² = 16
Thus, progression, 4, - 8, 16 ...............
Where first term a is 4.
Required GP. is
\(-\frac{4}{3},-\frac{8}{3},-\frac{16}{3}\), ........... or 4, - 8, 16, - 32

Question 17.
If the 4^th, 10^th and 16^th term of a GP. are x, y and z respectively. Prove that x, y, z are in G.P.
Answer:
Let first term of GP. be a and the common ratio be r.
So, GP. a, ar, ar², ar³, ar⁴, .................
Since, T_n = ar^{n - 1}
T₄ = ar^{4 - 1} = ar³ = x (According to question) ..... (1)
T₁₀ = ar^{10 - 1} = ar⁹ = x (According to question) ..... (2) [∵ T_n = ar^{n - 1}]
T₁₆ = ar^{16 - 1} = ar¹⁵ = z (According to question) .......... (3)
Multiplying equations (1) and (3),
ar³ × ar¹⁵ = x × z
⇒ a² r¹⁸ = xz
⇒ (ar⁹)² = xz ................ (4)
From equation (2) and (4),
(y)² = xz
⇒ y² = xz
Thus, x, y, z are in GP.
Hence Proved

Question 18.
Find the sum to n terms of the sequence 8, 88, 888, 8888 .
Answer:
Given, sequence 8, 88, 888, 8888 ................. n terms
Let sum of n terms of above sequence is S.
So, S = 8 + 88 + 888 + 8888 + ...................... n terms
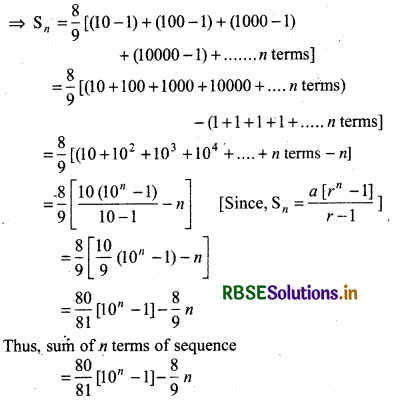
= 8[1 + 11 + 111 + 1111 + .................... n terms]
= \(\frac{8}{9}\) [9 + 99 + 999 + 9999 + nterms]
(Multiplying num. and denom. by 9)

Question 19.
Find the sum of the products of the corresponding terms of the sequences 2, 4, 8, 16, 32 and 128, 32, 8, 2, \(\frac{1}{2}\).
Answer:

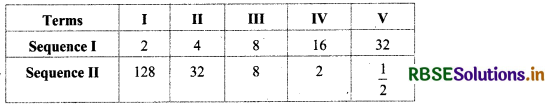
Multiplying corresponding terms of two sequences
256, 128, 64, 32, 16
This, sequence is formed by multiplying the corresponding terms of two sequences.

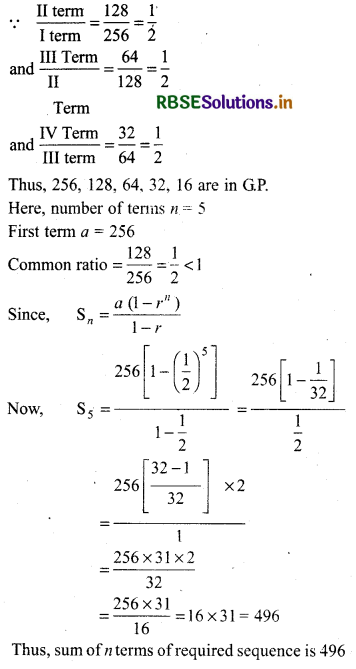
Question 20.
Show that the products of the
corresponding terms of the sequences a, ar, ar² ............ ar^{n - 1} and A, AR, AR², ...... AR^{n - 1} form a G.P. and find the common ratio.
Answer:
First sequence : a, ar, ar², ................... ar ^{n - 1}
Second sequence: A, AR, AR², .................. AR^{n - 1}
Sequence formed by the multiplication corresponding terms of two sequence
aA, ar.AR, ar² . AR², .................... ar^{n - 1}.AR^{n - 1}
⇒ aA, aA rR, aA. r² R², ..................... aA.r^{n - 1} R^{n - 1}
⇒ aA, aA (rR), aA (rR)², .................. aA (rR)^{n - 1}
Common ratio = \(\frac{a_2}{a_1}=\frac{2 \text { nd term }}{1 \text { st term }}=\frac{a \mathrm{~A} r \mathrm{R}}{a \mathrm{~A}}\) = rR
Common ratio = \(\frac{a_3}{a_2}=\frac{3 \mathrm{rd} \text { term }}{2 \mathrm{nd} \text { term }}=\frac{a \mathrm{~A}(r \mathrm{R})^2}{a \mathrm{~A} r \mathrm{R}}\) = rR
Thus, sequence so formed is GP. whose common ratio is rR.

Question 21.
Find four numbers forming a geometric progression in which the third term is greater than the first term by 9 and the second term is greater than the 4^th by 18 .
Answer:
Let the first four. terms of G.P. are a, ar, ar², ar³ respectively where a is first term and r is the common ratio.
We have
Third term First term + 9
ar² = a + 9
⇒ ar² - a = 9
∴ a(r² - 1) = 9 ............... (1)
We have
Second term = Fourth term + 18
ar = ar³ + 18
⇒ ar - ar³ = 18
∴ ar(1 - r²) = 18
Dividing equation (2) by (1),

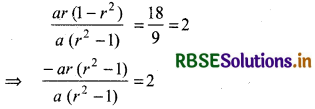
⇒ - r = 2
∴ r = - 2
So, common ratio of GP., r = - 2
Putting r = - 2.in equation (1),
a[(- 2)² - 1] = 9
or a(4 - 1) = 9
or a × 3 = 9
∴ a = 3
1^st term of progression = 3
2^nd term = ar = 3 (- 2) = - 6
3^rd term = ar² = 3(- 2)² = 12
4^th term = ar³ = 3(- 2)³ = - 24
Thus required terms of sequence are 3, - 6, 12, - 24

Question 22.
If p^th, q^th and r^th terms of G.P. are a, b and c respectively, then prove that
a^{q - r} b^{r - p} c^{p - q} = 1
Answer:
Let the first term of GP. is A and the common ratio is R.
Since n^th term of GP., T_n = ar^{n - 1}
Then p^th term = AR^{p - 1}
⇒ a = AR^{p - 1} ................ (1)
q^th term = AR^{q - 1}
⇒ b = AR^{q - 1} ................... (2)
and r^th term = AR^{r - 1}
⇒ c = AR^{r - 1} ................. (3)
From equation (1),
a = AR^{p - 1}
⇒ a^{q - r} = (AR^{p - 1})^{(q - r)}
⇒ a^{q - r} = A^{q - r} R^{(p - 1) (q - r)}

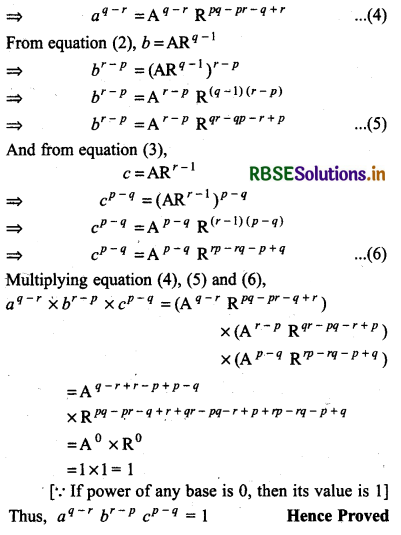
Question 23.
If the first and th term of a G.P. are a and b respectively and if P is the product of n terms, prove that
p² = (ab)ⁿ
Answer:
Let the common ratio of GP. is r.
Here, first term is a.
So, ar, ar², ar³, ...................., ar^{n - 1} are in GP.
But nth term
ar^{n - 1} = b ............. (1) [∵ Given, a_n = b]
⇒ r^{n - 1} = \(\frac{b}{a}\)
And product of all terms
a × ar × ar² × ar³ × ................. × ar^{n - 1} = P (Given)
or aⁿ [r^{1 + 2 + 3 + 4 ....... + (n - 1)}] = p

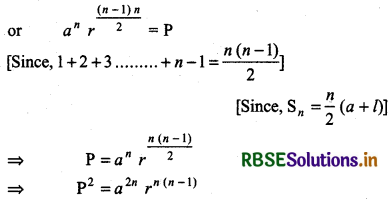
⇒ p² = (a² r^{n - 1})ⁿ
⇒ p² = (a.ar^{n - 1})ⁿ = (a × b)ⁿ
[Since b = ar^{n - 1}, from equation (1)]
Thus, P² = (ab)ⁿ
Hence Proved

Question 24.
Show that the ratio of the sum of first n terms of a GP. to the sum of terms from (n + 1)^th to (2n)^th term is \(\frac{1}{r^n}\).
Answer:
Let the first term of GP. be a and common ratio is r.
Sum of first n terms, if r < 1

Thus, sum of first n terms: sum from (n + 1)^th term to (2n)^th term = 1 : rⁿ
Hence Proved

Question 25.
If a, b, c and d are in G.P., then show that:
(a² + b² + c²) (b² + c² + d²) = (ab + bc + cd)²
Answer:
If a, b, c and d are in GP., then
common ratio \(\frac{b}{a}=\frac{c}{b}=\frac{d}{c}\) = r (say)
So, b = ar, c = br = ar²,
d = cr = br × r = br² = ar³
⇒ b = ar, c = ar², d = ar³ .................. (1)
L.H.S. = (a² + b² + c²) (b² + c² + d²)
= [a² + (ar)² + (ar²)²]
[(ar)² + (ar²)² + (ar³)²]
[From relation (1)]
=[a² + a²r² + a²r⁴] [a²r² + a²r⁴ + a²r⁶]
= a²[1 + r² + r⁴] . a²[r² + r⁴ + r⁶]
= a⁴ (1 + r² + r⁴). r² (1 + r² + r⁴)
= a⁴ r² [1 + r² + r⁴] [1 + r² + r⁴]
= a⁴ r² [1 + r² + r⁴]²
Now, R.H.S. = (ab + bc + cd)²
= [a × ar + ar × ar² + ar² × ar³]² [From ralation (1)]
= [a²r + a²r³ + a²r⁵]²
= (a² r)² [1 + r² + r⁴ ]²
= a⁴ r² [1 + r² + r⁴]² ............... (3)
From (2) and (3), it is clear that
L.H.S = R.H.S
Thus, [a² + b² + c²] [b² + c² + d²]
= [ab + bc + cd]²
Hence Proved.

Question 26.
Insert two numbers between 3 and 81 so that the resulting sequence is GP.
Answer:
Let required numbers are a and b then terms of sequence 3, a, b, 81 will be in G.P.
Common ratio r = \(\frac{a}{3}=\frac{b}{a}=\frac{81}{b}\)

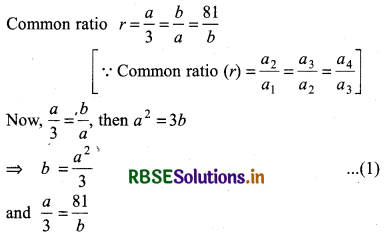
⇒ ab = 3 × 81
⇒ ab = 243
⇒ a × \(\frac{a^2}{3}\) = 243 [From equation (1)]
⇒ a³ = 243 × 3
⇒ a³ = 729
⇒ a³ = 9³
Comparing powers on both sides
a = 9
Putting a = 9, in equation (1)
b = \(\frac{9^2}{3}=\frac{81}{3}\) = 27 (∵ b = \(\frac{a^2}{3}\))
⇒ b = 27
Thus, between 3 and 81 two required numbers are 9 and 27.

Alternative Method:
Let between 3 and 81 two numbers G₁ and G₂ are such that
3, G₁, G₂, 81 are in GP.
Thus, first term a = 3 and fourth term a₄ = 81
Let common ratio of given GP. is r, then
fourth term a₄ = ar^{4 - 1}
[by formula a_n = ar^{n - 1}]
⇒ 81 = 3.r³
⇒ r³ =
⇒ r³ = 27
⇒ r³ = 3³
On comparing powers of both sides
r = 3
∴ G₁ = ar = 3 × 3 = 9
and , G₂ = ar₂ = 3 × (3)²
= 3 × 9 = 27
Thus, between 3 and 81, two required numbers are 9 and 27.

Question 27.
Find the value of n so that \(\frac{a^{n+1}+b^{n+1}}{a^n+b^n}\), may be the geometric mean between a and b.
Answer:
Geometric mean between numbers a and b

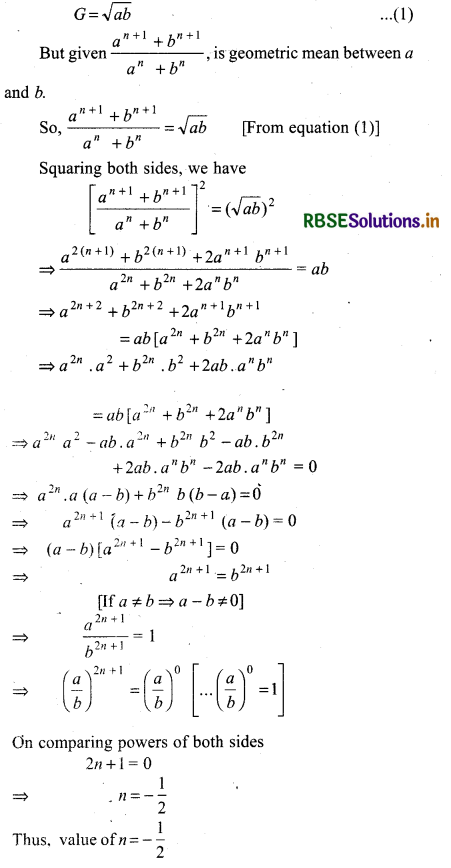
Question 28.
The sum of two numbers ¡s 6 times their G.M. Show that numbers are in the ratio
(3 + 2√2) : (3 - 2√2)
Answer:
Let the numbers be a and b.
Then their G.M. = √ab
We have: a + b = 6√ab ........... ( 1)
We know that
(a - b)² = (a + b)² - 4ab
⇒ (a - b)² = (6√ab)² - 4ab [From equation(1)]
⇒ (a - b)² = 36ab - 4ab
⇒ (a - b)² = 32ab
⇒ a - b = 4√2 √ab ........... (2)
Adding equation (1) and (2)

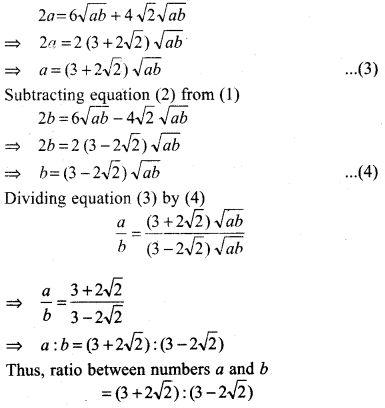
Alternative Method:
Let x and y are two numbers.
G.M. between x and y = √xy
We have, x + y = √xy × 6
⇒ x + y = 6√xy
⇒ x + y = 3 × 2√xy

Question 29.
If A and G be A.M. and G.M. respectively between two positive numbers. Prove that the numbers are A ± \(\sqrt{(A+G)(A-G)}\)
Answer:
Let, two positive numbers be a and b.
Then arithmetic mean = \(\frac{a+b}{2}\) .................. (1)
and geometric mean G = √ab ⇒ G² = ab .................. (2)
From equation (1), we have .................. (3)
(a+b)=2A
Squaring both sides, we have
⇒ (a + b)² = 4A²
⇒ a² + 2ab + b² = 4A²
⇒ a² + 2G² + b² = 4A² [From equation (2)]
⇒ a² + b² = 4A² - 2G²
⇒ a² + b² - 2ab = 4A² - 2G² - 2ab [Subtracting 2ab from both sides]
⇒ (a - b)² = 4A² - 2G² - 2G²
⇒ (a - b)² = 4A² - 4G²
∴ (a - b) = 2\(\sqrt{A^2-G^2}\)
∴ a - b = 2\(\sqrt{(A+G)(A-G)}\) .................... (4)
Adding equations (3) and (4), we have
2b = 2A - 2\(\sqrt{(A+G)(A-G)}\)
⇒ b = A - \(\sqrt{(A+G)(A-G)}\)
Thus numbers A ± \(\sqrt{(A+G)(A-G)}\)
Hence Proved.

Question 30.
The number of bacteria in a certain culture doubles every hour. If there were 30 bacteria present in the culture originally. How many bacteria will be present at the end of 2nd hour, 4th hour and n^th hour
Answer:
Initially number of bacteria a =30
Number of bacterias doubles every hour then common ratio r = 2
Now, after second hour or just before the third hour number of bacteria = ar^{3 - 1} = ar²
[∵ nth term of GP. = ar^{n - 1}]
= 30 × (2)² = 120
Similarly, after 4^th hour or just before the 5^th hour
number of bacteria = ar^{5 - 1} = ar⁴
= 30 × (2)⁴ = 480
Similarly, after nth hour or just before (n + 1)th hour, number of bacteria = ar^{(n + 1) - 1} = arⁿ
= 30 × (2)ⁿ = 30 × 2ⁿ
Thus, after 2^nd hour, number o bacteria =120
After 4^th hour, number of bacteria = 480
and after n^th hour, number of bacteria = 30 × 2ⁿ

Question 31.
What will ₹ 500 amounts to in 10 ears after its deposit ¡n a bank which pays annual interest rate of 10% compounded annually?
Answer:
Initial amount P =₹ 500
After 1 year, 10 % of P = \(\frac{P}{10}\) part
Value of P after 1 year

Question 32.
If A.M. and G.M. of roots of a quadratic equation are 8 and S respectively. Then obtain the quadratic equation.
Answer:
Let α and β be the roots of quadratic equation.
A.M. of roots = \(\frac{\alpha+\beta}{2}\) = 8
(Given A.M. of roots = 8)
∴ Sum of roots = α + β = 16 ................ (1)
∵ G.M. of roots = √αβ = 5 (Given, G.M. = 5)
Squaring both sides,
∴ Product of roots α β = 25
We know that quadratic equation
x² - (sum of roots) x + product of roots = O
⇒ x² - (α + β)x + α β = 0
⇒ x² - (16)x + 25 = 0
[From equation (1) and (2)]
⇒ x² - 16x + 25 = 0
Thus, required quadratic equation is x² - 16x + 25 = 0