RBSE Solutions for Class 11 Maths Chapter 5 Complex Numbers and Quadratic Equations Ex 5.3

Rajasthan Board RBSE Solutions for Class 11 Maths Chapter 5 Complex Numbers and Quadratic Equations Ex 5.3 Textbook Exercise Questions and Answers.

RBSE Class 11 Maths Solutions Chapter 5 Complex Numbers and Quadratic Equations Ex 5.3

Question 1.
x² + 3 = 0
Answer:
Given equation: x² + 3 = 0
or x² = - 3
∴ x = ± √- 3
= ± √3 (- 1)
= ± √3 √- 1
= ± √3i (∵ √- 1 = i)
Thus, solution of equation x = √3i and x = - √3i

Question 2.
2x² + x + 1 = 0
Answer:
Given equation 2x² + x + 1 = 0
Comparing with ax² + bx + c = 0
Discriminant of given equation = b² - 4ac
D = 1²- 4 × 2 × 1
= 1 - 8
D = - 7 < 0
Thus, solution of equation

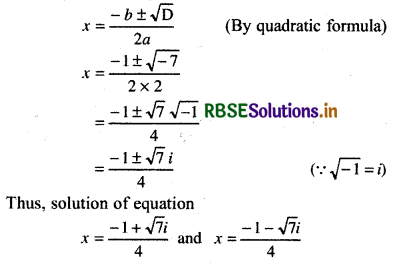
(positive and negative values are taken separately)

Question 3.
x² + 3x + 9 = 0
Answer:
Given equation: x² + 3x + 9=0.
On comparing with ax² + bx + c = 0
Here, a = 1, b = 3, c = 9
Here, discriminant of equation = b² - 4ac
D = 9 - 4 × 1 × 9
D = 9 - 36
D = - 27 < 0
Then solution of equation

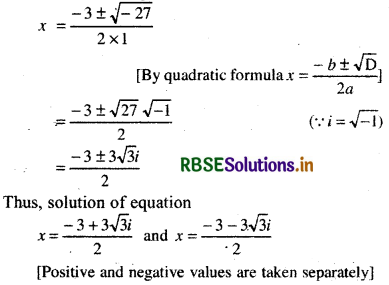
Question 4.
- x² + x - 2 = 0
Answer:
Given equation: - x² + x - 2 = 0,
On comparing with ax² + bx + c = 0
Here, a = - 1, b = 1, c = - 2
Discriminant for given equation,
= b² - 4ac
=1² - 4 × (- 1) (- 2)
= 1 - 8
D = - 7 < 0,

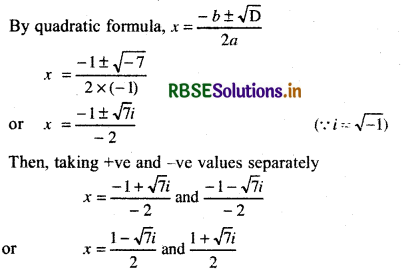
Question 5.
x² + 3x + 5 = 0
Answer:
Given equation: x² + 3x + 5 = 0
On comparing with ax² + bx + c = 0
Here, a = 1, b = 3, c = 5
Discriminant of equation = b² - 4ac
= 3² - 4 × 1 × 5
= 9 - 20
D = - 11 < 0

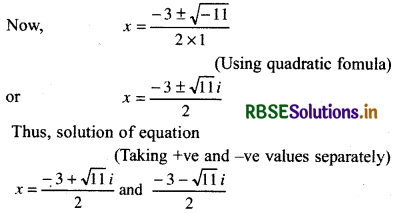
Question 6.
x² - x + 2 = 0
Answer:
Given equation: x² - x + 2 = 0
Comparing with ax² + bx + c = 0
Here, a = !1, b = - 1, c = 2
Discriminant of equation,
= b² - 4ac
= (- 1)² - 4 × 1 × 2
= 1 - 8
D = - 7 < 0

Question 7.
√2x² + x + √2 = 0
Answer:
Given equation: √2x² + x + √2 = 0
On comparing with ax² + bx + c = 0
Here, a = √2, b = 1, c = √2
Discriminant of equation = b² - 4ac
= 1² - 4 × √2 × √2
= 1 - 8
D = - 7 < 0

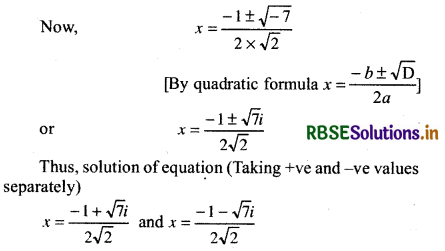
Question 8.
√3x² - √2x + 3√3 = 0
Answer:
Given equation: √3x² - √2x + 3√3 = 0
On comparing with ax² + bx + c = 0
Here, a = √3, b = - √2, c = 3√3
Discriminant of equation = b² - 4ac
= (- √2)² - 4 × √3 × 3√3
= 2 - 4 × 3 × 3 = 2 - 36
D = - 34 < 0

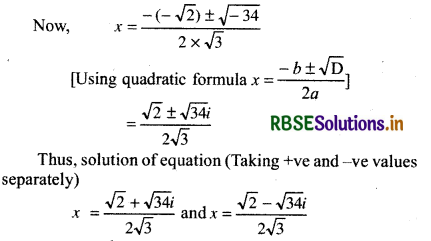
Question 9.
x² + x + \(\frac{1}{\sqrt{2}}\) = 0
Answer:
Given equation: x² + x + \(\frac{1}{\sqrt{2}}\) = 0
On comparing with ax² + bx + c = 0
Here, a = 1, b = 1, c = \(\frac{1}{\sqrt{2}}\)
Discriminant of equation = b² - 4ac

Question 10.
x² + \(\frac{x}{\sqrt{2}}\) + 1 = 0
Answer:
Given equation: x² + \(\frac{x}{\sqrt{2}}\) + 1 = 0
On comparing with ax² + bx + c = 0
Here, a = 1, b = \(\frac{1}{\sqrt{2}}\) and c = 1
Discriminant of equation = b² - 4ac