RBSE Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.4

Rajasthan Board RBSE Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.4 Textbook Exercise Questions and Answers.

RBSE Class 11 Maths Solutions Chapter 3 Trigonometric Functions Ex 3.4

Find the principal and general solutions of the following equations :

Question 1.
tan x = √3
Answer:
Since, tan \(\frac{\pi}{3}\) = √3
tan x = √3 = tan 60° = tan \(\frac{\pi}{3}\)
x = \(\frac{\pi}{3}\)
Again, tan x = √3 = tan(π + \(\frac{\pi}{3}\)) = tan\(\frac{4 \pi}{3}\)
∴ x = \(\frac{4 \pi}{3}\)

Since, value ofx less between 0 and 2π so principal solution or given equation is x = \(\frac{\pi}{3}\) or \(\frac{4 \pi}{3}\)
For general solution,
tan x = √3 = tan \(\frac{\pi}{3}\)
So, x = \(\frac{\pi}{3}\)
then general solution of x = nπ + \(\frac{\pi}{3}\), n ∈ Z

Question 2.
sec x = 2
Answer:
We know that sec \(\frac{\pi}{3}\) = 2
Thus, sec x = 2 = sec \(\frac{\pi}{3}\) = sec(2π - \(\frac{\pi}{3}\))
Thus, x = \(\frac{\pi}{3}\) or x = (2π - \(\frac{\pi}{3}\))
x = \(\frac{\pi}{3}\) or x = \(\frac{5 \pi}{3}\)
Thus, principal solution of given equation x = \(\frac{\pi}{3}\) or \(\frac{5 \pi}{3}\) since principal solution of trigonometric equation lies between 0 and 2π and for general value,
∵ sec x = 2
⇒ \(\frac{1}{cos x}\) = 2
⇒ cos x = \(\frac{1}{2}\)
⇒ cos x = cos \(\frac{\pi}{3}\)

General value x = 2nπ ± \(\frac{\pi}{3}\)
Thus, general solution x = 2nπ ± \(\frac{\pi}{3}\), n ∈ Z

Question 3.
cot x = -√3
Answer:
Since cot\(\frac{\pi}{6}\) = √3
So, cot(π - \(\frac{\pi}{6}\)) = -√3
or cot(2π - \(\frac{\pi}{6}\)) = -√3
or cot x = cot(π - \(\frac{\pi}{6}\)) or = cot (2π - \(\frac{\pi}{6}\))
x = \(\frac{5 \pi}{6}\) or \(\frac{11 \pi}{6}\)
Thus principal solution of given equatitn x = \(\frac{5 \pi}{6}\) or \(\frac{11 \pi}{6}\)
For general value cot x = -√3

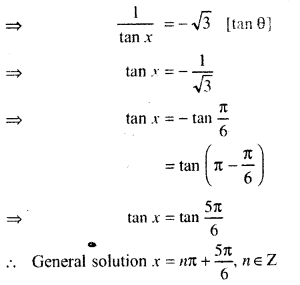
Question 4.
cosec x = -2
Answer:
cosec x = -2
Since, cosec \(\frac{\pi}{6}\) = 2

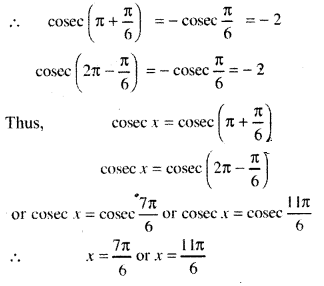
Thus, principal solution of given equation x = \(\frac{7 \pi}{3}\) or \(\frac{11 \pi}{3}\) and general equation x = nπ + (-1)ⁿ\(\frac{7 \pi}{6}\), n ∈ Z

Find the general equation for each of the following equations

Question 5.
cos 4x = cos 2x
Answer:
From given equation
cos 4x = cos 2x
cos (2nπ ± 2x) = cos 2x [∵ cos(2nπ + x) = cosx]
Thus, taking general value form equation
cos 4x = cos(2nπ ± 2x)
4x = 2nπ ± 2x

Taking '+' sign
⇒ 4x = 2nπ + 2x.
⇒ 4x - 2x = 2nπ
⇒ 2x = 2nπ,
⇒ x = nπ, n ↔ Z.

Taking '-' sign
⇒ 4x =2nπ - 2x
⇒ 4x + 2x = 2nπ
⇒ 6x = 2nπ
⇒ x = \(\frac{n \pi}{3}\), n ∈ Z
Thus, general solution of given equation
x = nπ or \(\frac{n \pi}{3}\). n ∈ Z

Question 6.
cos 3x + cos x - cos 2x = 0
Answer:
From given equation
cos 3x + cos x - cos 2x = 0
2 cos\(\frac{3 x+x}{2}\) cos\(\frac{3 x-x}{2}\) cos 2x = 0
[By formula cos x + cos y = 2 cos \(\frac{(x+y)}{2}\), cos \(\frac{(x-y)}{2}\)]
⇒ 2cos2x cosx - cos2x = 0
⇒ cos 2x(2cos x - 1) = 0
or then cos 2x = 0 or 2cos x - 1 = 0

It cos 2x = 0 = cos \(\frac{\pi}{2}\) or cos x = \(\frac{1}{2}\) = cos \(\frac{\pi}{3}\)
2x = (2n + 1)\(\frac{\pi}{2}\)
⇒ x = (2n + 1)\(\frac{\pi}{4}\), n ∈ Z
or cos x = cos\(\frac{\pi}{3}\)
x = 2mπ ± \(\frac{\pi}{3}\)
Thus general solution of given equation
x = (2n + 1)\(\frac{\pi}{4}\) or 2mπ ± \(\frac{\pi}{3}\), n. m ∈ Z

Question 7.
sin 2x + cos x = 0
Answer:
Given equation, sin 2x + cos x = 0
2 sin x cos x+cos x = 0 (∵ sin 2x = 2sin x cosx)
cos x(2sin x + 1) = 0
then cos x = 0, or 2 sin x + 1 = 0
cos x = 0, or 2 sin x = - 1
x = (2n + 1)\(\frac{\pi}{2}\) where n ∈ Z,
or sin x = -\(\frac{1}{2}\) = sin(π + \(\frac{\pi}{6}\))
∴ sin x = sin(π + \(\frac{\pi}{6}\)) ⇒ sin x = sin \(\frac{7 \pi}{6}\)
Taking general value x = \(\frac{7 \pi}{6}\), m ∈ Z
x = mπ + (-1)^m \(\frac{7 \pi}{6}\), m ∈ Z
Thus, General solution of given equation
x = mπ + (-1)^m \(\frac{7 \pi}{6}\)
(2n + 1)\(\frac{7 \pi}{2}\), n ∈ Z, m ∈ z

Question 8.
sec²2x = 1 - tan 2x
Answer:
From the equation sec²2x = 1 - tan 2x
1 + tan²x = 1 - tan 2x
tan²2x + tan 2x = 0
tan 2x(tan 2x + 1) = 0
tan 2x = 0 or tan 2x + 1 = 0

Taking general value
2x = nπ + 0 or tan2x = -1 = -tan\(\frac{\pi}{4}\)
x = \(\frac{n \pi}{2}\), n ∈ Z or tan 2x = tan(π - \(\frac{\pi}{4}\))
tan 2x = tan\( \frac{3 \pi}{4}\),

Taking general value
2x = mπ + \(\frac{3 \pi}{4}\)
x = \(\frac{m \pi}{2}+\frac{3 \pi}{8}\), m ∈ Z

Thus, general solution of given equation
x = \(\frac{n \pi}{2}\) or \(\frac{m \pi}{2}+\frac{3 \pi}{8}\), m ∈ Z

Question 9.
sin x + sin 3x + sin 5x = 0
Answer:
sin x + sin 3x + sin 5x = 0
sin 3x + 2sin\(\frac{5 x+x}{2}\) cos\(\frac{5 x-x}{2}\) = 0
{∵ sin x + sin y = 2 sin\(\frac{x+y}{2}\)cos\(\frac{x-y}{2}\)}
sin3x + 2sin3xcos2x = 0
sin3x(1 + 2cos2x) = 0
If sin 3x = 0
3x = nπ
x = \(\frac{n \pi}{2}\), n ∈ Z
If 1 + 2cos2x = 0
2 cos 2x = -1
cos 2x = -\(\frac{1}{2}\)
cos 2x = cos(π - \(\frac{\pi}{3}\)) = cos\(\frac{2 \pi}{4}\)
cos 2x = cos\(\frac{2 \pi}{4}\) (Taking general value)
2x = 2mπ ± \(\frac{2 \pi}{3}\)
x = mπ ± \(\frac{2 \pi}{3}\), m ∈ Z

Thus general solution of given equation
x = \(\frac{n \pi}{3}\), or mπ = ±\(\frac{\pi}{3}\), n, m ∈ Z