RBSE Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.2
Rajasthan Board RBSE Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.2 Textbook Exercise Questions and Answers.
Rajasthan Board RBSE Solutions for Class 11 Maths in Hindi Medium & English Medium are part of RBSE Solutions for Class 11. Students can also read RBSE Class 11 Maths Important Questions for exam preparation. Students can also go through RBSE Class 11 Maths Notes to understand and remember the concepts easily.
RBSE Class 11 Maths Solutions Chapter 3 Trigonometric Functions Ex 3.2
Find the values of other five trigonometric functions. In exercise 1 to 5.
Question 1.
cos x = \(\frac{1}{2}\), x lies in third quadrant.
Answer:
We have cos x = -\(\frac{1}{2}\) and x lies in third quadrant
Thus, sec x = -\(\frac{2}{1}\) = -2
Now sin2x + cos2x = 1
or sin2x = 1 - cos2x = 1 - \(\frac{1}{4}=\frac{3}{4}\)
∴ sin x = ±\(\frac{\sqrt{3}}{2}\)
(If x Lies in third quadrant than 180° ≤ x ≤ 270°, (π ≤ x ≤ \(\frac{3}{2}\)π) Thus, here only tan and cot will be positive all other trigonometric functions will be negative) Since x lies in third quadrant so value ofsin x will be negative, Thus,
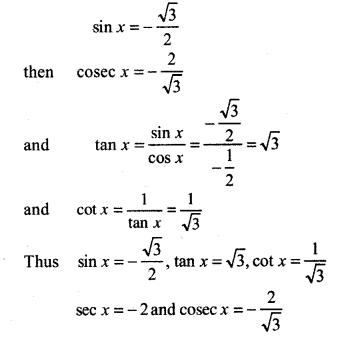
Question 2.
sin x = \(\frac{3}{5}\), x lies in second quadrant.
Answer:
Given, sin x = \(\frac{3}{5}\), and cosec x = \(\frac{5}{3}\)
since, sin2x + cos2x = 1
or cos2x = 1 - sin2x
= 1 - \(\left(\frac{3}{5}\right)^2\) = 1 - \(\frac{9}{25}\) or
cos2x = \(\frac{16}{25}\)
∴ cos x = ±\(\frac{4}{5}\)
For second quadrant 90° ≤ x ≤ 180°(\(\frac{\pi}{2}\) ≤ x ≤ π), in this sin x and cosec x will be positive rest will be negative according to ASTC
Since, x lies in second quadrant
Thus, cos will be negative
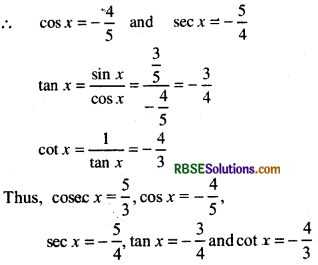

Question 3.
cot x = \(\frac{3}{4}\), x lies in third quadrant.
Answer:
cot x = \(\frac{3}{4}\), so tan x = \(\frac{4}{3}\)
cosec2x = 1 + cot2x
= 1 + \(\left(\frac{3}{4}\right)^2\) = 1 + \(\frac{9}{16}=\frac{25}{16}\)
∴ cosec x = ±\(\frac{4}{5}\)
(According to ASTC law, In third quadrant, except trigonometric functions of tan and cot all other trigonometric function will be negative)
cosec x = -\(\frac{5}{4}\) then sin x = -\(\frac{4}{5}\)
Now, cos2x + sin2x = 1
cos2x = 1 - sin2x
= 1 - \(\frac{16}{25}=\frac{9}{25}\)
cos x = ±\(\frac{3}{5}\)
In third quadrant, cos is negative
Thus, cos x = -\(\frac{3}{5}\) then sec x = -\(\frac{5}{3}\)
Thus, tan x = \(\frac{4}{3}\), sin x = -\(\frac{4}{5}\), cosec x = -\(\frac{5}{4}\)
cos x = -\(\frac{3}{5}\) and sec x = - \(\frac{5}{3}\)
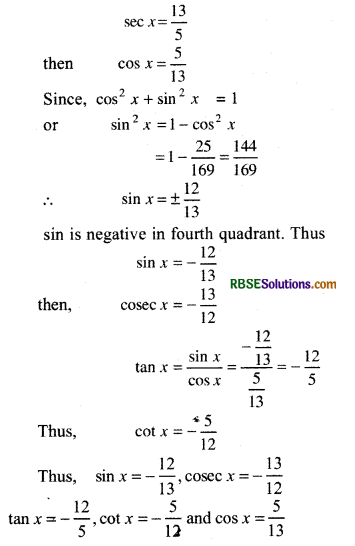
Question 4.
sec x = \(\frac{13}{5}\), x lies in fourth quadrant.
Answer:
Question 5.
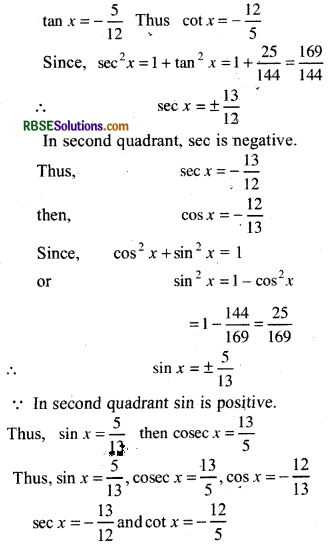
tan x = -\(\frac{5}{12}\), x lies in second quadrant.
Answer:
Question 6.
Find the value of sin 765°.
Answer:
We know that values of sin repeats after an interval or 2π
Thus, sin(765°) = sin(720° + 45°)
= sin (2 × 360° + 45°) = sin 450
(∵ After repeat it will come in first quadrant)
= \(\frac{1}{\sqrt{2}}\) Thus sin 765° = \(\frac{1}{\sqrt{2}}\)
Question 7.
Find the values of cosec(- 1410°)
Answer:
We know that values of cosec x repeats after an interval or 2π.
Thus, sec(-1410°) = cosec(-1410° + 4 × 360°)
= cosec (-1410° + 1440°)
= cosec 30°
(After repat this angle will shift to first quadrant)
= 2
Thus, cosec(-1410°) = 2
(Note : 4 × 360° is’taken since its approximate value is 1410°)
Question 8.
Find the value of tan 19 it
Answer:
We know that the values of tan x repeats after an interval of π. Therefore
tan \(\frac{19 \pi}{3}\) = tan(\(\frac{\pi}{3}\) + 6π) = tan(3 × 2π + \(\frac{\pi}{3}\))
= tan \(\frac{\pi}{3}\)
(After repeat this angle will shift to first quadrant and all trigonometric ratios are positive in first quadrant)
= √3
Thus tan \(\frac{19 \pi}{3}\) = √3
Question 9.
Find the value of sin\(\left(-\frac{11 \pi}{3}\right)\)
Answer:
We know that the values of sin x repeats after an interval of 2π.
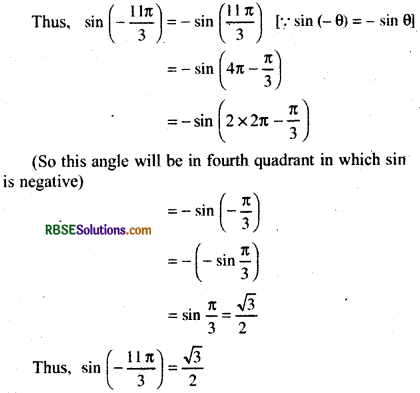

Question 10.
Find the value of cot\(\left(-\frac{15 \pi}{4}\right)\)
Answer:
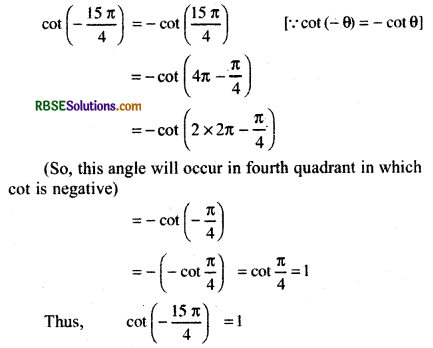

- RBSE Solutions for Class 11 Maths Chapter 3 त्रिकोणमितीय फलन Ex 3.1
- RBSE Solutions for Class 11 Maths Chapter 2 संबंध एवं फलन विविध प्रश्नावली
- RBSE Solutions for Class 11 Maths Chapter 2 संबंध एवं फलन Ex 2.3
- RBSE Solutions for Class 11 Maths Chapter 2 संबंध एवं फलन Ex 2.2
- RBSE Solutions for Class 11 Maths Chapter 2 संबंध एवं फलन Ex 2.1
- RBSE Solutions for Class 11 Maths Chapter 1 समुच्चय विविध प्रश्नावली
- RBSE Solutions for Class 11 Maths Chapter 1 समुच्चय Ex 1.6
- RBSE Solutions for Class 11 Maths Chapter 1 समुच्चय Ex 1.5
- RBSE Solutions for Class 11 Maths Chapter 1 समुच्चय Ex 1.4
- RBSE Solutions for Class 11 Maths Chapter 1 समुच्चय Ex 1.3
- RBSE Solutions for Class 11 Maths Chapter 1 समुच्चय Ex 1.2