RBSE Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.1

Rajasthan Board RBSE Solutions for Class 11 Maths Chapter 3 Trigonometric Functions Ex 3.1 Textbook Exercise Questions and Answers.

RBSE Class 11 Maths Solutions Chapter 3 Trigonometric Functions Ex 3.1

Question 1.
Find the radian measures corresponding to the following degree measures.
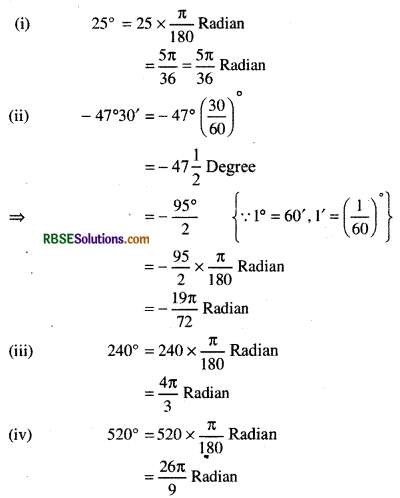
(i) 25°
(ii) - 47°30'
(iii) 240°
(iv) 520°
Answer:

Question 2.
Find the degree measures corresponding to the following radian measures (Use π = \(\frac{22}{7}\))
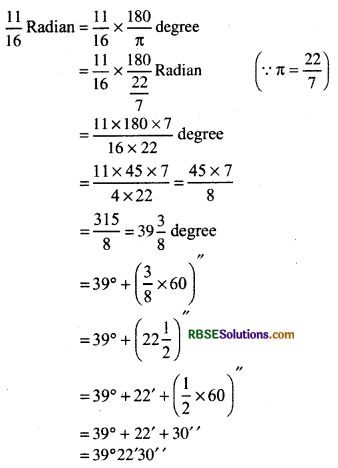
(i) \(\frac{11}{16}\)
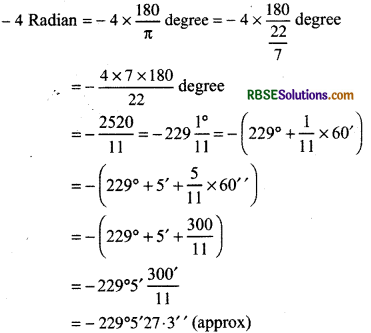
(ii) - 4
(iii) \(\frac{5 \pi}{3}\)
(iv) \(\frac{7 \pi}{6}\)
Answer:
(i) \(\frac{11}{16}\)

(ii) - 4

(iii) \(\frac{5 \pi}{3}\)
\(\frac{5 \pi}{3}=\frac{5 \pi}{3} \times \frac{180}{\pi}\)degree
= 300°

(iv) \(\frac{7 \pi}{6}=\frac{7 \pi}{6} \times \frac{180}{\pi}\) degree = 210°

Question 3.
A wheel makes 360 revolutions in one minute. Through how many radians does it turn in one second?
Answer:
Given, wheel makes 360 revolution in 1 minute.
So, in 60 seconds 360 revolution, then
In 1 second = \(\frac{360}{60}\) = 6 revolution
Angie formed in 1 revolution = 2π Radian
then angle formed in 6 revolution
= 6 × 2π Radian = 12π Radian
Thus, Angle formed by wheel in 1 second = 12π Radian

Question 4.
Find the degree measure of the angle subtended at the centre of a circle of radius 100 cm. by an arc of length 22 cm. (Use π = \(\frac{22}{7}\)).
Answer:
Given radius of circle (r) = 100 cm
Length of arc (l) = 22 cm

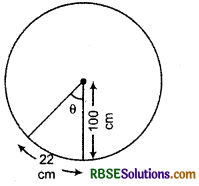
Angle formed at centre (θ) = \(\frac{l}{r}\) Radian

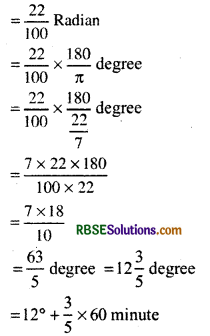
[∵ 1° = 60 minute]
= 12°36'
Thus, Angle formed at centre of circle by given arc
= 12°36'

Question 5.
In a circle of diameter 40 cm. the length of a chord is 20 cm. Find the length of minor arc of the chord.
Answer:
Given diameter of circle = 40 cm
Radius of circle (r) = 20 cm
Let AB be a chord of circle whose length is 20 cm.
By joining centre of circle O with A and B, an equilateral triangle OAB is obtained.
∠AOB = 60° = \(\frac{\pi}{3}\) Radian 3
Let length of arc ACB is l
Then ∵ l = r θ {where θ is in radian}

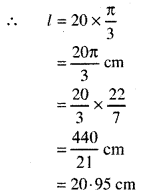
Thus, length of arc corresponding to chord of circle.
= \(\frac{20 \pi}{3}\) cm. or 20.95 cm

Question 6.
If in two circles, arcs of the same length subtend angles 60° and 75° at the centre, find the ratio of their radii.
Answer:
Let r₁ and r₂ are radii of circle.

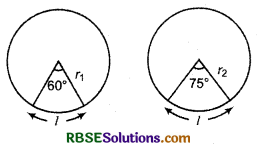
Then, angle subtended by an arc of one circle at the centre.
θ = 60° = \(\frac{\pi}{3}\)Radian
Angle subtend by an arc of second circle at the centre = 75°
By formula: Length of arc l = Radius (r) × angle (θ)
∴ Length of arc of first circle = \(\frac{\pi}{12}\) × r₁
Length of arc of second circle = \(\frac{5 \pi}{12}\) × r₂

Arc of two circles are equal.
Thus, \(\frac{\pi}{3}\) × r₁ = \(\frac{5 \pi}{12}\) × r₂
or \(\frac{r_1}{r_2}=\frac{5 \times 3}{12}=\frac{5}{4}\)
∴ r₁ : r₂ = 5: 4
Thus, ratio of radii of circles
r₁: r₂ = 5 : 4

Question 7.
Find the angle in radian through which a pendulum swings if its length is 75 cm and the tip describes an arc of length.
(i) 10 cm
(ii) 15 cm
(iii) 21 cm
Answer:
(i) Length of pendulum (r) = 75 cm
Length of arc (l) = 10 cm
Let pendulum angle is θ
then θ = \(\frac{l}{r}=\frac{10}{75}\) Radian = \(\frac{2}{15}\) Radian

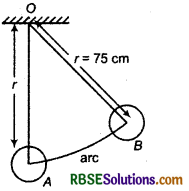
Thus, angle formed by oscillation of pendulum Radian

(ii) Length of pendulum (r)= 75 cm
length of arc (l) = 15 cm
Let, oscillation angle is θ, then
θ = \(\frac{l}{r}=\frac{15}{75}\) Radian
= \(\frac{1}{5}\) Radian
Thus, angle formed by oscillation of pendulum = \(\frac{1}{5}\) Radian

(iii) length of pendulum (r) = 75 cm
length of arc (l) = 21 cm
Let oscillation angle is θ, then
θ = \(\frac{l}{r}=\frac{21}{75}\) Radian
= \(\frac{7}{25}\) Radian
Thus, required angle = \(\frac{7}{25}\)Radian