RBSE Solutions for Class 11 Maths Chapter 12 Introduction to three Dimensional Geometry Miscellaneous Exercise

Rajasthan Board RBSE Solutions for Class 11 Maths Chapter 12 Introduction to three Dimensional Geometry Miscellaneous Exercise Textbook Exercise Questions and Answers.

RBSE Class 11 Maths Solutions Chapter 12 Introduction to three Dimensional Geometry Miscellaneous Exercise

Question 1.
Three vertices of a parallelogram A(3, - 1, 2), 8(1, 2, - 4) are C(- 1, 1, 2). Find the coordinates of fourth vertex D.
Answer:
Let A(x₁, y₁, z₁) = 4(3, - 1, 2)
B(x₂, y₂, z₂) = B(1, 2, - 4)
C(x₃, y₃, z₃) = C(- 1, 1, 2)
Let the coordinates of point D are (x₄, y₄, z₄) and M is the mid-point the of the diagonals AC and BD.

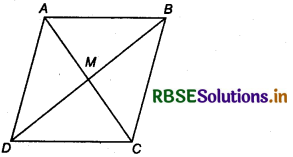
Coordinates of mid-point of diagonal AC

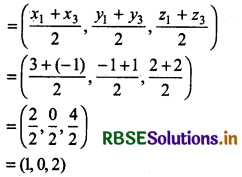
So, coordinates of point M = (1, 0, 2)
Coordinates of mid-point of diagonal BD

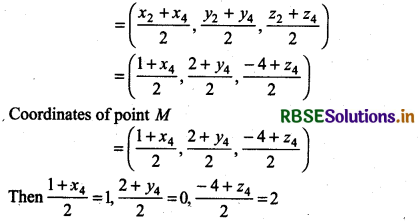
⇒ 1 + x₄ = 1 × 2, 2 + y₄ = 2 × 0, - 4 + z₄ = 2 × 2
⇒ 1 + x₄ = 2, 2 + y₄ = 0, - 4 + z₄ = 4
⇒ x₄ = 2 - 1 = 1, y₄ = - 2, z₄ = 4 + 4 = 8
Then D(x₄, y₄, z₄) = (1, - 2, 8)
Thus, the coordinates of fourth vertex D are (- 1, - 2, 8)

Question 2.
Find the length of the medians of the triangle with vertices A(0, 0, 6), B(0, 4, 0) and C(6, 0, 0).
Answer:
Let mid-point of sides BC, AC and AB of the triangle are D, E and F respectively.
Then D = mid-point of BC

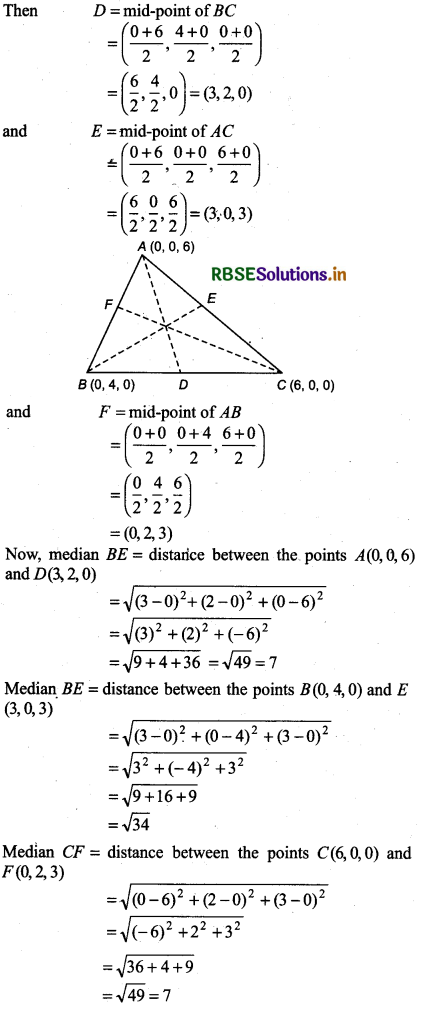
Thus, lengths of the medians of the triangle are 7 units, √34 units, 7 units

Question 3.
If the origin is the centroid of the triangle PQR with vertices P(2a, 2, 6), Q(- 4, 3b, - 10) and R(8, 14, 2c), then find the values of a, b and c.
Answer:
We know that coordinates of centroid of triangle are \(\left(\frac{x_1+x_2+x_3}{3}, \frac{y_1+y_2+y_3}{3}, \frac{z_1+z_2, z_3}{3}\right)\) where (x₁, y₁, z₁), (x₂, y₂, z₂) and (x₃, y₃, z₃) are vertices of triangle
Here, centroid is origin (0, 0, 0).

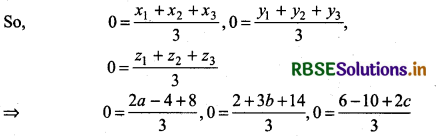
Since (x₁, y₁, z₁) = (2a, 2, 6),
(x₂, y₂, z₂) = (- 4, 3b, - 10)
and (x₃, y₃, z₃) = (8, 14, 2c)
Then 3 × 0 = 2a + 4, 0 × 3 = 3b + 16, 0 × 3 = 2c - 4
⇒ 2a + 4 = 0, 3b + 16 = 0, 2c - 4 = 0
2a = - 4, 3b = - 16, 2c = 4
or a = -\(\frac{4}{2}\), b = -\(\frac{16}{3}\), c = \(\frac{4}{2}\) = 2
Thus, a = - 2, b = - \(\frac{16}{3}\) and c = 2

Question 4.
Find the coordinates of a point on y-axis which are at a distance of 5√2 from the point P(3, - 2, 5).
Answer:
Let (0, y, 0) be the coordinates of any point on y-axis.
Let point Q lie on y axis and its coordinates are (0, y, 0).
Distance between point P and point Q is

But given distance = 5√2
So, 5√2 = \(\sqrt{(-3)^2+(y+2)^2+(-5)^2}\)
squaring both sides, we have .
(5√2)² = \(\left[\sqrt{9+(y+2)^2+25}\right]^2\)
⇒ 50 = 9 + y² + 4y + 4 + 25
⇒ y² + 4y + 38 - 50 = 0
⇒ y² + 4y - 12 = 0
⇒ y² + (6 - 2)y - 12 = 0
⇒ y² + 6y - 2y - 12 = 0
⇒ y(y + 6) - 2(y + 6) = 0
⇒ (y + 6) (y - 2) = 0
⇒ y + 6 = 0 and y - 2 = 0
∴ y = - 6 and y = 2
Thus, coordinates of required point = (0, 2, 0) or (0, - 6, 0).

Question 5.
A point R with x-coordinates 4 lies on the line segment joining the points P(2, -3, 4) and Q(8, 0, 10). Find the coordinates of the point R.
[Hint : Suppose R divides PQ in the ratio K : 1. The coordinates of the point R are given by \(\left(\frac{8 K+2}{K+1}, \frac{-3}{K+1}, \frac{10 K+4}{K+1}\right)\)]
Answer:
Let point R divides the line segment PQ in the ratio k : 1 and is R (x, y, z)
Again P(x₁, y₁, z₁) = P(2, -3, 4)
and Q(x₂, y₂, z₂) = Q(8, 0, 10)
Then x = \(\frac{k x_2+x_1}{k+1}\)
It is given that, x-coordinate of R is 4
Thus, 4 = \(\frac{k x_2+x_1}{k+1}=\frac{k \times 8+2}{k+1}\)
⇒ 4 = \(\frac{8 k+2}{k+1}\) = \(\frac{k \times 8+2}{k+1}\)
⇒ 4 = \(\frac{8 k+2}{k+1}\)
⇒ 4(k + 1) = 8k + 2
⇒ 4k + 4 = 8k + 2
⇒ 8k - 4k = 4 - 2
⇒ k = \(\frac{2}{4}=\frac{1}{2}\)
Thus k : 1 = 1 : 2
Then m₁ : m₂ = 1 : 2,
m₁ + m₂ = 1 + 2 = 3

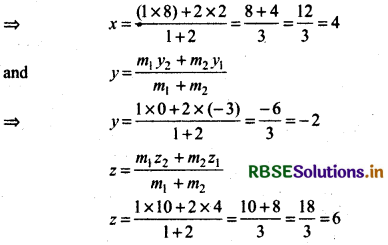
ThenR(x, y, z) = R(4, - 2, 6)
Thus, the coordinates of point R are (4, - 2, 6)

Question 6.
If A and B be the points(3, 4, 5) and (- 1, 3, - 7) respectively. Find the equation of the set of point P such that PA² + PB² = k², where k is a constant.
Answer:
Let the coordinates of variable point P are (x, y, z)
and A(x₁, y₁, z₁) = A(3, 4, 5)
B(x₂, y₂, z₂) = B(- 1, 3, - 7)
Then , PA = \(\sqrt{\left(x-x_1\right)^2+\left(y-y_1\right)^2+\left(z-z_1\right)^2}\)
PA = \(\sqrt{(x-3)^2+(y-4)^2+(z-5)^2}\)
Squaring both sides, we have
PA² = (x - 3)² + (y - 4)² + (z - 5)²
⇒ PA = x² - 6x + 9 + y - 8y + 16 + z - 10z + 25
⇒ PA = x² + y² + z² - 6x - 8y - 10z + 50
⇒ PB = \(\sqrt{(x+1)^2+(y-3)^2+(z+7)^2}\)
Squaring both sides, we have
PB² = (x + 1)² + (y - 3)² + (z + 7)²
⇒ PB² = x² + 2x + 1 + y² - 6y + 9 + z² + 14z + 49
⇒ PB² = x² + y² + z² + 2x - 6y + 14z + 59
Given, PA² + PB² = k²
So x² + y² + z² - 6x - 8y - 10z + 50 + x² + y² + z² + 2x - 6y + 14z + 59 = k²
⇒ 2x² + 2y² + 2z² - 4x - 14y + 4z = k² - 109
⇒ 2(x² + y² + z² - 2x - 7y + 2z) = k² - 109
⇒ 2(x² + y² + z² - 2x - 7y + 2z) = k² - 109
⇒ x² + y² + z² - 2x - 7y + 2z = \(\frac{k^2-109}{2}\)
Thus the equation of the set formed by variable-point P is
x² + y² + z² - 2x - 7y + 2z = \(\frac{k^2-109}{2}\)