RBSE Solutions for Class 11 Maths Chapter 12 Introduction to three Dimensional Geometry Ex 12.3

Rajasthan Board RBSE Solutions for Class 11 Maths Chapter 12 Introduction to three Dimensional Geometry Ex 12.3 Textbook Exercise Questions and Answers.

RBSE Class 11 Maths Solutions Chapter 12 Introduction to three Dimensional Geometry Ex 12.3

Question 1. 
Find the coordinates of the point which divides the line segment joining the points (- 2, 3, 5) and (1, - 4, 6) in the ratio (i) 2 : 3 internally (ii) 2 : 3 externally.
Answer:
(i) Let point A(x₁, y₁, z₁) = A(- 2, 3, 5) and B(x₂, y₂, z₂) = B(1, - 4, 6)
Let point P(x, y, z) divides the line segment AB in the ratio 2 : 3, then

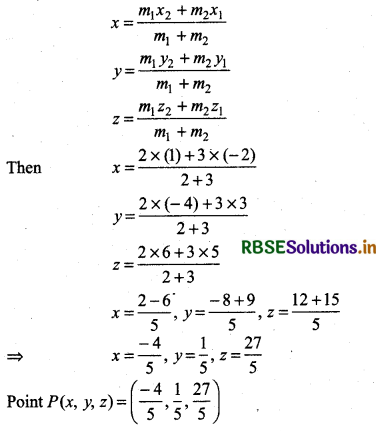
(ii) Let point P(x, y, z) divides line segment joining the points (- 2, 3, 5) and (1, - 4, 6) internally in the ratio 2 : 3 then

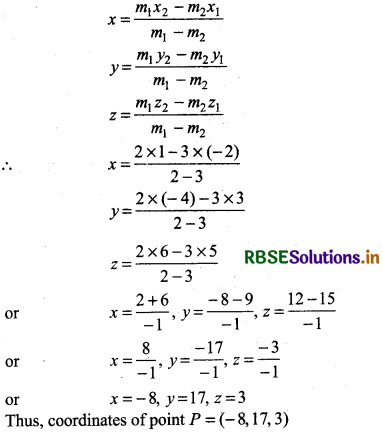
Question 2.
Given that P(3, 2, - 4) Q(5, 4, - 6) and R(9, 8, - 10) are collinear. Find the ratio in which Q divides PR.
Answer:
Let point P(x₁, y₁, z₁) = P(3, 2, - 4)
⇒ R(x₂, y₂, z₂) = R(9, 8, - 10)
and Q(x, y, z) = Q(5, 4, - 6)
Let point Q divides line segment PR in the ratio m₁ : m₂

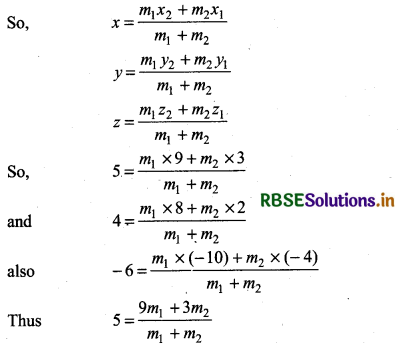
⇒ 5(m₁ + m₂) = 9m₁ + 3m₂
⇒ 5m₁ + 5m₂ = 9m₁ + 3m₂
⇒ 5m₂ - 3m₁ = 9m₁ + 5m₁
⇒ 2m₂ = 4m₁
⇒ \(\frac{m_2}{m_1}=\frac{4}{2}\)
⇒ \(\frac{m_1}{m_2}=\frac{2}{4}=\frac{1}{2}\)
⇒ m₁ : m₂ = 1 : 2
Thus, the required ratio = 1 : 2

Question 3.
Find the ratio in which the YZ-plane divides the line segment formed by joining the points (- 2, 4, 7) and (3, - 5, 8).
Answer:
Let A(x₁, y₁, z₁) = A(- 2, 4, 7)
Then B(x₂, y₂, z₂) = B(3, - 5, 8)
Let coordinates of point P is YZ-plane are (0, y, z) and plane YZ divides the line segment joining the points A and B in the ratio m₁ : m₂
So, 0 = \(\frac{m_1 x_2+m_2 x_1}{m_1+m_2}\)
⇒ 0 = \(\frac{m_1 \times(3)+m_2 \times(-2)}{m_1+m_2}\)
⇒ 3m₁ + m₂ (- 2) = 0
⇒ 3m₁ - 2m₂ = 0
⇒ 3m₁ = 2m₂
⇒ \(\frac{m_1}{m_2}=\frac{2}{3}\)
Thus, m₁ : m₂ = 2 : 3

Question 4.
Using section formula show that the points A(2, -3, 4), B(- 1, 2, 1) and c(0, \(\frac{1}{3}\), 2) are collinear.
Answer:
Let point P(x, y, z) divides the line segment AB in the ratio k : 1.
Here A(x₁, y₁, z₁) = A(2, - 3, 4)
and B(x₂, y₂, z₂) = B(- 1, 2, 1)

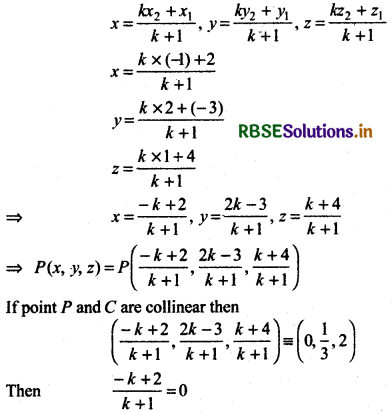
⇒ - k + 2 = 0
⇒ - k = - 2
⇒ k = 2
So, k : 1 = 2 : 1
So, (0, \(\frac{1}{3}\), 2) divides line segment AB in the ratio 2 : 1
Thus, point A, B and C are collinear.
Hence proved.

Question 5.
Find the coordinates of the points which trisect the line segment joining the points P(4, 2, - 6)and Q(10, - 16, 6)
Answer:
Let A and B be two points on line segment PQ and which trisect the line segment PQ

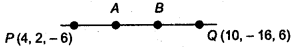
i.e., PA = AB = BQ
Then PA : AQ = 1 : 2
and PB : BQ = 2 : 1
Let P(4, 2, - 6) = P(x₁, y₁, z₁)
and Q(10, - 16, 6) = Q(x₂, y₂, z₂)
So, coordinates of points A (x, y, z)

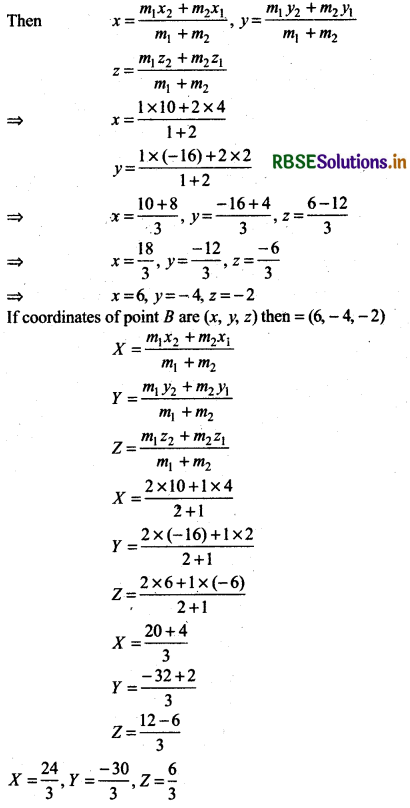
∴ x = 8, y = - 10, z = 2
Then coordinate on point B = (8, - 10, 2)
Thus, coordinate of the points which trisect the line segment are (6, - 4, - 2) and (8, - 10, 2).