RBSE Solutions for Class 9 Maths Chapter 4 दो चरों वाले रैखिक समीकरण Ex 4.4

Rajasthan Board RBSE Solutions for Class 9 Maths Chapter 4 दो चरों वाले रैखिक समीकरण Ex 4.4 Textbook Exercise Questions and Answers.

RBSE Class 9 Maths Solutions Chapter 4 दो चरों वाले रैखिक समीकरण Ex 4.4

प्रश्न 1.
(i) एक चर वाले (ii) दो चर वाले समीकरण के रूप में y = 3 का ज्यामितीय निरूपण कीजिए।
हल:
(i) एक चर वाले समीकरण के रूप में y = 3 को संख्या रेखा पर निरूपित किया गया है।

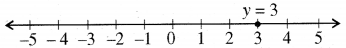
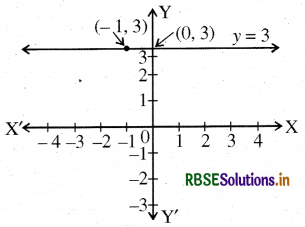
(ii) दो चर वाले समीकरण के रूप में y = 3 को कार्तीय निर्देशांक पद्धति से निरूपण इस प्रकार है

प्रश्न 2.
(i) एक चर वाले (ii) दो चर वाले समीकरण के रूप में 2x + 9 = 0 का ज्यामितीय निरूपण कीजिए।
हल:
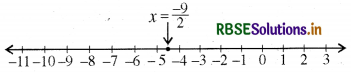
(i) एक चर वाले समीकरण के रूप में 2x + 9 = 0 को संख्या रेखा पर निरूपण किया गया
2x + 9 = 0
2x = - 9
x = \(\frac{-9}{2}\)

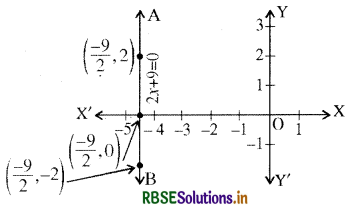
(ii) 2x + 9 = 0 अर्थात् x = \(\frac{-9}{2}\) को दो चर वाले समीकरण के रूप में कार्तीय निर्देशांक पद्धति में निरूपित किया जाता है।

2x + 9 = 0 को 2x + 0.y + 9 = 0 के रूप में लिख सकते हैं।
अब हम y के सभी मान लेंगे
चूँकि 0.y = 0 लेकिन x के द्वारा इस सम्बन्ध को सन्तुष्ट करना आवश्यक है
2x + 9 = 0
अर्थात् x = \(\frac{-9}{2}\)
अतः दी गई समीकरण के तीन हल हैं।

x =\(\frac{-9}{2}\), y = 0
x = \(\frac{-9}{2}\) =2, y = 2
और x = \(\frac{-9}{2}\), y = -2
बिन्दुओं (\(\frac{-9}{2}\).0). (\(\frac{-9}{2}\), 2), (\(\frac{-9}{2}\), -2) को ज्यामितीय निरूपण करने पर और इनको मिलाने पर रेखा AB, Y-अक्ष से बायीं ओर \(\frac{9}{2}\), की दूरी पर प्राप्त होती है।