RBSE Solutions for Class 6 Maths Chapter 7 Fractions InText Questions
Rajasthan Board RBSE Solutions for Class 6 Maths Chapter 7 Fractions InText Questions Textbook Exercise Questions and Answers.
Rajasthan Board RBSE Solutions for Class 6 Maths in Hindi Medium & English Medium are part of RBSE Solutions for Class 6. Students can also read RBSE Class 6 Maths Important Questions for exam preparation. Students can also go through RBSE Class 6 Maths Notes to understand and remember the concepts easily. Students are advised to practice अनुपात और समानुपात के प्रश्न class 6 of the textbook questions.
RBSE Class 6 Maths Solutions Chapter 7 Fractions InText Questions
(Try These Page 137)
Question 1.
Show \(\frac{3}{5}\) on a number line.
Answer:
∵ \(\frac{3}{5}\) is an proper fraction so it should lie between 0 and 1.
For representing \(\frac{3}{5}\) on number line we divide the portion between 0 and 1 into 5 equal parts
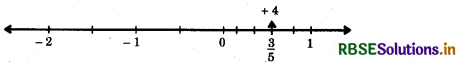
Question 2.
Show \(\frac{1}{10}, \frac{0}{10}, \frac{5}{10}\) and \(\frac{10}{10}\) on a number line.
Answer:
To locate \(\frac{1}{10}, \frac{0}{10}, \frac{5}{10}\) and \(\frac{10}{10}\) number line, we divide the portion between 0 and 1 into 10 equal parts.
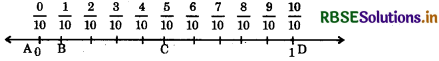
Point A represents \(\frac{0}{10}\), point B represents \(\frac{1}{10}\), point C represents \(\frac{5}{10}\) and point D represents \(\frac{10}{10}\).
Question 3.
Can you show any other fraction between 0 and 1?
Write five more fractions that you can show and depict them on the number line.
Answer:
Yes, we can locate infinite numbers between 0 and 1.
Let \(\frac{4}{7}, \frac{5}{6}, \frac{3}{4}, \frac{1}{2}, \frac{7}{8}\) are same fractions.
Let us locate these fractions on the number line.

(i) \(\frac{4}{7}\), we divide the gap between 0 and 1 into 7 equal parts then take 4 parts.

(ii) \(\frac{5}{6}\), we divide the gap between 0 and 1 into 6 equal parts, then take 5 parts.
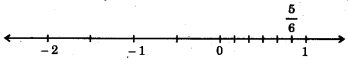
(iii) \(\frac{3}{4}\),we divide the gap between 0 and 1 into four equal parts, then take 3 parts.

(iv) \(\frac{1}{2}\) we divide the gap between 0 and 1 into two equal parts then take one part.

(v) \(\frac{7}{8}\), we divide the gap between 0 and 1 into 8 equal parts then take 7 parts.

Question 4.
How many fractions lie between 0 and 1? Think, discuss and write your answer.
Answer:
There are infinite fractions between 0 and 1.
(Try These Page No: 138)
Question 1.
Give a proper fraction :
(a) Whose numerator is 5 and denominator is 7.
Answer:
Numerator of fraction = 5
Denominator of fraction = 7
∴ Required fraction = \(\frac{\text { Numerator }}{\text { Denominator }}=\frac{5}{7}\)
(b) Whose denominator is 9 and numerator is 5.
Answer:
Numerator of fraction = 5
Denominator of fraction = 9
∴ Required fraction = \(\frac{\text { Numerator }}{\text { Denominator }}=\frac{5}{9}\)

(c) Whose numerator and denominator add up to 10. How many fractions of this kind can you make ?
Answer:
If sum of numerator and denominator is 10, then equal.
∵ 0 + 10 = 1 + 9, 2 + 8 = 3 + 7, 4 + 6 = 10
Therefore, required fractions are
\(\frac{0}{10}, \frac{1}{9}, \frac{2}{8}, \frac{3}{7}\) and \(\frac{4}{6}\).
(d) Whose denominator is 4 more than the numerator.
(How many more can you make? Give any five.)
Answer:
We can mate infinite numbers of fractions whose denominator is 4 more than the numerator, some of them are \(\frac{5}{9}, \frac{1}{5}, \frac{2}{6}, \frac{3}{7}\) and so on.
Other five examples are :
(1) Fraction whose numerator is 11 and denominator is 12.
Answer:
Numerator of fraction = 11
Denominator of fraction = 12
Required fraction = \(\frac{\text { Numerator }}{\text { Denominator }}=\frac{11}{12}\)

(2) Fraction whose denominator is 7 and numerator is 9.
Answer:
Numerator of fraction = 9
Denominator of fraction = 7
Required fraction = \(\frac{\text { Numerator }}{\text { Denominator }}=\frac{9}{7}\)
(3) Fraction whose denominator is 7 more than the numerator.
Answer:
We can make infinite numbers of fraction whose denominator is 7 more than the numerator, some of them are \(\frac{2}{9}, \frac{3}{10}, \frac{4}{11}, \frac{5}{12}, \frac{7}{14}\) and so on.
(4) Fraction whose denominator is 15 and numerator is 20.
Answer:
Denominator = 15
Numerator = 20
Required fraction = \(\frac{\text { Numerator }}{\text { Denominator }}=\frac{20}{15}\)
(5) Fraction whose numerator is 5 more than the denonirator.
Answer:
We can make infinite numbers of fraction whose denominator is 5 more than the numerator, some of them are: \(\frac{2}{7}, \frac{4}{9}, \frac{5}{10}, \frac{7}{12}, \frac{9}{14}\) and so on.
Question 2.
A fraction is given. How will you decide, by just looking at it, whether, the fraction is:
(a) less than 1?
Answer:
If numerator is less than the denominator, i.e. if it is a proper fraction, then the fraction is less than 1.
(b) equal to 1?
Answer:
If numerator and denominator of a fraction becomes equal then fraction is equal to 1.
Question 3.
Fill up using one of these: '>', '<' or '='
(a) \(\frac{1}{2}\) ________ 1
Answer:
\(\frac{1}{2}\) < 1
(b) \(\frac{3}{5}\) ________ 1
Answer:
\(\frac{3}{5}\) < 1
(c) 1 _______ \(\frac{7}{8}\)
Answer:
1 > \(\frac{7}{8}\)
(d) \(\frac{4}{4}\) ________ 1
Answer:
\(\frac{4}{4}\) = 1
(e) \(\frac{2005}{2005}\) ________ 1
Answer:
\(\frac{2005}{2005}\) = 1

(Try These Page No: 142)
Question 1.
Are \(\frac{1}{3}\) and \(\frac{2}{7}\); \(\frac{2}{5}\) and \(\frac{2}{7}\), \(\frac{2}{9}\) and \(\frac{6}{27}\) equivalent? Give reason.
Answer:
(i) \(\frac{1}{3}\) and \(\frac{2}{7}\)
∵ 1 × 7 = 7, 3 × 2 = 6
∵ 7 ≠ 6, i.e. 1 × 7 ≠ 3 × 2
∴ \(\frac{1}{3}\) and \(\frac{2}{7}\) are not equivalent fractions.
(ii) \(\frac{2}{5}\) and \(\frac{2}{7}\)
∵ 2 × 7 = 14 and 2 × 5 = 10
∵ 14 ≠ 10, i.e. 2 × 7 ≠ 2 × 5
\(\frac{2}{5}\) and \(\frac{2}{7}\) are not equivalent fractions.
(iii) \(\frac{2}{9}\) and \(\frac{6}{27}\)
∵ 2 × 27 = 54 and 6 × 9 = 54
∵ 2 × 27 = 6 × 9 = 54
∴ \(\frac{2}{9}\) and \(\frac{6}{27}\) are equivalent fractions.
Question 2.
Give example of four equivalent fractions.
Answer:
(i) \(\frac{3}{7}\) and \(\frac{6}{14}\)
(ii) \(\frac{2}{8}\) and \(\frac{8}{32}\)
(iii) \(\frac{8}{11}\) and \(\frac{16}{22}\)
(iv) \(\frac{4}{24}\) and \(\frac{2}{12}\)

Question 3.
Identify the fractions in each. Are these fractions equivalent?
(i)

Answer:
The given figure represents the fraction \(\frac{6}{8}\).
We have \(\frac{6}{8}=\frac{6 \div 2}{8 \div 2}=\frac{3}{4}\)
(ii)

Answer:
The given figure represents the fraction \(\frac{9}{12}\)
We have \(\frac{9}{12}=\frac{9 \div 3}{12 \div 3}=\frac{3}{4}\)
(iii)

Answer:
The given figure represents the fraction \(\frac{12}{16}\)
We have \(\frac{12}{16}=\frac{12 \div 4}{16 \div 4}=\frac{3}{4}\)
(iv)

Answer:
The given figure represents the fraction \(\frac{15}{20}\).
We have \(\frac{15}{20}=\frac{15 \div 5}{20 \div 5}=\frac{3}{4}\)
∵ \(\frac{6}{8}=\frac{9}{12}=\frac{12}{16}=\frac{15}{20}\) (each \(\frac{3}{4}\))
Hence, all these fractions are equivalent.

(Try These Page No: 143)
Question 1.
Find five equivalent fractions of each of the following:
(i) \(\frac{2}{3}\)
Answer:
\(\frac{2}{3}\)
Five equivalent fractions of \(\frac{2}{3}\) are:
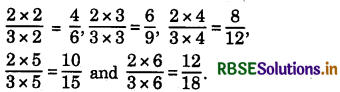
(ii) \(\frac{1}{5}\)
Answer:
\(\frac{1}{5}\)
Five equivalent fractions of \(\frac{1}{5}\) are:
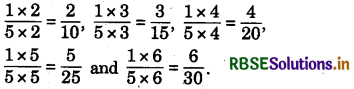
(iii) \(\frac{3}{5}\)
Answer:
\(\frac{3}{5}\)
Five equivalent fractions of \(\frac{3}{5}\) are:

(iv) \(\frac{5}{9}\)
Answer:
\(\frac{5}{9}\)
Five equivalent fractions of \(\frac{5}{9}\) are:
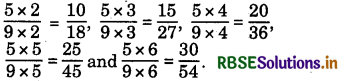

(Try These Page No: 146)
Question 1.
Write the simplest form of:
(i) \(\frac{15}{75}\)
Answer:
\(\frac{15}{75}\)
∵ HCF of 15 and 75 is 15.
∴ Now \(\frac{15}{75}=\frac{15 \div 15}{75 \div 15}=\frac{1}{5}\)
Thus simplest form of \(\frac{15}{75}\) is \(\frac{1}{5}\).
(ii) \(\frac{16}{72}\)
Answer:
\(\frac{16}{72}\)
∵ HCF of 16 and 72 is 15.
∴ Now \(\frac{16}{72}=\frac{16 \div 8}{72 \div 8}=\frac{2}{9}\)
Thus simplest form of \(\frac{16}{72}\) is \(\frac{2}{9}\).
(iii) \(\frac{17}{51}\)
Answer:
\(\frac{17}{51}\)
∵ HCF of 17 and 51 is 17.
∴ Now \(\frac{17}{51}=\frac{17 \div 17}{51 \div 17}=\frac{1}{3}\)
Thus simplest form of \(\frac{17}{51}\) is \(\frac{1}{3}\).
(iv) \(\frac{42}{28}\)
Answer:
\(\frac{42}{28}\)
∵ HCF of 42 and 28 is 14.
∴ Now \(\frac{42}{28}=\frac{42 \div 14}{28 \div 14}=\frac{3}{2}\)
Thus simplest form of \(\frac{42}{28}\) is \(\frac{3}{2}\).
(v) \(\frac{80}{24}\)
Answer:
\(\frac{80}{24}\)
∵ HCF of 24 and 80 is 8.
∴ Now \(\frac{80}{24}=\frac{80 \div 8}{24 \div 8}=\frac{10}{3}\)
Thus simplest form of \(\frac{80}{24}\) is \(\frac{10}{3}\).
Question 2.
Is \(\frac{49}{64}\) in its simplest form?
Answer:
Yes, \(\frac{49}{64}\) is in its simplest form, since HCF of 49 and 64 is 1.

(Try These Page No: 148)
Question 1.
You get one-fifth of a bottle of juice and your sister gets one-third of the same size of a bottle of juice. Who gets more?
Answer:
The quantity of juice in a bottle showed by a rectangular strip.
I get one-fifth of a bottle of juice, i.e. one part out of 5 equal parts, then we divide the rectangle into 5 equal parts and shade one part out of these to represent one-fifth.
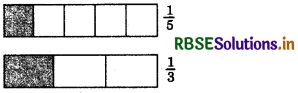
Since, \(\frac{1}{3}\) is greater than \(\frac{1}{5}\), so my sister gets
(Try These Page No: 149)
Question 1.
Which is the larger fraction?
(i) \(\frac{7}{10}\) or \(\frac{8}{10}\)
(ii) \(\frac{11}{24}\) or \(\frac{13}{24}\)
(iii) \(\frac{17}{102}\) or \(\frac{12}{102}\)
Why are these comparisons easy to mske?
Answer:
(i) \(\frac{7}{10}\) or \(\frac{8}{10}\)
∵ These are like fractions, by comparing numerators we have 7 < 8.
∴ \(\frac{7}{10}\) < \(\frac{8}{10}\)
(ii) \(\frac{11}{24}\) or \(\frac{13}{24}\)
∵ These are like fractions, by comparing numerators we have 11 < 13.
∴ \(\frac{11}{24}\) < \(\frac{13}{24}\)
(iii) \(\frac{17}{102}\) or \(\frac{12}{102}\)
∵ These are like fractions, by comparing numerators we have 17 > 12.
∴ \(\frac{17}{102}\) > \(\frac{12}{102}\)
(These comparisons are easy to make because these are like fractions.)

Question 2.
Write these in ascending and also in descending order
(a) \(\frac{1}{8}, \frac{5}{8}, \frac{3}{8}\)
Answer:
\(\frac{1}{8}, \frac{5}{8}, \frac{3}{8}\)
∵ These are like fractions, so by comparing their numerators, we have
5 > 3 > 1
∴ \(\frac{5}{8}\) > \(\frac{3}{8}\) > \(\frac{1}{8}\)
(b) \(\frac{1}{5}, \frac{11}{5}, \frac{4}{5}, \frac{3}{5}, \frac{7}{5}\)
Answer:
\(\frac{1}{5}, \frac{11}{5}, \frac{4}{5}, \frac{3}{5}, \frac{7}{5}\)
∵ These are like fractions, by comparing their numerators, we have
11 > 7 > 4 > 3 > 1
∴ \(\frac{11}{5}\) > \(\frac{7}{5}\) > \(\frac{4}{5}\) > \(\frac{3}{5}\) > \(\frac{1}{5}\)
(c) \(\frac{1}{7}, \frac{3}{7}, \frac{13}{7}, \frac{11}{7}, \frac{7}{7}\)
Answer:
\(\frac{1}{7}, \frac{3}{7}, \frac{13}{7}, \frac{11}{7}, \frac{7}{7}\)
∵ These are like fractions, by comparing their numerators, we have
13 > 11 > 7 > 3 > 1
∴ \(\frac{13}{7}\) > \(\frac{11}{7}\) > \(\frac{7}{7}\) > \(\frac{3}{7}\) > \(\frac{1}{7}\)

(Try These Page No: 151)
Question 1.
Arrange the following in ascending and descending order:
(a) \(\frac{1}{12}, \frac{1}{23}, \frac{1}{5}, \frac{1}{7}, \frac{1}{50}, \frac{1}{9}, \frac{1}{17}\)
Answer:
\(\frac{1}{12}, \frac{1}{23}, \frac{1}{5}, \frac{1}{7}, \frac{1}{50}, \frac{1}{9}, \frac{1}{17}\)
As we know that in unlike fractions having same numerator, the greater the value of the denominator, the smaller the value of the fractional number.
∵ 50, 23, 17, 12, 9, 7 and 5 are in descending order.
∴ Fractions in ascending order are:
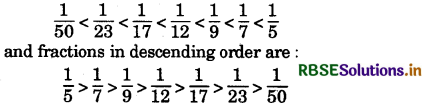
(b) \(\frac{3}{7}, \frac{3}{11}, \frac{3}{5}, \frac{3}{2}, \frac{3}{13}, \frac{3}{4}, \frac{3}{17}\)
Answer:
\(\frac{3}{7}, \frac{3}{11}, \frac{3}{5}, \frac{3}{2}, \frac{3}{13}, \frac{3}{4}, \frac{3}{17}\)
∵ 17, 13, 11, 7, 5, 4 and 2 are in descending order.
∴ Fractions in ascending order are:
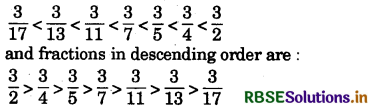
(c) Write 3 more similar example and arrange them in ascending and descending order.
Answer:
Three more examples
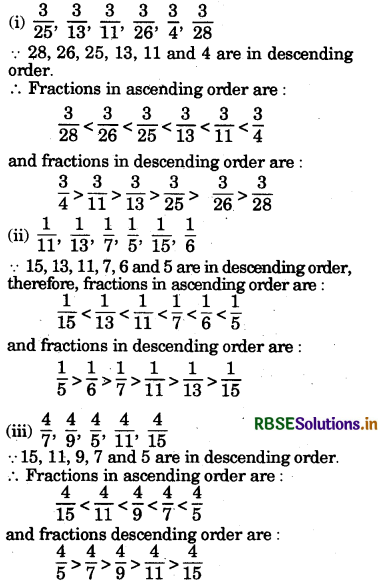

(Try These Page No: 155)
Question 1.
My mother divided an apple into 4 equal parts. She gave me two parts and my brother one part. How much apple did she give to both of us together?
Answer:
Number of equal part of an apple = 4
Parts given to me = \(\frac{2}{4}\)
Parts given to my brother = \(\frac{1}{4}\)
∴ Parts of apple given to both of us = \(\frac{2}{4}+\frac{1}{4}=\frac{3}{4}\)
Hence, my mother gave \(\frac{3}{4}\) part of apple to both of us.
Question 2.
Mother asked Neelu and her brother to pick stones from the wheat. Neelu picked one-fourth of the total stones in it and her brother also picked up one-fourth of the stones. What fraction of the stones did both pick up together?
Answer:
Parts of stones picked by Neelu = \(\frac{1}{4}\)
Parts of stones picked by her brother = \(\frac{1}{4}\)
∴ Fraction of stones picked up by both = \(\frac{1}{4}+\frac{1}{4}=\frac{1+1}{4}=\frac{2}{4}=\frac{1}{2}\)
Hence, both together picked \(\frac{1}{2}\) fraction of the stones.
Question 3.
Sohan was putting covers on his notebooks. He put one-fourth of the covers on Monday. He put another one-fourth on Tuesday and the remaining on Wednesday. What fraction of the covers did he put on Wednesday?
Answer:
Fraction of covers put on Monday = \(\frac{1}{4}\)
Fraction of covers put on Tuesday = \(\frac{1}{4}\)
There, fraction of covers put on Wednesday
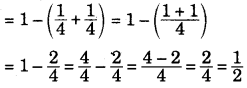
Hence, he put \(\frac{1}{2}\) fraction of covers on Wednesday.

(Try These Page No: 156)
Question 1.
Add with the help of a diagram:
(i) \(\frac{1}{8}+\frac{1}{8}\)
Answer:
In the following figure, a rectangle is divided into 8 equal parts:

(ii) \(\frac{2}{5}+\frac{3}{5}\)
Answer:
In the following figure, a rectangle is divided into 5 equal parts:
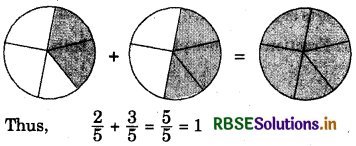
(iii) \(\frac{1}{6}+\frac{1}{6}+\frac{1}{6}\)
Answer:
In the following figure, a rectangle is divided into 6 equal parts:
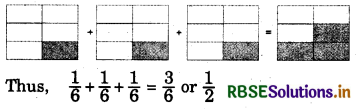

Question 2.
Add \(\frac{1}{12}+\frac{1}{12}\). How will we show this pictorially? Using paper folding?
Answer:
\(\frac{1}{12}+\frac{1}{12}\)
To show pictorially, we cut a rectangle into 12 equal parts then:
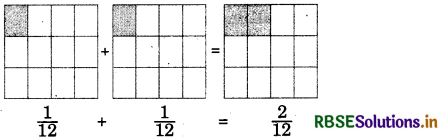
Question 3.
Make 5 more examples of problems given in 1 and 2 above.
Answer:
Solve them with your friends.
(Try These Page No: 157)
Question 1.
Find the difference between \(\frac{7}{8}\) and \(\frac{3}{8}\) is:
Answer:
The difference between \(\frac{7}{8}\) and \(\frac{3}{8}\) is
\(\frac{7}{8}-\frac{3}{8}=\frac{7-3}{8}=\frac{4}{8}=\frac{1}{2}\)
Question 2.
Mother made a gud-patti in a round shape. She divided it into 5 parts. Seema ate one piece from it. If I eat another piece then how much would be left?
Answer:
Total number of equal parts of gud-patti = 5 .
Parts eaten by Seema = \(\frac{1}{5}\)
Eaten by me = \(\frac{1}{5}\)
So, fraction of gud-patti eaten by Seema and me

Hence, \(\frac{3}{5}\) part of gud-patti would be leftover.
Question 3.
My elder sister divided the watermelon into 16 parts. I ate 7 out of them and my friend ate 4. How much did we eat between us? How much more of the watermelon did I eat than my friend? What portion of the watermelon remained?
Answer:
Total number of equal parts of watermelon = 16
Eaten by me = \(\frac{7}{16}\)
Eaten by my friend = \(\frac{4}{16}\)
Total number of parts eaten by me and my friend = \(\frac{7}{16}+\frac{4}{16}=\frac{7+4}{16}=\frac{11}{16}\) = parts
More part eaten by me than my friend = \(\frac{7}{16}-\frac{4}{16}=\frac{7-4}{16}=\frac{3}{16}\)
Hence, I ate \(\frac{3}{16}\) portion of watermelon more than my friend.
Watermelon that remained
= 1 - \(\frac{11}{16}=\frac{16-11}{16}=\frac{5}{16}\)
Hence, \(\left(\frac{5}{16}\right)^{\text {th }}\) portion of the watermelon remained.
Question 4.
Make five problems of this type and solve them with your friends.
Answer:
Do it yourself

(Try These Page No: 159)
Question 1.
Add \(\frac{2}{5}\) and \(\frac{3}{7}\).
Answer:
\(\frac{2}{5}\) + \(\frac{3}{7}\)
∵ LCM(5, 7) = 35
By converting given fractions into equivalent fraction with denominator 35, we have
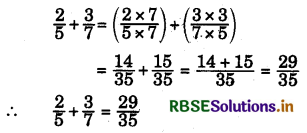
Question 2.
Subtract \(\frac{2}{5}\) from \(\frac{5}{7}\)
Answer:
\(\frac{2}{5} - \frac{5}{7}\)
∴ LCM of 7 and 5 is 35.
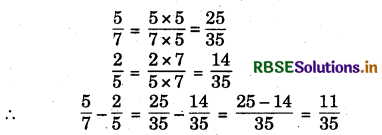

- RBSE Solutions for Class 6 Maths Chapter 2 Whole Numbers InText Questions
- RBSE Class 6 Maths Important Questions Chapter 1 अपनी संख्याओं की जानकारी
- RBSE Solutions for Class 6 Maths Chapter 7 भिन्न Intext Questions
- RBSE Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.4
- RBSE Solutions for Class 6 Maths Chapter 1 Knowing our Numbers Ex 1.1
- RBSE Solutions for Class 6 Maths Chapter 1 Knowing our Numbers InText Questions
- RBSE Solutions for Class 6 Maths in Hindi Medium & English Medium
- RBSE Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.6
- RBSE Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.5
- RBSE Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.3
- RBSE Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas InText Questions