RBSE Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.4

Rajasthan Board RBSE Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.4 Textbook Exercise Questions and Answers.

RBSE Class 6 Maths Solutions Chapter 7 Fractions Ex 7.4

Question 1. 
Write shaded portion as fraction. Arrange them in ascending and descending order using correct sign ’<', '=', ’>' between the fractions:

(c) Show \(\frac{2}{6}, \frac{4}{6}, \frac{8}{6}\) and \(\frac{6}{6}\) line. Put appropriate signs between the fractions given below:

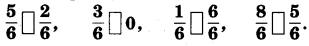
Answer:
(a) Fraction of shaded portion in fig. (i) = \(\frac{3}{8}\)
Fraction of shaded portion in fig. (ii) = \(\frac{6}{8}\)
Fraction of shaded portion in fig. (iii) = \(\frac{4}{8}\)
Fraction of shaded portion in fig. (iv) = = \(\frac{1}{8}\)
∵ These are like fractions, so we arrange them in ascending and descending order by arranging their numerators in ascending and descending order.

∴ Ascending order : \(\frac{1}{8}<\frac{3}{8}<\frac{4}{8}<\frac{6}{8}\)
Descending order: \(\frac{6}{8}>\frac{4}{8}>\frac{3}{8}>\frac{1}{8}\)

(b) Fraction of shaded portion in fig. (i) = \(\frac{8}{9}\)
Fraction of shaded portion in fig. (ii) = \(\frac{4}{9}\)
Fraction of shaded portion in fig. (iii) = \(\frac{3}{9}\)
Fraction of shaded portion in fig. (iv) = \(\frac{6}{9}\)
∵ In ascending and descending order by arranging their numerators in ascending and descending order.

∴ Ascending order : \(\frac{3}{9}<\frac{4}{9}<\frac{6}{9}<\frac{8}{9}\)
Descending order: \(\frac{3}{9}<\frac{4}{9}<\frac{6}{9}<\frac{8}{9}\)

(c) Given fraction : \(\frac{2}{6}, \frac{4}{6}, \frac{8}{6}, \frac{6}{6}\)

Now, \(\frac{5}{6}>\frac{2}{6}, \frac{3}{6}>0, \frac{1}{6}>\frac{6}{6}, \frac{8}{6}>\frac{5}{6}\)

Question 2.
Compare the fractions and put an appropriate sign:

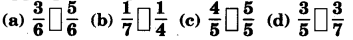
Answer:
(a) <, (b) <x (c) < (d) >.

Question 3. 
Make five more such pairs and put appropriate signs:

Answer:
(a) \(\frac{5}{9}, \frac{2}{9}\)
∵ These are like fractions, so by comparing their numerators, we have 5 > 2.
∴ \(\frac{5}{9} > \frac{2}{9}\)

(b) \(\frac{7}{15}, \frac{11}{15}\)
∵ These are like fractions, so by comparing their numerators, we have 7 < 11.
∴ \(\frac{7}{15}<\frac{11}{15}\)

(c) \(\frac{3}{7}, \frac{3}{11}\)
These are unlike fractions having same numerators so by comparing their denominators, we have 7 < 11.
∴ \(\frac{3}{7} > \frac{3}{11}\)

(d) \(\frac{7}{5}, \frac{3}{8}\)
Here, the denominators are different, so we cross multiply.
7 × 8 ___ 3 × 5
56 > 15
\(\frac{7}{5} > \frac{3}{8} \)

(e) \(\frac{1}{7}, \frac{1}{9}\)
These are unlike fractions having same numerators so by comparing their denominators, we have 7 < 9.
\(\frac{1}{7} > \frac{1}{9}\)

Question 4.
Look at the figures and write '<’ or '>' or '=' between the given pairs of fractions:

Make five more such problems and solve them with your friends.
Answer:
(a) \(\frac{1}{6}, \frac{1}{3}\)
In the given figure, \(\frac{1}{6}\) lies on the left of \(\frac{1}{3}\) so, \(\frac{1}{6} < \frac{1}{3}\)

(b) \(\frac{3}{4}, \frac{2}{6}\)
In the riven figure, \(\frac{3}{4}\) lies on the right of \(\frac{2}{6}\) so, \(\frac{3}{4} > \frac{2}{6}\)

(c) \(\frac{2}{3}, \frac{2}{4}\)
\(\frac{2}{3}\) is on the right of \(\frac{2}{4}\), therefore, \(\frac{2}{3} > \frac{2}{4}\)

(d) \(\frac{6}{6}, \frac{3}{3}\)
\(\frac{6}{6}\) and \(\frac{3}{3}\) lies at the same point, therefore, \(\frac{6}{6} = \frac{3}{3}\)

(e) \(\frac{5}{6}, \frac{5}{5}\)
\(\frac{5}{6}\) lies in the left of \(\frac{5}{5}\), therefore, \(\frac{5}{6} < \frac{5}{5}\)

Five more examples can be as given below:

Answer:
(i) \(\frac{1}{2}, \frac{1}{5}\)
\(\frac{1}{2}\) is in the right of \(\frac{1}{5}\) so \(\frac{1}{2} > \frac{1}{5}\)

(ii) \(\frac{2}{6}, \frac{3}{5}\)
\(\frac{2}{6}\) is the left of \(\frac{3}{5}\), so \(\frac{2}{6} < \frac{3}{5}\)

(iii) \(\frac{2}{4}, \frac{1}{5}\)
\(\frac{2}{4}\) is in the right of \(\frac{1}{5}\) so \(\frac{2}{4} > \frac{1}{5}\)

(iv) \(\frac{5}{6}, \frac{1}{4}\)
\(\frac{5}{6}\) is in the right of \(\frac{1}{4}\), so \(\frac{5}{6} > \frac{1}{4}\)

(iv) \(\frac{2}{2}, \frac{4}{4}\)
\(\frac{2}{2}\) and \(\frac{4}{4}\) are at the same point, so \(\frac{2}{2} = \frac{4}{4}\)

Question 5.
How quickly ean you do this? Fill appropriate sign ('<', '=', '>')

Answer:
For quickly comparison we use the cross¬multiplication method:

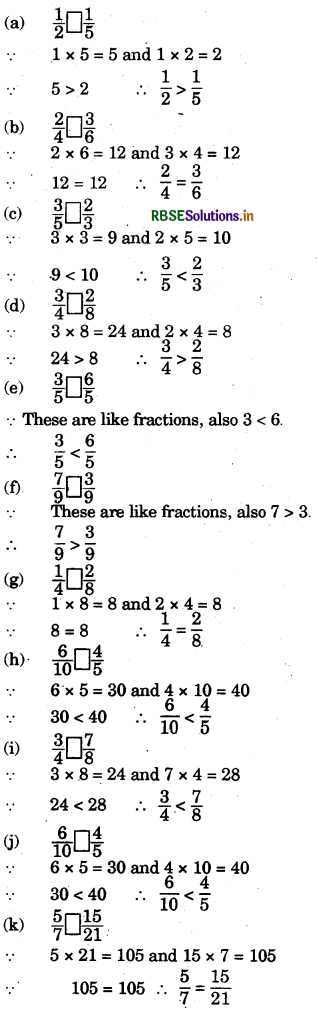
Question 6. 
The following fractions represent just three different numbers. Separate them into three groups of equivalent fractions, by changing each one to its simplest form.

Answer:

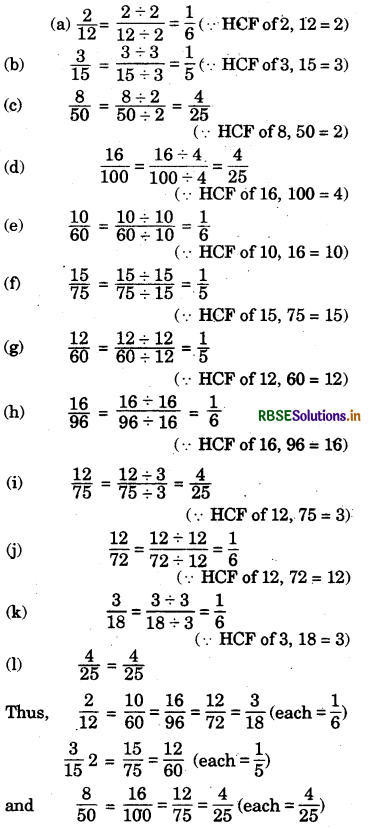
Question 7.
Find answers to the following. Write and indicate, how you solved them?
(a) Is \(\frac{5}{9}\) equal to \(\frac{4}{5}\)?
Answer:
By cross-multiplication, we have
5 × 5 = 25 and 4 × 9 = 36
∵ 25 ≠ 36
\(\frac{5}{9}\) is not equal to \(\frac{4}{5}\).

(b) Is \(\frac{9}{16}\) equal to \(\frac{5}{9}\)
Answer:
By cross-multiplication, we have
9 × 9 = 81 and 16 × 5 = 80 
81 ≠ 80
\(\frac{9}{16}\) is not equal to \(\frac{5}{9}\).

(c) Is \(\frac{4}{5}\) equal to \(\frac{16}{20}\)
Answer:
By cross-multiplication, we have
4 × 20 = 80 and 16 × 5 = 80
80 = 80
∴ \(\frac{4}{5}=\frac{16}{20}\)

(d) Is \(\frac{1}{15}\) equal to \(\frac{4}{30}\)
Answer:
By cross-multiplication, we have
1 × 30 = 30 and 15 × 4 = 60 
30 ≠ 60 
i.e. \(\frac{1}{15}\) is not equal to \(\frac{4}{30}\)

Question 8.
Ila read 25 pages of a book containing 100 pages. Lalita read \(\frac{2}{5}\) of the same book. Who read less?
Answer:
Total pages in a book = 100 pages 
Ila reads = 25 pages
Fraction of pages Ila read = \(\frac{25}{100}=\frac{1}{4}\)
Fraction of pages Lalita read = \(\frac{2}{5}\)

To compare \(\frac{1}{4}\) and \(\frac{2}{5}\).
1 × 5 = 5 and 2 × 4 = 8
5 < 8 ⇒ \(\frac{1}{4} < \frac{2}{5}\)
Thus, Ha read less.

Question 9.
Rafiq exercised for \(\frac{3}{6}\) of an hour, while Rohit exercised for \(\frac{3}{4}\) of an hour. Who exercised for a longer time?
Answer:
Rafiq exercised \(\frac{3}{6}\) of an hour.
Rohit exercised \(\frac{3}{4}\) of an hour.
Making the denominator equal,
\(\frac{3}{6}\) can be written as \(\frac{3 \times 2}{6 \times 2}=\frac{6}{12}\)
and \(\frac{3}{4}\) can be written as \(\frac{3 \times 3}{6 \times 3}=\frac{9}{12}\)
Thus, \(\frac{9}{12}>\frac{6}{12}\), So, \(\frac{3}{4}>\frac{3}{6}\)
Therefore, Rohit exercised for a longer time.

Question 10.
In a class A of 25 students, 20 passed with 60% or more marks; in another class B of 30 students, 24 passed with 60% or more marks. In which class was a greater fraction of students getting with 60% or more marks?
Answer:
∵ In class A, fraction of students passed with 60% or more marks
= \(\frac{20}{25}=\frac{20 \div 5}{25 \div 5}=\frac{4}{5}\)
In class B, fraction of students passed with 60% or more marks = \(\frac{24}{30}=\frac{24 \div 6}{30 \div 6}=\frac{4}{5}\)
So, same fraction of students passed with 60% or more marks in both the classes.