RBSE Solutions for Class 6 Maths Chapter 6 Integers InText Questions

Rajasthan Board RBSE Solutions for Class 6 Maths Chapter 6 Integers InText Questions Textbook Exercise Questions and Answers.

RBSE Class 6 Maths Solutions Chapter 6 Integers InText Questions

(Do This Page 114-115)

Question 1.
Represent the following positions with + or - sign :
(a) 8 steps to the left of zero
Answer: 
- 8

(b) 7 steps to the right of zero
Answer: 
+ 7

(c) 11 steps to the right of zero
Answer: 
+ 11

(d) 6 steps to the left of zero
Answer: 
- 6

(Do This Page No: 115)

Question 1. 
Write the successor of the following numbers: 10, 8, - 5, - 3, 0.
Answer:

Number	Successor
10	10 + 1 = 11
8	8 + 1 = 9
-5	- 5 + 1 = - 4
-3	- 3 + 1 = - 2
0	0 + 1 = 1

Question 2. 
Write the predecessor of the following numbers : 10, 8, 5, 3,0.
Answer:

Number	Predecessor
10	10 - 1 = 9
8	8 - 1 = 7
5	5 - 1 = 4
3	3 - 1 = 2
0	0 - 1 = - 1

(Try These Page No: 116)

Question 1.
Write the following numbers with appropriate signs:
(a) 100 m below sea level.
Answer:
- 100 m,

(b) 25°C above 0°C temperature.
Answer:
25°C,

(c) 15°C below 0°C temperature.
Answer:
- 15°C,

(d) Any five numbers less than 0.
Answer:
- 1, - 2, - 3, - 4, - 5

(Try These Page No: 118)

Question 1.
Mark 3, 7, - 4, - 8, - 1 and - 3 on the number line.
Answer: 
Given numbers are marked on the number line as shown below:

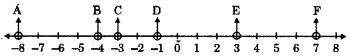
The integer - 8 is at A. The integer - 4 is at B. The integer - 3 is at C. The integer - 1 is at D. The integer 3 is at E. The integer 7 is at F.

(Try These Page No: 119)

Question 1.
Compare the following pairs of numbers using > or < :
0 _____ -8;    
Answer:
0 > - 8;    

- 1 _____ - 15
Answer:
- 1 > - 15

5 _____ -5    
Answer:
5 > - 5;    

11 _____ 15
Answer:
11 > 15

0 _____ 6
Answer:
0 < 6;    

- 20 _____ 2    
Answer:
- 20 < 2    

From the above exercise, Rohini arrived at the following conclusions:

(a) Every positive integer is larger than every negative integer.
(b) Zero is less than every positive integer.
(c) Zero is larger than every negative integer.    
(d) Zero is neither a negative integer nor a positive integer.
(e) Farther a number from zero on the right, larger is its value.
(f) Farther a number from zero on the left, smaller is its value.
Do you agree with her ? Give examples.
Answer:
(a) Yes, 5 is larger than - 5.
(b) Yes, 0 is less than 2.
(c) Yes, 0 is larger than - 2.
(d) Yes.
(e) 0,3, 6, 7, 8    
(f) - 4,- 3, - 2, - 1,0.

(Try These Page No: 125)

Question 1. 
Find the answers of the; following additions:
(a) (- 11) + (- 12) 
Answer:
(- 11) + (- 12) = - 11 - 12 = - 23

(b) (+ 10) + (+ 4)
Answer:
(+ 10) + (+ 4) = 10 + 4 = 14

(c) (- 32) + (- 25) 
Answer:
(- 32) + (- 25) = - 32 - 25 = - 57

(d) (+ 23) + (+ 40)
Answer:
(+ 23) + (+ 40) = 23 + 40 = 63

Question 2. 
Find the solution of the following:
(a) (- 7) + (+ 8)    
Answer:
(- 7) + (+ 8) = - 7 + 8 = 1

(b) (- 9) + (+ 13)
Answer:
(- 9) + (+ 13) = - 9 + 13 = 4

(c) (+ 7) + (-10)    
Answer:
(+ 7) + (- 10) = 7 - 10 = - 3

(d) (+ 12) + (- 7)
Answer:
(+ 12) + (- 7) = 12 - 7 = 5

(Try These  Page No: 127)

Question 1. 
Find the solution of the following additions using a number line :
(a) (- 2) + 6    
(b) (- 6) + 2
Make two such questions and solve them using the number line.
Answer: 
First part: (a) (- 2) + 6 
First we move 2 steps to the left of 0 to reach - 2, from here we move 6 steps to the right of - 2 to reach 4.

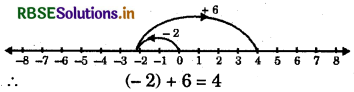
∴ (- 2) + 6 = 4

(b) (- 6) + 2
First we move 6 steps to the left of 0 to reach - 6 then we move 2 steps to the right of - 6 to reach at - 4.

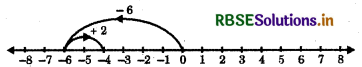
∴ (- 6) + 2 = - 4

Second part: Make two such questions and solve them using number line.
(a) Solve (- 8) + 5
First we move 8 steps to the left of 0 to reach at - 8 then we move 5 steps to the right of - 8 to reach at -3.

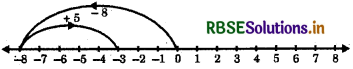
Hence, (- 8) + 5 = - 3

(b) Solve (- 5) + 7
First we move 5 steps to the left of 0 to reach at - 5 then we move 7 steps to the right of - 5 to reach at 2.

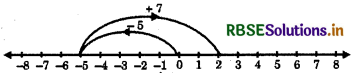
Hence, (- 5) + 7 = 2.

Question 2. 
Find the solution of the following without using number line :
(a) (+ 7) + (-11)    
(b) (-13) + (+ 10)
(c) (- 7) + (+ 9)
(d) (+ 10) + (- 5)
Make five such questions and solve them.
Answer:
(a) (+ 7) + (- 11)    
∴ (- 11) = (-    7) + (- 4)
(+ 7) + (- 11) = (+ 7) + (- 7) + (- 4) = 0 + (- 4) {∵ (+7) + (- 7) = 0} = - 4
Thus, (+ 7) + (- 11) = - 4

(b) (- 13) + (+ 10)
∵ (-13)  =  (- 10) + (- 3)
∴ (- 13) + (+ 10) = (- 10) + (- 3) + (+    10)
= (- 10) + (+ 10) + (- 3)
= 0 + (- 3) = - 3
{∵ (-10) + (+10) = 0}
Thus, (- 13) + (+ 10) = - 3

(c) (- 7) + (+ 9)
∵ (+ 9) = (+ 7) + (+ 2)    
∴ (- 7) + (+ 9) = (- 7) + (+ 7) + (+ 2)
= 0 + (+ 2) = + 2 { ∵ (- 7) + (+ 7) = 0}
Thus, (- 7) + (+ 9)= + 2

(d) (+ 10) + (- 5) 
∵ (+10) = (+ 5) + (+ 5)
∴ (+10) + (-5) = (+ 5) + (+ 5) + (- 5)    
= + 5 + 0 {∵ (+ 5) + C- 5) = 0}
= + 5

Five such questions with solution are:
(a) (+ 3) + (- 11)
(-11) = (- 9) + (- 2)
(+ 9) + (-11) = (+ 9) + (- 9) + (- 2)
= 0 + (- 2)
= - 2 {∵ (+ 9) + (- 9) = 0}

(b) (- 11) + (+ 10)
(- 11) = (- 10) + (- 1)
(- 11) + (+ 10) = (- 10) + (- 1) + (- 10)
= 0 + (- 1)
= - 1 {∵ (-10) +(+10) = 0}

(c) (- 5) + (+ 8)
(+ 8) = (+ 5) + (+ 3)
(- 5) + (+ 8) = (- 5) + (+ 5) + (+ 3)
= 0 + (+ 3) 
= + 3 {∵ (- 5) + (+ 5) = 0}

(d) (+15) + (-5)
( + 15) = (+ 10) + (+ 5)
(+ 15) + (- 5) = (+ 10) + (+ 5) + (- 5)
= (+ 10) + 0 
= + 10  { ∵ (+ 5) + (- 5) = 0}

(e) (- 4) + (+ 8)
(+ 8) = (+ 4) + (+ 4)
(- 4) + (+ 8) = (+ 4) + (+ 4) + (- 4)
= + 4 + 0 
= + 4 {∵ (+ 4) + (_ 4) = 0}