RBSE Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1

Rajasthan Board RBSE Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1 Textbook Exercise Questions and Answers.

RBSE Class 6 Maths Solutions Chapter 5 Understanding Elementary Shapes Ex 5.1

Question 1. 
What is the disadvantage in comparing line segments by mere observation?
Answer:
When we compare two line segments of almost same lengths, we cannot be sure about the length segment of greater length, there may be a chance of error due to improper viewing. It is an inappropriate method to compare line segments having a slight difference in their lengths.
 
Question 2.
Why is it better to use a divider than a ruler, while measuring the length of a line segment?
Answer:
It is better to use a divider than a ruler, because while using a ruler positioning error may occur due to incorrect positioning of the eye. Also, thickness of the ruler may cause difficulties in reading off the length.  

Question 3.
Draw any line segment, say AB. Take any point C lying in between A and B. Measure the lengths of AB, BC and AC. Is AB = AC + CB?
(Note : If A, B, C are any three points on a line such that AC + CB = AB, then we can be sure that C lies between A and B.)
Answer:
It is given that point C is lying somewhere in between A and B. Therefore, all the points A, B, C are lying on same line segment AB.
Example : Let’s take AB = 6.5 cm,
AC = 3 cm, CB = 3.5 cm

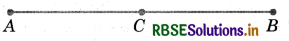
AB = AC + CB 
= 3 cm + 3.5 cm
∴ AB = 6.5 cm

Question 4.
If A,B, C are three points on a line such that AB = 5 cm, BC = 3 cm and AC = 8 cm, which one of them lies between the other two?
Answer:
Given: AB = 5 cm, BC = 3 cm, AC = 8 cm
It can be observed that AC = AB + BC
8 = 5 + 3
Point B lies in between A and C.

Question 5.
Verify, whether D is the mid-point of AG.
Answer:
Given : AD = 3 units, (4-1) units

DG = 3 units, (7 - 4) units
AG = 7 - 1 = 6 units
AD = DG
Thus, D is the mid-point of \(\overline{A G}\).

Question 6.
If B is the mid-point of \(\overline{A C}\) and C is the mid-point of \(\overline{B D}\), where A, B, C, D lie on a straight line, say why AB = CD?
Answer:

B is the mid-point of \(\overline{A C}\).
AB = BC ...................(i)
and C is the mid-point of \(\overline{B D}\).
BC = CD ..................(ii)
From (i) and (ii), we get 
AB = CD.

Question 7.
Draw five triangles and measure their sides. Check in each case, if the sum of the lengths of any two sides is always less than the third side.
Answer:

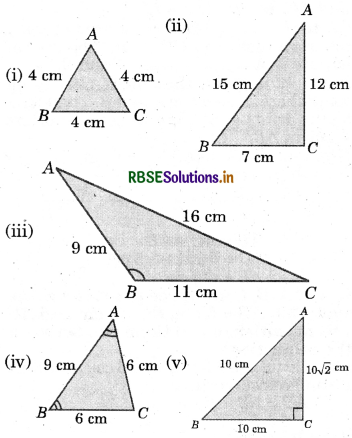
Clearly in each triangle,
AB + BC > AC 
BC + AC > AB
and AC + AB > BC
Hence, sum of two sides of a triangle is always greater than the third side.