RBSE Solutions for Class 6 Maths Chapter 11 Algebra Ex 11.5

Rajasthan Board RBSE Solutions for Class 6 Maths Chapter 11 Algebra Ex 11.5 Textbook Exercise Questions and Answers.

RBSE Class 6 Maths Solutions Chapter 11 Algebra Ex 11.5

Question 1. 
State which of the following are equations (with a variable). Give reason for your answer. Identify the variable from the equations with a variable.    
(a) 17 = x + 7
Answer: 
It is an equation of variable as both the Sides are equal. The variable is x.

(b) (t - 7) > 5
Answer: 
It is not an equation as LHS is greater than RHS.

(c) \(\frac{4}{2}\) = 2
Answer: 
It is an equation with no variable.

(d) (7 × 3) - 19 = 8
Answer: 
It is not an equation.
∵ LHS ≠ RHS. It has no variable.

(e)    5 × 4 - 8 = 2
Answer: 
It is an equation of variable as both the sides are equal, The variable is x.

(f) x - 2 = 0
Answer:
It is an equation of variable x.

(g) 2m < 30    
Answer: 
It is not an equation as LHS is less than RHS.

(h) 2n + 1 = 11
Answer: 
It is an equation as LHS is equal to RHS. 
(∴ Variable is n.) 

(i) 7 = (11 × 5) - (12 × 4)
Answer:
It is an equation with no variables as its both sides are equal.

(j) 7 = (11 × 2) + p 
Answer:
It is an equation of variables p, as both sides are equal.

(k) 20 = 5y
Answer:
It is an equation of variables y, as both sides are equal.

(l) \(\frac{3 q}{2}\) < 5    
Answer:
It is not an equation as LHS is less than RHS. 

   

(m) z + 12 > 24
Answer: 
It is not an equation as LHS is greater than RHS.    

(n)    20 - (10 - 5) = 3 × 5
Answer: 
It is an equation with no variable.

(o)    7 - x = 5
Answer: 
It is an equation of variable x, as both sides are equal.

Question 2. 
Complete the entries in the third column of the table.
Answer:

Question 3. 
Pick out the solution from the values given in the bracket next to each equation. Show that the other values do not satisfy the equation.
(a) 5m = 60    (10, 5, 12, 15)
Answer:
5m = 60
For,    m = 10
Putting the given values in LHS,
5 × 10 = 50
∵ LHS ≠ RHS
∴ m = 10 is not the solution.
For,    m    = 5
5 × 5    = 25
∵ LHS ≠ RHS
∴ m = 5 is not the solution.
For, m = 12 
5 × 12 = 60 
LHS = RHS 
∴ m    = 12 is a solution.
For, m = 15 
5 × 15 = 75 
∵ LHS ≠ RHS
∴ m = 15 is not the solution.

(b) n + 12 = 20    (12, 8, 20, 0)
Answer:
n + 12 = 20
For,    n = 12
Putting the given values in LHS,
12 + 12 = 24 
∵ LHS ≠ RHS
∵ n = 12 is    not the solution.
For,    n = 8
8 + 12 = 20 
∵ LHS = RHS
∴ n = 8 is a solution.
For,    m = 20
20 + 12 = 32 
∵ LHS ≠ RHS
∴ n = 20 is not the solution.
For,    n = 0
0 + 12 = 12 
∵ LHS ≠ RHS
∴ n = 0 is not the solution.

(c)    p - 5 = 5    (0, 10, 5, - 5)
Answer:
p - 5 = 5
For,    p = 0
Putting the given values in LHS, 
0 - 5 = - 5
∵ LHS ≠ RHS
p = 0 is not the solution.
For, p = 10 10 - 5 = 5
∵ LHS = RHS
∴ p    = 10 is a solution.
For, p = 5 
5 - 5 = 0 
∵ LHS ≠ RHS
∴ p = 5 is not the solution.
For, p = - 5    
- 5 - 5 = - 10    
∵ LHS ≠ RHS 
∴ p = - 5 is not the solution,

(d) \(\frac{q}{2}\) = 7    (7,2,10,14)
Answer:
\(\frac{q}{2}\) = 7
Putting the given values in LHS, 
For, q    = 7
\(\frac{7}{2}=\frac{7}{2}\)
∵ LHS ≠    RHS
∴ q = 7 is not the solution.
For,    q = 10
\(\frac{10}{2}\) = 5
∵ LHS ≠    RHS
∴ q = 10 is not the solution. 
For,    q = 2
\(\frac{2}{2}\) = 1
∵ LHS ≠  RHS
∴  ∵ = 2 is not the solution.
For,    ∵ = 14
\(\frac{14}{2}\) = 7
∵ LHS ≠ RHS
∴ q = 14 is a solution.

(e) r - 4 = 0    (4, - 4, 8, 0)
Answer:
r - 4 = 0
Putting the given values in LHS, 
For, r = 4 
4 - 4 = 0
∵ LHS ≠ RHS
∴ q = 4 is a solution 
For,    r =    8
8 - 4 = 4
∵ LHS ≠ RHS
∴ r = 8 is not the solution.
For, r = - 4 
- 4 - 4 = - 8
∵ LHS ≠ RHS
∴ r = - 4 is not the solution. 
For, r = 0 
0 - 4 =  4
∵ LHS ≠ RHS
∴ r = 0 is not the solution,

(f)    x + 4 = 2 (- 2, 0, 2, 4)
Answer:
x + 4 = 2
Putting the given values in LHS, 
For, x = - 2 
- 2 + 4 = 2
∵ LHS = RHS
∴ x = - 2 is a solution.
For,  x = 2
2 + 4 = 6
∵ LHS ≠ RHS
∴ x = 2 is not the solution.
For,    x = 0
0 + 4 = 4
∵ LHS ≠ RHS
∴ x = 0 is not the solution.
For,  x = 4
4 + 4 = 8
∵ LHS ≠ RHS
∴ x = 4 is not the Solution.

Question 4.
(a) Complete the table and by inspection of the table, find the solution to the equation m + 10 = 16.

Answer:

∵ At m = 6, m + 10 = 16 
∴ m = 6 is the solution.

(b) Complete the table and by inspection of the table, find the solution to the equation 5t = 35.
Answer:

Answer:

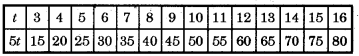
∵ At t = 7, 5t = 35
∴ t = 7 is the solution.

(c) Complete the table and find the solution of the equation z/3 = 4 using the table.

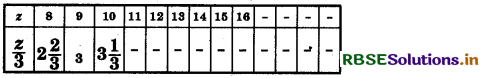
Answer:

∵ At z = 12,
\(\frac{z}{3}\) = 4
∴ z = 12 is the solution.

(d) Complete the table and find the solution of the equation m- 7 = 3.

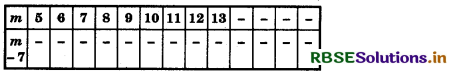
Answer:

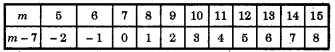
∵ At m = 10,
m - 7 = 3
∴ m = 10 is the solution.

Question 5. 
Solve the following riddles. You may yourself construct such riddles. Who am I?
(i) Go round a square Counting every corner Thrice and no more! Add the count to me To get exactly thirty-four.

Answer:
Let the required number be x.
Number of corners of the square = 4
Number obtained on counting every corner thrice = 4 × 3 = 12
According to the question,
x + 12 = 34
⇒ x = 34 - 12
⇒ x = 22
Hence, I am 22.

(ii) For each day of the week Make an upcount from me If you make no mistake  You will get twenty-three!
Answer:
Let the required number be x.
Number of days of a week = 7
According to the question,
x + 7 = 23
⇒ x = 23 - 7
⇒ x = 16
Hence, I am 16.

(iii) I am a special number Take away from me a six! A whole cricket team You will still be able to fix!

Answer:
Let the required number be x.
Number of players in cricket team = 11
According to the question,
x - 6 = 11
⇒ x = 11 + 6 = 17
Hence, I am 17.

(iv) Tell me who I am I shall give a pretty clue! You will get me back If you take me out of twenty-two! 

Answer:
Let the required number be x.
According to the question,
22 - x = x
⇒ 22 = x + x
⇒ 2x = 22
∴ x =  \(\frac{22}{2}\) = 11
Hence, I am 11.